Lagrange’s Mean Value Theorem

Last Updated : 02 Sep, 2024
Comments
Improve
Suggest changes
Like Article
Like
Save
Share
Report
News Follow

Lagrange’s Mean Value Theorem (LMVT) is a fundamental result in differential calculus, providing a formalized way to understand the behavior of differentiable functions. This theorem generalizes Rolle’s Theorem and has significant applications in various fields of engineering, physics, and applied mathematics. This article explores Lagrange’s Mean Value Theorem, its mathematical formulation, proof, and applications in engineering.

Table of Content

Lagrange’s Mean Value Theorem

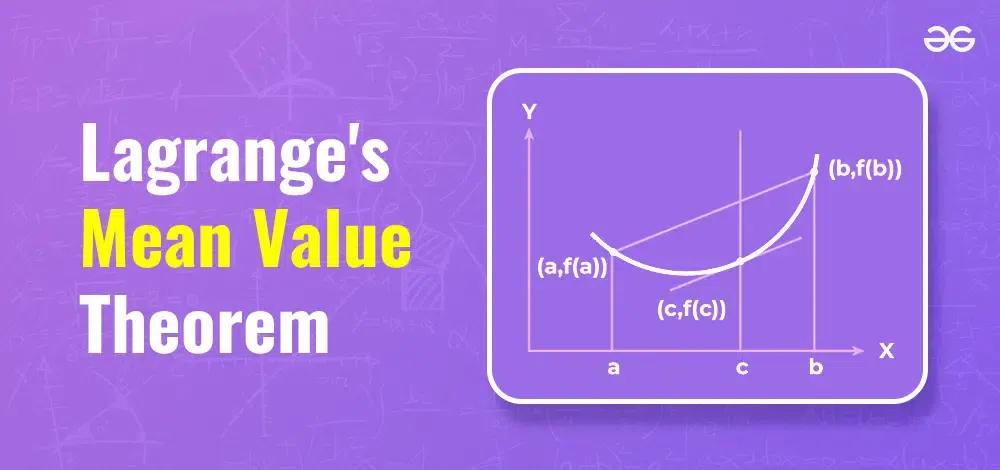
What is Lagrange’s Mean Value Theorem?

Lagrange’s Mean Value Theorem states that if a function f satisfies the following conditions on the interval [a,b]:

  1. f is continuous on [a,b]
  2. f is differentiable on (a,b)

then there exists at least one point c in (a,b) such that:

[Tex]f'(c) = \frac{f(b) – f(a)}{b – a} [/Tex]

This means that there is at least one point where the instantaneous rate of change (the derivative) of the function is equal to the average rate of change over the interval.

Proof of Lagrange’s Mean Value Theorem

To prove LMVT, consider the function f(x) and the auxiliary function g(x) defined by:

g(x) = f(x) – ([Tex]\frac{f(b) – f(a)}{b – a}[/Tex]) (x -a)

  1. The function g(x) is continuous on [a,b][a, b][a,b] and differentiable on (a,b)
  2. At x = a, g(a) = f(a).
  3. At x = b, g(b) = f(b) – ([Tex]\frac{f(b) – f(a)}{b – a}[/Tex]) (b − a) = f(b)−(f(b)−f(a)) = f(a).

Thus, g(a) = g(b), and by Rolle’s Theorem, there exists a point c ∈ (a,b) such that g′(c) = 0.

Differentiating g(x):

g′(x) = f′(x) – [Tex]\frac{f(b) – f(a)}{b – a}[/Tex]

Setting g′(c) = 0:

f′(c) = [Tex]\frac{f(b) – f(a)}{b – a}[/Tex]

Hence, the theorem is proven.

Lagrange's-mean-value-theorem

Applications of Lagrange’s Mean Value Theorem in Engineering

1. Motion Analysis

In mechanical and aerospace engineering, LMVT is used to analyze the motion of objects. By considering the displacement of an object over time, LMVT helps in understanding the object’s velocity at specific points, crucial for designing and controlling the movement of machinery and vehicles.

2. Structural Engineering

In structural engineering, LMVT assists in analyzing stress and strain distributions in materials. By applying LMVT to the deformation functions, engineers can predict points where the material experiences maximum or minimum stress, essential for ensuring structural integrity and safety.

3. Electrical Engineering

In electrical engineering, LMVT is used in the analysis of electrical circuits. For instance, when analyzing the change in voltage or current over a period, LMVT helps in identifying points where the rate of change is equivalent to the average rate, aiding in the design and optimization of circuits.

4. Fluid Dynamics

In fluid dynamics, LMVT helps in understanding the flow rate of fluids through pipes and channels. By applying LMVT to the velocity profiles of fluids, engineers can determine points where the instantaneous flow rate equals the average flow rate, which is crucial for designing efficient fluid transport systems.

5. Control Systems

In control systems engineering, LMVT is used to analyze the response of systems to various inputs. By applying LMVT to the output response of a system, engineers can identify points where the system’s response rate matches the average rate, aiding in the design and tuning of controllers.

Related Articles:

Lagrange’s Mean Value Theorem – Sample Problems

Example 1: Consider f(x) = x^2 on the interval [1, 3].

Step 1: Verify the conditions:

f(x) = x^2 is continuous on [1, 3] and differentiable on (1, 3).

Step 2: Calculate [f(b) – f(a)] / (b – a):

[f(3) – f(1)] / (3 – 1) = (9 – 1) / 2 = 4

Step 3: Find f'(x):

f'(x) = 2x

Step 4: Solve f'(c) = 4:

2c = 4

c = 2

Therefore, c = 2 satisfies the Mean Value Theorem.

Example 2: Consider f(x) = sin(x) on the interval [0, π/2].

Step 1: Verify the conditions:

sin(x) is continuous on [0, π/2] and differentiable on (0, π/2).

Step 2: Calculate [f(b) – f(a)] / (b – a):

[sin(Ï€/2) – sin(0)] / (Ï€/2 – 0) = (1 – 0) / (Ï€/2) = 2/Ï€

Step 3: Find f'(x):

f'(x) = cos(x)

Step 4: Solve cos(c) = 2/Ï€:

c = arccos(2/π) ≈ 0.6435 radians

Therefore, c ≈ 0.6435 satisfies the Mean Value Theorem.

Example 3: Consider f(x) = ln(x) on the interval [1, e].

Step 1: Verify the conditions:

ln(x) is continuous on [1, e] and differentiable on (1, e).

Step 2: Calculate [f(b) – f(a)] / (b – a):

[ln(e) – ln(1)] / (e – 1) = (1 – 0) / (e – 1) = 1/(e – 1)

Step 3: Find f'(x):

f'(x) = 1/x

Step 4: Solve 1/c = 1/(e – 1):

c = e – 1

Therefore, c = e – 1 satisfies the Mean Value Theorem

Example 4:f(x) = x^3 on the interval [0, 2]

f(x) = x^3 on the interval [0, 2]

Step 1: f(x) = x^3 is continuous on [0, 2] and differentiable on (0, 2).

Step 2: [f(2) – f(0)] / (2 – 0) = (8 – 0) / 2 = 4

Step 3: f'(x) = 3x^2

Step 4: Solve 3c^2 = 4

c = √(4/3) ≈ 1.15

Example 5: f(x) = e^x on the interval [0, 1]

Step 1: e^x is continuous on [0, 1] and differentiable on (0, 1).

Step 2: [f(1) – f(0)] / (1 – 0) = (e – 1) / 1 = e – 1

Step 3: f'(x) = e^x

Step 4: Solve e^c = e – 1

c = ln(e – 1) ≈ 0.54

Example 6: f(x) = cos(x) on the interval [0, π]

Step 1: cos(x) is continuous on [0, π] and differentiable on (0, π).

Step 2: [f(Ï€) – f(0)] / (Ï€ – 0) = (-1 – 1) / Ï€ = -2/Ï€

Step 3: f'(x) = -sin(x)

Step 4: Solve -sin(c) = -2/Ï€

c = arcsin(2/π) ≈ 0.69

Example 7: f(x) = x^2 – 3x + 2 on the interval [1, 4]

Step 1: x^2 – 3x + 2 is continuous on [1, 4] and differentiable on (1, 4).

Step 2: [f(4) – f(1)] / (4 – 1) = [(16 – 12 + 2) – (1 – 3 + 2)] / 3 = 6/3 = 2

Step 3: f'(x) = 2x – 3

Step 4: Solve 2c – 3 = 2

c = 2.5

Example 8: f(x) = √x on the interval [1, 9]

Step 1: √x is continuous on [1, 9] and differentiable on (1, 9).

Step 2: [f(9) – f(1)] / (9 – 1) = (3 – 1) / 8 = 1/4

Step 3: f'(x) = 1 / (2√x)

Step 4: Solve 1 / (2√c) = 1/4

c = 4

Example 9: f(x) = x^3 – x on the interval [-1, 2]

Step 1: x^3 – x is continuous on [-1, 2] and differentiable on (-1, 2).

Step 2: [f(2) – f(-1)] / (2 – (-1)) = [(8 – 2) – (-1 + 1)] / 3 = 6/3 = 2

Step 3: f'(x) = 3x^2 – 1

Step 4: Solve 3c^2 – 1 = 2

3c^2 = 3

c = ±1

Both c = 1 and c = -1 satisfy the theorem.

Example 10: f(x) = ln(x+1) on the interval [0, e-1]

Step 1: ln(x+1) is continuous on [0, e-1] and differentiable on (0, e-1).

Step 2: [f(e-1) – f(0)] / ((e-1) – 0) = [ln(e) – ln(1)] / (e-1) = 1 / (e-1)

Step 3: f'(x) = 1 / (x+1)

Step 4: Solve 1 / (c+1) = 1 / (e-1)

c+1 = e-1

c = e-2 ≈ 0.72

Practice Problems on Lagrange’s Mean Value Theorem

1. Find the value of c guaranteed by the Mean Value Theorem for f(x) = x2 + 2x on the interval [0, 3].

2. Verify that f(x) = x3 satisfies the conditions of the Mean Value Theorem on [-1, 2], and find all values of c that satisfy the conclusion of the theorem.

3. Determine whether there is a value of c that satisfies the Mean Value Theorem for f(x) = |x| on the interval [-2, 2].

4. Find the value of c guaranteed by the Mean Value Theorem for f(x) = sin(x) on the interval [0, π/3].

5. For f(x) = ex, find the value of c guaranteed by the Mean Value Theorem on the interval [ln 2, ln 5]

6. Verify that f(x) = 1/x satisfies the conditions of the Mean Value Theorem on [1, 4], and find the value of c that satisfies the conclusion of the theorem.

7. Find all values of c that satisfy the Mean Value Theorem for f(x) = x4 – 2x2 on the interval [-1, 1].

8. Determine whether there is a value of c that satisfies the Mean Value Theorem for f(x) = tan(x) on the interval [0, π/4].

9. For f(x) = ln(x2 + 1), find the value of c guaranteed by the Mean Value Theorem on the interval [0, 1].

10. Verify that f(x) = cos(x) satisfies the conditions of the Mean Value Theorem on [0, 2Ï€], and find all values of c in this interval that satisfy the conclusion of the theorem.

Conclusion

Lagrange’s Mean Value Theorem is a powerful tool in calculus, providing essential insights into the behavior of differentiable functions. Its applications in engineering are vast, aiding in the analysis and design of systems in motion analysis, structural engineering, electrical circuits, fluid dynamics, and control systems. Understanding and applying LMVT is crucial for solving complex engineering problems involving rates of change and function behaviors.

Lagrange’s Mean Value Theorem – FAQs

What is Lagrange’s Mean Value Theorem?

Lagrange’s Mean Value Theorem states that if a function is continuous on [a,b] and differentiable on (a,b), there exists at least one point c∈(a,b) such that f′(c) = [Tex]\frac{f(b) – f(a)}{b – a}[/Tex]

How does LMVT differ from Rolle’s Theorem?

Rolle’s Theorem is a special case of LMVT where f(a) = f(b). LMVT generalizes this to functions where f(a) ≠ f(b).

What are some practical applications of LMVT in engineering?

LMVT is used in motion analysis, structural engineering, electrical circuit analysis, fluid dynamics, and control systems to understand and design systems based on the rates of change.

How is LMVT applied in motion analysis?

In motion analysis, LMVT helps determine points where an object’s instantaneous velocity matches its average velocity over a period, aiding in the design and control of mechanical systems.

Why is LMVT important in fluid dynamics?

LMVT helps identify points in fluid flow where the instantaneous flow rate equals the average flow rate, which is crucial for designing efficient fluid transport systems.



https://media.geeksforgeeks.org/auth/avatar.png
GeeksforGeeks
News
Improve
Previous Article
Rolle's Mean Value Theorem
Next Article
Rolle's Theorem and Lagrange's Mean Value Theorem

Similar Reads

Rolle's Theorem and Lagrange's Mean Value Theorem
Rolle's Theorem and Lagrange's Mean Value Theorem: Mean Value Theorems (MVT) are the basic theorems used in mathematics. They are used to solve various types of problems in Mathematics. Mean Value Theorem is also called Lagrenges's Mean Value Theorem. Rolle’s Theorem is a subcase of the mean value theorem and they are both widely used. These theore
11 min read
Real-Life Application of Lagrange Theorem
Lagrange's theorem, an important concept in abstract algebra, has wide-ranging applications beyond math. It is crucial in cryptography for secure data transmission and aids in network analysis and error correction coding. This theorem's practical use extends to ensuring digital security and optimizing information systems, making it a vital tool in
4 min read
Mean Value Theorem
Mean Value Theorem is one of the important theorems in calculus. Mean Value Theorem states that for a curve passing through two given points there exists at least one point on the curve where the tangent is parallel to the secant passing through the two given points. Mean Value Theorem is abbreviated as MVT. This theorem was first proposed by an In
13 min read
Mean Value Theorem Practice Problems
Mean Value Theorem (MVT) is a fundamental concept in calculus which is useful in both differential and integral calculus. Mean Value Theorem guarantees the existence of at least one point where the instantaneous rate of change (derivative) of a function equals the average rate of change over a given interval. Applying the Mean Value Theorem MVT thr
6 min read
Rolle's Mean Value Theorem
Rolle's theorem one of the core theorem of calculus states that, for a differentiable function that attains equal values at two distinct points then it must have at least one fixed point somewhere between them where the first derivative of the function is zero. Rolle's Theorem and the Mean Value Theorem are fundamental results in differential calcu
8 min read
Cauchy's Mean Value Theorem
Cauchy's Mean Value theorem provides a relation between the change of two functions over a fixed interval with their derivative. Cauchy Mean Value Theorem is a special case of Lagrange Mean Value Theorem. Cauchy's Mean Value theorem is also called the Extended Mean Value Theorem or the Second Mean Value Theorem. In this article, we will learn about
8 min read
Lagrange Interpolation Formula
Lagrange Interpolation Formula finds a polynomial called Lagrange Polynomial that takes on certain values at an arbitrary point. It is an nth-degree polynomial expression of the function f(x). The interpolation method is used to find the new data points within the range of a discrete set of known data points. In this article, we will learn about, L
7 min read
Lagrange Multipliers
In mathematics, a Lagrange multiplier is a potent tool for optimization problems and is applied especially in the cases of constraints. Named after the Italian-French mathematician Joseph-Louis Lagrange, the method provides a strategy to find maximum or minimum values of a function along one or more constraints. It is used in problems of optimizati
7 min read
Four Color Theorem and Kuratowski’s Theorem in Discrete Mathematics
The Four Color Theorem and Kuratowski's Theorem are two fundamental results in discrete mathematics, specifically in the field of graph theory. Both theorems address the properties of planar graphs but from different perspectives. In this article, we will understand about Four Color Theorem and Kuratowski’s Theorem in Discrete Mathematics, their de
14 min read
Arithmetic Mean vs Geometric Mean
Arithmetic mean is arithmetic average of numbers, while geometric mean is root of nth product of numbers. The article explains the difference between arithmetic mean and geometric mean, which are expressed in their respective formulas. It also discusses the applications of these means, their sensitivity to outliers, and the suitability of a particu
6 min read
Extreme Value Theorem - Formula, Examples, Proof, Statement
Extreme value theorem proves the existence of the maximum and minimum value of the function if the function is continuous in the closed interval [a, b]. In this article, we will discuss the extreme value theorem in depth along with the extreme value theorem statement, extreme value theorem proof and how to use the extreme value theorem. We will als
8 min read
Intermediate Value Theorem
Intermediate Value Theorem is a theorem in calculus which defines an important property of continuous functions. It is abbreviated as IVT. The theorem is quite intuitive one but provides a significant result for the interpretation of the behavior of functions its says that any continuous function that takes on two values must also take on any value
9 min read
How to use DeMoivre's Theorem to find the value of i√3?
A complex number can be called a summation of both real and imaginary numbers written or represented usually in the form of z = a + ib, where i (iota) is the imaginary component and represents √(-1). Complex numbers are commonly depicted in their rectangular or standard form as a + ib. For example, 69 + 25i is a complex number where 100 is the real
4 min read
How to Calculate the Mean or Expected Value of a Discrete Random Variable
WMean or Expected Value of a Discrete random variable 'X' is calculated by multiplying each value of the random variable with its probability and adding them. The resultant value gives the mean or expected value of a given discrete random variable. In this article, we will explore the expected value, mean formula, and steps to find the expected val
5 min read
Class 12 RD Sharma Solutions - Chapter 15 Mean Value Theorems - Exercise 15.1
In Class 12 mathematics, the concept of Mean Value Theorems plays a crucial role in understanding the behavior of functions and their derivatives. This chapter explores various theorems that provide the important insights into how functions change and behave over an interval. Exercise 15.1 of Chapter 15 specifically focuses on applying these theore
15+ min read
Class 12 RD Sharma Solutions - Chapter 15 Mean Value Theorems - Exercise 15.2
Question 1 (i). Verify Lagrange's mean value theorem for the following function on the indicated interval. In each case find a point 'c' in the indicated interval as stated by Lagrange's mean value theorem f(x) = x2 - 1 on [2, 3]Solution: Given that f(x) = x2 - 1 Since the given f(x) = x2 - 1 is a polynomial function. So, the given f(x) is continuo
15+ min read
Advanced master theorem for divide and conquer recurrences
The Master Theorem is a tool used to solve recurrence relations that arise in the analysis of divide-and-conquer algorithms. The Master Theorem provides a systematic way of solving recurrence relations of the form: T(n) = aT(n/b) + f(n) where a, b, and f(n) are positive functions and n is the size of the problem. The Master Theorem provides conditi
5 min read
Consensus Theorem in Digital Logic
Prerequisite - Properties of Boolean algebra, Minimization of Boolean Functions Redundancy theorem is used as a Boolean algebra trick in Digital Electronics. It is also known as Consensus Theorem: AB + A'C + BC = AB + A'C The consensus or resolvent of the terms AB and A'C is BC. It is the conjunction of all the unique literals of the terms, excludi
3 min read
Arden's Theorem in Theory of Computation
Arden's theorem state that: "If P and Q are two regular expressions over "∑", and if P does not contain "∈" , then the following equation in R given by R = Q + RP has a unique solution i.e., R = QP*." That means, whenever we get any equation in the form of R = Q + RP, then we can directly replace it with R = QP*. So, here we will first prove that R
4 min read
Theorem - The lengths of tangents drawn from an external point to a circle are equal - Circles | Class 10 Maths
Tangent is a straight line drawn from an external point that touches a circle at exactly one point on the circumference of the circle. There can be an infinite number of tangents of a circle. These tangents follow certain properties that can be used as identities to perform mathematical computations on circles. Here, in this article, we will learn
5 min read
Kleene's Theorem in TOC | Part-1
A language is said to be regular if it can be represented by using Finite Automata or if a Regular Expression can be generated for it. This definition leads us to the general definition that; For every Regular Expression corresponding to the language, a Finite Automata can be generated. For certain expressions like:- (a+b), ab, (a+b)*; It's fairly
4 min read
Theorem - The tangent at any point of a circle is perpendicular to the radius through the point of contact - Circles | Class 10 Maths
Tangent is a straight line drawn from an external point that touches a circle at exactly one point on the circumference of the circle. There can be an infinite number of tangents of a circle. These tangents follow certain properties that can be used as identities to perform mathematical computations on circles. Here, in this article, we will learn
3 min read
Multiplication Theorem
Probability refers to the extent of the occurrence of events. When an event occurs like throwing a ball, picking a card from the deck, etc ., then there must be some probability associated with that event. In terms of mathematics, probability refers to the ratio of wanted outcomes to the total number of possible outcomes. The probability of simulta
6 min read
Theorem - There is one and only one circle passing through three given non-collinear points | Class 9 Maths
Theorem Statement: There is one and only one circle passing through three given non-collinear points. Required Diagram: Given: Three non-collinear points P, Q, and R. To Prove: There is one and only one circle passing through P, Q, and R. Construction: Join PQ and QR. Draw perpendicular bisectors AL and BM of PQ and RQ respectively. Since P, Q, R i
3 min read
Understanding Markov's theorem with example
In this article, we will discuss the overview of the Markov theorem and also will discuss the expression of the Markov theorem, and finally concluded with an example to understand the Markov theorem. Let's discuss it one by one. Markov's Theorem : Markov theorem states that if R is a non-negative (means greater than or equal to 0) random variable t
2 min read
Class 11 NCERT Solutions - Chapter 8 Binomial Theorem - Exercise 8.2
Question 1. Find the coefficient of x5 in (x+3)8 Solution: The (r+1)th term of (x+3)8 is given by Tr+1 = 8Cr(x)8-r(3)r (eq1). Therefore for x5 we need to get 8-r =5 (Because we need to find x5. Therefore, power ox must be equal to 5) So we get r=3. Now, put r=3 in eq1. We get, Coefficient of x5 = 8C3(x)5(3)3 = 8!*33/(4!*4!) = 1512 Coefficient of x5
5 min read
Class 11 RD Sharma Solution - Chapter 18 Binomial Theorem- Exercise 18.2 | Set 1
Question 1. Find the 11th term from the beginning and the 11th term from the end in the expansion of (2x – 1/x2)25. Solution: We are given, (2x – 1/x2)25. The given expression contains 25 + 1 = 26 terms. So, the 11th term from the end is the (26 − 11 + 1) th term = 16th term from the beginning. Hence, T16 = T15+1 = 25C15 (2x)25-15 (−1/x2)15 = 25C15
9 min read
Class 11 RD Sharma Solutions - Chapter 18 Binomial Theorem- Exercise 18.2 | Set 3
Question 27. If the 3rd, 4th, 5th and 6th terms in the expansion of (x + α)n be respectively a, b, c, and d, prove that [Tex]\frac{b^2-ac}{c^2-bd}=\frac{5a}{3c} [/Tex]. Solution: We are given, (x + α)n So, T3 = a = nC2 xn-2 α2 T4 = b = nC3 xn-3 α3 T5 = c = nC4 xn-4 α4 T6 = d = nC5 xn-5 α5 We need to prove that, [Tex]\frac{b^2-ac}{c^2-bd}=\frac{5a}{
8 min read
Class 11 RD Sharma Solutions- Chapter 18 Binomial Theorem - Exercise 18.1
Question 1. Using binomial theorem, write down the expressions of the following:(i) (2x + 3y)5 Solution: Using binomial theorem, we have, (2x + 3y)5 = 5C0 (2x)5 (3y)0 + 5C1 (2x)4 (3y)1 + 5C2 (2x)3 (3y)2 + 5C3 (2x)2 (3y)3 + 5C4 (2x)1 (3y)4 + 5C5 (2x)0 (3y)5 = 32x5 + 5 (16x4) (3y) + 10 (8x3) (9y)2 + 10 (4x)2 (27y)3 + 5 (2x) (81y4) + 243 y5 = 32x5 + 2
15 min read
Finding Derivative with Fundamental Theorem of Calculus
Integrals are the reverse process of differentiation. They are also called anti-derivatives and are used to find the areas and volumes of the arbitrary shapes for which there are no formulas available to us. Indefinite integrals simply calculate the anti-derivative of the function, while the definite integrals have limits and usually denote the are
5 min read
Article Tags :