Trigonometry Formulas
Trigonometry Formulas
Uploaded by
Viviana Rios GuerreroCopyright:
Available Formats
Trigonometry Formulas
Trigonometry Formulas
Uploaded by
Viviana Rios GuerreroOriginal Title
Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
Trigonometry Formulas
Trigonometry Formulas
Uploaded by
Viviana Rios GuerreroCopyright:
Available Formats
TRIGONOMETRY FORMULAS
cos 2 ( x) sin 2 ( x) 1 1 tan 2 ( x) sec 2 ( x ) cot 2 ( x ) 1 csc 2 ( x)
cos( x �y ) cos( x ) cos( y ) msin( x )sin( y ) tan( x) tan( y )
tan( x y )
sin( x �y ) sin( x) cos( y ) �cos( x) sin( y ) 1 tan( x) tan( y )
sin(2 x) 2 sin( x) cos( x)
cos 2 ( x) sin 2 ( x)
c 2 a 2 b 2 2ab cos(C )
cos(2 x) 2 cos 2 ( x) 1 sin( A) sin( B) sin(C )
1 2 sin 2
( x) a b c
2 tan( x)
tan(2 x)
1 tan 2 ( x)
1 cos(2 x) x 1 cos( x)
sin 2 ( x) cos
2 2
2
1 cos(2 x) x 1 cos( x )
cos 2 ( x) sin
2 2 2
1 cos( 2 x)
tan 2 ( x) x 1 cos( x)
1 cos(2 x) tan
2 1 cos( x)
sin( x) sin( y ) 12 [cos x y cos( x y )]
cos( x) cos( y ) 12 [cos x y cos( x y )]
sin( x) cos( y ) 12 [sin x y sin( x y )]
cos( x) sin( y ) 12 [sin x y sin( x y )]
x y x y
sin( x) sin( y ) 2 sin cos
2 2
x y x y
sin( x) sin( y ) 2 sin cos
2 2
x y x y
cos( x) cos( y ) 2 cos cos
2 2
x y x y
cos( x) cos( y ) 2 sin sin
2 2
FORMULAS, EXAM III, MAC 1147, Fall 2006 1
You might also like
- Burkard Rainer E., Dell'Amico Mauro, Martello Silvano - Assignment Problems (2009, Wiley)Document402 pagesBurkard Rainer E., Dell'Amico Mauro, Martello Silvano - Assignment Problems (2009, Wiley)Max Neves100% (3)
- Chuyên Đề Lượng Giác Hệ Thức Cơ Bản Công Thức Biến Đổi Tích Thành TổngDocument2 pagesChuyên Đề Lượng Giác Hệ Thức Cơ Bản Công Thức Biến Đổi Tích Thành TổngHuy Thịnh NguyễnNo ratings yet
- D6-2-A1-May 2024-Trigonometry.Document3 pagesD6-2-A1-May 2024-Trigonometry.Azel mbeweNo ratings yet
- Chapter 2Document8 pagesChapter 2novabbz7No ratings yet
- 12 Indefinite Integration (II) : DiscussionDocument32 pages12 Indefinite Integration (II) : DiscussionWong TonyNo ratings yet
- Science Data BookletDocument581 pagesScience Data BookletManjunath.RNo ratings yet
- Trigonometric Identities Basic Integrals of Elementary FunctionsDocument4 pagesTrigonometric Identities Basic Integrals of Elementary FunctionsCynthia ManurungNo ratings yet
- Maths Formulas TrigonometryDocument3 pagesMaths Formulas Trigonometryrajkumarbhai400No ratings yet
- Luong GiacDocument8 pagesLuong GiacNguyễn Bùi HậuNo ratings yet
- Trigonometry Cheat SheetDocument4 pagesTrigonometry Cheat SheetVaishnav VIPANCHIKANo ratings yet
- Precalc Identities TriglawsDocument2 pagesPrecalc Identities TriglawsJulio Alejo Ruiz0% (1)
- Vector and Calculus - DPP 06 (Of Lec 08) - Arjuna JEE Fastrack 2024Document2 pagesVector and Calculus - DPP 06 (Of Lec 08) - Arjuna JEE Fastrack 2024Arjun AlbenkarNo ratings yet
- Trigonometric Functions of Acute AnglesDocument16 pagesTrigonometric Functions of Acute Anglesbyunbacooon456No ratings yet
- Differential EquationDocument4 pagesDifferential EquationUtkarsh GuptaNo ratings yet
- M14 - Indefinite IntegrationDocument24 pagesM14 - Indefinite IntegrationBhawna SharmaNo ratings yet
- Ri 2023 Math TP SolnDocument17 pagesRi 2023 Math TP SolnVenkateswaran Gayathri (Bbss)No ratings yet
- 15.trigonometry Password RemovedDocument18 pages15.trigonometry Password RemovedfameNo ratings yet
- CPP - Indefinite IntegrationDocument13 pagesCPP - Indefinite Integrationcjoshi1710No ratings yet
- Calculus 1 FormulaDocument2 pagesCalculus 1 FormulaAuto CadNo ratings yet
- Ahformulae 1Document5 pagesAhformulae 1ImmortalsmNo ratings yet
- (Maa 5.20) Integration by Parts - SolutionsDocument6 pages(Maa 5.20) Integration by Parts - Solutionsdhruvkhera2No ratings yet
- Integration FormulaDocument5 pagesIntegration Formularedowanahmed116No ratings yet
- Trigonometry (EX-1)Document18 pagesTrigonometry (EX-1)prasannachaganamNo ratings yet
- 01-Indefinite Integration PDFDocument11 pages01-Indefinite Integration PDFShubhankar SinhaNo ratings yet
- Practice 3Document1 pagePractice 3menam8722No ratings yet
- Calculus (Diff) Note 02Document11 pagesCalculus (Diff) Note 02amy.like.cooking.77No ratings yet
- Formula List Math 1Document2 pagesFormula List Math 1IntanNo ratings yet
- 2.4 Differentiation of Trigonometric FunctionsDocument16 pages2.4 Differentiation of Trigonometric FunctionsAhmad SolihuddinNo ratings yet
- Math2041 Worksheet I and IIDocument2 pagesMath2041 Worksheet I and IIhabtebayu086No ratings yet
- HCI H2 2021 Prelim P1 SolutionsDocument15 pagesHCI H2 2021 Prelim P1 SolutionsVivienNo ratings yet
- EC8553 - Discrete Time Signal Processing (Ripped From Amazon Kindle Ebooks by Sai Seena)Document736 pagesEC8553 - Discrete Time Signal Processing (Ripped From Amazon Kindle Ebooks by Sai Seena)aashok52505No ratings yet
- Chapter - 7 - Integrals & Definite IntegralDocument5 pagesChapter - 7 - Integrals & Definite Integraljaiswalbebo024No ratings yet
- Problem33 61Document1 pageProblem33 61IENCSNo ratings yet
- Fourier Series PresentationDocument22 pagesFourier Series PresentationÊãglê Ēyês100% (1)
- K03052 - 20200424155345 - 7.3 Trigo SubsDocument32 pagesK03052 - 20200424155345 - 7.3 Trigo Subshariz syazwanNo ratings yet
- Trig l1 SolDocument17 pagesTrig l1 SolmangareaderandlightnovelsNo ratings yet
- JEE Differentiation McqsDocument41 pagesJEE Differentiation Mcqsharshabhvani15009No ratings yet
- 4 Determinants: Level-IIDocument27 pages4 Determinants: Level-IIBaljeet Singh100% (1)
- Integral Calculus - Practice SheetDocument4 pagesIntegral Calculus - Practice Sheetsamarahwatson02No ratings yet
- Class - Xii (Pre - Board) Term - I: General InstructionsDocument5 pagesClass - Xii (Pre - Board) Term - I: General InstructionsBamolians FamilyNo ratings yet
- Guc 2 61 38781 2023-11-25T16 29 33Document8 pagesGuc 2 61 38781 2023-11-25T16 29 33joeplays451No ratings yet
- Integ Module 3Document9 pagesInteg Module 3jkperochoNo ratings yet
- 32.inverse TrigonometricDocument8 pages32.inverse TrigonometricPrietesh PatilNo ratings yet
- MTH501 Assignment No.1Document4 pagesMTH501 Assignment No.1Umar KhanNo ratings yet
- MA1511 CheatsheetDocument2 pagesMA1511 CheatsheetbobNo ratings yet
- Math12-1 - Lesson 7 - Trigonometric IdentitiesDocument15 pagesMath12-1 - Lesson 7 - Trigonometric IdentitiesKobe MartinezNo ratings yet
- Indefinite Integration - QuestionDocument4 pagesIndefinite Integration - Questionbest badmintonNo ratings yet
- A2 Level Trigonometry Revision: Three Further Trig RatiosDocument7 pagesA2 Level Trigonometry Revision: Three Further Trig RatiosLauren ChikwehwaNo ratings yet
- Formulae SheetDocument1 pageFormulae Sheetrorosoto1No ratings yet
- Test2 - 20182019 SchemeDocument11 pagesTest2 - 20182019 SchemenorsyahirahafiqahNo ratings yet
- Class-12 DifferentitationDocument6 pagesClass-12 DifferentitationchithrakshrubanvtNo ratings yet
- Differentiation Question FinalDocument19 pagesDifferentiation Question FinalAnubhav vaishNo ratings yet
- Formula CompilationDocument6 pagesFormula CompilationKirstie Alley San JoseNo ratings yet
- 2021fall Test1Document1 page2021fall Test1ellessewongNo ratings yet
- Rvhs 2023 j2 H2ma Test 1 4b Soln (STD)Document7 pagesRvhs 2023 j2 H2ma Test 1 4b Soln (STD)Venkateswaran Gayathri (Bbss)No ratings yet
- Indefinite Integration (ADV) QPDocument7 pagesIndefinite Integration (ADV) QPAthang MalgundkarNo ratings yet
- Calculus - Trigonometric FunctionsDocument2 pagesCalculus - Trigonometric FunctionsThilini PereraNo ratings yet
- De Moiver's Theorem (Trigonometry) Mathematics Question BankFrom EverandDe Moiver's Theorem (Trigonometry) Mathematics Question BankNo ratings yet
- Trigonometric Ratios to Transformations (Trigonometry) Mathematics E-Book For Public ExamsFrom EverandTrigonometric Ratios to Transformations (Trigonometry) Mathematics E-Book For Public ExamsRating: 5 out of 5 stars5/5 (1)
- Factoring and Algebra - A Selection of Classic Mathematical Articles Containing Examples and Exercises on the Subject of Algebra (Mathematics Series)From EverandFactoring and Algebra - A Selection of Classic Mathematical Articles Containing Examples and Exercises on the Subject of Algebra (Mathematics Series)No ratings yet
- Cbse-Doe SQP 2022-23-24Document194 pagesCbse-Doe SQP 2022-23-24vebbin00No ratings yet
- Unit 4 Exam ReviewDocument4 pagesUnit 4 Exam ReviewChloe MathewsonNo ratings yet
- L 06 Hankel TransformDocument11 pagesL 06 Hankel TransformimtiazNo ratings yet
- JarnickiDocument147 pagesJarnickifelipeplatziNo ratings yet
- Enlargement WorksheetDocument10 pagesEnlargement WorksheetAmjadNo ratings yet
- Assignment MAT102 2023-24Document3 pagesAssignment MAT102 2023-24raviiinagar1212No ratings yet
- Revised Tetrad Manual-JUNE-2017 PDFDocument103 pagesRevised Tetrad Manual-JUNE-2017 PDFLuisaFernandaCastañedaNo ratings yet
- Class Notes For InterpolationDocument21 pagesClass Notes For InterpolationAryan BhattaraiNo ratings yet
- Discrete Maths PracticesDocument16 pagesDiscrete Maths PracticesmakiicosplaysNo ratings yet
- Module 7: Discrete State Space Models: Lecture Note 4Document7 pagesModule 7: Discrete State Space Models: Lecture Note 4deepak1133No ratings yet
- Antilinear OperatorsDocument12 pagesAntilinear OperatorsjofoltssonNo ratings yet
- 1 5 Hong Kong Baptist University SEMESTER 1 EXAMINATION, 1999-2000Document5 pages1 5 Hong Kong Baptist University SEMESTER 1 EXAMINATION, 1999-2000KudzaiNo ratings yet
- 20CSE027 Shakil AhmedDocument11 pages20CSE027 Shakil Ahmedzahidul.cse7.buNo ratings yet
- TreesDocument6 pagesTreesRajendranbehappyNo ratings yet
- ALP Solutions Application of Derivatives Maths Eng JEE AlDocument17 pagesALP Solutions Application of Derivatives Maths Eng JEE Alvejoshi21699No ratings yet
- Dawson College Mathematics Department 2.6-The Definition of Derivatives and The Tangent LineDocument5 pagesDawson College Mathematics Department 2.6-The Definition of Derivatives and The Tangent LineSophie L.No ratings yet
- Algebra AssignmentDocument46 pagesAlgebra AssignmentDaRo SimNo ratings yet
- PRINT3 - Limits Cheat Sheet - SymbolabDocument2 pagesPRINT3 - Limits Cheat Sheet - SymbolabPamela RicaforteNo ratings yet
- Lax, Peter D. Change of Variables in Multiple Integrals. II. Amer. Math. Monthly 108 (2001)Document6 pagesLax, Peter D. Change of Variables in Multiple Integrals. II. Amer. Math. Monthly 108 (2001)jaburicoNo ratings yet
- Merger Graph ChartDocument27 pagesMerger Graph ChartBharadwaja PisupatiNo ratings yet
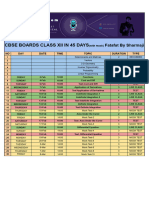
- FINAL SCHEDULE - XII 45 Days With MockDocument1 pageFINAL SCHEDULE - XII 45 Days With MockVaibhav SharmaNo ratings yet
- Differentiation QuestionsDocument5 pagesDifferentiation Questionssujathar870No ratings yet
- Elements of Fourier AnalysisDocument50 pagesElements of Fourier AnalysisNaledi xuluNo ratings yet
- Sanet - ST - Algebraic Analysis of Social NetworksDocument419 pagesSanet - ST - Algebraic Analysis of Social NetworksMarta TeofilovaNo ratings yet
- FEM Assignment 3Document11 pagesFEM Assignment 3mulualem100% (1)
- (Carus Mathematical Monographs 28) John P. D'Angelo - Inequalities From Complex Analysis-The Mathematical Association of America (2002)Document284 pages(Carus Mathematical Monographs 28) John P. D'Angelo - Inequalities From Complex Analysis-The Mathematical Association of America (2002)Cecilio Sánchez FernándezNo ratings yet
- Cut SetDocument6 pagesCut SetRashmi ShankarNo ratings yet
- 1 Matrices Booklet PDFDocument32 pages1 Matrices Booklet PDFRebeccaPrivitera100% (1)
- Lambert W FunctionDocument8 pagesLambert W FunctionSmurf 01No ratings yet