Downloaded from http://rspa.royalsocietypublishing.

org/ on November 7, 2016

Proc. R. Soc. A (2006) 462, 2017–2038

doi:10.1098/rspa.2006.1668
Published online 21 February 2006

Enhancement of mass transport and

separation of species by oscillatory
electroosmotic flows
B Y H SIN -F U H UANG AND C HUN -L IANG L AI *
Department of Mechanical Engineering, National Taiwan University,
Taipei 10617, Taiwan, Republic of China

Mass transport driven by oscillatory electroosmotic flows (EOF) in a two-dimensional

micro-channel is studied theoretically. The results indicate that the velocity and
concentration distributions across the channel-width become more and more non-
uniform as the Womersley number W, or the oscillation frequency, increases. It is also
revealed that, with a constant tidal displacement, the total mass transport rate increases
with the Womersley number W due to both the stronger convective and the transverse
dispersion effects. The total mass transport rate also increases with the tidal
displacement (with a fixed oscillation frequency) because of the associated stronger
convective effects. The cross-over phenomenon of the mass transport rates for different
species becomes possible with sufficiently large Debye lengths and at sufficiently large
values of W. Consequently, with proper choices of the Debye length, oscillation
frequency and tidal displacement, oscillatory EOF may become a good candidate for the
first-step separation of the mass species.
Keywords: electrical double layers; oscillatory electroosmotic flow;
mass transport enhancement; cross-over phenomenon; separation of species

1. Introduction

Biochips are the essential elements of bioengineering designs and are often
applied in drug diagnosis, tumour cell analyses, DNA sequencing systems and
biological/environmental-monitoring sensors. Because of this important role in
its own discipline, the technical integration and the design of ‘Lab-on-a-Chip’
devices become a major research interest both academically and industrially.
Micro-fluidic components such as micro-channels, micro-mixers, micro-pumps
and micro-reaction-chambers are commonly implemented in biochip designs. In
order to build biochips with high efficiency and quality, the physical factors and
phenomena that will affect the performance of the micro-fluidic components are
needed to be studied carefully and thoroughly. For example, electrokinetic (EK)
effects, originated from the presence of the electrical double layer (EDL) at the
contact interface of the working liquid and the solid substrate, are long discussed
in literature (Hunter 1981; Probstein 1994; Karniadakis & Beskok 2002), and are
* Author for correspondence (cllai@ntu.edu.tw).

Received 27 December 2005

Accepted 6 January 2006 2017 q 2006 The Royal Society
 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2018 H.-F. Huang and C.-L. Lai

Table 1. Nomenclature.

species concentration field (mol mK3) c

convective part of the concentration field (mol mK3) cu
dimensionless convective part of the concentration field cu!
diffusion Peclet number PeD
effective mass flux, or the time and space-averaged mass-transfer (mol mK2 sK1) Qx
dimensionless time and space-averaged mass transfer Qx!
Schmidt number Sc
x-velocity component (m sK1) ux
dimensionless x-velocity component ux!
Helmholtz–Smoluchowski (slip) velocity (m sK1) UHS
Womersley number W
(averaged) peak-to-peak oscillation amplitude (m) Dx 0
Lagrangian displacement (m) a
tidal displacement (m) b
Debye length (m) lD
micro-channel width to Debye length ratio l!
charge density due to the electrical double layer potential (C mK3) re
oscillation time period (s) t
angular frequency of the oscillation (rad sK1) u
electrical double layer potential (V) j
dimensionless electrical double layer potential j!

usually referred as a promising candidate to be applied in micro-fluidic devices.

EK effects are recommended because of the great potential in designing and
building the devices with non-moving parts so that flow control with a higher
working reliability can be achieved (Dutta & Beskok 2001a; Karniadakis &
Beskok 2002).
EDLs are formed at the solid–liquid interfaces when electrolytes or liquids of
aqueous nature, such as water, are brought into contact with the solid surfaces
which possess electro-static charges. The counter-ions (ions that possess opposite
charges to those on the solid surface) in the liquid are, thus attracted and the co-
ions are then repelled by the solid surfaces. Since the counter-ions at the very
vicinity of the charged-wall are actually adhered to the wall, they are motionless
when the liquid has relative motion to the solid surface. This non-mobile layer is
called the Stern layer, and the characteristic electrical potential of this layer is
regarded as the Zeta potential denoted by z. Beyond the Stern layer, counter-
ions are relatively free to move about in a diffusive manner, however, most of the
counter-ions are confined within a region near the boundary, which is determined
by the balance between electrical forces and thermal motion, namely, the
Brownian motion. The Debye length, lD, is thus defined by the above energy
balance and depicts how far most of the counter-ions can diffuse into the bulk
liquid away from the boundary (table 1).
When an external electrical field is applied parallel to the solid surface, the co-
and counter-ions will be attracted towards the anode and cathode, respectively;
liquid particles in the neighbourhood of the ions will then be dragged and
accelerated by the migrating ions. Net flow motion will, thus be observed for the
amount of counter-ions is excessive to that of the co-ions near the solid walls in

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2019

the presence of the EDL. This phenomenon is called ‘electroosmosis,’ which was
first observed by Reuss who performed a series of experiments on EK effects in
1809 (Probstein 1994). The resulting flow is called the electroosmotic flow (EOF)
with a shear layer formed between the motionless Stern layer and the electric-
active diffusive region. Many novel studies about EOF have been carried out
recently. For instance, laminar oscillatory EOFs were described and discussed in
detail by Dutta & Beskok (2001b) and Erickson & Li (2003); unstable oscillatory
EOFs and their application to the design of high efficiency micro-mixers were
presented by Oddy et al. (2001); in later studies performed by Lin et al. (2004)
and Suresh & Homsy (2004), the physical mechanisms of electrokinetic
instability (EKI) were investigated systematically and thoroughly.
Laminar oscillatory flow fields are remarkable because of the dramatic mass
dispersion enhancement when concentration gradients of miscible mass species
are introduced into the flow field. The basic mechanism that contributes to the
above process is the non-uniform velocity distribution between the wall
boundaries, which require no-slip of the fluid, and the bulk flow region; radial
or lateral concentration gradients across the flow region are therefore generated.
This is the basic idea of ‘Taylor dispersion’ first proposed by Taylor (1953). Aris
(1956, 1960) then extended the idea into more general flow situations by the
method of moments, and broadened the adequate parametric ranges and usage of
the dispersion concept. Recently, Probstein (1994) presented an inductive scaling
illustration of the dispersion enhancement under various conditions when flow
convection is introduced in a circular tube.
In addition to the enhancement of mass dispersion (or transfer), the separation
of different species and cross-over phenomenon in which the slow diffuser
eventually travels faster than the fast diffuser in an oscillatory flow under specific
frequencies may exist and have been shown experimentally by Kurzweg & Jaeger
(1987). Such an effect has been applied to the air revitalization process in the
space station life-supporting systems as described by Thomas (2003) and
Thomas & Narayanan (2002b). Thomas and Narayanan (2001, 2002a) also
presented results due, respectively, to the pressure-driven and boundary-driven
flow oscillations in their studies. Three dimensionless parameters, namely, the
Womersley number W (the ratio of viscous diffusion time-scale to the oscillation
time-scale), the Schmidt number Sc (the ratio of species diffusion time-scale to
the viscous diffusion time-scale), and length ratio of the oscillation amplitude to
the channel-width, were identified by them to be essential in understanding the
separation process among species. This separation process was also studied
analytically by Kurzweg (1988) for oscillatory liquid flows in a bundle of
cylindrical capillaries. Therein, he suggested that despite the considerably low
diffusivities of mass species in liquid solutions, the method using flow oscillation
for species separation was still better than the traditionally used chromatography
method because of the relatively low-operation pressure gradient needed in the
oscillatory system. Nevertheless, little effort has been devoted to understand the
fundamental physics of the enhancement of mass transport and separation of
species in an oscillatory EOF.
In order to understand the fundamental physics as well as provide useful
information and criteria for designing micro-fluidic devices, the present study is,
thus aimed at the theoretical investigation of the transport and separation
phenomena of mass species in a periodically oscillatory EOF in two-dimensional

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2020 H.-F. Huang and C.-L. Lai

A/C
Exsin(w t)

C1
ux h
A C
CL
y

x C2

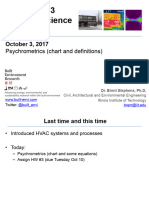
Figure 1. Model of the system considered with the associated coordinates and dimensions.

micro-channels. The flow configuration, assumptions, non-dimensionalization

scheme and governing equations will be given in next section. The analytic
solutions for the electrical potential, velocity distribution and species
concentration field are obtained in §3, followed by the presentation and
systematic discussion of the results in §4. Important aspects derived from this
study will finally be summarized in §5.

2. Assumptions, non-dimensionalization and formulation

The isothermal system considered here is a two-dimensional rectangular channel

of length L and width h filled with a liquid (the solvent) which is an electrolyte or
of aqueous nature. EDLs are, thus established at the upper and lower boundaries
when the carrier liquid is brought into contact with the channel walls. In
addition, carried with the liquid is a neutral species of concentration c. An
oscillatory electrical field is then imposed on the system through the anode and
cathode installed at the two ends of the channel, as shown in figure 1. As a result,
a periodically oscillatory flow is generated via the electroosmotic effect. By
assuming no net flow in a period of oscillatory motion, the present study is aimed
at investigating the effect on the mass transport due to convection of such an
oscillatory EOF.
Three fields thus need to be analysed; they are the electrical, velocity and
concentration fields. In this section, the appropriate assumptions, mathematical
formulations and non-dimensionalization schemes for these three fields are to be
presented and derived, respectively. Following in the next section are the
mathematical solutions of these fields in analytical forms.

(a ) Electrical field
Due to the symmetry of the system, the EDL potentials extending from the
upper and lower walls are symmetrical with respect to the centreline (depicted
by CL in figure 1) of the channel and are assumed not to overlap with each other.
Such a non-overlapping condition is valid when the Debye length of EDL is much
smaller compared with the width of the channel as suggested by Probstein
(1994), e.g. l! h h=lD O 10. In the above expression, lD is the Debye length

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2021

defined as
rffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffiffi
3RT
lD Z ; ð2:1Þ
2F 2 z 2 c 0
with F being the Faraday constant, z the valence of the co- and counter-ions in
the carrier liquid (the solvent is assumed to be a 1 : 1 symmetric electrolyte), 3
the permittivity of the carrier liquid, R the universal gas constant, T the absolute
temperature in Kelvin and c 0 the averaged molar concentration of the counter-
ions.
To further simplify the calculation of the EDL potential, other assumptions
are needed. Firstly, the excessive charge distribution is assumed to vary
significantly merely in the y-direction and hence the electrical potential of the
EDL is viewed as a function of y only. The Boltzmann distribution of the charge
density then applies, which gives
" #
zFj
re ZK2Fzc0 sinh ; ð2:2Þ
RT
where re represents the charge density, j, the EDL potential and c0, the ion
concentration far from the charged-walls. Secondly, because of the slow velocities
of EOFs (about the order of 10K4 m sK1) and the relatively small quantities of
the excessive charges, the convective effect of ions, i.e. the possible electro–
magneto interactions are assumed negligible, as suggested by Karniadakis &
Beskok (2002). The EDL potential is then described by the following Poisson–
Boltzmann equation
" #
2 d2 j re 2Fzc0 zFj
V j Z 2 ZK Z sinh : ð2:3Þ
dy 3 3 RT
Equation (2.3) can be further simplified. With the Debye–Huckel approxima-
tion, the above equation for the electrical potential distribution can then be
linearized as
d2 j 1
2
Z j: ð2:4Þ
dy lD
To non-dimensionalize the above equation, the following scheme is employed:
y!Zy/h, j!Zj/z, where the variables with a superscript ‘!’ denote the
dimensionless ones and z is the Zeta potential at the Stern layer. With such a
non-dimensionalization scheme, the governing equation for the EDL potential in
dimensionless form becomes
d2 j!
!2
Z l!2 j! : ð2:5Þ
dy
The associated boundary conditions are
at the boundaries; y ! Z 0 : j! Z 1; ð2:5aÞ
1 dj!
!
at the centreline; y Z : ! Z 0: ð2:5bÞ
2 dy
In equation (2.5a), the electrical potential at the channel wall is approximated by
the Zeta potential at the Stern layer based on the argument of immobility of ions
therein.

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2022 H.-F. Huang and C.-L. Lai

(b ) Velocity field
The flow considered is assumed to be continuum, isothermal, Newtonian, two-
dimensional and incompressible. The governing equations are the continuity and
momentum equations, i.e.
vux vuy
C Z 0; ð2:6Þ
vx vy
" #
vu
rf C ðu$VÞu ZKVp C mV2 u C re E: ð2:7Þ
vt
In the above equations, (ux, uy) denotes the velocity vector u in the coordinate
system (x, y); rf and m are the density and dynamic viscosity of the carrier liquid;
E is the applied electrical field with re being the charge density. The last term in
equation (2.7), i.e. reE, denotes the Lorenz force which is the main driving force
to generate the EOF.
By assuming the external electrical field be applied only in the x-direction, E
then reduces to the following form:
h i
E Z E x Z Ex sinðutÞ Z Im E fx eiut : ð2:8Þ

As proposed by Oddy et al. (2001) and Morgan & Green (2003), the magnitude
of Ex should be maintained below 100 V mmK1 to avoid possible EK instability
and significant Joule’s heating for an oscillatory EOF, respectively.
By further assuming the flow be fully developed in the x-direction and mainly
driven by the electroosmotic effect, which Dutta & Beskok (2001b) termed ‘pure
electroosmotic flow’, the y-component velocity, i.e. uy, vanishes from equation
(2.6) and the pressure effect in the momentum equation, i.e. equation (2.7),
becomes negligibly small when compared to the Lorenz effect. The momentum
equation (2.7) then reduces to
vux v2 u r h i
Z n 2x C e Im E fx eiut : ð2:9Þ
vt vy rf
To non-dimensionalize the above equation, the following scheme is applied:
g
ux! Z ux =UHS , where
fx z
3E
g
U HS hK ; ð2:10Þ
m
is a moving referenced velocity (Thomas & Narayanan 2002a) suggested by the
Helmholtz–Smoluchowski equation; t!Zt/t with tZ2p/u; and y!Zy/h. In terms
of the above non-dimensional variables and using the linearized EDL potential,
i.e.
3
re ZK 2 j; ð2:11Þ
lD
the momentum equation then assumes the following dimensionless form:
1 vu! v2 u ! !
W 2 !x Z !2x C l!2 j! ei2pt ; ð2:12Þ
2p vt vy

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2023

where
rffiffiffiffi sffiffiffiffiffiffiffiffiffiffi rffiffiffiffiffi
u h 2 =n tn
W hh Z Z ; ð2:13Þ
n 1=u tu
is the Womersley number, a ratio of the viscous diffusion time-scale tn to the
oscillation time-scale tu.
The associated boundary conditions for ux are the no-slip condition at the
channel walls, i.e.
ux! Z 0 at y ! Z 0; 1: ð2:12aÞ
The above condition implies that finite Debye length effects (Dutta & Beskok
2001a) are taken into account in the present study so that a more general
situation of the EOF can be analysed.

(c ) Concentration field
The species to be transported through the carrier liquid is assumed neutral so
that the transport phenomenon will not be affected by any of the electrical
potentials. In addition, the species concentration is also assumed to be infinitely
dilute, so that the concentration gradients of all the species (if there are two or
more species present in the system) will not interfere with each other.
Meanwhile, for an infinitely dilute solution, the molar average velocity is the
same as the mass average velocity which is simply the flow velocity u of the
carrier liquid. Assuming Fick’s law apply, the mass transport equation can then
be written as " 2 #
vc vc v c v2 c
C ux ZD C ; ð2:14Þ
vt vx vx 2 vy 2
where D is the diffusivity coefficient and uyZ0 from the discussion of §2b has
been applied.
The micro-channel in figure 1 is installed between two reservoirs where the
concentration of species are maintained at constant values, c1 for the left
reservoir and c2 for the right one, with c1Oc2. If there is no flow motion of the
carrier liquid, diffusion will be the only mechanism responsible for the transport
of mass species throughout the channel, and the steady-state concentration
distribution will assume a linear form in the x-direction and be uniform in the
y-direction. Now, with a fully developed oscillatory EOF, i.e. uxZux (y, t), as
discussed in §2b, the concentration distribution of the species will not remain
precisely uniform in any cross-section of the channel because of the non-uniform
velocity distribution (no-slip required on the channel walls) across the channel-
width. However, the linear variation of the species concentration along the flow
direction remains unchanged except near the end regions when the system
reaches steady-state. Therefore, it is reasonable to assume the following form for
the species distribution when a fully developed, steady-state oscillatory EOF in a
micro-channel connecting two reservoirs is considered, i.e.
c Kc1
cðx; y; tÞ Z c1 C 2 x C cu ðy; tÞ; ð2:15Þ
L
where cu(y, t) denotes the imposed flow oscillation effect on the concentration
distribution. The boundary conditions at the two ends of the channel, i.e.
c(0, y, t)Zc1 and c(L, y, t)Zc2, are not satisfied exactly by the above equation

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2024 H.-F. Huang and C.-L. Lai

due to the cu(y, t) effect. However, it is within the approximation by neglecting

the end effect of a low-aspect-ratio configuration with AZ h=L/ 1.
Substituting equation (2.15) into equation (2.14), it then yields
vcu $ c Kc % v2 cu
2 1
C ux ZD 2 ; ð2:16Þ
vt L vy
with the following non-penetration condition to be satisfied at the channel walls, i.e.
& &
vcu && vcu &&
Z Z 0: ð2:16aÞ
vy &yZ0 vy &yZh
Let the concentration of the species be non-dimensionalized by cu! Z cu =ðc2 Kc1 Þ,
and the y variable by y!Zy/h, the dimensionless form of equation (2.16) then
reduces to
W2 vcu! 2 !
gD Aux! Z v cu ;
Sc ! C Pe ð2:17Þ
2p vt vy!2
with A and W being the aspect-ratio and Womersley number as defined
previously. The other two dimensionless parameters in the above equation, i.e. Sc
gD , are defined as follows:
and Pe
n
Sc h Z Schmidt number; ð2:18Þ
D
g
gD h h UHS Z diffusion Peclet number:
Pe ð2:19Þ
D
The dimensionless form of the non-penetration boundary condition at the
channel walls becomes
& &
vcu! && vcu! &&
Z Z 0: ð2:17aÞ
vy ! &y!Z0 vy ! &y!Z1
In summary, equations (2.5)–(2.5b) are to be solved to determine the electrical
potential distribution. Equations (2.12) and (2.12a) are for the velocity
distribution and the concentration field is determined by equations (2.17) and
(2.17a). In next section, the mathematical solutions for the above equations will
be pursued and presented.

3. Mathematical solutions

In this section, the analytic solutions for the electrical potential, velocity and
concentration fields are to be determined; the time and space-averaged mass
transfer rate will also be defined and calculated as an estimation of the overall
mass transport. While deriving the expressions for the averaged mass-transfer
rate, the Lagrangian displacement a and the tidal displacement b as suggested by
Kurzweg (1985, 1988) will be employed so that a reasonable comparison of the
overall mass transport among various flow situations can be achieved.

(a ) Electrical field
From equations (2.5)–(2.5b), the linearized EDL potential is solved as
'" ! # " l! # (
! ! 1KeKl y ! l! e K1 Ky! l!
j ðy Þ Z ! ! e C l! Kl! e ; ð3:1Þ
el KeKl e Ke

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2025

which is a rather simple solution satisfying the symmetry condition as requested.

Moreover, the ratio of channel-width to Debye length, i.e. l!, becomes the only
parameter determining the distribution of the linearized EDL potential.

(b ) Velocity field
Due to the linearity of the ux-momentum equation, i.e. equation (2.12), the
expression for ux! is assumed to possess the following form:
h !
i
ux! ðy ! ; t ! Þ Z Im uex! ðy! Þei2pt ; ð3:2Þ
as suggested by the imposed electrical field which is the only forcing term of the
equation. With the substitution of the above equation and the EDL potential
(3.1) into equation (2.12), the following equation governing the space
distribution of ux! is thus obtained
'" ! # " l! # (
d2 uex! 2 e! !2 1KeKl l! y ! e K1 Kl! y!
K iW ux ZKl ! ! e C l! Kl! e : ð3:3Þ
dy!2 el KeKl e Ke
The boundary conditions become as follows:
uex! ð0Þ Z uex! ð1Þ Z 0: ð3:3aÞ
The Green’s function method is then applied to solve equations (3.3) and (3.3a),
and the corresponding Green’s function is derived as
8 $ ! pffi pffi %
> ðy K1ÞW i Kðy!K1ÞW i $
> e Ke pffi pffi %
>
> KxW i
>
>
> pffi Wpffii KWpffii exW i
Ke ; 0% x! y! ;
< 2W iðe Ke Þ
G u ðx; y! Þ Z $ ! pffi p ffi % ð3:4Þ
> y W i Ky! W i $ %
>
> e Ke pffi p ffi
>
> ðxK1ÞW i
KeKðxK1ÞW i ; y ! ! x% 1:
>
> pffi $ p ffi pffi % e
: 2W i eW i KeKW i

The distribution of uex! is thus solved and the expression of ux is obtained, i.e.
h !
i
! ! g e! ! i2pt
ux ðy ; t Þ Z Im UHS ux ðy Þe ;
with
!
pffi !
pffi ! ! ! !
uex! ðy! Þ Z 41 ey W i C 42 eKy W i C 43 ey l C 44 eKy l ; ð3:5Þ
where the coefficients 4i’s are given as
$ ! pffi !
pffi ! !
%
l!2 eKðl CW iÞ Keðl KW iÞ C el KeKl
41 Z ) !2 *+ ) pffi * ) pffi *, ; ð3:6aÞ
2 l K iW2 cosh l! C W i Kcosh l! KW i
$ ! pffi !
pffi ! !
%
l!2 eðl CW iÞ KeKðl KW iÞ C eKl Kel
42 Z ) !2 *+ ) pffi * ) pffi *, ; ð3:6bÞ
2 l K iW2 cosh l! C W i Kcosh l! KW i
$ ! pffi !
pffi pffi pffi %
l!2 eKðl KW iÞ KeKðl CW iÞ C eKW i KeW i
43 Z ) !2 *+ ) pffi * ) pffi *, ; ð3:6cÞ
2 l K iW2 cosh l! C W i Kcosh l! KW i

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2026 H.-F. Huang and C.-L. Lai

and
$ pffi pffi pffi pffi %
!2 ðl!KW iÞ ðl!CW iÞ W i KW i
l e Ke Ce Ke
44 Z ) !2 * + ) p ffi * ) pffi * , : ð3:6dÞ
2 l K iW2 cosh l! C W i Kcosh l! KW i

(c ) The Lagrangian displacement and tidal displacement

After the velocity distribution is solved, the Lagrangian displacement and
tidal displacement can then be calculated. Since the Lagrangian displacement
represents the moving distance of each fluid particle during time interval
DtZtK0, it can be expressed as
ðt
aðy ; tÞ Z ux ðy! ; t 0 Þdt 0 ;
!
ð3:7Þ
0
which is the same for every cross-section in the present study except near the two
end regions. Therefore, the x-position of each fluid particle at time t is denoted by
xZx0Ca. To calculate the averaged moving distance of each cross-section, the
tidal displacement is thus defined and expressed as
& ðh $ & &ð &
&1
& p % && && 1 $ ! p % ! &&
b Z& a y; dy & Z & a y ; dy &: ð3:8Þ
h 0 u 0 u
In equation (3.8), one half-cycle of oscillation has been applied for calculation to
have a maximum averaged moving distance, which will thence be used
equivalently as the averaged amplitude Dx 0 of the periodically oscillatory EOF
(Kurzweg 1985, 1988; Thomas & Narayanan 2001) in the present study. In order
to perform a reasonable comparison of the averaged mass transport, the
oscillation amplitude Dx 0 or b has to be kept the same for each flow situation
considered. As a result, the applied electrical field Efx , the slip velocity U
g HS , and
the corresponding Peclet number Pe gD will no longer remain constant for all the
flow situations. Instead, they have to be adjusted in accordance with the
frequency so that the condition bZDx 0 Zconst. will be preserved, i.e.
& &
& KmuDx 0 &
jEfx ðuÞj Z & & ð3:9Þ
& 2i3zhðuÞ &;
& &
& uDx 0 &
g
jU &
HS ðuÞj Z &
&; ð3:10Þ
2ihðuÞ &
and
g
gD ðuÞ Z h UHS ðuÞ :
Pe ð3:11Þ
D
The factor h(u) in equations (3.9) and (3.10) is given by
41 $ Wpffii % 42 $ KWpffii % 4 $ ! % 4 $ ! %
hZ p ffi e K1 K p ffi e K1 C !3 el K1 K !4 eKl K1 : ð3:12Þ
W i W i l l

(d ) Concentration field
From the previous discussion in §2c, the convective effect on the total
concentration distribution depends only on the oscillatory flow motion. Thus, in
order to be timewise consistent with the flow field, the concentration distribution

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2027

due to the convective effect cu! ðy! ; t ! Þ is assumed to possess the following form
h i
! ! ! e! ! Ki2pt !
cu ðy ; t Þ ZKIm cu ðy Þe : ð3:13Þ

By substituting equations (3.2) and (3.13) into equation (2.17), the time and
space coordinates are decoupled, and equation (2.17) reduces to the following
form
d2 cu! gD Auex! ;
K iScW2 cu! Z Pe ð3:14Þ
dy !2
where cu! is the complex conjugate of ceu! and PegD ðuÞ is the frequency-dependent
Peclet number. With the non-penetration boundary conditions
&
dcu! &&
Z 0; ð3:14aÞ
dy ! &y!Z0;1
the Green’s function of equations (3.14) and (3.14a) is derived as
8 $ ! pffiffiffiffiffi pffiffiffiffiffi %
> ðy K1ÞW iSc Kðy! K1ÞW iSc $
> e Ce pffiffiffiffiffi pffiffiffiffiffi %
>
> KxW iSc
>
> $
pffiffiffiffiffiffiffi KWpffiffiffiffi ffi pffiffiffiffiffi % e xW iSc
Ce ; 0%x!y! ;
>
< 2W iSc e iSc Ke W iSc
G c ðx;y! ÞZ $ ! pffiffiffiffiffi p ffiffiffiffiffi %
>
> e y W iSc
Ce Ky ! W iSc $ pffiffiffiffiffi pffiffiffiffiffi %
>
> ðxK1ÞW iSc KðxK1ÞW iSc
>
>
>
$
pffiffiffiffiffiffiffi KWpffiffiffiffi ffi pffiffiffiffiffi % e Ce ; y! !x%1:
: 2W iSc e iSc KeW iSc

ð3:15Þ
After integrating
ð1
cu! ðy! Þ gD
Z APe G c ðx; y ! Þuex! ðxÞdx; ð3:16Þ
0

and taking the complex conjugate of cu! , the non-dimensional spacewise

distribution of the convective part of the concentration field, i.e. ceu! ðy! Þ, is solved as
$ !
pffiffiffiffiffi !
pffiffiffiffiffi !
pffi
e
cu ðy Þ Z APeD g1 ey Wi iSc C g2 eKy Wi iSc C g3 ey Wi i
! !

pffi % ð3:17Þ
Ky! Wi i l! y ! Kl! y !
Cg4 e C g5 e C g6 e ;

with PeD being the complex conjugate of Pe gD , and the coefficients gi’s being
!
functions of l , W and Sc, i.e.
$ pffi pffiffiffiffiffi % $ pffiffiffiffiffi pffi %
KWi i KWi iSc KWi iSc Wi i
41 e Ke C 42 e Ke
g1 Z p ffiffiffiffiffi ) p ffiffiffiffiffiffi
ffi*
2ðScK1ÞW2 i Sc sinh Wi iSc
$ ! pffiffiffiffiffi % $ pffiffiffiffiffi !
%
l! 43 el KeKWi iSc C l! 44 eKWi iSc KeKl
K pffiffiffiffiffiffiffi) * ) pffiffiffiffiffiffiffi* ; ð3:18aÞ
2Wi iSc l!2 C iScW2 sinh Wi iSc

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2028 H.-F. Huang and C.-L. Lai

$ pffi pffiffiffiffiffi % $ pffiffiffiffiffi pffi %
41 eKWi i KeWi iSc C 42 eWi iSc KeWi i
g2 Z pffiffiffiffiffi ) pffiffiffiffiffiffiffi*
2ðScK1ÞW2 i Sc sinh Wi iSc
$ ! pffiffiffiffiffi % $ pffiffiffiffiffi !
%
l! 43 el KeWi iSc C l! 44 eWi iSc KeKl
K pffiffiffiffiffiffiffi) * ) pffiffiffiffiffiffiffi* ; ð3:18bÞ
2Wi iSc l!2 C iScW2 sinh Wi iSc
42
g3 Z ; ð3:18cÞ
ðScK1ÞiW2
41
g4 Z ; ð3:18dÞ
ðScK1ÞiW2
43
g5 Z !2
; ð3:18eÞ
l C iScW2
and
44
g6 Z !2
; ð3:18f Þ
l C iScW2
where 4i ’s are the complex conjugates of 4i’s. From equations (2.15), (3.13) and
(3.17), the total concentration distribution can then be expressed as
c Kc1 + ,
cðx; y; tÞ Z c1 C 2 x KIm ceu ðyÞeKiut : ð3:19Þ
L

(e ) The time and space-averaged mass-transfer rate

As discussed previously, although there is no net flow in each period of
oscillation, there exists a net mass transport from upstream to downstream,
because of either the pure diffusion effect or the convective effect. In order to
calculate this net mass transport, the following time-and-space averaged mass-
transfer rate, called the effective mass flux, is then defined for each cross-section
of the channel in one period of oscillation (Thomas & Narayanan 2001, 2002a,b;
Thomas 2003), i.e. 'ð ð (
1 u h ð2p=uÞ
Qx Z Jx dt dy : ð3:20Þ
h 2p 0 0
In the above expression, Jx denotes the instant mass flux in the x-direction
caused by both the diffusion and convective effects, i.e.
c Kc1 $ c Kc1 %
Jx ZKD 2 C c1 C 2 x C cu ðy; tÞ ux ðy; tÞ; ð3:21Þ
L L
or
c Kc1 + , c Kc1 + , + , + ,
Jx ZKD 2 C c1 Im uex eiut C x 2 Im uex eiut KIm ceu eKiut Im uex eiut :
L L
ð3:21aÞ
After substituting equation (3.21a) into equation (3.20) and then integrating it
with respect to time, Qx reduces to
ð
c2 Kc1 1 h
Qx ZKD C ðce ue C cu ux Þdy; ð3:22Þ
L 4h 0 u x

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2029

where cu and ux are the complex conjugates of ceu and uex , respectively. The above
relation can also be expressed in the dimensionless form as
ð1 $ %
1
!
Qx Z 1K jPeD j 2
ceu! uex! C cu! ux! dy! ; ð3:23Þ
4 0
with the parameters defined as Qx! Z ðQx LÞ=ðDðc1 Kc2 ÞÞ, ceu! Z ceu! =PeD A and
gD A. The integral in equation (3.23) is expressed in terms of 4i’s
cu! Z cu! =Pe
and gi’s, and their complex conjugates as well, i.e.
ð1 $ %
ceu! uex! C cu! ux! dy! Z 43 g6 C 44 g5 C 43 g6 C 44 g5
0
+) ! * ) ! * ,
e2l K1 ð43 g5 C 43 g5 ÞK eK2l K1 ð44 g6 C 44 g6 Þ
C
2l!
) !2 *n) ! pffi *h $ l!CWipffii % $ ! pffi % io
l KW2 i l KWi i e K1 ð43 g3 C 42 g5 ÞK eKðl CWi iÞ K1 ð44 g4 C 41 g6 Þ
C
l!4 C W4
) !2 *n) ! pffi *h $ l!KWipffii % $ pffi % io
K1 ð43 g4 C 41 g5 ÞK eKðl KWi iÞ K1 ð44 g3 C 42 g6 Þ
!
l KW2 i l C Wi i e
C
l!4 C W4
) !2 *n) ! pffi *h $ l!CWpffii % $ pffi % io
K1 ð41 g5 C 43 g4 ÞK eKðl CW iÞ K1 ð42 g6 C 44 g3 Þ
!
l C W2 i l KW i e
C
l!4 C W4
) !2 *n) ! pffi *h $ l!KWpffii % $ pffi % io
K1 ð42 g5 C 43 g3 ÞK eKðl KW iÞ K1 ð41 g6 C 44 g4 Þ
!
l C W2 i l CW i e
C
l!4 C W4
) pffi pffi *h $ WpffiiCWipffii % $ pffi pffi % i
W i KWi i e K1 ð41 g3 C 42 g4 ÞK eKðW iCWi iÞ K1 ð42 g4 C 41 g3 Þ
C
2W2 i
) pffi pffi *h $ WpffiiKWipffii % $ pffi pffi % i
W i C Wi i e K1 ð41 g4 C 41 g4 ÞK eKðW iKWi iÞ K1 ð42 g3 C 42 g3 Þ
C
2W2 i
) pffi pffiffiffiffiffiffiffi*h $ pffi pffiffiffiffiffi % $ pffi pffiffiffiffiffi %i
W i KWi iSc 41 g1 eW iCWi iSc K1 K42 g2 eKðW iCWi iScÞ K1
C
ð1 C ScÞW2 i
) pffi pffiffiffiffiffiffiffi*h $ pffi pffiffiffiffiffi % $ pffi pffiffiffiffiffi %i
W i C Wi iSc 41 g2 eW iKWi iSc K1 K42 g1 eKðW iKWi iScÞ K1
C
ð1 C ScÞW2 i
) pffi pffiffiffiffiffiffiffi*h $ pffi pffiffiffiffiffi % $ pffi pffiffiffiffiffi %i
Wi i KW iSc 42 g2 eWi iCW iSc K1 K41 g1 eKðWi iCW iScÞ K1
K
ð1 C ScÞW2 i
) pffi pffiffiffiffiffiffiffi*h $ pffi pffiffiffiffiffi % $ pffi pffiffiffiffiffi %i
Wi i C W iSc 42 g1 eWi iKW iSc K1 K41 g2 eKðWi iKW iScÞ K1
K
ð1 C ScÞW2 i
) !2 *n) ! pffiffiffiffiffiffiffi*h $ ! pffiffiffiffiffi % $ pffiffiffiffiffi %io
l KWi iSc 43 g1 el CWi iSc K1 K44 g2 eKðl CWi iScÞ K1
!
l KW2 iSc
C
l!4 C W4 Sc2

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2030 H.-F. Huang and C.-L. Lai

) *n) pffiffiffiffiffiffiffi*h $ ! pffiffiffiffiffi % $ pffiffiffiffiffi %io

l! C Wi iSc 43 g2 el KWi iSc K1 K44 g1 eKðl KWi iScÞ K1
!
l!2 KW2 iSc
C
l!4 C W4 Sc2
) !2 *n) ! pffiffiffiffiffiffiffi*h $ ! pffiffiffiffiffi % $ pffiffiffiffiffi %io
l C W iSc 43 g1 el KW iSc K1 K44 g2 eKðl KW iScÞ K1
!
l C W2 iSc
C
l!4 C W4 Sc2
) !2 *n) ! pffiffiffiffiffiffiffi*h $ ! pffiffiffiffiffi % $ pffiffiffiffiffi %io
l KW iSc 4 3 g2 el CW iSc K1 K44 g1 eKðl CW iScÞ K1
!
l C W2 iSc
C : ð3:24Þ
l!4 C W4 Sc2

4. Results and discussion

Results in this section will generally be presented in the dimensionless forms so

that effects on the velocity distribution, mass transport, etc. can be discussed
systematically through the variations of the dimensionless parameters.
Dimensional results will also be given when exact values of the averaged or
effective mass flux are preferred. Specific dimensions and conditions of the system
as well as the transport properties of certain species used for calculations in this
study are given in tables 2 and 3.
In order to maintain a similar convective contribution to the mass transport, a
fixed tidal displacement, or oscillation amplitude, i.e. Dx 0 , is required for both
high and low-frequency flow oscillations in a given micro-channel filled with
electrolyte buffer. The applied electric field Efx , the slip velocity U
g HS , and the
g
Peclet number PeD are, therefore, functions of the oscillation frequency or
Womersley number. However, the detrimental effects of Joule’s heating and EKI
limit the maximum employable applied electric field, and thus, through
equations (3.7)–(3.12), limit the maximum employable oscillation frequency for
mass transport while the tidal displacement is fixed to a constant. In accordance
with the above argument, the Womersley number is generally maintained below
0.6, which corresponds to approximately 570 Hz for hZ10 mm, nw10K6 m2 sK1
and Dx 0 /hZ1.0, in the following results and discussion. Although frequencies
beyond the above limit may intentionally be applied in the following parametric
study for demonstrating the trends of certain physical phenomena, the employed
frequencies are always smaller than 1 MHz as reported in Green et al. (2000) for
settings of hZ10 mm and nw10K6 m2 sK1.

(a ) The velocity profile

The velocity distributions ux! ðy! Þ for various values of Womersley number W
and the dimensionless Debye length l! at tuZ(2nC0.5)p are shown in figure 2;
figure 2a is for WZ0.5, l!Z20, 70 and 700, figure 2b is for WZ5, l!Z20, 70 and
700 and figure 2c is for WZ15, l!Z20, 70 and 700, i.e. for hZ10 mm channels,
frequencies from 400 Hz to 360 kHz, and Debye lengths from 15 to 500 nm, a
Debye length range that corresponds to electrolyte buffer concentrations from
approximately 10K4 to 10K6 M (Hunter 1981; Dutta & Beskok 2001b). From
figure 2, it can be learnt that when the Womersley number is small, i.e. figure 2a
with WZ0.5, the flow remains in a plug profile generally without phase variation
across the channel-width. However, the velocity distribution tends to vary more

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2031

Table 2. System dimensions, conditions and related physical constants.

item notation value units

aspect ratio A 1.00!10K3 –

channel length L 1.000!10K2 m
channel-width h 1.00!10K5 m
concentration gradient (mass species) (c1Kc2)/L 10.0 mol mK4
dynamic viscosity of carrier (water)a m 8.74!10K4 Ns mK2
Faraday’s constanta F 9.65!104 C mol
kinematic viscosity of carrier (water)a n 0.877!10K6 m2 sK1
permittivity of system studied (water)b 3 6.99!10K10 F mK1
temperature T 298 K
universal gas constant R 8.314 J KK1
valence of electrolyte z G1 –
zeta potentiala z K0.1 V
a
Probstein (1994).
b
Hunter (1981).

Table 3. Mass transport properties and the corresponding Schmidt number for four example mass
species.

mass species diffusivity in water (m2 sK1) Schmidt number

ethanola 1.2!10K9 730.833

glucosea 0.69!10K9 1271.01
glycineb 1.064!10K9 824.248
sucroseb 0.523!10K9 1676.86
a
Çengel (2003).
b
Probstein (1994).

gradually near the solid wall with a smaller value of l!, which indicates a larger
Debye length. As the Womersley number increases, the velocity profile tends to
be non-uniformly distributed across the channel-width as shown in figure 2b and
c, indicating phase lags between the velocity distribution and the imposed
electrical field. Since the counter-ions spread farther into the bulk liquid with a
larger Debye length, i.e. a smaller value of l!, more fluid particles are dragged by
the counter-ions driven by the electrical force. Consequently, the velocity
boundary layer becomes thicker with the peak value of the non-dimensional
velocity distribution being smaller.

(b ) The convective concentration distribution

To calculate cu! ðy! ; t ! Þ for the various mass species, the corresponding Schmidt
numbers of these species are to be specified in advance. From table 3, the
Schmidt numbers for ethanol, glycine, glucose and sucrose are all found to be
around the order of 103. Therefore, the Schmidt number used in the following
computations will be specified as ScZ1000.

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2032 H.-F. Huang and C.-L. Lai

(a) 1.00

0.98

0.96
u*x
0.94

0.92

(b)
0.8

0.6
u*x 0.4

0.2

(c)
0.8 l∗=700
l∗=70
0.6 l∗=20
u*x 0.4

0.2
0
0 0.2 0.4 0.6 0.8 1.0
y*

Figure 2. The dimensionless velocity distributions ux! ðy ! Þ across the channel-width for various
values of Womersley number W and the dimensionless Debye length l! at tuZ(2nC0.5)p (a)
WZ0.5, l!Z20, 70 and 700. (b) WZ5, l!Z20, 70 and 700.(c) WZ15, l!Z20, 70 and 700.

Shown in figure 3 are the calculated concentration distributions cu! ðy ! ; t ! Þ for

WZ0.1, 0.3 and 0.6 at tuZ(2nC0.5)p and with ScZ1000, l!Z70 (lDw140 nm),
and Dx 0 /hZ1.0. As already seen in figure 2a, the velocity distributions around
this frequency range are almost uniform across the channel-width except near the
wall boundaries where a rapid decrease from a finite value to zero prevails
because of the no-slip condition. Consequently, the convective concentration
distributions, as shown in figure 3, also possess similar profiles with significant
variations occurring only near the boundaries, which are approximately the
regions of y!Z0–0.2 and y!Z0.8–1.0. However, it is these variations near the
boundaries that would incur transverse dispersion through the strong
concentration gradient, and thus increase the mass transport rate in the flow
direction as similar to the ‘Taylor dispersion’ effect in the pressure-driven flows.
Such a mass transport phenomenon will be presented and discussed in the
following section. The velocity and concentration distributions for Dx 0 /hZ1.5

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2033

–2
W= 0.1
–4 W= 0.3
W= 0.6
–6
0 0.2 0.4 0.6 0.8 1.0
y*

Figure 3. The dimensionless convective concentration distributions cu! across the channel-width for
WZ0.1, 0.3 and 0.6 at tuZ(2nC0.5)p, with ScZ1000, l!Z70 and Dx 0 /hZ1.0.

and 2.0 are qualitatively similar to those shown in figure 3 deferring only slightly
in the numerical values and hence, not included.

(c ) The time/space-averaged mass transport

The dimensionless averaged mass transport rates, Qx! , due to an oscillatory
EOF are determined by equation (3.23) and are shown in figure 4. The conditions
used for calculations are l!Z70, ScZ1000 and Dx 0 /hZ1.0, 1.5 and 2.0. One of
the results described by figure 4 is that at a fixed value of W, or frequency, the
mass transport rate increases as the tidal displacement enlarges. This is because
a larger tidal displacement magnifies the local, instantaneous convective and
dispersion effects in the concentration field and eventually enhances the mass
transport rate in comparison to the cases with small tidal displacements under a
fixed operation frequency. Another result from figure 4 is that for a fixed tidal
displacement, the mass transport rate increases with the dimensionless frequency
W. In fact, increasing W requests necessarily an equivalent amplification on the
characteristic slip velocity so that a constant tidal displacement can be
preserved. Meanwhile, as W enlarges, the concentration distribution near the
wall boundaries becomes more non-uniform (figure 3) which thus strengthens the
local dispersion effects due to the steeper concentration gradients near the wall
boundaries.
Shown in figure 5a are the dimensionless averaged mass transport rates, Qx! ,
for l!Z20, 70 and 700 with Dx 0 /hZ1.0; all the other conditions are the same as
those used in figure 4. Results from this figure suggest that for a fixed value of W,
the mass transport rate is larger in a flow system with a larger Debye length, or a
smaller value of l!, because of the stronger dispersion effect in the transverse
direction due to the more non-uniform convective concentration distributions as
shown in figure 5b with WZ0.3, or w140 Hz for hZ10 mm and nw10K6 m2 sK1.

(d ) Cross-over phenomenon and separation of species

Shown in figure 6 are the variations of Qx! versus W with ScZ100, 500, 1000,
1500 and 2000 (mass diffusivities from 10K8 to 5!10K10 m2 sK1 in water with

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2034 H.-F. Huang and C.-L. Lai

1.35
1.30
1.25 ∆ x'/ h = 2.0

Q*x 1.20 ∆ x'/ h = 1.5

1.15 ∆ x'/ h = 1.0
1.10
1.05
1.00
0 0.1 0.2 0.3 0.4 0.5
W

Figure 4. The dimensionless averaged mass transport rate Qx! as a function of Womersley number
W with l!Z70, ScZ1000 and Dx 0 /hZ1.0, 1.5 and 2.0.

(a)
1.035
1.030
1.025
1.020
Q*x
1.015
1.010
1.005
1
0 0.1 0.2 0.3 0.4 0.5
W
(b)
2
0
–2
c*u × 10 5

–4 l*= 20
–6 l* = 70
l* = 700
–8
– 10
0 0.2 0.4 0.6 0.8 1.0
y*

Figure 5. The dimensionless averaged mass transport rates Qx! and the associated convective
concentration distributions cu! for l!Z20, 70 and 700 with Dx/hZ1.0. (a) Qx! versus W. (b) cu!
distribution across the channel-width.
nw10K6 m2 sK1); figure 6a is for l!Z20, figure 6b is for l!Z70, and figure 6c is
for l!Z700, all with Dx 0 /hZ1.0. The results indicate that an oscillatory EOF is
always more conducive to the enhancement of mass transport rate of the species
with a larger Sc number, or a smaller mass diffusivity and this phenomenon
becomes more and more obvious with a smaller value of l!. Thus, with a
sufficiently low value of l! and at a sufficiently high frequency, the cross-over

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2035

(a) 1.7
1.6 Sc = 2000
1.5 Sc =1500
Q*x 1.4 Sc =1000
1.3 Sc =500
1.2 Sc =100
1.1
1.0
(b)
1.030
1.025
1.020
Q*x 1.015
1.010
1.005
1.000
(c) 1.0004

1.0003

Q*x 1.0002

1.0001

1.0000
0 0.1 0.2 0.3 0.4 0.5
W

Figure 6. The variations of Qx! versus W with Dx 0 /hZ1.0 and ScZ100, 500, 1000, 1500 and 2000.
(a) l!Z20. (b) l!Z70. (c) l!Z700.

phenomenon as appearing in the variations of the ‘dimensional’ mass transport

rate Qx versus the Womersley number W becomes possible.
Examples of the cross-over phenomenon are shown in figure 7a for glucose and
sucrose and in figure 7b for ethanol and sucrose both with l!Z20 and Dx 0 /hZ
10.0, wherein the first and second cross-over points are identified. Note that when
the oscillation frequency is high enough, a second cross-over point exists. When
the second cross-over exists, the oscillatory EOF at sufficiently high W becomes
effectively conducive to the enhancement of mass transport rate of the species
with a higher mass diffusivity. With the first and second cross-over points
identified, the application of oscillatory EOF in the species separation becomes
very interesting. For the flow situation falling in the region beyond the second
cross-over point, the species with a high diffusivity will be transported
downstream even faster than that with a lower diffusivity. Under such a flow
condition, the oscillatory EOF can be utilized as the first-step separation of
species. However, under certain circumstances, the species with a lower
diffusivity needs to be separated from the one with a higher diffusivity and
transported to a second container with more appropriate environment; the flow
situation falling in between the first and second cross-over points, thus becomes
preferable. In conclusion, with proper choices of the Debye length, oscillation

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2036 H.-F. Huang and C.-L. Lai

(a) 15.0
12.5 sucrose
10.0 glucose

Qx ×10 8
7.5
5.0 2nd cross-over
2.5
1st cross-over
0

(b) 15.0
12.5 sucrose
Qx ×10 8
10.0 ethanol 2nd cross-over
7.5
5.0
2.5
1st cross-over
0 0.05 0.10 0.15 0.20 0.25 0.30
W

Figure 7. The cross-over phenomenon with l!Z20 and Dx 0 /hZ10.0. (a) Glucose and sucrose. (b)
Ethanol and sucrose. The material properties are listed in table 3.

frequency, and tidal displacement, oscillatory EOFs may become a good

candidate for the first-step separation of mass species.

5. Concluding remarks

The mass transport phenomena driven by an oscillatory EOF in a two

dimensional micro-channel are studied theoretically herein. With the assump-
tions of non-overlapping EDLs, linearized electrical potentials, a fully developed
incompressible flow in the streamwise direction, and an infinitely dilute solution
with neutral species, the governing equations for the electrical potential, as well
as the velocity and the concentration distributions are uncoupled so that
analytical solutions become attainable. Results are then presented and discussed
systematically with the following conclusions drawn from the present study.

(i) The velocity and concentration distributions across the channel-width

become more and more non-uniform as the Womersley number W
increases, which might then induce a stronger dispersion effect in the
transverse direction.
(ii) With a constant tidal displacement, the total mass transport rate Qx!
increases with W due to both the stronger convective and transverse
dispersion effects.
(iii) With a fixed value of W, the total mass transport rate Qx! also increases
rapidly with the tidal displacement because of the associated stronger
convective effects.
(iv) With a sufficiently low value of l! and at a sufficiently large value of W,
the cross-over phenomenon of the mass transport rates for different
species becomes possible.

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

Oscillatory electroosmotic flows 2037

(v) With proper choices of the Debye length, oscillation frequency and tidal
displacement, a first-step separation of species by means of an oscillatory
EOF becomes achievable.

Future work may include the removal of the linear electrical potential
assumption; the transient effect on the velocity and concentration distributions
may also need to be considered for the flow situations with WR1. Numerical
results will then become inevitable.
The authors gratefully acknowledge the financial support from the National Science Council of
Taiwan, ROC, through grant no. NSC93-2212-E-002-036.

References
Aris, R. 1956 On the dispersion of a solute in a fluid flowing through a tube. Proc. R. Soc. A 235,
67–77.
Aris, R. 1960 On the dispersion of a solute in pulsating flow trough a tube. Proc. R. Soc. A 259,
370–376.
Çengel, Y. A. 2003 Heat transfer: a practical approach, 2nd edn, p. 726. New York: McGraw-Hill.
Dutta, P. & Beskok, A. 2001a Analytical solution of combined electroosmotic/pressure-driven
flows in two-dimensional straight channels: finite Debye layer effects. Anal. Chem. 73,
1979–1986. (doi:10.1021/ac001182i)
Dutta, P. & Beskok, A. 2001b Analytical solution of time periodic electroosmotic flows: analogies
to Stokes’ second problem. Anal. Chem. 73, 5097–5102. (doi:10.1021/ac015546y)
Erickson, D. & Li, D. 2003 Analysis of alternating current electroosmotic flows in a rectangular
microchannel. Langmuir 19, 5421–5430. (doi:10.1021/la027035s)
Green, N. G., Ramos, A., Gonzalez, A., Morgan, H. & Castellanos, A. 2000 Fluid flow induced by
nonuniform ac electric fields in electrolytes on microelectrodes. I. Experimental measurements.
Phys. Rev. E 61, 4011–4018. (doi:10.1103/PhysRevE.61.4011)
Hunter, R. J. 1981 Zeta potential in colloid science, pp. 359–359, 1–121, London: Academic Press.
Karniadakis, G. E. & Beskok, A. 2002 Micro flows: fundamentals and simulation, pp. 193–210. New
York: Springer.
Kurzweg, U. H. 1985 Enhanced heat-conduction in oscillating viscous flows within parallel-plate
channels. J. Fluid Mech. 156, 291–300.
Kurzweg, U. H. 1988 Enhanced diffusional separation in liquids by sinusoidal oscillations. Sep. Sci.
Tech. 23, 105–117.
Kurzweg, U. H. & Jaeger, M. J. 1987 Diffusional separation of gases by sinusoidal oscillations.
Phys. Fluids 30, 1023–1025. (doi:10.1063/1.866300)
Lin, H., Storey, B. D., Oddy, M. H., Chen, C. H. & Santiago, J. G. 2004 Instability of electrokinetic
microchannel flows with conductivity gradients. Phys. Fluids 16, 1922–1935. (doi:10.1063/1.
1710898)
Morgan, H. & Green, N. G. 2003 AC electrokinetics: colloids and nanoparticles, pp. 81–158.
Oxford: Research Studies Press.
Oddy, M. H., Santiago, J. G. & Mikkelsen, J. C. 2001 Electrokinetic instability micromixing. Anal.
Chem. 73, 5822–5832. (doi:10.1021/ac0155411)
Probstein, R. F. 1994 Physicochemical hydrodynamics: an introduction, pp. 21–29, 37–50, 82–96,
190–202, 2nd edn. New York: Wiley
Suresh, V. & Homsy, G. M. 2004 Stability of time-modulated electroosmotic flow. Phys. Fluids 16,
2349–2356. (doi:10.1063/1.1736677)
Taylor, G. I. 1953 Dispersion of soluble matter in solvent flowing slowly through a tube. Proc. R.
Soc. A 219, 186–203.

Proc. R. Soc. A (2006)

 Downloaded from http://rspa.royalsocietypublishing.org/ on November 7, 2016

2038 H.-F. Huang and C.-L. Lai

Thomas, A. M. 2003 Unusual effects of oscillating flows in an annulus on mass transfer and
separation. Adv. Space Res. 32, 279–285. (doi:10.1016/S0273-1177(03)90263-8)
Thomas, A. M. & Narayanan, R. 2001 Physics of oscillatory flow and its effect on the mass transfer
and separation of species. Phys. Fluids 13, 859–866. (doi:10.1063/1.1351549)
Thomas, A. M. & Narayanan, R. 2002a A comparison between the enhanced mass transfer in
boundary and pressure driven oscillatory flow. Int. J. Heat Mass Transfer 45, 4057–4062.
(doi:10.1016/S0017-9310(02)00111-4)
Thomas, A. M. & Narayanan, R. 2002b The use of pulsatile flow to separate species. Ann. NY
Acad. Sci. 974, 42–56.

Proc. R. Soc. A (2006)