Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd Logo
Upload

Welcome to Scribd!

  • 154 views

    BY Moses Ssali at GHS 2020 0775620833/0704221195: Prov 1:7

    Uploaded by

    Athiyo Martin
    1. The document contains 14 multi-part vector problems involving triangles and parallelograms. The problems involve expressing vectors in terms of given variables, finding ratios and collinearity, and using vector methods. 2. Common tasks include expressing vectors in terms of variables like a and b representing sides of shapes, finding ratios such as of line segments or vectors, showing collinearity of points, and using properties of shapes like triangles and parallelograms. 3. Vector diagrams are used to represent the information visually and solve problems step-by-step using vector properties and relationships between lengths, ratios, and collinearity of points.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd

    BY Moses Ssali at GHS 2020 0775620833/0704221195: Prov 1:7

    Uploaded by

    Athiyo Martin
    154 views5 pages
    1. The document contains 14 multi-part vector problems involving triangles and parallelograms. The problems involve expressing vectors in terms of given variables, finding ratios and collinearity, and using vector methods. 2. Common tasks include expressing vectors in terms of variables like a and b representing sides of shapes, finding ratios such as of line segments or vectors, showing collinearity of points, and using properties of shapes like triangles and parallelograms. 3. Vector diagrams are used to represent the information visually and solve problems step-by-step using vector properties and relationships between lengths, ratios, and collinearity of points.

    Original Title

    1593678854

    Copyright

    © © All Rights Reserved

    Available Formats

    PDF, TXT or read online from Scribd

    Did you find this document useful?

    Is this content inappropriate?

    1. The document contains 14 multi-part vector problems involving triangles and parallelograms. The problems involve expressing vectors in terms of given variables, finding ratios and collinearity, and using vector methods. 2. Common tasks include expressing vectors in terms of variables like a and b representing sides of shapes, finding ratios such as of line segments or vectors, showing collinearity of points, and using properties of shapes like triangles and parallelograms. 3. Vector diagrams are used to represent the information visually and solve problems step-by-step using vector properties and relationships between lengths, ratios, and collinearity of points.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    154 views5 pages

    BY Moses Ssali at GHS 2020 0775620833/0704221195: Prov 1:7

    Uploaded by

    Athiyo Martin
    1. The document contains 14 multi-part vector problems involving triangles and parallelograms. The problems involve expressing vectors in terms of given variables, finding ratios and collinearity, and using vector methods. 2. Common tasks include expressing vectors in terms of variables like a and b representing sides of shapes, finding ratios such as of line segments or vectors, showing collinearity of points, and using properties of shapes like triangles and parallelograms. 3. Vector diagrams are used to represent the information visually and solve problems step-by-step using vector properties and relationships between lengths, ratios, and collinearity of points.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    of 5

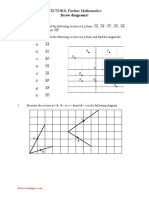
    VECTOR EXERCISE PART TWO

    1. In a triangle OAB, OA  a, OB  b, P and Q are points on OA and AB


    respectively such that 3OP = PA, AQ = 2QB and N is the midpoint of OQ.
    ANM is a straight line which is such that AN = 5NM. Given also that
    OM  hOB, where h is a scalar.
    (a) Express the following vectors in terms of a and b:
    (i) OQ
    (ii) AN
    (iii) PN
    (iv) NB

    (b) Show that the points P, N and B are collinear


    (c) Find the value of h.

    2. In the figure below, P is a point on AD such that PD : AP = 1 : 2, OA  a,


    OB  b, 3OB = 2BD and OC = 3CE = 3AP.
    A P D

    a B
    b
    C E
    O 
    (a) Express the following vectors in terms of a and b:
    (i) AD
    (ii) BP
    (iii) DC
    (b) Show that AD : OE = 3 : 8

    3. In the figure below, PT  3a, PR  3b, PQ  4PT, 2PS  PV and


    3RS  2RT.
    P 3a T Q
    S
    3b V

    R
    (a) Express the following vectors in terms of a and b:
    (i) RS
    (ii) PV
    (iii) RQ
    (b) Find the ratio of RV to RQ.

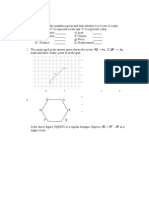
    4. In the figure below, OA  a, OB  b, F and G are points on AC such that


    AF : AB = 3 : 4 and AG : AC = 2 : 3. Point D is on OA such that
    OD : DA = FB : BG = 1 : 2.

    BY Moses Ssali @ GHS 2020 0775620833/0704221195 mssali87@gmail.com Prov 1:7 1


    A

    F
    a B
    D G
    b
    O C
    (a) Express AG and AC in terms of AB. Hence find the following vectors in
    terms of a and b:
    (i) AB
    (ii) AC
    (iii) DG
    (iv) OF
    (b) Find the ratio DG : OC
    5. In the figure below, OP  p, OQ  q, OS  3 OP and PR : RQ = 2 : 1
    4
    P

    S R
    T
    p

    O Q
    q
    (a) Express the following vectors in terms of p and q:
    (i) PQ
    (ii) OR
    (iii) SQ
    (b) Line OR and SQ meet at point T such that OT = hOR and ST = kSQ.
    (i) By expressing OT in two different ways, find the values of h and k
    (ii) Determine the ratio in which T divides SQ

    6. In the figure below, OABC is a parallelogram where OA = a and


    AB = b. Point N is on OA such that ON:NA = 1:2.
    C B

     X

    O N A
    (a) Express the following vectors in terms of a and b:
    (i) AC
    (ii) BN
    (b) Line AC and BN meet at point X such that AX = hAC and BX = kBN
    (i) By expressing OX in two different ways, find the values of h and k
    (ii) Determine the ratio in which X divides AC

    BY Moses Ssali @ GHS 2020 0775620833/0704221195 mssali87@gmail.com Prov 1:7 2


    7. In a triangle OAB, OA  a, OB  b, N and M are points of AB and OB
    respectively. Line ON and AM meet at point T such that AT = TM and
    OT  3 ON. Given that OM = xOB and AN = yAB, Express the vectors:
    4
    (i) AM and OT in terms of a, b and x.
    (ii) ON and OT in terms of a, b and y, hence find the values of x and y.

     6 5 
    8. ABCD is a parallelogram with CB   ,
     CD    and point C is (5, 2). Find the:
     10 
     6   
    (a) coordinates of:
    (i) B (ii) D (iii) A
    (b) length of the diagonal AC
    (c) point of intersection of the diagonal AC and BD

     1   7   6
    OP =  

    OQ =  

    PN =  .

    9. The vectors  15  ,  11  and 3

    (a) Find the:


    (i) position vector of N
    (ii) length of ON
    (iii) coordinates of point E, where E divides PQ in the ratio 1:3.
    (b) Use the vector method to show that N lies on PQ. Hence state the ratio PN : PQ.

    10. Given that OA = a, OB = b and C is the midpoint of AB,


    (a) Draw a vector diagram showing vector AB.
    (b) Express in terms of a and b the vectors:
    (i) AB
    (ii) OC

    11. In the triangle OAB, OA  a, OB  b, C is a point on AB such that


    AC:AB = 1:3 and D is the midpoint of OB.
    A

    a C
    X

    O D b B
    (a) Express the following vectors in terms of a and b:
    (i) AB (ii) OC (iii) AD

    (b) X is a point on AD such that AX : AD = 1 : 2. Find in terms of a and b the


    vectors:
    (i) AX
    (ii) OX

    (c) Find in simplest form the ratio OX : OC.

    BY Moses Ssali @ GHS 2020 0775620833/0704221195 mssali87@gmail.com Prov 1:7 3


    12. In the triangle OAB, OA  a, OB  b, D is a point on OB such that
    OD:OB = 2:5 and E is the midpoint of AB.
    A

    a E
    F
    E

    O D b B
    (a) Express the following vectors in terms of a and b:
    (i) OE (ii) AD

    (b) Given that AF = tAD and OF = hOE, find the values of t and h

    (c) Show that the points O, F and E are collinear

    13. In the triangle ABC, AB  b, AC  c, D is a point on AC such that


    AD:DC = 3:2 and E is the midpoint of BC.
    B

    b E
    F
    E

    A D c C
    (a) Express the following vectors in terms of a and b:
    (i) BD (ii) AE

    (b) Given that BF = tBD and AF = nAE, find the values of t and n

    (c) State the ratio of BD to BF

    14. In the triangle OAB, OA  a, OB  b, Point R divides AB in the ratio 2:5


    and point T divides OB in the ratio 1:3.
    A

    a R
    D

    O T b B

    BY Moses Ssali @ GHS 2020 0775620833/0704221195 mssali87@gmail.com Prov 1:7 4


    (a) Express the following vectors in terms of a and b:
    (i) BT (ii) OR (iii) AT

    (b) Given that AD = kAT and RD = hRO, find the values of k and h. Hence
    express vector AD in terms of a and b

    15. In the triangle OPQ, OP  p, OQ  q, OS  1 OP, OR  1 OQ and point T is


    3 3
    on QS such that QT  3 QS
    4
    P

    S
    p T

    O Q
    R q
    (a) Express the following vectors in terms of p and q:
    (i) SR (ii) QS (iii) PT (iv) TR
    (b) Show that the points P, T and R are collinear.

    16. 2019 P2 No. 8, 11


    17. 2018 P2 No. 10, 16
    18. 2017 P2 No. 10, 15
    19. 2016 P2 No. 8, 16
    20. 2015 P2 No. 4, 13
    21. 2014 P2 No. 10, 14
    22. 2013 P2 No. 3, 16
    23. 2012 P2 No. 2, 13
    24. 2011 P2 No. 9, 15
    25. 2010 P1 No. 17 P2. No. 7
    26. 2009 P2 No. 6, 9, 16
    27. 2008 P1 No. 14 P2 No. 4
    28. 2007 P1 No. 13.
    29. 2006 P2 No. 15
    30. 2005 P1 No. 5. P2 No. 16
    31. 2004 P2 No. 16.
    32. 2003 P2 No. 14.
    33. 2002 P1 No. 7. P2 No. 4, 12
    34. 2001 P1 No. 8. P2 No. 14
    35. 2000 P1 No. 7. P2 No. 13.

    The end.
    Practice makes perfect

    BY Moses Ssali @ GHS 2020 0775620833/0704221195 mssali87@gmail.com Prov 1:7 5

    You might also like