CHAPTER 23

Chapter 23 : Capital Budgeting Methodology: The Financial

Analysis Stage
LEARNING OBJECTIVES After studying this chapter, you should be able to:
1. Discuss the discounted cash flow methods, and explain the net present value (NPV), internal rate of
return (IRR), and present value index (PVI) methods.
2. Discuss the nondiscounted cash flow methods, which are the payback period (PP) method and the
accounting rate of return (ARR) method.
3. Describe the impact of income taxes, purchasing versus leasing, and inflation on capital budgeting
financial analysis.
4. Explain how sensitivity analysis assists managers in making capital budgeting decisions.

INTRODUCTION
Once the first stage of the capital budgeting methodology (covered in the previous chapter) has linked
capital projects with an enterprise's vision and strategy, and estimated the quantity and timing of cash
flows, the data are subjected to financial analysis. Using financial analysis methods (the second stage of
the capital budgeting methodology), managers evaluate and compare alternative projects included in the
Capital Projects Portfolio Statement. Such candidate capital projects will usually differ in the amount of
initial investment required, terms of useful life, amount and timing of cash flows, salvage value, and cost
of capital.
Two types of capital budgeting financial analysis methods are covered in this chapter:
• Discounted cash flow methods
• Nondiscounted cash flow methods.

DISCOUNTED CASH FLOW METHODS

The main methods that managers use to financially analyze capital projects are called discounted cash
flow (DCF) methods, which include the following:
• Net present value (NPV) method
• Internal rate of return (IRR) method
• Present value index (PVI) method
These methods rely on the time value of money, a concept that combines two basic principles:
• A dollar today is worth more than a dollar in the future (the idea of present value).
• The longer one waits for a dollar, the more uncertain the receipt is (the idea of risk).
Methods that incorporate the time value of money are dependent on a discount rate, which is based on cost
of capital.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 1

LEARNING
OBJECTIVE 1

Discuss the dis- THE COST OF CAPITAL AND THE DISCOUNT RATE
counted cash flow
methods, and Most authorities lean toward some type of weighted-average cost of capital, which is viewed as a pool of
explain the net capital investment funds that come from debt and equity sources. The correct weighted-average cost of
present value
(NPV), internal rate capital is the one that reflects an enterprise's expected financing costs for its desired long-term capital
of return (IRR), and structure mix. Under this approach, the weighted-average cost of capital reflects market conditions for
present value index securing incremental financing and enables an enterprise to evaluate its capital structure based on future
(PVI) methods.
events. New projects being evaluated must earn a rate of return equal to or higher than the marginal cost of
capital in order to maintain or increase the value of an enterprise. Alternatively, weighted-average cost of
capital based on historical capital sourcing values may not be relevant to future sources of investment
funds.
Given a mix of debt, preferred stock, common stock, retained earnings, and recovered capital, a weighted-
average cost of capital can be calculated. To do this, the cost of raising a dollar from each source in the
capital investment pool is estimated and weighted according to its proportion in the mix.
To measure the cost of debt, the effective rate of interest that would have to be paid to acquire capital from
new debt is used. The effective interest rate should be net of income taxes because interest is deductible for
tax purposes.
Preferred stock usually has a contractual dividend rate. Consequently, this rate can be used to determine
the effective cost of acquiring additional capital from the issuance of preferred stock. Unlike interest on
debt, however, dividends are not deductible for income tax purposes. Thus, the effective rate is the annual
contractual dividend per share divided by the estimated future share price.
The most troublesome aspect of calculating cost of capital is determining the cost of the common equity
component of capital. Authorities do not agree on how this should be done. Some argue that the proper rate
is the expected earnings per share divided by the current market value of the stock. Others contend that the
cost of common equity funds is a function of expected dividends and expected share price. This theory
must allow for an expected growth in dividend payments.
For capital resulting from retained earnings and capital recoveries (from depreciation), it must be recog-
nized that shareholders do not pay income tax on undistributed assets. Presumably, shareholders would be
willing to accept a smaller return on this source of capital than on common stock.
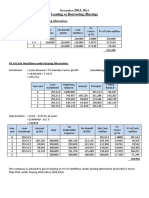
Exhibit 23-1 illustrates the cost of capital calculations for Cyberlink Corporation using a traditional inter-
nally-focussed method. There are other ways to compute the WACC that you will learn in your finance
classes. The interest rate on debt is expected to be 10 percent, and debt is 20 percent of the sources of capi-
tal funds. Cyberlink's income tax rate is expected to be 40 percent over the next number of years. Preferred
stock contributes up to 10 percent of capital funds, and its effective rate is expected to be 12 percent. Com-
mon stock comprises 40 percent of capital funds at an estimated future cost of 18 percent. Retained earn-
ings and recovered capital provide 30 percent of capital funds at an estimated future cost of 16 percent.
Based on the computations in the exhibit, Cyberlink's expected weighted-average cost of capital is 14.4
percent. This means that it will cost Cyberlink an average of 15 percent (rounded up) of each dollar annu-
ally to finance capital projects.
A discount rate, also referred to as a hurdle rate, should, in most cases, equal or exceed the enterprise's
cost of capital. In other words, the discount rate is the required rate of return.
Some managers set the discount rate higher than the cost of capital because they recognize that indirect
costs sometimes increase as the enterprise expands. Also, some capital projects are riskier than others
because their outcome is more difficult to estimate. Managers will need some type of counterbalance or
cushion for accepting the riskier alternatives. This cushion frequently takes the form of a higher discount
rate. For example, in the case of Cyberlink, the discount rate for a low-risk project may be set at 12 percent
(less than the cost of capital); for a moderate-risk project, 15 percent; for an average-risk project, 16 per-
cent; for a high-risk project, 20 percent; and for a super high-risk project, 30 percent or more. Because of
the compounding nature of discounted cash flow methods, this method of adjusting for risk implicitly
assumes that risk increases over time.
Managers exercise a significant amount of subjectivity in choosing the amount by which the discount rate
will be increased to account for risk. In any capital budgeting decision, the estimation of costs, benefits,
and cash flows, as well as risk, will always involve subjectivity. As the previous chapter pointed out, man-

Page 2 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Exhibit 23-1 Weighted-Average Cost of Capital for Cyberlink Corporation—Traditional Method

Source of capital funds Desired long- Aftertax cost of financ- Weighted-aver-

term proportion ing age cost of capi-
of total capital tal
funds
Debt 20% 6% (10% x 60%)* 1.2%
Preferred stock 10 12 1.2
Common stock 40 18 7.2
Retained earnings and recovered capital 30 16 4.8
Weighted-average cost of capital 14.4%
*Cyberlink's income tax rate is expected to be 40%; thus, its proportion of aftertax net income is 60%.

agers may also allow for risk in their estimates of feasibility factors and the amount and timing of cash
flows. For example, the technology feasibility factor of a state-of-the-art capital project may be purposely
weighted lower than a capital project involving traditional technology with which the organization is
familiar. Or, the estimated future cash inflows of a risky project may be systematically reduced.
Another reason for raising the discount rate is the shareholder short-term mindset for quick returns. Amer-
ican managers often feel pressure from fidgety shareholders to recoup investments fast. At public compa-
nies, the average holding period for stocks is two years. Consequently, the discount rates used to evaluate
projects are higher than in other countries and much higher than their cost of capital. Higher discount rates
do not encourage managers to make long-term capital investments.
DETERMINING THE PRESENT VALUE OF UNEQUAL CASH FLOWS USING A DISCOUNT
RATE. The Present Value of One Dollar Table, or a calculator, can be used to determine the present value
of some lump-sum amount of cash in the future. Using a table, the discount rates are shown at the top of
the table, while the number of periods appear at the left. You may have seen this in an introductory class.
The value at which a discount rate and a number of periods intersect is the present value factor. For exam-
ple, the value of $1.00 received three years from now at a discount rate of 14 percent is $0.675, or a pres-
ent value factor of 0.675. To determine the present value of $1,750 received three years from now at a
discount rate of 14 percent, the following calculation is performed:
Present value = $1,750 x .675 = $1,181.25
To find the present value of two unequal cash inflows of $1,000 and $3,000 occurring in years 1 and 2,
respectively, and discounted at 12 percent, the following computations are performed:
Year Cash Inflows Present Value Factor (12%) Present Value
1 $1,000 .893 $ 893
2 3,000 .797 2,391
Total present value $3,284

DETERMINING THE PRESENT VALUE OF EQUAL CASH FLOWS. Some-times cash flows
occur in equal amounts per period; this is termed an annuity. In an ordinary annuity, the series of cash
inflows occur at the end of the periods. If a capital project generates $2,000 of annual cash inflows for four
years at a discount rate of 10 percent, then the present value of this annuity is $6,340 ($2,000 annuity x
3.170 present value factor).
Please refer to any PV tables, or any financial calculator. Each calculator is different and you need to prac-
tice with the one you have.
The values in the Present Value of an Ordinary Annuity of One Dollar Table are cumulative values from
the Present Value of One Dollar Table. For example, the $2,000 annuity is handled as if it were a series of
unequal amounts as follows:
The $2 difference between the amount computed by the factor in the Present Value of an Ordinary Annu-
ity of One Dollar Table ($6,340 = $2,000 x 3.170) and the amount computed by the sum of present value
factors in the Present Value of One Dollar Table ($6,338 = $2,000 x each present value factor) is due to
rounding. Both tables assume that cash inflows occur at the end of the year. The phrase an annuity due
means the payment happens at the beginning of the year.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 3

 Year Cash Inflows Present Value Present
Factor (10%) Value
1 $2,000 .909 $1,818
2 2,000 .826 1,652
3 2,000 .751 1,502
4 2,000 .683 1,366
3.169 $6,338

THE NET PRESENT VALUE METHOD

The net present value (NPV) method measures the difference in the present value of cash inflows and
outflows associated with a capital project. Future cash flows are discounted to their present value using a
desired discount rate. The basic decision rule for the NPV method is:
When NPV is: Decision:
>0 Accept project
<0 Reject project
=0 Indifferent
The accompanying cases help demonstrate the application of the NPV method.
In the Tahoe Ski Lodge case (see next Insights and Applications), the capital project generated equal peri-
odic cash inflows of $160,000. In many projects, however, the periodic cash inflows are unequal. This situ-
ation can be seen in the Gold Nugget case (next page).

THE INTERNAL RATE OF RETURN METHOD

The internal rate of return (IRR) method (also called the time-adjusted rate of return) uses a specified
rate of return as a hurdle rate against which the IRR is compared. The fundamental decision rule for the
IRR method is:
When IRR is: Decision:
> some specified hurdle rate Accept project
<specified hurdle rate Reject project
=specified hurdle rate Indifferent
When the cash inflows are not uniform, as is usually the case, the rate is calculated by a trial-and-error pro-
cess. Various rates are tried until the correct one is found. The correct rate is the one where the present
value of the cash inflows is equal to the present value of the investment. The Euclid Machinery case on the
next page illustrates the calculation of the IRR.

THE PRESENT VALUE INDEX METHOD

If capital projects involve different amounts of investment, it is useful to prepare a relative ranking of the
capital projects by using a present value index (PVI), computed as follows:
PVI = Present value of cash inflows / Present value of cash outflows

According to the analysis, Tahoe Ski Lodge should purchase the new ski lift because it generates a net
present value of $42,880 ($542,880 total present value of cash inflows - $500,000 initial investment, which
is more than the desired 16 percent rate of return). In other words, Tahoe could spend up to $542,880 for
the new ski lift chase the new ski lift and still obtain the 16 percent rate of return it desires. The net present
value of $42,880, therefore, provides a “cushion” or “margin of error” for the company in estimating the
benefits of the new project.
The present value index (PVI) method, also termed the profitability index (PI) and cost/benefit ratio,
measures the ratio of the present value of cash inflows to the present value of cash outflows. The index will
equal 1.0 when the present value of cash inflows equals the present value of cash outflows. A PVI of 1.30
indicates that for every dollar of present value invested in the project, the enterprise will receive cash
inflows with a present value of $1.30. The higher a project's PVI, the more profitable that project is per
investment dollar. The case about Maxximum Corporation on the following pages uses the PVI in ranking
two projects.

Page 4 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

INSIGHTS & APPLICATIONS From the 16 percent column for five periods in
Exhibit 23-3, the discount factor is 3.274.The
Tahoe Ski Lodge's NPV Analysis of a Project with annuity of $160,000 annually is discounted to its
Equal Net Cash Inflows and a Residual Value present value of $523,840 ($160,000 annual cash
inflow x 3.274 discount factor). The residual
Tahoe Ski Lodge is considering acquiring a new value of $40,000 is another form of cash inflow
ski lift that will cost $500,000. The ski lift will that must be discounted to its present value and
last five years, and at the end of the five-year added to the present value of the project. The
period, will have a $40,000 residual value. Use of $40,000 must be discounted as a single amount,
the ski lift will increase cash inflows by $160,000 not as an annuity, because it will be received only
per year. Management at Tahoe requires a 16 per- at the end of the ski lift's useful life when it is
cent target rate before taxes on all investment sold. Therefore, the discount factor is found in the
projects. Management also requires that capital 16 percent column for five periods in Exhibit 23-
budgeting decisions be based on NPV analy- 2. The resulting discount factor is .476. The resid-
sis.These cash inflows represent a stream of peri- ual value of $40,000 is multiplied by .476, giving
odic amounts that can be referred to as an annuity. a present value of $19,040. This present value is
The present value of an annuity is calculated by added to the present value of the $160,000 annu-
multiplying the periodic amount ($160,000 annu- ity, giving a total present value of $542,880
ally in this case) by the present value of an annu- ($523,840 present value of five periodic cash
ity of $1 for five periods discounted at 16 percent. inflows of $160,000 + $19,040 present value of
residual value of $40,000, both discounted at 16
percent). A summary of this analysis follows:

Ski Lift Project Present Value factor Cash inflows Present Value Of Cash
(16%) Inflows
Present value of equal cash 3.274 X
$160,000 per year = $523,840
inflows
Present value of residual value .476 X 40,000 = 19,040
Present value of the project's total cash inflows $542,880
Cash outflow (initial invest- <500,000>
ment)
NPV of project $ 42,880

As the preceding analysis shows, if capital projects being compared involve different dollar amounts of
investment, the project with a greater NPV may not be the more attractive project financially if it also
requires a larger investment. For example, an NPV of $5,000 on an investment of $200,000 is not as finan-
cially attractive as an NPV of $4,000 on an investment of $50,000, provided that the $150,000 difference
in investments can be used to realize an NPV of at least $1,001 in other projects. In this case, the PVI
should be used rather than the NPV dollar figure.

RANKING INDEPENDENT PROJECTS UNDER LIMITED INVESTMENT

FUNDS
Independent projects are capital projects that have no specific bearing on one another. For example,
acquisition of a local area network is not related to the acquisition of a fleet of trucks.
Theoretically, an enterprise should invest in all independent capital projects with a positive NPV. Nor-
mally, however, an enterprise has limited capital and therefore cannot invest in all candidate projects. In
such situations, managers should select those projects that maximize return on dollars invested. The PVI
can be used to rank projects by following these steps:
• Rank all projects in descending order.
• Allocate the available investment funds to the projects in rank order until all funds are depleted. (It is
assumed that partial investments are possible.)

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 5

 INSIGHTS & APPLICATIONS Year Cash Inflows
1 $ 50,000
Gold Nuggets NPV Analysis of a Project with
Unequal Cash Inflows and Zero Residual Value 2 60,000
3 10,000
Gold Nugget is a large casino located on the east Total cash inflows $120,000
coast.The gaming manager is contemplating pur-
chasing BigWinner, a giant computerized payday Management's cutoff rate (i.e., discount rate or
poker machine that randomly selects lucky win- hurdle rate) for gaming investments is 14 percent;
ners when they cash their paychecks at Gold Nug- NPV analysis is required. The accountant at Gold
get. Big Winner has multiple lighting features and Nugget prepared the following NPV analysis:
sound effects and automatically dispenses prizes, The NPV of the Big Winner project is negative
such as jewelry, gift certificates, show tickets, and $3,260, which means that its return is less than the
so forth, as soon as a lucky winner is announced. 14 percent discount rate. Therefore, management
The machine will cost $100,000 and has zero at Gold Nugget rejected the project.
residual value. It is expected that the machine will
generate the following cash inflows:

Big Winner Project

Period Present Value Cash Present Value Of
Factor (14%) Inflows Cash Inflows
1 .877 $50,000 $ 43,850
2 .769 60,000 46,140
3 .675 10,000 6,750
Total present value of cash inflows $ 96,740
Investment < 100,000>
NPV of project <$ 3,260>

Exhibit 23-4 illustrates how the PVI is used to rank projects. All capital projects will generate a positive
NPV except Project E, which is rejected without further analysis. Investment in the other four projects
requires a total of $65,000, but only $55,000 of investment funds are available. Which capital projects
should be selected? Using the PVI, the projects should be ranked in descending order and funded as shown
in the exhibit. Project D, with the highest PVI of 1.4, is funded first, followed by Project C and Project A.
After these projects are funded, no funds remain and, consequently, Project B is not funded. By funding
these projects, the enterprise achieves a total NPV of $15,000 ($12,000 NPV of D + $1,000 NPV of C +
$2,000 NPV of A + $0 NPV of B). A greater total NPV is not possible given the limited funds available.
The PVI yields the return per investment dollar, and the greater the PVI, the greater the return per dollar
invested. Therefore, selecting the projects with the highest PVI maximizes the NPV.

INSIGHTS & APPLICATIONS The machine has an estimated useful life of four
years and has zero salvage value. Euclid's hurdle
Euclid's Use of the IRR Method rate is 12 percent in the following computations, a
16 percent IRR is tried first. The present value of
Euclid is considering the purchase of a machine the cash inflows at 16 percent is $16,320 larger
for $200,000 that will reduce production costs by than the investment ($216,320 - $200,000). There-
$50,000, $80,000, $100,000, and $90,000 during fore, the IRR is larger than 16 percent. Next, an 18
year 1, year 2, year 3, and year 4, respectively. percent IRR is tried.

IRR = .18 + ( $7,130 / $8,680) (.20-.18)

= .18 + .82 (.02)
= .1964 or 19.64%

Page 6 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

16% 18% 20%

Year Cash Present Value Present Present Present Present Present
inflows factor Value Value factor value Value factor value
1 $ .862 $ 43,100 .847 $ 42,350 .833 $ 41,650
50,000
2 80,000 .743 59,440 .718 57,440 .694 55,520
3 100,000 .641 64,100 .609 60,900 .579 57,900
4 90,000 .552 49,680 .516 46,440 .482 43,380
$216,320 $207,130 $198,450
Present value at 18% $207,130 $207,130
Present value at 20% 198,450
PV of investment 200,000
Difference $ 8,680 $ 7,130

The results show that the 18 percent rate is An approximation of the present value factor
still too low. With a 20 percent IRR, the pres- is calculated as follows:
ent value is $1,550 less than the investment
($200,000 - $198,450). Thus, the true IRR is
between 18 and 20 percent. By interpolating, IRR factor = $335,000 / 100,000
the exact rate is 19.64 percent. The IRR of = 3.350
19.64 percent is much larger than the 12 per- Looking at the table in Exhibit 23-3 for five
cent desired rate of return, or hurdle rate. So, years, the present value for 14 percent is
the proposed investment is very attractive. 3.433, and the factor for 16 percent is 3.274.
Euclid is also considering another capital Thus, the IRR with a factor of 3.350 would be
project of $335,000 that provides uniform very close to 15 percent.
cash inflows of $100,000 for five years with
zero salvage value.

INSIGHTS & APPLICATIONS The NPV of each machine is:

NPV(A) = $14,000 - $10,000 = $4,000
Maxximum Corporation's Financial Analysis of Two
Capital Projects Using the PVI and NPV Methods NPV(B) = $36,000 - $30,000 = $6,000

Maxximum manufactures truck parts. Management is Machine B is preferable under the NPV method. The
considering two new computer-controlled machining PVI for machine A is:
devices with the following present value of cash
flows: ($5,000 + 6,000 + $3,000) / $10,000 = 1.4

PERIOD MACHINE A MACHINE B

0 <$10,000> <$30,000> The PVI for machine B is:

1 5,000 14,000 ($14,000 + $15,000 + $7,000) / $30,000 = 1.2

2 6,000 15,000
3 3,000 7,000 Machine A, however, is preferable under the PVI
method.The PVI indicates that although machine B
has the larger excess of present value over the amount
to be invested, it is not as desirable as machine A in
terms of the amount of present value per dollar
invested.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 7

 RANKING MUTUALLY EXCLUSIVE PROJECTS UNDER LIMITED
INVESTMENT FUNDS
With mutually exclusive projects, accepting one of a set of candidate capital projects causes all the others
to be rejected. By accepting a particular project, the other projects in the set are excluded from further con-
sideration because they would provide unneeded or redundant capability. Acquiring computer A from an
array of computers that perform essentially the same functions is a mutually exclusive project. A replace-
ment project, such as whether to keep an old machine or acquire a new one, is another example. There may
be some differences in capabilities and operating costs between the old and new machines, but if the com-
pany keeps the old machine, it will not acquire the new one; alternatively, if the new one is acquired, the
old one will be sold. Therefore, the two machines are mutually exclusive.
Exhibit 23-2 Ranking Capital Projects with the PVI

Five capital project opportunities

Project Present value of cash Present value of cash PVI
inflows outflows (investment)
A $22,000 $20,000 1.1
B 10,000 10,000 1.0
C 6,000 5,000 1.2
D 42,000 30,000 1.4
E* Reject: 0.9 < 3,600 4,000 0.9*

Three capital projects selected for implementation

Project PVI Investment required Amount funded Cumulative funding
D 1.4 $30,000 $30,000 $30,000
C 1.2 5,000 5,000 35,000
A 1.1 20,000 20,000 55,000
B 1.0 10,000 Not funded -

When investment funds are unlimited, the project with the greatest NPV is selected from a set of mutually
exclusive projects. When investment funds are limited, selecting a project in this manner may not produce
the greatest total NPV.
Exhibit 23-5 illustrates a situation in which managers are evaluating Projects 1, 2, and 3. Projects 1 and 2
are mutually exclusive. Both are uninterruptible power supply systems for the company's computer sys-
tem. Project 3 is a truck, which is an independent project.
If unlimited funds were available, management would select Project 2 over Project 1 and also invest in
Project 3. If only $90,000 of investment funds were available, management would have two choices. It
could invest all its funds in Project 2 with an NPV of $40,000, or it could invest in Projects 1 and 3, which
have a combined NPV of $45,000. Investing in Projects 1 and 3 is the better choice.
It is also interesting to note that if the PVI had been used, Project 2 would have been selected, with a PVI
of 1.44 compared to a PVI of 1.42 for Project 1. Clearly, this is not the wiser choice because the remaining
$30,000 can be put to better use. Therefore, if investments are mutually exclusive, the NPV method may
be a better approach to ranking projects. The PVI indicates the best return per dollar invested, but does not
consider the alternative possibilities of unused funds.
Can a conclusion be drawn about ranking mutually exclusive projects? The problem with mutually exclu-
sive projects is that they may rank differently with each of the discounted cash flow methods. One project
may have the highest NPV, another the highest PVI, while another has the highest IRR. In most situations,
however, the NPV is preferable to either the IRR or the PVI for ranking mutually exclusive projects.

COMPARING NET PRESENT VALUE AND INTERNAL RATE OF RETURN

WHEN ANALYZING MUTUALLY EXCLUSIVE PROJECTS
The choice of the NPV over the IRR is easy to demonstrate with a simple example. Assume an enterprise
must choose between investing $1 that will return $2 in one year or investing $100,000 that will return
$150,000 in one year. The IRRs of the projects are 100 percent and 50 percent, respectively, which makes
the first alternative seem to be the better choice. Assuming the discount rate is 12 percent, the NPVs of the

Page 8 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

projects are $0.79 [($2.00 x 0.893) - $1.00] and $33,950 [($150,000 x 0.893) - $100,000], respectively.
Selecting the first project based on the IRR criterion would lead to a return of $0.79 instead of $33,950.
Therefore, the NPV is the better criterion when choosing between mutually exclusive projects. As the
example shows, a manager concerned with the greatest absolute profitability should choose the project
with the greatest NPV, not the largest IRR. The IRR is a percentage measure of profitability, while the
NPV is an absolute measure.
Exhibit 23-3 Required Investment for Two Mutually Exclusive
Projects and One Independent Project

Project Investment Present value NPV

1 $60,000 $ 85,000 $25,000
2 90,000 130,000 40,000
3 30,000 50,000 20,000

Generally, managers are more comfortable with the IRR method, though, because its results are in per-
centage terms. For example, a manager can say that a 20 percent IRR is desirable because it exceeds a hur-
dle rate of 15 percent and that a 5 percent IRR is undesirable. On the other hand, an NPV of $50,000 may
not be as easy to interpret with respect to a desired discount rate.
The discount rate is the rate used to determine the NPV. If the NPV is zero, the discount rate equals the
IRR. If the NPV is positive, the IRR is greater than the discount rate. If the NPV is negative, the IRR is
less than the discount rate.
The NPV method assumes that cash inflows will be reinvested at the discount rate, while the IRR method
assumes that the cash inflows will be reinvested at the rate earned by the project; that is, at the internal rate
of return. If the project's cash inflows are not reinvested at the IRR, the ranking calculations obtained from
the IRR method may be in error. In many situations, the NPV method may give more reasonable results
because the reinvestment is assumed to be the cost of capital, a more likely scenario.
Under certain conditions, the NPV method indicates that a certain alternative has the highest NPV and
therefore should be selected while the IRR method indicates another project is better because it produces
the highest return. Such a situation occurs because the two methods make different assumptions about the
reinvestment of cash inflows.
Two mutually exclusive capital projects are compared in Exhibit 23-6. The discount rate is 12 percent.
Project A generates the higher NPV, and Project B produces a larger IRR. The NPV method assumes that
cash inflows from Project B will be reinvested to earn 12 percent, the discount rate. Alternatively, the IRR
method assumes that the cash inflows generated by Project B will be reinvested to earn 22.90 percent. If
this is true, then Project B is the better alternative. Finding new capital projects that yield such high rates
of return would be extremely difficult, however.
The problem arises because Projects A and B have unequal useful lives and cash inflows. One approach to
resolving the problem of unequal project lives is to replace Project B at the end of two years with another
project that covers the estimated useful life of Project A so there is a common termination date.

NONDISCOUNTED CASH FLOW METHODS

LEARNING
OBJECTIVE 2

Nondiscounted cash flow methods include the following: Discuss the nondis-
counted cash flow
• Payback period method methods, which are
• Accounting rate of return method the payback period
(PP) method and
These methods ignore the time value of money and, therefore, do not use any present value techniques. the accounting rate
of return (ARR)
Because they ignore the time value of money, many accountants consider these methods to be inferior to method.
the discounted cash flow methods. Nevertheless, the payback period and accounting rate of return meth-
ods should still be studied for two reasons:
Many organizations use these methods. Even in organizations where the use of discounted cash flow
methods has increased, nondiscounted cash flow methods are also used in conjunction with them.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 9

 Perhaps the most important reason to study these methods, however, is that in many economic environ-
ments they can perform better than the more theoretically correct methods using time value of money con-
cepts. The main reason for this is that in many businesses the time horizon is so short that there is no long
run! For example there are very few companies that can tell you with certainty what products they will be
selling 3 years from now. If you cannot state with some certainty what you will be doing in 3 years, it
makes little sense to claim that you can forecast the cashflows for that period. It these cases setting a 2 or 3
year payback period for all investments is easy to understand and actually gives essentially the same
answer to an NPV-type analysis with accurate assessment of distant cashflows.

Exhibit 23-4 Comparing NPV and IRR When Mutually Exclusive Projects Are Involved

Project A Project B
year Cash Present Value factor Present value Year Cash Present Value factor Present
Inflows (12%) inflows (12%) value

1 $2,000 .893 $1,786 1 $6,000 .893 $5,358

2 3,000 .797 2,391 2 3,200 .797 2,550
3 5,000 .712 3,560
4 1,000 .636 636
Present value of cash inflows $8,373 $7,908
Present value of investment <7,000> <7,000>
Net present value $1,373 $908
Internal rate of return 20.96% 22.90%

The internal rates of return for Projects A and B are calculated as fol-
lows:
Project A
20% 22%
Year Cash Present Present Present Present
inflows value factor value value factor value
1 $2,000 .833 $1,666 .820 $1,640
2 3,000 .694 2,082 .672 2,016
3 5,000 .579 2,895 .551 2,755
4 1,000 .482 482 .451 451
$7,125 $6,862

Present value at 20% $7,125 $7,125

Present value at 22% 6,862
Present value of investment 7,000
Difference $ 263 $ 125
Internal rate of return = .20 + ($125 / $263) (.22-.20)
= .20+.48(.02)
= .2096 or 20.96%

THE PAYBACK PERIOD METHOD

The payback period (PP) method measures the number of years needed for a project to accumulate
enough cash to pay for the initial cost of the investment. This span of time is termed the payback period.
The shorter the payback period, the more desirable the investment. The PP method is expressed in years.
The formula used in computing the payback period is:

Page 10 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Project B
22% 24%
Year Cash inflows Present Present value Present value factor Present
value factor value
1 $6,000 .820 $4,920 .806 $4,836
2 3,200 .672 2,150 .650 2,080
$7,070 $6,916
Present value at 22% $7,070 $7,070
Present value at 24% 6,916
Present value of investment 7,000
Difference $ 154 $ 70

Internal rate of return = .22 + ($70 / $154) (.24

- .22)
= .22 + .45 (.02)
= .2290 or 22.90%

INSIGHTS & APPLICATIONS According to the PP method calculations, Metric

should purchase machine A, because it has a
Metric Company's Use of the Payback Period shorter payback period than machine B. But this
may not be the wisest decision because the PP
Metric Company manufactures instrumentation method does not measure the profitability of this
panels for small aircraft. Management is consider- decision, only its liquidity. Additional information
ing two machines, A and B. Machine A costs about the machines under consideration reveals
$323,000 and will reduce annual operating costs that machine A has a useful life of four years and
by $95,000. Machine B costs $396,000 and will machine B has a useful life of eight years. It
reduce annual operating costs by $88,000. If man- would take two purchases of machine A to pro-
agers at Metric acceptor reject capital projects vide the same length of service as a single pur-
based on the PP method, which machine should chase of machine B. Under these circumstances,
be purchased? machine B would be a significantly better invest-
ment than machine A, even though machine A has
a shorter payback period. Machine A's total cash
Machine A payback period = $323,000 / $95,000
inflow is $380,000 ($95,000 annual cash inflow x
= 3.4 years
4 years of useful life), and machine B's total cash
inflow is $704,000 ($88,000 annual cash inflow x
Machine B payback period = $396,000/ $88,000 8 years of useful life). Therefore, machine B will
= 4.5 years give Metric an additional cash inflow of $324,000
based on the difference in the two machines' use-
ful lives. Considering this additional information,
management at Metric should select machine B.

Payback period = Initial investment / Expected annual cash inflows

This method is applied in the above Metric Company case.
When the cash inflows associated with a capital investment are uneven, the results from the payback for-
mula are not directly comparable. Instead, the payback calculations take on a cumulative form. Each
year's cash inflows are accumulated until the amount invested is recovered. This situation is demonstrated
in the Hercules case on the next page.
The major strengths of the PP method, used in both even and uneven cash flow cases, is (1) its simplicity
and (2) that it assists managers in weeding out capital projects not warranting further consideration. For
example, some managers automatically reject a capital project if its payback period is more than five
years. These managers may want a shorter payback period because the shorter the period, the sooner the

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 11

 investment will be recovered and the more accurate the forecasts are likely to be. Thus, the shorter the pay-
back period, the less risk.
The weakness of the PP method is threefold. As already indicated in the Metric case, the PP method does
not measure profitability and ignores cash inflows beyond the payback period. A further criticism of the
PP method is that it does not consider the time value of money. A cash inflow to be received several years
in the future is weighted equally with a cash inflow to be received today. Moreover, by taking a short-term
perspective, management disregards long-term benefits and can place an enterprise in jeopardy with com-
petitors.
Should the PP method be eliminated as a viable capital budgeting technique? Not necessarily. It can be
useful to managers in making capital budgeting decisions so long as the managers fully understand its lim-
itations.

INSIGHTS & APPLICATIONS CASH CUMULATIVE

YEAR INFLOWS CASH INFLOWS
Hercules Construction Company's Payback Period
Analysis 1 $400,000 $ 400,000
2 200,000 600,000
Hercules, a highway construction company, plans to 3 600,000 1,200,000
buy an earth-moving machine costing $1,000,000. It
is estimated that the machine will produce a total cash 4 400,000 1,600,000
inflow of $1,600,000 over four years as follows:
Because $600,000 will be received in the first two
years, only $400,000 will remain to be recovered in
year 3.Therefore, the payback period is two years and
eight months [two years + ($400,000 / $600,000) of
year 3].

THE ACCOUNTING RATE OF RETURN METHOD

The accounting rate of return (ARR) method (also called the unadjusted rate of return and the book
value rate of return) relates the capital project's net income (income after income taxes) to the capital
investment. The ARR is the ratio of the expected average aftertax net income to the investment required to
generate this net income. The ARR is determined as follows:
ARR = Average aftertax net income / Investment (original or average)
The average aftertax net income is determined by adding the aftertax net income for each year of the proj-
ect and then dividing this total by the number of years. Average aftertax net income can be approximated
by subtracting average depreciation from average cash inflow. Assuming that all revenue earned in a
period is collected and that depreciation is the only noncash expense, the approximation is exact.
The amount of investment can be the original investment or the average investment. If average investment
is used, it is calculated as the original investment plus salvage value of the project, if any, divided by two.
The ARR method estimates the revenues that will be generated by the proposed capital project and then
deducts from these revenues all estimated operating costs associated with them including depreciation and
income taxes. The Vulcan case on the next page shows how these calculations are performed. In this case,
average investment is used.
The Vulcan case used the average investment. The Cascade industries case uses the original investment.
Although the ARR method measures profitability, it has a major weakness. It does not consider the time
value of money. With ARR, a dollar received ten years from now is viewed as being just as valuable as a
dollar received today. This defect becomes more significant the further one advances in time.

Page 12 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

ADDITIONAL CAPITAL BUDGETING CONSIDERATIONS

LEARNING
OBJECTIVE 3

Thus far, this chapter has provided basic treatment of discounted and non-discounted cash flow methods. Describe the impact
This section builds on the NPV method by incorporating the following additional considerations: of income taxes,
purchasing versus
leasing, and infla-
tion on capital bud-
geting financial
analysis.

INSIGHTS & APPLICATIONS

Soon after these calculations were made, it was learned
Vulcan Machine Shop's Application of the ARR that the machine would, in fact, have a salvage value of
Method Using Average Investment $30,000. The new annual depreciation is $17,000 [ie.,
($200,000 - $30,000) / 10 years]. The new ARR is:
The superintendent at Vulcan Machine Shop is consid-
ering acquiring an automatic welding machine.This
machine would aid in the fabrication of concrete mix- ARR = [$100,000 - ($68,000 + $17,000)] / [($200,000
ing tanks, the sales of which would increase revenues + $30,000) / 2]
by $100,000 per year. Cash operating costs and income = $15,000 / $115,000
axes would be $68,000 per year. The automatic welding
machine would cost $200,000 and have a ten-year life = 13.04%
with no salvage value. Annual straightline depreciation
expense is $20,000 ($200,000 / 10 years). The ARR for Management at Vulcan rejected the proposal for pur-
this project is computed as: chasing the automatic welding machine because the
ARR = [$100,000 - ($68,000 + $20,000)] / ($200,000 / ARR of 13.04 percent did not meet the minimum
2) desired rate of return of 20.00 percent.
= $12,000 / $100,000
=12.00%

• Income taxes
• Purchasing versus leasing
• Inflation

IMPACT OF INCOME TAXES ON CAPITAL PROJECT DECISIONS

Clearly, aftertax cost is applicable to capital project financial analysis because it measures the actual
amount of cash outflow resulting from expenditures. Therefore, it is necessary to place all financial analy-
ses on an aftertax basis.
USING DEPRECIATION AS A TAX SHIELD. An enterprise does not pay or receive any cash for
depreciation. Depreciation expense, however, provides a tax shield that protects revenues against the pay-
ment of taxes. This tax shielding produces a tax benefit equal to the amount of the depreciation expense
multiplied by the tax rate. As a result the tax laws of each country have an effect on our ability to compute
the size of the “tax shield”.
A particularly interesting example is the Canadian Income Tax treatment of depreciation. Under the Cana-
dian Federal Income Tax code and regulations, fixed assets are placed in pools which are taxed as a pool.
There are, as you might imagine a very large number of rules on how assets are added and removed from
the pools. The effect of this procedure is that the record keeping cost is lowered a great deal since individ-
ual assets have no tax reporting consequence. The difficulty is for decision-makers to compute the after
tax “shield” amount when a given assets “shield” is an incremental effect on a pool. Fortunately Canadian
analysts have developed specialized NPV equations that directly compute the value of this “shield” with-
out separately computing the cash flows. So, Canadian analysts do compute the value of the “shield” even
though they do not directly compute the cashflows themselves. As we conduct more business internation-
ally, it is important to keep in mind that the general model of “identify cash flows—then compute NPV”

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 13

 may not work easily if the cash flows are too difficult to identify, as in the Canadian situation. In those
cases we hope that the local experts have come up with a specialized way to compute the value, and that
we are wise enough to look for it before spinning our wheels on such an analysis.
Back to traditional analysis: For example, if the depreciation expense is $100,000 and the tax rate is 34
percent, then the tax benefit is $34,000 ($100,000 x .34). The impact of the tax shield is illustrated in
Exhibit 23-7. Company D has a depreciation expense; Company E does not.
Notice that Company D's cash inflow exceeds Company E's by $40,800. To determine Company D's cash
inflow, it is necessary to add the $120,000 depreciation deduction back to Company D's net income. This
step is necessary because depreciation expense is a noncash deduction on the income statement. Although
Company D's cash inflow is $40,800 greater than Company E's, its net income is $79,200 lower than Com-
pany E's ($264,000 - $184,800). How can this be? The higher cash inflow occurs because depreciation
expense is acting as a tax shield. The reduction in tax payments, made possible by the depreciation tax
shield, will always be equal to the amount of the depreciation expense deduction multiplied by the tax rate,
as shown by the following formula:

INSIGHTS & APPLICATIONS The machine will have zero salvage value after
the five years, and all revenues earned within a
Cascade Industries Application of the ARR year are collected in that year. The total cash
Method using the Original Investment inflow for the five years is $210,000, making the
average cash inflow $42,000 ($210,000 / 5 years).
A drilling machine requires an initial outlay of Average depreciation is $20,000 ($100,000 / 5
$100,000. The life of the project is five years with years). The average net income is $22,000
the following cash inflows: $40,000, $50,000, ($42,000 - $20,000). Using the average net
$50,000, $40,000, $30,000. income and original investment, the ARR is 22
percent ($22,000 / $100,000). If the average
investments used instead of the original invest-
ment, the ARR is 44 percent ($22,000 / $50,000).

Exhibit 23-5 The Impact of Depreciation Deductions on Tax Payments

Income Statements Company D Company E

Sales $800,000 $800,000
Expenses: Cash operating costs 400,000 400,000
Depreciation expense 120,000 -
Total expenses 520,000 400,000
Net income before taxes 280,000 400,000
Income taxes (34%) 95,200 136,000
Net income $184,800 $264,000

Cash Flow Comparison,

Cash inflow from operations: Net income (from above) $184,800 $264,000
Add-noncash deduction for depreciation 120,000 -
Cash inflow $304,800 $264,000
Greater amount of cash available to Company D $40,800

Tax savings from the depreciation tax shield =

= Tax rate x Depreciation expense deduction
= .34 x $120,000
= $40,800

Page 14 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

CHOOSING DEPRECIATION METHODS. Since the purpose of taxes is to collect money, the
national governments can develop almost any system they want. In most systems there is some attempt to
make things easy on the taxpayer since the less costly the tax system, the more taxes that can be collected.
The US system is a good example of one that is half-way between a financial accounting-type system and
the completely tax centered Canadian version mentioned above. Basically the US system has been slowly
changing over to a more arbitrary, but much (much, much) cheaper to run system based on having the gov-
ernment allow only preset amounts for any asset based on assignment of assets to a very small number of
categories. So, individual asset lives do not need to be assessed (or, audited!). In the following section you
will see an example of the US version of such a mixed system.
Current US tax laws require that the modified accelerated cost recovery system (MACRS) be used for tax
depreciation purposes. MACRS specifies the amount allowable each year as a depreciation deduction
(called “capital recovery” under MACRS). Estimates of future salvage values are ignored under MACRS.
Any salvage or disposal price of the project is taxed at the same rate as ordinary income at the time of the
sale.
As shown in Exhibit 23-8, eight different recovery periods are possible. These recovery periods do not
necessarily reflect the estimated useful life of the assets included in each category. MACRS offers compa-
nies the option of using straight-line depreciation (called the alternative depreciation system, or ADS)
within any recovery period class.

Exhibit 23-6 Modified Accelerated Cost Recovery System (MACRS)

Recovery period Recovery method Examples of projects in recovery period

(years) class
3 200% declining balance ­ Light tools­ Food processing devices
5 200% declining balance ­ Automobiles and light trucks­ Computers,
copiers, typewriters, and calculators
7 200% declining balance ­ Office furniture­ Publishing equipment
10 200% declining balance ­ Barges and tugs­ Oil refining equipment
15 150% declining balance ­ Cement manufacturing equipment­ Sew-
age treatment plants
20 150% declining balance ­ Farm buildings
27.5 Straight-line ­ Residential rental properties
31.5 Straight-line ­ Nonresidential real property
The accelerated depreciation method prescribed under MACRS for most property other than real estate is
Exhibit 23-7 Depreciation Percentages for Three-Year and Five-Year
Property

MACRS Three-Year Property Five-Year Property

33.33% 20.00%
44.45 32.00
14.81 19.20
7.41 11.52
11.52
5.76
the 200 percent declining-balance method with a half-year convention (i.e., only a half-year's depreciation
is allowed in the year of acquisition, and the other half-year is taken in the last year). Exhibit 23-9 lists the
percentages of recovery for three-year property and five-year property. Because of the half-year conven-
tion, an additional year is added on to each property year category; that is, there are four depreciation per-
centages in the three-year category and six in the five-year category.
Exhibit 23-10 illustrates how this works. It is assumed that a company whose tax rate is 34 percent pur-
chases a delivery truck at a cost of $20,000, an estimated useful life of six years, and an estimated residual
value of $4,000. Under MACRS, the delivery truck is a five-year property and is depreciated for tax pur-
poses using the 200 percent declining-balance method with a half-year convention. Under MACRS, esti-

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 15

 mated residual value is ignored. Depreciation expense for federal income tax purposes is calculated at the
right side of the exhibit.
The cash inflow from activities performed by the delivery truck is estimated to be $8,000 annually. The
bottom of the exhibit shows how the present value of the depreciation tax shield resulting from investing in
the delivery truck is calculated. Annual cash savings from the tax shield are multiplied by the appropriate
present value factors to obtain the present value of each annual cash inflow.
The positive NPV of $14,072 shown on the last line indicates that investment in the delivery truck is
expected to return more than the company's required rate of return. It should also be noted that once the tax
effects of the cash flows are computed and included in the calculations, the financial analysis proceeds the
same way as for the methods presented earlier in this chapter. Income taxes are simply one more cash flow
to consider when making a capital budgeting decision.
The choice of recovery method depends on the amount and timing of cash flows. If the enterprise will
enjoy large cash inflows in the early years, the declining-balance method will be more beneficial. If, on the
other hand, an enterprise is experiencing economic difficulties and will have little or no cash inflow in the
early years, the straight-line method is better because it will stretch out the depreciation deductions rather
than accelerate them.
Exhibit 23-8 Impact of Depreciation Tax Shield on Net Present Value

Depreciation tax shield computations

Year Annual Computation deprecia- Tax rate Depreciation
tion % tax shield
1 ($20,000 x 20.00%) $4,000 34 $1,360
2 ($20,000 x 32.00%) 6,400 34 2,176
3 ($20,000 x 19.20%) 3,840 34 1,306
4 ($20,000 x 11.52%) 2,304 34 783
5 ($20,000 x 11.52%) 2,304 34 783
6 ($20,000 x 5.76%) 1,152 34 392
Total $20,000 $6,800

Net present value including the depreciation tax shield

Year Cash flow Present value Present
factor (16%) value
Cash outflow 0 <$20,000> 1.000 <$20,000>
Cash inflow 1-6 8,000 3.685 29,480
Tax shield: 1 $1,360 .862 $1,172
2 2,176 .743 1,617
3 1,306 .641 837
4 783 .552 432
5 783 .476 373
392 .410 161
NPV of cash $14,072
flows

PURCHASING VERSUS LEASING

Capital budgeting investment decisions are judgments about which capital projects will be accepted for
implementation. As already illustrated in this and the previous chapter, a number of methods are available
to assist managers in making such decisions. If these methods produce favorable decisions, then optimal
financing (i.e., acquisition) strategies must be determined. Common financing strategies involve purchase
or lease decisions. Under certain situations, making or constructing the capital project is a third alternative.

Page 16 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

In reality, a lease is just a substitute for a loan. Each lease payment implicitly contains a repayment of a
portion of the loan and an interest charge.
For example, Protex Manufacturing has decided to acquire a computer numerical control machine with a
five-year life at a purchase price of $500,000. Alternatively, the machine can be leased for $140,000 per
year. The project has been accepted from previous NPV analysis. Now the optimal financing strategy-
lease or purchase-must be determined. Protex can negotiate a loan at Central Bank for 10 percent. With a
marginal tax rate of 40 percent, this loan has an aftertax cost of 6 percent [.10 x (1 - .40)]. Why discount
cash flows at 6 percent rather than at Protex's discount rate, which would probably be higher than 6 per-
cent? The company would use the 6 percent rather than the discount rate because investment decisions
(which either accept or reject projects for implementation) and financing decisions are separate. Discount
rates based on weighted-average cost of capital apply to investment decisions but not to financing deci-
sions. The incremental cost of debt is the basic rate for discounting in financing decisions. Thus, in
Exhibit 23-11, both sets of cash flows are discounted at 6 percent. Because the effective aftertax interest
rate of the lease is higher than 6 percent, the purchase option is the wiser choice financially.
Exhibit 23-9 Comparison of Purchasing and Leasing

Purchase
Cost of project $500,000

Less present value of depreciation

tax shield:
Year Annual depre- Tax Deprecia- Present Pres-
ciation rate tion tax value fac- ent
shield tor (6%) value
1 ($500,000 x $100,000 .40 $40,000 .943 $37,720
.200)
2 ($500,000 x $160,000 .40 64,000 .890 56,960
.320)
3 ($500,000 x $96,000 .40 38,400 .840 32,256
.192)
4 ($500,000 x $57,500 .40 23,000 .792 18,216
.115)
5 ($500,000 x $57,500 .40 23,000 .747 17,181
.115)
6 ($500,000 x $29,000 .40 11,600 .705 8,178 <170,511>
.058)
Net cost $329,489
Lease
Present value of aftertax lease payments ($140,000 x .60 x $353,808
4.212)

ADJUSTING FOR INFLATION

Inflation is a decline in the general purchasing power of a monetary unit. Providers of both debt and equity
funds demand higher rates when they expect significant inflation. Similarly, cash flows to be received in
the future are less valuable due to inflation.
The discounted cash flow methods can be adjusted for inflation by increasing the discount rate and the
expected cash flows by a factor to allow for the anticipated inflation. The inflation adjustment is made on
the basis of some specific index that management believes to be applicable to the industry in which the
enterprise operates.
Another option is to project all cash flows in terms of the current year's monetary unit without adjusting
the discount rate or the cash flow projections for inflation. Whichever approach is used, management
should use it consistently. Thus, both cash flows and the discount rate must be adjusted, or neither should
be adjusted.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 17

 It might appear that both approaches will produce the same present value results. However, the results will
be different because depreciation is deductible for tax purposes on the basis of historical cost only, not on
the basis of historical cost adjusted for inflation.
The superior method adjusts both cash flows and the discount rate for inflation, as illustrated in Exhibit 23-
12. Justrite Company is analyzing a machine that will help reduce its costs of quality, especially rework
costs. The discount rate for all capital investments is 15 percent. This rate does not include an adjustment
for inflation, which is expected to average 8 percent over the next five years. Justrite's income tax rate is 40
percent.
The new machine would be placed into service in early 20X4. Costs-of-quality savings are estimated to be
$250,000 annually over the next four years. However, the machine will require additional annual costs of
supplies and power of $10,000 and $40,000, respectively, over the same time frame. Cost savings and
additional costs are in current dollars.
Exhibit 23-10 Adjustment of Cash Flows and Discount Rate for Justrite Company

Schedule showing the net aftertax annual cash inflows adjusted for inflation
20X4 20X5 20X6 20X7
Costs-of-quality savings $250,000 $250,000 $250,000 $250,000
Cost of additional supplies <10,000> <10,000> <10,000> <10,000>
Cost of additional power <40,000> <40,000> <40,000> <40,000>
Net cost savings 200,000 200,000 200,000 200,000
Inflation index x 1.08 x 1.17 x 1.26 x 1.36
Inflation-adjusted net cost savings 216,000 234,000 252,000 272,000
Income taxes (40%) <86,400> <93,600> <100,800> <108,800>
Inflation-adjusted net aftertax cost 129,600 140,400 151,200 163,200
savings
Depreciation tax shield 42,600 42,600 42,600 42,600
Net aftertax cash inflow adjusted $172,200 $183,000 $193,800 $205,800
for inflation

Net present value

Year Net aftertax cash inflows Present value Present value
adjusted for inflation factor (24%)a
0 <$426,000> 1.000 <$426,000>
1 172,200 .806 138,793
2 183,000 .650 118,950
3 193,800 .524 101,551
4 205,800 .423 87,053
Net present value $20,347

a. Discount rate with the inflation factor of 8% is 24%: (1 + .15) (1 + .08) - 1 = .24.

The new machine would be purchased and installed at the end of 20X3 at a net cost of $426,000. If pur-
chased, the machine would be depreciated using the straight-line method for both financial accounting and
tax purposes. The machine would become obsolete in four years and have no salvage value at that time.
Management at Justrite incorporates inflation into capital budgeting decisions by adjusting the expected
cash flows by an industry index. The adjusted aftertax cash flows are then discounted using an adjusted
discount rate. The estimated year-end industry index values for each of the next five years are as follows:

Page 18 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Year Industry Index

20X3 1.00
20X4 1.08
20X5 1.17
20X6 1.26
20X7 1.36
Each element of cost savings is multiplied by the inflation index. The tax shield from the annual deprecia-
tion expense is not multiplied by the inflation factor, however, since this increase in value due to inflation
is not allowed as a deduction by the Internal Revenue Service.

SENSITIVITY ANALYSIS
LEARNING
OBJECTIVE 4

As the previous discussions have shown, estimates are used for various parameters, such as discount rates, Explain how sensi-
cash flows, tax rates, useful lives, salvage values, and inflation indexes. The results of financial analysis tivity analysis
assists managers in
are directly dependent on the parameter values used. Yet, since these parameter values are not known with making capital;
certainty, it is quite possible that a change in a particular parameter's value might significantly affect the budgeting deci-
financial results. Conversely, a change in a particular parameter's value might not affect the results at all, sions.
or very little.
Sensitivity analysis is a process by which managers and management accountants determine how
changes in parameter values affect the financial results. Sensitivity analysis helps managers determine the
amount that parameter values must change before the decision will be affected. It is a what-if process. For
example, sensitivity analysis addresses such questions as what is the effect on the decision to invest in the
candidate project if the cash inflows are 10 percent less than expected and the salvage value is $20,000
more than estimated. Or, what is the effect (or financial results) if the discount rate is 2 percent higher than
expected and the original investment is 14 percent less than estimated? The objective of management
accountants is to present various possible results using numerous parameters so that management can
evaluate and gain insight into a wide range of possibilities. Sensitivity analysis is the tool that enables
management accountants to meet this objective.
The basic idea behind using sensitivity analysis in capital budgeting is to alter input parameter values and
observe the effect on financial results. There are two broad classes of sensitivity analysis:
• Deterministic
• Probabilistic
The deterministic class involves altering parameters to specific or determined values and observing the
specific results. The probabilistic class involves altering parameter values randomly within a specified
probability distribution and observing the probability distribution and statistics (i.e., mean or expected
value, standard deviation, and variance) of the results.

COMMON APPROACHES TO SENSITIVITY ANALYSIS

Four primary approaches are commonly used in sensitivity analysis:
• Graphical
• Spreadsheet
• Dedicated computer programs
• Monte Carlo Simulation
Before any of these approaches can be used, the financial analysis problem must first be defined in terms
of mathematical equations, with specified input parameter values and output results. This collectively is
often referred to as the mathematical model. A mathematical model can be created for any financial analy-
sis situation, as well as for nonfinancial problems such as project timing. As an example, for a simple
NPV analysis:
NPV=Y x P - C
where Y is a yearly annuity amount, P is the present value percentage, and C is the original cost.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 19

 THE GRAPHICAL METHOD. The graphical method works best for simple models where only one or
two input parameter values will be varied deterministically (others are held constant), and where there is a
single output result of interest. Although it is possible to use this method for probabilistic analysis, it is not
recommended.
The objective with the graphical method is to create a set of lines on a graph that represents the result value
for various input values. The lines are typically generated by selecting two or more values of each input
parameter, calculating the result values (by hand, with a calculator, or with a computer), plotting the val-
ues, and connecting the points.
The resulting shape and relation of the lines can tell managers a lot about the sensitivity of the parameters.
Relatively flat lines indicate relative insensitivity, while sharply sloped lines indicate greater sensitivity. In
other words, with a steeply sloping relationship, a small change in the input parameter value will cause a
large change in the result. Further, lines grouped closely together indicate a relative insensitivity to
changes in the parameter. Examples of these concepts are shown in Exhibit 23-13. It is important to real-
ize, though, that the scale of the graph is very important when making relative comparisons of slopes and
distances between lines. Caution is advised.
The previous example of Tahoe Ski Lodge's NPV calculation can be used to illustrate the graphical
method.
THE SPREADSHEET METHOD. The spreadsheet method is most appropriate for financial analysis
problems that are too complex for the graphical method, but are simple enough to “program” into a com-
puter spreadsheet. This method simply uses the ability of computer spreadsheets to recalculate results
quickly for changes in any input parameter value. The effects of changes in input parameters can be
quickly observed in any of the output result values.
When using spreadsheets, the previous results are “erased” when new values are entered unless you learn
to use the “scenario” management part of the spreadsheet. Scenario management will save you a great deal
of time. If not, it is important to keep a record of the results and input values-either in a separate part of the
spreadsheet itself (which may lend itself to quicker plotting or reviewing when finished) or by hand.

Exhibit 23-11 Examples of Possible Sensitivity Relationships with the Graphical Method

Slightly
Different values of Different values of
Parameter 2 Parameter 2
Result Value
Highly

Different values of Parameter 1 Different values of Parameter 1

Different values of Different values of

Parameter 2 Parameter 2
Result Value
Slightly

Different values of Parameter 1 Different values of Parameter 1

Although most often used for the deterministic class, computer spreadsheet add-on products can be pur-
chased that will perform probabilistic analysis. The first step in using these programs is to define the prob-
ability distribution for all variable input parameter cells in the spreadsheet and then to specify the output

Page 20 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

cells. The program does the necessary repetitive calculations, storing output cell results and displaying
them as probability distribution plots when finished.

INSIGHTS & APPLICATIONS These values were then plotted as shown in Exhibit 23-
14. Note that the x-axis is the yearly increased cash
Sensitivity Analysis of Tahoe Ski Lodge's NPV Calcula- inflows-from $130,000 to $190,000-and the three lines
tion represent the three possible residual values. By choos-
ing a residual value and a yearly cash flow value, the
As previously given, the expected additional yearly NPV can be read on the y-axis.
cash inflows for a new ski lift are $160,000. The lift
would cost $500,000 and have a useful life of five years
and a salvage value of $40,000. These values result in Examination of the graph indicates that the NPV is very
an NPV of $42,880 at a discount rate of 16 percent. sensitive to the yearly revenue, but not particularly sen-
sitive to changes in the residual value. Since the project
can more rapidly achieve a $0 NPV for higher values of
Management at Tahoe Ski Lodge, however, is uncertain yearly increased cash inflows, management should
of the yearly increased cash inflows, thinking that they focus further analysis effort on refining the yearly cash
could be as high as $180,000 or as low as $140,000. inflow estimates and expend less effort on refining the
Also, management believes the residual value of the lift residual value estimate.
could be $0, $40,000, or $65,000. With these determin-
istic values, NPVs were calculated, resulting in the
table below.

Lift Cost Yearly Cash Residual Npv

Inflows value
$500,000 $140,000 $ -0- $ <41,640>
500,000 160,000 -0- 23,840
500,000 180,000 -0- 89,320
500,000 140,000 40,000 <22,600>
500,000 160,000 40,000 42,880
500,000 180,000 40,000 108,360
500,000 140,000 65,000 <10,700>
500,000 160,000 65,000 54,780
500,000 180,000 65,000 120,260
THE DEDICATED COMPUTER PROGRAM METHOD. The dedicated computer program
method is used for large complex problems with many input parameters and many desired output results.
These programs are often custom designed for individual company requirements (e.g., future price fore-
casts, inflation forecasts, corporate tax structure, unique financial analysis methods), and used consistently
throughout the enterprise.
THE MONTE CARLO SIMULATION METHOD. The Monte Carlo Simulation method is a tech-
nique for probabilistic sensitivity analysis that is used in a wide variety of disciplines, including account-
ing, project management (illustrated in the next chapter), and scientific research. It can be used in any area
where a mathematical model exists and input values can be derived from a probability distribution. The
method involves randomly choosing input parameter values (usually more than one) based on specified
probability distributions, performing the model calculations, and recording the output values. This process
is typically performed thousands of times to ensure the accuracy of the simulation.
The accuracy of a Monte Carlo Simulation is greatly influenced by the number of iterations performed. As
a general rule, the more iterations, the more accurate the results will be. Typically, the iterations continue
until the random input parameter value distribution is representative of the desired probabilistic distribu-
tion and the output probability distributions are stable. Stability of the distributions is measured by exam-
ining the change in the mean, standard deviation, and variance of the distribution after each iteration.
When the changes in these statistics become marginally small, the Monte Carlo iterations can stop. Due to
the usually large number of iterations required for stability, Monte Carlo Simulations can require signifi-
cant computation time, and the more complex simulations are routinely performed on the fastest super-

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 21

Exhibit 23-12 Calculations and Plots of the Tahoe Ski Lodge Case

140
120 Residual value = $ 65 000

net Present Value [thousands $]

100 Residual value = 40 000

80 Residual value = $ 0

60
40

20
0

-20
-40
-60
130 140 150 160 170 180 190
yearly Increased cash inflows [thousands $]

computers, though small and moderate simulations can be adequately and quickly performed on personal
computers.

SUMMARY OF LEARNING OBJECTIVES

The major goals of this chapter were to enable you to achieve four learning objectives:

Learning objective 1. Discuss the discounted cash flow methods, and explain the net
present value (NPV), internal rate of return (IRR), and present value index (PVI)
methods.
Discounted cash flow methods consider the time value of cash flows by discounting them to their present
value using a discount rate based on the weighted-average cost of capital. The following capital budgeting
financial analysis methods are based on discounted cash flow concepts:
• Net present value (NPV)
• Internal rate of return (IRR)
• Present value index (PVI)
The NPV technique calculates the expected net financial gain or loss from a capital project by discounting
all estimated cash inflows and outflows to the present, using some predetermined discount rate. The IRR
method calculates the rate at which the present value of estimated cash inflows from a capital project
equals the present value of estimated cash outflows of the capital project. The PVI method calculates a
ratio that compares the present value of cash inflows to the present value of the investment outlay. Because
more profitable capital projects have higher indexes, an enterprise can rank its independent capital projects
according to the PVI indexes.
The NPV, IRR, and PVI methods assist managers in deciding if capital projects are worthwhile financially.
The decision rules for these methods are as follows:
Method Condition Decision
NPV >$0 Accept
IRR >hurdle rate Accept
PVI > 1.0 Accept
It is often argued that NPV is the most reasonable method, but many people who want to relate returns to a
percentage figure find IRR easier to understand.

Learning objective 2. Discuss the nondiscounted cash flow methods, which are the
payback period (PP) method and the accounting rate of return (ARR) method.

Page 22 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Nondiscounted cash flow methods do not consider the time value of money. The following nondiscounted
cash flow methods are widely used:
• Payback period (PP)
• Accounting rate of return (ARR)
The PP method calculates the amount of time needed to recoup the initial investment that the capital proj-
ect requires. The ARR method divides the original or average investment into the estimated average
annual income after depreciation and income taxes.
A summary of the capital budgeting financial analysis methods appears in Exhibit 23-15.

Learning objective 3. Describe the impact of income taxes, purchasing versus leas-
ing, and inflation on capital budgeting financial analysis.
Unless an enterprise is a tax-exempt organization, such as a government entity, income taxes should be
considered in capital budgeting financial analysis. Tax-deductible cash expenditures must be converted to
an aftertax basis by multiplying the expenditure by (I - Tax rate). Only the aftertax amount is used in deter-
mining the desirability of a capital project. Similarly, taxable cash inflows must be placed on an aftertax
basis by multiplying them by the same formula.
Although depreciation deductions do not involve a cash outflow, they represent tax deductions. These
deductions shield income from taxation, resulting in less taxes being paid. These savings in income taxes
are calculated by multiplying the depreciation deduction by the tax rate itself. Because accelerated depre-
ciation methods provide the largest amount of tax shield early in the life of depreciable capital projects,
they are normally superior to the straight-line method of depreciation. On the other hand, the straight-line
method would be appropriate for start-up companies or companies that expect to experience low income
or losses over the next several years.
A company that plans to acquire a capital project must determine how it will finance its acquisition and
implementation. Two common methods used to finance acquisitions are purchasing and leasing. If a com-
pany purchases the project, funds can come from equity investment, debt, or other sources. When leasing,
funds are provided by the lessor at some cost. Thus, the cost of leasing can be compared to the cost of
owning to determine the least expensive method of financing an acceptable project.
Inflation is rampant in some countries. Inflation makes a dollar received in the future less valuable than a
dollar on hand today. Inflation is generally incorporated into capital budgeting financial analysis by
including an inflation factor in the discount rate. This inflation-adjusted discount rate is then used to esti-
mate the future cash flows over the life of the project.

Learning objective 4: Explain how sensitivity analysis assists managers in making

capital budgeting decisions.
All capital investment decisions involve risk. Management can never be absolutely sure that the right cap-
ital projects are selected for implementation. This will not be known with any degree of certainty until
several postimplementation audits are conducted. So, what can management accountants do to help deal
with this risk and increase the level of certainty?
Up to this point, the management accountant has already set up a systematic way for management to par-
ticipate in the capital budgeting methodology. In addition, promising capital projects were subjected to
various financial analysis methods, such as the NPV and PVI. Conducting sensitivity analysis provides
management with still another way to gain insight into the capital investment decisions that must be made.
In almost any decision-making process, conducting sensitivity analysis will enable the situation to be eval-
uated more thoroughly so that a better decision can be made.

IMPORTANT TERMS
Accounting rate of return (ARR) method (unadjusted rate of return and book value rate of return)
Calculates the return from a capital project by dividing the average annual net income (after
depreciation and income taxes) by the average investment or initial investment.
Annuity A series of equal cash flows (either inflows or outflows) per period.
Dedicated computer program method Customized software package for conducting specialized sensi-

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 23

 Exhibit 23-13 Summary of Advantages and Disadvantages of Capital Budgeting Financial Analysis
Methods

Advantages Disadvantages
Net Present Value (NPV)
­ Considers time value of money. ­ Discount rate may not be valid.
­ Considers cash flows over the entire life of the ­ Timing and size of cash flows may not be reliable.
project.
­ When projects are mutually exclusive, NPV is ­ Difficult for some people to understand.
generally the superior method to use in selecting
the best project.
­ It is assumed that if the shorter-lived of two projects
is selected, the cash inflows of that project will con-
tinue to earn the discount rate of return through the life
of the longer-lived project.
­ If projects being compared involve different dollar
amounts of investment, the project with more profit-
able dollars may not be the better project if it also
requires a larger investment.
Internal Rate of Return (IRR)
­ Considers time value of money. ­ Hurdle rate may not be valid.
­ Considers cash flows over the entire life of the ­ Timing and size of cash flows may not be reliable.
project.
­ The percentage figure enables a reasonable rank- - Difficult to calculate.
ing of projects that require different initial cash out-
lays and have unequal lives.
­ Results in terms of a percentage may be more - It is assumed that if the shorter-lived of two projects
meaningful to managers than the NPV or PVI. is selected, the cash inflows of that project will con-
tinue to earn the IRR through the life of the longer-
lived project (generally not a reasonable assumption).
•Projects are ranked for funding using the IRR rather
than the absolute dollar return.
Present Value Index (PVI)
­ Same as NPV, except that it simplifies ranking of an ­ Same as NPV.
optimum set of independent projects when the total ­ Gives relative answer but does not reflect absolute
capital budget is limited. dollars of NPV.
­ It can be misleading on mutually exclusive projects
that have different total initial investments.

Payback Period (PP)

- It is simple to calculate and understand. ­ It ignores the time value of money (discounted
payback helps overcome this problem).
- It signals the level of risk, i.e„ shorter payback means ­ It ignores cash flows that may occur beyond the
lower risk. payback period.
- It may be used to select those capital projects yield- - It fails to consider salvage value that may exist
ing a quick return of cash, thus placing an emphasis on after the payback period.
liquidity.
­ It does not favor profitable projects with longer
payback periods.
tivity analysis.

Page 24 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Accounting Rate of Return (ARR)

Data for its calculation are relatively easy to - It ignores the time value of money.
obtain from the financial accounting system.
­ It considers income over the entire life of the - It depends on averaging techniques that
project. may yield inaccurate results, particularly
when cash flows are unequal throughout a
project's life.
Deterministic class involves altering parameters to specific or determined values and observing the spe-
cific results.
Discount rate (hurdle rate) Expected return on the capital funds invested at a given level of risk.
Discounted cash flow (DCF) methods The NPV, IRR, and PVI methods that deter-mine the present value
of future cash flows.
Graphical method A simple method of performing sensitivity analysis where only one or two input
parameter values are varied deterministically.
Independent projects Capital projects that have no significant bearing on one another.
Internal rate of return (IRR) method (time-adjusted rate of return) The rate of return at which the
present value of the net cash inflows minus the investment in the capital project equals zero.
Monte Carlo Simulation method A technique used to perform probabilistic sensitivity analysis in a wide
variety of disciplines.
Mutually exclusive projects A set of candidate projects from which one is accepted.
Net present value (NPV) method The total cash inflows less the total cash outflows discounted by the
discount rate to their current value.
Nondiscounted cash flow methods The payback period (PP) method and accounting rate of return
(ARR) method.
Payback period (PP) method Measures how long in years it takes to recover in cash inflows an amount
equal to the initial investment.
Present value index (PVI) method (profitability index [PI] and cost/benefit ratio) The ratio of the
present value of a capital project's net cash inflows to the initial investment required.
Probabilistic class Involves altering parameter values randomly within a specified probability distribu-
tion and observing the probability distribution and statistics.
Sensitivity analysis A process by which managers and management accountants determine how changes
in parameter values affect the financial results.
Spreadsheet method Used to conduct sensitivity analysis, usually on a deterministic class of parameters.
Tax shield A reduction in the amount of taxable income resulting from a depreciation deduction (or other
deductions). The reduction in tax is calculated by multiplying the depreciation deduction by the
prevailing tax rate.
Time value of money The value attributed to money as a result of its ability to earn a return over time. A
dollar today is worth more than a dollar in the future, and the longer one waits for a dollar, the
more uncertain the receipt is.
Weighted-average cost of capital A composite of the cost of the various sources of funds that comprise
an enterprise's capital structure. It is the minimum rate of return that must be earned on new
investments so as not to dilute shareholder interests.

DEMONSTRATION PROBLEMS
DEMONSTRATION PROBLEM 1 Comprehensive analysis.
Primerate Bank is considering the purchase of a local area network (LAN) for $800,000 that will reduce
operating costs by $300,000, $400,000, $500,000, and $600,000 during 20X4, 20X5, 20X6, and 20X7,
respectively. The LAN has an estimated life of four years with no salvage value. Primerate uses straight-
line depreciation. The income tax rate is 40%, and Primerate's desired rate of return on capital project
investments is 14%. The aftertax increase in net income and the cash flow from the proposed LAN invest-
ment are as follows:
Required:
a. Calculate the internal rate of return (IRR) and give a brief description of it.
b. Calculate the net present value (NPV) and give a brief description of it.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 25

 20X4 20X5 20X6 20X7
Increase in net income before $300,000 $400,000 $500,000 $600,000
taxes and depreciation
Depreciation <200,000> <200,000> <200,000> <200,000>
Net increase before taxes 100,000 200,000 300,000 400,000
Taxes (40%) <40,000> <80,000> <120,000> <160,000>
Net increase in net income after 60,000 120,000 180,000 240,000
taxes
Depreciation 200,000 200,000 200,000 200,000
Cash savings $260,000 $320,000 $380,000 $440,000

c. Calculate the payback period and give a brief description of it.

d. Apply the payback period (PP) method in a way that will overcome its weakness of ignoring the time
value of money.
e. Calculate the accounting rate of return (ARR) and give a brief description of it.
SOLUTION TO DEMONSTRATION PROB-
LEM
Prime Rate Bank
Internal Rate Of Return
Lan Project
22% 24% 26%
Year Cost Sav- Present Value Present Present Present Present Present
ings factor Value Value factor value Value factor value
1 $260,000 .820 $213,200 .806 $209,560 .794 $206,440
2 320,000 .672 215,040 .650 208,000 .630 201,600
3 380,000 .551 209,380 .524 199,120 .500 190,000
4 440,000 .451 198,440 .423 186,120 .397 174,680
$836,060 $802,800 $772,720

Present value at 24% $802,800 $802,800

Present value at 26% 772,720
Present value of invest- 800,000
ment
Difference $ 30,080 $ 2,800

IRR = .24 + $ 2,800 / $30,080 (.26 -

.24)
= .24 + .09(.02)
= .2418 or 24.18%
The IRR is the actual discounted rate of return expected to be earned on the LAN. The process for comput-
ing the rate when the cash inflows are not uniform is based on trial and error. Various rates are tried until
the correct one is found, usually by interpolation. The rate is correct when the present value of cash inflows
is equal to the present value of cash outflows (e.g., investment).
If the IRR is greater than the discount rate (or hurdle rate), then the LAN is accepted. In this case, the
return is 24.18%, which is much larger than the bank's discount rate of 14%.
b.
Under the NPV method, the future cash inflows are discounted at the discount rate of 14%. The difference
between the present value of the cash inflows and the present value of cash outflows (i.e., the investment)
represents the NPV. The NPV of the LAN project is $191,080 and is accepted.
c.

Page 26 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Prime Rate Bank

Net Present Value
Lan Project
Present value of cash
inflows:
Year Cost Savings Present Value Present
Factor (14%) Value
1 $260,000 .877 $228,020
2 320,000 .769 246,080
3 380,000 .675 256,500
4 440,000 .592 260,480
Total present value of cash $991,080
inflows
Present value of cash outflow: Invest- <800,000>
ment, beginning of 20X4
Net present value $191,080

Year Cash Cumulative Years

Inflows Cash Inflows Needed
1 $260,000 $ 260,000 1.00
2 320,000 580,000 1.00
3 380,000 960,000 .58
4 440,000 1,400,000 -
2.58
Percentage of year needed = Cash needed to pay back
investment / Cash inflow for year
= ($800,000 investment - $580,000 cumulative cash
flows) / $380,000
= .58 of a year
The payback period is the time it takes for the cumulative sum of the cash inflows to equal the initial cash
outlay (i.e., the investment). For Primerate, the payback period for the LAN project is 2.58 years.
The PP method is widely used because it is easily calculated and understood. It has two weaknesses, how-
ever. First, it fails to measure profitability by ignoring all cash flows after the end of the payback period.
Second, it does not consider the time value of money.
d.
Primerate Bank
Payback Period using Discounted Cashflows
lan Project
Year Cash Present Value Present Cumulative
Inflows Factor (14%) Value Cash Inflows
1 $260,000 .877 $228,020 $228,020
2 320,000 .769 246,080 474,100
3 380,000 .675 256,500 730,600-

*Payback period using a 14% discount rate = Amount needed / Total cash inflow
in year 4 = (($800,000 - $730,600)/ $260480) + 3 years = 3.27 years

This calculation eliminates the criticism that the payback period necessarily ignores the time value of
money. Using the 14% discount rate and the present value of the cash inflows, the payback period is 3.27
years.
e.
Under the ARR method, the expected annual net income is divided by the investment. The net income is
the average net cash inflows minus depreciation.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 27

 Primerate Bank
Accounting Rate Of Return
Lan Project
ARR = (Average cash inflow - Depreciation) / Investment

where:
Average cash inflow =( $1,400,000 / 4 years) = $350,000
per year
Depreciation = $800,000 / 4 years = $200,000 per year

Therefore, the ARR using the original investment is:

ARR = ($350,000 - $200,000) / $800,000 = 18.75%
The ARR using the average investment as the denominator
is:

ARR = (Average cash inflow - Depreciation) / (Investment

/ 2)
= ($350,000 - $200,000) / ($800,000 / 2)
= 37.50%
The ARR can be calculated by dividing the average cash inflow by the original investment or by the aver-
age investment (investment plus salvage value divided by 2).
The ARR does not account for the time value of money. It is an averaging method, and if cash flows are
not uniform during the life of the project, the degree of error can he substantial.
DEMONSTRATION PROBLEM 2 The tax effects of MACRS.
The Comstock Company is considering purchasing a machine costing $10,000. The useful life of the
machine is five years. Comstock's discount rate is 10%. Its income tax rate is 40%.
Required: Calculate the NPV under the straight-line and MACRS methods using a half-year convention.
SOLUTION TO DEMONSTRATION PROBLEM 2
The depreciation tax shield is the amount by which income taxes are reduced because of depreciation
expense. Calculating it is similar to calculating the tax effect of any deductible expense:
Depreciation tax shield = Annual depreciation deduction x Tax rate
Straight-Line Method
Year Depreciation Tax Rate Tax Shield Present Value Present
Expense Factor (10%) Value
1 $1,000 .40 $ 400.00 .909 $ 363.60
2 2,000 .40 800.00 .826 660.80
3 2,000 .40 800.00 .751 600.80
4 2,000 .40 800.00 .683 546.40
5 2,000 .40 800.00 .621 496.80
6 1,000 .40 400.00 .564 225.60
Net pres- $2,894.00
ent value

As can be seen from this analysis, the NPV of the tax shield (i.e., tax savings) from MACRS is greater than
that from the straight-line depreciation method.
DEMONSTRATION PROBLEM 3 The lease-versus-purchase decision.

Page 28 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Macrs Method
Year Depreciation tax rate tax shield Present Value Present
expense factor (10%) value
1 $2,000 .40 $ 800.00 .909 $ 727.20
2 3,200 .40 1,280.00 .826 1,057.28
3 1,920 .40 768.00 .751 576.77
4 1,152 .40 460.80 .683 314.73
5 1,152 .40 460.80 .621 286.16
6 576 .40 230.40 .564 129.95
NPV $3,092.09

Mercy Hospital's management accountant, Mary Balinsky, has just finished performing an NPV analysis
for a $1,090,000 CAT scanner with a five-year useful life. Top management has agreed with Mary's rec-
ommendation and has asked her to analyze the alternative financing available.
After careful analysis, Mary has narrowed the financing of the project to two alternatives. The first alter-
native is a lease agreement with the manufacturer of the CAT scanner. The manufacturer is willing to lease
the scanner to Mercy Hospital for five years. The lease agreement calls for Mercy to make annual pay-
ments of $250,000 at the beginning of each year.
The second alternative would be for Mercy to purchase the scanner outright from the manufacturer for
$1,090,000. The hospital can claim an investment tax credit of $90,000 if it purchases the scanner. Prelim-
inary negotiations with Mercy's bank indicate that the hospital would be able to finance the scanner acqui-
sition with a 10% term loan.
If the scanner is purchased, Mercy plans to depreciate the scanner over five years using the straight-line
method. Salvage value is zero.
All maintenance, taxes, and insurance costs are the same under both alternatives and are paid by Mercy.
Mercy is subject to a 40% income tax rate.
Required:
a. Calculate the relevant present value cost of the purchasing alternative.
b. Calculate the relevant present value cost of the leasing alternative.
c. Financially, which is the better alternative?
SOLUTION TO DEMONSTRATION PROBLEM 3
a.Purchase
Cost of project = = (cash outflow of $1,000,000 - $90,000 x present value factor of 1.000) =$1,000,000
Demonstration Problem 3 part b. Less pres-
ent value of depreciation tax shield:
Present 1,000,00
Value 0
Year Annual Tax Deprecia- Present Pres-
Deprecia- rate tion tax Value Fac- ent
tion Shield tor (10%) value
1 $200,000 .40 $80,000 .909 $72,720
2 200,000 .40 80,000 .826 66,080
3 200,000 .40 80,000 .751 60,080
4 200,000 .40 80,000 .683 54,640
5 200,000 .40 80,000 .621 49,680 <303,200
>
Net present value of pur- $ 696,800
chase
b See table following.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 29

 Lease
Time After tax Cost of Lease Pay- Present Value factor (10%) Present
ment value
0 ($250,000 x .60) = $150,000 1.000 $ 150,000
1-4 ($250,000 x .60) = $150,000 3.170 475,500
Net present value of lease $ 625,500

c. The lease alternative is better financially because the NPV (i.e., cost) of this alternative is $71,300
($696,800 - $625,500) less than the NPV (cost) of the purchase alternative.
DEMONSTRATION PROBLEM 4 The graphical method of sensitivity analysis. As presented in the
text, the expected additional yearly revenue for a new automatic welding machine for Vulcan Machine
Shop is $100,000. The yearly cash operating costs and income taxes are estimated at $68,000. The
machine would cost $200,000, have a ten-year life, and have a salvage value of $30,000. A straight-line
depreciation method is used. Management at Vulcan is uncertain of the yearly revenue, however, thinking
that it could be as high as $125,000 or as low as $90,000. Also, management believes the salvage value of
the machine could be $0, $30,000, or $50,000.
Required:
a. Create the mathematical model and perform a sensitivity analysis.
b. Show the results in graphical form.
c. Comment on the results.
SOLUTION TO DEMONSTRATION PROBLEM 4 a. The mathematical model for this problem is:
ARR = R - (OT + DE) / ((C+S) / 2)
where:
• R = Increased yearly revenue
• OT = Yearly increased operating costs and taxes
• C = Cost of the machine
• S = Salvage value of the machine
• DE = Yearly depreciation expense calculated by DE = (C-S) / L
• L = Life (years) of the machine

Calculating The Various Scenarios Results In The Follow-

ing Table:
Machine Revenues Costs Sal- ARR
Cost And vage
Taxes Value
$200,000 $ 90,000 $68,000 $_0_ 2.00
200,000 100,000 68,000 -0- 12.00
200,000 125,000 68,000 -0- 37.00
200,000 90,000 68,000 30,000 4.35
200,000 100,000 68,000 30,000 13.04
200,000 125,000 68,000 30,000 34.78
200,000 90,000 68,000 50,000 5.60
200,000 100,000 68,000 50,000 13.60
200,000 125,000 68,000 50,000 33.60

b. Note that the x-axis is the yearly revenue-from $80,000 to $130,000-and the three lines represent the
three possible salvage values. By choosing a salvage value and a yearly revenue value, the ARR can be
read on the y-axis.

Page 30 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Salvage value $ 0
35

25
APR %

15 Salvage value $ 30 000

Salvage value $ 50 000

65 95 105 115 125

Yearly revenue increase [thousands $]

c. Examination of the graph indicates that the ARR is very sensitive to the yearly revenue, but not particu-
larly sensitive to changes in the salvage value. Since the project can achieve the minimum desired rate of
20% for high values of yearly revenue, management should focus further analysis effort on refining the
yearly revenue estimates and expend less effort on refining the salvage value estimate.
It can also be seen that the three lines are not parallel. This is a direct result of the formulation of the math-
ematical model. Since the salvage value is used in two different places, changes in this value will cause
the slope to change.
DEMONSTRATION PROBLEM 5 The spreadsheet method of sensitivity analysis. Management at
Secure System is considering acquiring a machine that will manufacture a new automobile security
device. The machine will cost $200,000 with a useful life of four years and no salvage value. There is
some management disagreement as to the amount of net cash inflows the machine will produce. Estimates
are $50,000, $70,000, and $90,000 annually. Cash inflows for each year are equal. Also, management
believes the project should be analyzed assuming discount rates of 8, 10, and 12%, respectively.
Required: Use sensitivity analysis to compute the NPV under each assumption.
Solution To Demonstration problem 5
Useful Dis- Initial Present Value Of Cash Net Pres-
life count Cash Out- Inflows ent Value
rate flow
4 8% $200,000 $165,600 = ($50,000 x 3.312) <$34,400>
4 10 200,000 158,500 = ($50,000 x 3.170) < 41,500>
4 12 200,000 151,850 = ($50,000 x 3.037) < 48,150>
4 8 200,000 231,840 = ($70,000 x 3.312) 31,840
4 10 200,000 221,900 = ($70,000 x 3.170) 21,900
4 12 200,000 212,590 = ($70,000 x 3.037) 12,590
4 8 200,000 298,080 = ($90,000 x 3.312) 98,080
4 10 200,000 285,300 = ($90,000 x 3.170) 85,300
4 12 200,000 273,330 = ($90,000 x 3.037) 73,330

Note: This type of problem is especially applicable to the spreadsheet method.

DEMONSTRATION PROBLEM 6 Sensitivity analysis with Monte Carlo Simulations.
Zero Luggage Company is planning to introduce a new line of titanium briefcases. The estimated develop-
ment costs are $110,000 with net cash inflows estimated at $85,000 and $50,000 for the two-year life of
the product line. Management, knowing that these values could vary, performed further analysis and
derived the following probability distributions.
Required: Assuming a discount rate of 14%:
a. Calculate the NPV for the original estimates.
b. Using Monte Carlo Simulation, calculate the mean of the iterations and the probability of the NPV

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 31

 being lower than $0.
c. Compare the results from Requirements (a) and (b) and comment. Make any assumptions you deem nec-
essary.:
Development Costs Year 1 prob- Cash Year 2 prob- Cash
probability ability inflows ability inflows
0.1 $150,000 0.2 $100,000 0.2 $65,000
0.3 120,000 0.4 90,000 0.4 60,000
0.5 105,000 0.2 80,000 0.2 40,000
0.1 65,000 0.2 65,000 0.2 25,000

SOLUTION TO DEMONSTRATION PROBLEM 6 a. The mathematical equation for the NPV is:
NPV = (0.877 x Y1) + (0.769 x Y2) - D
where:
• NPV = Net present value
• Y1 = First-year cash inflow
• Y2 = Second-year cash inflow
• D = Initial development costs
Using this equation, the original NPV would be:
NPV = (0.877 x $85,000) + (0.769 x $50,000) - $110,000 NPV = $2,995
b. The Monte Carlo sensitivity analysis was simplified by assuming step functions for each of the probabil-
ity distributions. Then, a table of ten values for each parameter was created by using the probabilities. For
example, for the development costs parameter, one of the ten values would be $150,000, three of the values
would be $120,000, and so forth, resulting in the following:
Development costs Year 1 cash Inflows Year 2 cash Inflows
$150,000 $100,000 $65,000
120,000 100,000 65,000
120,000 90,000 60,000
120,000 90,000 60,000
105,000 90,000 60,000
105,000 90,000 60,000
105,000 80,000 40,000
105,000 80,000 40,000
105,000 65,000 25,000
65,000 65,000 25,000

Then, for each Monte Carlo iteration, obtaining a random number for each parameter becomes a simple
process of selecting one value from each column randomly. After obtaining the random parameter values,
the NPV calculation was done. The NPV was saved for each iteration, and a cumulative mean value was
calculated. The iterations were stopped when the cumulative mean value did not vary more than $250 and
the cumulative variance did not vary more than $62,500 ($250 x $250) from iteration to iteration.
In all, 347 iterations were required, with the total number of NPVs below $0 being 151. Thus, the probabil-
ity for the NPV being below $0 is:
Probability = 151 / 347 = 43.5%
The mean of the NPVs was $2,117.10.
The 43.5% probability that the project would have a negative NPV, even though the mean estimates show a
positive NPV, might cause management to require further refinement of the parameter estimates and prob-
ability distributions before approving the project.
c. The mean values from Requirements (a) and (b) are somewhat different. This can be attributed to the
limited number of iterations required to meet the cumulative mean and variance change limits. In a com-
puterized Monte Carlo system, with a significantly smaller difference limit of $50, a total of 665 iterations
were required for stabilization. The cumulative mean was $3,003.53-very close to the results in (a)-and the

Page 32 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

probability that the NPV would be less than $0 was 44.1 %, not much of a change from that calculated in
(b).

REVIEW QUESTIONS
23.1 The second stage of the capital budgeting methodology deals with:
a. Decisions affecting high-tech companies.
b. Financial analysis of long-term decisions.
c. Financial analysis of short-term decisions.
d. Capital asset tracking systems.
23.2 Present value is:
a. The sum of cash inflows discounted to time zero.
b. The sum of cash outflows discounted to time zero.
c. Both (a) and (b).
d. None of the above.
23.3 An enterprise's relevant weighted-average cost of capital:
a. Should be the same as the prime rate.
b. Should be unaffected by the enterprise's capital structure.
c. Is a weighted average of the enterprise's required future returns on debt and equity.
d. Is a weighted average of common stock less paid-in capital.
23.4 The basis for measuring the cost of capital derived from debt and preferred stock, respectively, is the
a. Pretax rate of interest for debt and stated annual dividend rate for preferred stock.
b. Aftertax rate of interest for debt and stated annual dividend rate for preferred stock.
c. Aftertax rate of interest for debt and stated annual dividend rate less the expected earnings per
share for preferred stock.
d. Pretax rate of interest for debt and stated annual dividend rate less the expected earnings per
share for preferred stock.
23.5 The discount rate ordinarily used in present value calculations is:
a. The prime rate.
b. The savings bond rate.
c. The Federal Reserve rate.
d. The desired rate of return set by management.
23.6 Net present value is:
a. The sum of discounted cash inflows plus discounted cash outflows.
b. The sum of discounted cash outflows.
c. The sum of discounted cash inflows less the sum of discounted cash outflows.
d. The sum of discounted cash inflows.
23.7 The IRR and NPV methods usually produce identical rankings of candidate projects. Under certain
conditions, however, dissimilar rankings can occur. When such differences occur, the method
normally selected is:
a. NPV because all reinvestment of funds occurs at the discount rate based on the cost of capital
and because it takes into account the relative size of the original investment.
b. IRR because all reinvestment of funds occurs at the discount rate that will make the NPV of
the project equal to zero.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 33

 c. NPV because all reinvestment of funds occurs at the discount rate that will make the NPV of
the project equal to zero.
d. IRR because all reinvestment of funds occurs at the rate of the cost of capital and because it
takes into account the relative size of the original investment.
23.8 A manager at Coretop Company has questioned the accuracy of the NPV method because the present
value factors are based on the assumption that cash flows occur at the end of the year. She argues
that the cash flows actually occur uniformly throughout each year. What is your response to her?
a. The NPV is totally accurate.
b. The NPV is slightly understated but usable.
c. The NPV gives a consistent 10% error of the estimate.
d. The NPV is slightly overstated but usable.
23.9 A company is considering the purchase of a conveyor belt that will link to one of its major customers
for just-in-time delivery of parts. The system will cost $900,000 and will have a life of seven
years. It will provide a number of benefits, such as reduced transportation and storage costs,
reduced handling costs, and increased revenue. The company's discount rate is 12%. What
method should the management accountant select to evaluate the cost/benefit relationship of this
capital project?
a. CVP analysis.
b. Regression analysis.
c. EOQ analysis.
d. NPV or IRR analysis.
23.10 Which of the following is an assumption regarding NPV computation?
a. The life of all capital projects is less than the payback period.
b. Reinvestment of the cash inflows will be made at the discount rate.
c. All capital projects will generate long-term profits.
d. The life of all projects is greater than five years.
23.11 Which of the following is a method of capital selection that considers the time value of money?
a. IRR method.
b. ARR method (based on original investment).
c. ARR method (based on average investment).
d. Linear programming.
23.12 The IRR method, as contrasted with the NPV method:
a. Is considered better for analyzing lease financing.
b. Is considered inferior because it fails to calculate compounded interest.
c. Assumes that the rate of return on the reinvestment of the cash inflows is at the indicated rate
of return of the project analyzed rather than at the discount rate.
d. Uses average net income over the project's life.
23.13 Which of the following methods implies that cash inflows are reinvested at the rate of return earned
by the capital project investment?
a. ARR method.
b. NPV method.
c. IRR method.
d. PVI method.
23.14 The present value index (PVI) is also called the cost/benefit ratio (or benefit/ cost ratio). What are
the benefits? Discuss what role Chapter 22 plays in providing data necessary to use the PVI or
cost/benefit ratio, as well as the NPV, IRR, PP, and ARR methods.

Page 34 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

23.15 The PVI is:

a. Another term for profitability index (PI).
b. The ratio of the present value to the original investment.
c. Both (a) and (b).
d. None of the above.
23.16 When the PVI for a project equals one:
a. The NPV equals zero.
b. The present value of project returns is less than the present value of the project cost.
c. The IRR is less than the discount rate.
d. The payback period is equal to one year.
23.17 A candidate project has an expected life of five years. In the computation of the NPV of the candi-
date project, salvage value would be:
a. Included as a cash inflow at the future amount of the estimated salvage value.
b. Included as a cash inflow at the present value of the estimated salvage value.
c. Included as a cash inflow at the estimated salvage value.
d. Excluded from NPV's computation.
23.18 Which of the following capital budgeting financial analysis methods assumes that funds are rein-
vested at the firm's cost of capital?
a. NPV method.
b. IRR method.
c. ARR method.
d. PP method.
23.19 An advantage of using the PP method of evaluating capital projects is that:
a. It considers the time value of money.
b. It is easy to apply and managers readily understand it.
c. It incorporates inflation.
d. It considers the full life of the project.
23.20 Deficiencies associated with using the PP method to evaluate capital projects include:
a. The present value of cash inflows is ignored.
b. Cash inflows of different time periods are treated equally.
c. Disproportionate weight is given to cash flows occurring in the future.
d. Cash flows after the payback period are ignored.
e. All of the above.
23.21 The PP method measures:
a. The total profitability of the project.
b. How quickly investment dollars may be recovered.
c. The cash inflow generated by the project's salvage value.
d. The economic life of an investment.
23.22 Which of the following capital budgeting financial analysis methods has been criticized because it
fails to consider investment profitability?
a. NPV method.
b. IRR method.
c. PP method.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 35

 d. ARR method.
23.23 A major criticism of the PP method is that it doesn't consider the time value of money. Explain how
this criticism can be overcome.

23.24 Depreciation is incorporated in the discounted cash flow methods because it:
a. Reduces the cash outlay for income taxes.
b. Represents the initial cash outflow spread over the life of the project.
c. Represents a hedge against inflation.
d. Represents cash outflow that cannot be avoided.
23.25 Which of the following best describes the effect that changing from straight-line to accelerated
depreciation will have on the financial analysis of a capital project?
a. The calculations will be invalid because only the straight-line depreciation method can be used
for tax purposes.
b. The risk of the proposed investment will be larger than if the straight-line depreciation method
is used.
c. The NPV of the proposed investment will be larger than if the straight-line depreciation
method is used.
d. The cash inflows in each period after income tax effects will be smaller than with the straight-
line depreciation method.
23.26 When applying one of the discounted cash flow methods to evaluate the desirability of a capital proj-
ect, which of the following factors is generally not considered?
a. The impact of inflation.
b. The impact of income taxes.
c. The timing and quantity of cash flows.
d. The method of financing the project.

CHAPTER-SPECIFIC PROBLEMS
These problems require responses based directly on concepts and techniques presented in the text.
23.27 Weighted-average cost of capital. Excello Company is in the process of reengineering its activities
based on activity-based management (ABM) analyses. The projects contained in the Capital Projects Port-
folio Statement that support the reengineered activities involve nearly equal risk. They are independent of
each other so that Excello may invest in a single project, any combination, or all of them. The capital out-
lay for each project is as follows:
Project A $ 800,000
Project B 200,000
Project C 900,000
Project D 400,000
Total $2,300,000

Excello intends to maintain a capital structure of 40% long-term debt and 60% equity, of which 80% is
common stock and 20% retained earnings. The weighted-average cost of capital is 9% when historical val-
ues are used.
The expected annual cost of generating earnings available for the capital projects is 12%. Excello can issue
long-term bonds at an annual interest rate of 10%. Issuance of common stock will cost 20%. Excello is
subject to a 40% income tax rate.
Required:

Page 36 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

a. Explain why new capital investments should be evaluated on the basis of future weighted-
average cost of capital rather than a weighted-average cost of capital based on historical values.
b. Calculate the weighted-average cost of capital Excello should use in its discounted cash flow
analysis.
23.28 Using the PVI and NPV methods. Seth Milam has collected the following data during the first
stage of the capital budgeting methodology. These data relate to Projects Alpha and Beta, which are of
equal risk.
Required:
Which of the projects would be selected using the PVI and NPV methods?
Present Value Of Cash Flows
Year Alpha Beta
0 <$10,000> <$30,000>
1 4,550 13,650
2 4,150 12,450
3 3,750 11,250

23.29 Capital rationing. Arco has the opportunity to invest in the following independent projects:
Project Required investment Npv
A $ 80,000 $2,000
B 100,000 1,000
C 40,000 1,200
D 80,000 1,500
E 92,000 2,200

Although Arco would like to invest in all five projects, it has only $240,000 of capital funds available.
Required: Using the NPV and PVI methods, determine the combination of projects that produces the
greatest value to Arco's stockholders. Assume that the projects permit partial investment. Which method,
the NPV or PVI, would you use to ration the capital funds of $240,000? Justify your answer.
23.30 Calculating the payback period given unequal cash inflows. A machine costing$2,000 produces
total cash inflows of $3,000 over four years as follows:
Year After tax cash Inflows Cumulative cash flows
1 $600 $600
2 800 1,400
3 1,000 2,400
4 600 3,000

Required:
Calculate the payback period.
23.31 Calculating the payback period and accounting rate of return. [AICPA adapted] Hanley Company
purchased a machine for $125,000. The machine will be depreciated $25,000 each year for five years. At
the end of five years, it will have zero salvage value, The related cash flow from operations, net of income
taxes, is expected to be $45,000 annually. Hanley's income tax rate is 40% for all years.
Required:
a. Calculate the payback period.
b. Calculate the accounting rate of return.
23.32 Calculating the payback period given equal cash inflows. Tarmack Company is considering the
purchase of a machine for $350,000 with a life of five years and a salvage value of $50,000. The machine
will be depreciated using the straight-line method. (Ignore the half-year convention.) The machine is
expected to produce cash inflows from operations, net of income taxes, of $100,000 annually in each of
the next five years.
Required: Calculate the payback period.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 37

 23.33 Calculating and comparing all the financial analysis methods. Dynameg Corporation is trying to
decide whether to select a mainframe-based centralized information system or a network-based distributed
information system. Both systems will perform the same data processing tasks. Based on various tangible
and intangible benefits, the annual cash inflows produced by these benefits are estimated to be $200,000
for the mainframe system and $100,000 for the network system. The original investment will require
$500,000 for the mainframe and $217,000 for the network. The network has a life of four years and the
mainframe five years.
Dynameg's discount rate is 10%. Neither capital project will have a salvage value at the end of its useful
life. Both capital projects will be depreciated using the straight-line method. For the following calcula-
tions, ignore income taxes and the half-year convention.
Required:
a. Calculate the NPV for each investment.
b. Calculate the IRR (approximations are acceptable) for each investment.
c. Calculate the PVI for each investment.
d. Calculate the payback period for each investment.
e. Calculate the ARR for each investment. Use the original investment as the denominator.
f. Which project should be selected for implementation? Justify your answer.
23.34 Considering income taxes. [CMA adapted] Rockyford Company must replace some machinery,
This machinery has zero book value, but its current market value is $1,800. One possibility is to invest in
new machinery that will cost $40,000. This new machinery would produce estimated annual pretax operat-
ing cash savings of $12,500.
Assume the new machinery will have a useful life of four years and have depreciation of $10,000 each
year for book and tax purposes. (Ignore the half-year convention.) It will have no salvage value at the end
of four years. The investment in this new machinery would require an additional investment in working
capital of $3,000.
If Rockyford accepts this investment proposal, the disposal of the old machinery and the investment in the
new equipment will take place on December 31 of this year. The cash flows from the investment will occur
during the next four calendar years.
Rockyford is subject to a 40% income tax rate for all ordinary income and capital gains and has a 10%
aftertax cost of capital. All operating and tax cash flows are assumed to occur at year-end.
Required:
a. Calculate the present value of the aftertax cash inflow arising from the disposal of the old machinery.
b. Calculate the present value of the aftertax cash inflows for the next four years attributable to the operat-
ing cash savings.
c. Calculate the present value of the depreciation tax shield at the end of year 1.
d. Rockyford's additional investment in working capital of $3,000 required in the current year is:
1. A sunk cost that is not recovered.
2. Considered part of the original investment when determining the NPV.
3. An item requiring amortization.
4. Spread over the four-year life of the asset as a cash outflow.
23.35 Calculating the net present value under a depreciation tax shield. Virtual Optical Disk Company is
considering the purchase of a laser device for $400,000 that will reduce operating costs by $150,000,
$200,000, $250,000, and $300,000 in years 1, 2, 3, and 4, respectively. Its useful life is four years, and Vir-
tual uses the sum-of-the-years-digits (SYD) depreciation method. Virtual's income tax rate is 40%, and its
discount rate is 12%.
Required: Calculate the NPV of the laser device using the SYD method. Then calculate the NPV using the
straight-line method. Which depreciation method produces the greater NPV and by how much?
23.36 Selecting projects. Mercken Industries is contemplating four projects, D, E, F, and G. The capital
costs for the initiation of each project and its estimated aftertax cash inflows are listed below. Mercken's
desired aftertax opportunity cost is 12%, and the company has a capital budget for the year of $450,000.
Idle funds cannot be reinvested at greater than 12%.

Page 38 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Project Project E Project F Project

D G
Initial Cost $200,00 $235,000 $190,00 $210,00
0 0 0
Annual Cash Flows
Year 1 $56,500 $90,000 $45,000 $40,000
Year 2 56,500 85,000 55,000 50,000
Year 3 56,500 75,000 65,000 60,000
Year 4 56,500 55,000 70,000 65,000
Year 5 56,500 50,000 75,000 75,000
NPV $3,683 $29,845 $27,345 <$7,845>
IRR 12.8% 17.6% 17.2% 10.6%
PVI 1.02 1.13 1.14 0.96
Required:
a. Which projects should Mercken select?
b. If Mercken was able to accept only one project, which one would it select? [CMA adapted]
23.37 Discounting cash flows using MACRS. On January 1, 20X4, Crane Comany will acquire a new
asset that costs $400,000 and is anticipated to have a salvage value of $30,000 at the end of four years.
The new asset:
• Qualifies as three-year property under the modified accelerated cost recovery system (MACRS).
• Will replace an old asset that currently has a tax base of $80,000 and can be sold now for $60,000.
• Will continue to generate the same operating revenues as the old asset ($200,000 per year). However,
savings in operating costs will be experienced as follows: a total of $120,000 in each of the first three
years and $90,000 in the fourth year.
Crane is subject to a 40% tax rate and rounds all computations to the nearest dollar. Assume that any gain
or loss affects the taxes paid at the end of the year in which it occurred. The company uses the NPV
method to analyze projects using the following factors and rates:

Period Present Value of $1 At 14% Present Value Of $1 Annuity At 14% Macrs

1 .88 .88 33%
2 .77 1.65 45
3 .68 2.32 15
4 .59 2.91 7

Required:
a. What is the present value of the depreciation tax shield for the 20X7 MACRS depreciation of Crane's
new asset?
b. What is the discounted net-of-tax amount that should be factored into Crane's analysis for the disposal
transaction?
c. What are the relevant discounted operating cash flows that should be factored into Crane's analysis?
[CMA adapted]
23.38 Calculating the tax shield and payback period for a new machine. Britelite Company is consider-
ing purchasing a new machine that will cost $120,000 and will have annual depreciation for tax purposes
of $24,000 for five years. Cash inflows of $40,000 annually will be generated by the new machine.
Required: If the tax rate is 40%, what is the payback period for the new machine?
23.39 Considering mutually exclusive projects and income tax consequences. [CMA adapted] Garrison
Corporation is considering the replacement of an old machine that is currently being used. The old
machine is fully depreciated but can be used by the corporation through 20X7. If Garrison decides to
replace the old machine, Picco Company has offered to purchase it for $60,000 on the replacement date.
The old machine would have no salvage value in 20X7.
If the replacement occurs, a new machine would be acquired from Hillcrest Industries on January 2, 20X3.
The purchase price of $1,000,000 for the new machine would be paid in cash at the time of replacement.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 39

 Due to the increased efficiency of the new machine, estimated annual cash savings of $300,000 would be
generated through 20X7, the end of its expected useful life. The new machine is not expected to have any
salvage value at the end of 20X7.
All operating cash receipts, operating cash expenditures, and applicable tax payments and credits are
assumed to occur at the end of the year. Garrison employs the calendar year for reporting purposes. Fol-
lowing are additional assumptions:
• Garrison requires all investments to earn a 12% aftertax rate of return to be accepted.
• The new machine will have depreciation as follows:
Year Depreciation
20X3 $ 250,000
20X4 380,000
20X5 370,000
$1,000,000

Required:
a. Calculate the present value of the aftertax cash inflow associated with the salvage value of the old
machine.
b. Before consideration of any depreciation tax shield, calculate the annual after-tax cash savings that arise
from the increased efficiency of the new machine throughout its life.
c. Calculate the present value of the depreciation tax shield for 20X4.
d. Assume that the new machine has a salvage value of $80,000 on December 31, 20X7, instead of zero
salvage value. Calculate the present value of the additional aftertax cash inflow.
23.40 Calculations of capital budgeting financial analysis methods. [CMA adapted] Hazman Company
plans to replace an old piece of equipment that is obsolete and expected to be unreliable under the stress of
daily operations. The equipment is fully depreciated and will have no salvage value.
One piece of equipment being considered would provide annual cash savings of $7,000 before income
taxes. The equipment would cost $18,000 and have annual depreciation of $3,600 for five years, for both
book and tax purposes. It would have no salvage value at the end of five years.
The company is subject to a 40% tax rate and has a 14% aftertax cost of capital. Assume all operating reve-
nues and expenses occur at the end of the year.
Required:
a. Calculate the aftertax payback period.
b. Calculate the aftertax ARR.
c. Calculate the aftertax NPV.
d. Calculate the aftertax PVI.
e. Calculate the aftertax IRR.
23.41 Analyzing alternative financing arrangements. [CMA adapted] Crown Corporation has agreed to
sell some used computer equipment to Bob Parsons, one of the company's employees, for $5,000. Crown
and Parsons have been discussing alternative financing arrangements for the sale and the present and
future values of each alternative.
Required:
Following are alternative financing arrangements:
a. Crown Corporation has offered to accept a $1,000 down payment and set up a note receivable for Par-
sons that calls for four $1,000 payments at the end of each of the next four years. If Crown uses a
6% discount rate, what would be the present value of the note receivable?
b. Parsons has agreed to the immediate down payment of $1,000 but would like the note for $4,000 to be
payable in full at the end of the fourth year. Because of the increased risk associated with the
terms of this note, Crown would apply an 8% discount rate. What would be the present value of
this note?
c. If Parsons borrowed the $5,000 at 8% interest for four years from his bank and paid Crown the full price
of the equipment immediately, Crown would invest the $5,000 for three years at 7%. What would
be the future value of this investment?

Page 40 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

23.42 Analyzing the lease-versus-purchase decision. LeToy Company produces a wide variety of chil-
dren's toys, most of which are manufactured from stamped parts. The Production Department recom-
mended that a new stamping machine be acquired. The Production Department further recommended that
the company consider using the new stamping machine only for five years. Top management has con-
curred with the recommendation and has assigned Ann Mitchum of the Budget and Planning Department
to supervise the acquisition and to analyze the alternative financing available.
After careful analysis and review, Mitchum has narrowed the financing of the project to two alternatives.
The first alternative is a lease agreement with the manufacturer of the stamping machine. The manufac-
turer is willing to lease the equipment to LeToy for five years even though it has an economic useful life of
ten years. The lease agreement calls for LeToy to make annual payments of $62,000 at the beginning of
each year. The manufacturer (lessor) retains the title to the machine, and there is no purchase option at the
end of five years. Investment credit is claimed by the lessor and does not flow through to LeToy (lessee).
This agreement would be considered a lease by the Internal Revenue Service.
The second alternative would be for LeToy to purchase the equipment outright from the manufacturer for
$240,000. LeToy can claim an investment tax credit of $16,000 if it purchases the equipment. Preliminary
discussions with LeToy's bank indicate that the firm would be able to finance the asset acquisition with a
15% term loan.
LeToy would depreciate the equipment over five years using the sum-of-the-years-digits method. The
market value of the equipment at the end of five years would be $45,000; consequently, LeToy would use
a salvage value of $45,000 for depreciation purposes.
All maintenance, taxes, and insurance are the same under both alternatives and are paid by LeToy. LeToy
requires an aftertax cutoff return of 18% for investment decisions and is subject to a 40% corporate
income tax rate on both operating income and capital gains and losses.
Required:
a. Calculate the relevant present value cost of the leasing alternative for LeToy Company.
b. Calculate the relevant present value cost of the purchase alternative for LeToy Company.

THINK-TANK PROBLEMS
Although these problems are based on chapter material, reading extra material, reviewing previous chap-
ters, and using creativity may be required to develop workable solutions.
23.43 Comprehensive discussion of capital budgeting financial analysis methods. [CMA adapted]
Plasto Corporation is a manufacturer of plastic products. The company is embarking on a five-year mod-
ernization and expansion plan. Thus, management is identifying all of the capital projects that it should
consider. Financial analyses will be prepared for each identified project. Plasto will not select and imple-
ment all of the projects because some may not be financially attractive and some are mutually exclusive.
In addition, not all projects can be implemented because capital funds are limited.
All modernization and expansion projects would be completed in three years. The projects have varying
lives, but none exceed seven years. Plasto's criteria for evaluating and selecting projects are maximization
of return and quickness of investment recovery. The projects included in the Capital Projects Portfolio
Statement are as follows:
Required:
a. When attempting to determine if a project is profitable and should be implemented, Plasto should be
sure that the project's:
1. Return exceeds a hurdle rate specified by Plasto's management.
2. Return exceeds the interest rate that is charged on any debt that is incurred to finance a capital project.
3. Return exceeds the company's historical return on stockholders' equity.
4. Payback is three years or less.
b. The overriding concern for Plasto's maintenance project should be to:
1. Minimize the IRR.
2. Minimize the present value of cash outlays.
3. Maximize the payback period.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 41

 Project Identification and Description Investment Estimated life
in Years
• Maintenance: Extensive maintenance of current manu- $ 2,000,000 3
facturing facilities, including repairs and some replace-
ment of equipment. This work must be completed in
order to keep existing facilities in operation until reengi-
neering and expansion projects are completed.
•Reengineering: Major reengineering of current manu- 6,000,000 5
facturing activities using general-purpose equipment.
•Reengineering: Major reengineering of current manu- 8,500,000 5
facturing activities using special-purpose equipment
(this project and the prior project are mutually exclu-
sive).
•Make versus buy: Construction of new facilities to 5,000,000 7
manufacture parts and supplies used in making prod-
ucts. Parts and supplies are currently being purchased.
•Expansion: Construction of new facilities to introduce 10,000,000 7
new product NX-42.
•Expansion: Construction of new facilities to introduce 12,000,000 7
new product LV-221.
4. Minimize the PVI.
c. Which of the following pairs of capital budgeting financial analysis methods would best satisfy Plasto's
criteria for selecting capital projects?
1. ARR and present value payback.
2. NPV and PP.
3. IRR and present value payback.
4. ARR and IRR.
d. If Plasto is faced with capital rationing, the best general method of ranking the projects would be:
1. Present value payback.
2. PVI.
3. NPV.
4. IRR.
5. Payback.
e. If Plasto must decide between the two mutually exclusive reengineering projects, the best general
method of deciding between these projects would be:
1. Present value payback.
2. PVI.
3. NPV.
4. IRR.
5. Payback
23.44 Analyzing mutually exclusive projects. The Beta Corporation manufactures office equipment and
distributes its products through wholesale distributors.
Beta Corporation recently learned of a patent on the production of a semiautomatic paper collator that can
be obtained at a cost of $60,000 cash. The semiautomatic model is vastly superior to the manual model that
the corporation now produces. At a cost of $40,000, the present equipment could be modified to accommo-
date the production of the new semiautomatic model. Such modifications would not affect the equipment's
remaining useful life of four years or its salvage value of $10,000. Variable costs, however, would increase
by $1 per unit. Fixed costs, other than relevant amortization charges, would not be affected. If the equip-
ment is modified, the manual model cannot be produced.
The current income statement relative to the manual collator appears as follows:
Market research has disclosed three important findings relative to the new semiautomatic model. First, a
particular competitor will certainly purchase the patent if Beta does not. If this were to happen, Beta's sales

Page 42 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Sales (100,000 units @ $4) $400,000

Variable costs $180,000
120,000
Fixed costsa
Total costs <300,000>
Net income before income taxes 100,000
Income taxes (40%) <40,000>
Net income after income taxes $ 60,000

a.All fixed costs are directly allocatable to the production of the manual collator and include
depreciation on equipment of $20,000, calculated on the straight-line basis with a useful life of
ten years.

of the manual collator would fall to 70,000 units per year. Second, if the selling price is not increased, Beta
could sell approximately 190,000 units per year of the semiautomatic model. Third, because of the
advances being made in this area, the patent will be completely worthless at the end of four years.
Because of the uncertainty of the current situation, the raw materials inventory has been almost com-
pletely exhausted. Regardless of the decision reached, substantial and immediate inventory replenishment
will be required. The Engineering Department estimates that if the new model is to be produced, the aver-
age monthly raw materials inventory will be $20,000. If the old model is continued, the inventory balance
will average $12,000 per month.
Required:
a. Prepare a schedule that shows the incremental aftertax cash flows for comparison of the two alterna-
tives. Assume that Beta will use the sum-of-the-years-digits method for depreciating the costs of modify-
ing the equipment. (Ignore MACRS and the half-life convention.)
b. Using the cash inflows in Requirement (a), if Beta has a cost of capital of 18%, will it decide to manu-
facture the semiautomatic collator?
Period Present value of Interest Factors present For 18% accumu- Accumulated value Of $1
$1 value of $1 per Period lated value of $1 per Period received At
received At end of Period end Of Period
1 .85 .85 1.18 1.00
2 .72 1.57 1.39 2.18
3 .61 2.18 1.64 3.57
4 .52 2.70 1.94 5.21

c. Calculate the ARR for each project. Using this method, would you recommend that Beta manufacture
the semiautomatic collator? Explain.
d. What additional analytical methods, if any, would you consider before presenting a recommendation to
management? Why?
e. What concerns would you have about using the information given in the problem to reach a decision in
this case? [CMA adapted]
23.45 Financing decision. HMG Corporation is a for-profit health care provider, which operates three
hospitals. One of these hospitals, Metrohealth, plans to acquire new X-ray equipment that management
has already decided will be cost-beneficial and will enhance the technology available in the outpatient
diagnostic laboratory. Before Metrohealth submits a purchase requisition for the equipment to corporate
headquarters, Paul Monden, Metrohealth's management accountant, has to prepare an analysis comparing
financing alternatives.
The new equipment is a Supraimage X-ray 400 machine priced at $1,000,000, including shipping and
installation, and would be delivered January 1, 1984. Its annual depreciation expense will be $400,000,
$240,000, $144,000, $108,000, and $108,000, respectively, over five years. The machine will have no sal-
vage value at the end of five years.
Metrohealth is considering the following financing alternatives:
• Finance internally. HMG Corporation would provide Metrohealth with the funds to purchase the equip-
ment. The supplier would be paid on the day of delivery.
• Finance with a bank loan. Metrohealth could obtain a bank loan to finance 90% of the equipment cost at
10% annual interest with five annual payments of $237,420 each due at the end of each year, with the

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 43

 first payment due on December 31, 20X4. The loan amortization schedule follows. Metrohealth would
provide the remaining $100,000, which would be paid upon delivery.
Year Beginning balance Payment Interest Principal reduction
1 $900,000 $237,420 $90,000 $147,420
2 752,580 237,420 75,258 162,162
3 590,418 237,420 59,042 178,378
4 412,040 237,420 41,204 196,216
5 215,824 237,420 21,582 215,838

• Lease from a lessor. The equipment could be leased from MedLeasing with an initial payment of $50,000
due on equipment delivery and five annual payments of $220,000 each, commencing on December 31,
20X4. At the option of the lessee, the equipment can be purchased at the fair market value at lease termi-
nation (the lessor is currently estimating a 30% residual value). The lease satisfies the requirements of an
operating lease for both FASB and income tax purposes. Due to expected technological changes in med-
ical equipment, Metrohealth is not planning to purchase the X-ray equipment at the end of the lease com-
mitment.
Both HMG Corporation and Metrohealth have an effective income tax rate of 40%, an incremental bor-
rowing rate of 10%, and an aftertax corporate hurdle rate of 12%. Income taxes are paid at the end of the
year. Present value tables for several interest rates are as follows:
Present Value Of $1 Present Value Of An Annuity Of $1
Received At The end Of The Received at The End Of Each period (Ordi-
Period nary)
PERIOD 6% 10% 12% 20% 6% 10% 12% 20%
1 .94 .91 .89 .83 .94 .91 .89 .83
2 .89 .83 .80 .69 1.83 1.74 1.69 1.53
3 .84 .75 .71 .58 2.67 2.49 2.40 2.11
4 .79 .68 .64 .48 3.47 3.17 3.04 2.59
5 .75 .62 .57 .40 4.21 3.79 3.61 2.99
Required:
a. Prepare a present value analysis as of January 1, 20X4, of the expected aftertax cash inflows for each of
the three financing alternatives available to Metrohealth for acquiring the new X-ray equipment. As part of
your present value analysis:
1. Justify the discount rate(s) you employed.
2. Identify the financing alternative that is most advantageous to Metrohealth.
b. Discuss the qualitative factors Paul Monden should include for management consideration before a final
decision is made regarding the financing of this new equipment. [CMA adapted]
23.46 Comprehensive lease analysis. On December 29, 20X4, Dallas Corporation leased
20 trucks to Reutzel Express Company under a six-year, noncancelable lease.
Additional information regarding the lease and the trucks follows:
• The lease requires equal payments of $145,200 that are due on December 31 each year, and the first rent
was paid December 31, 20X4. These payments provide Dallas Corporation with a 12% return on the net
investment; this implicit interest rate is known by Reutzel.
• The lease does not pass ownership of the trucks at the end of the lease, but Reutzel may purchase all of
the trucks at the end of the lease for a total of $10,000. The estimated residual value of all of the trucks is
$25,000 at the end of the lease term and $1,000 at the end of nine years.
• The fair value of the trucks is $674,000. The cost of the trucks to Dallas is $650,000, and each truck has
an expected useful life of nine years.
• Reutzel's incremental borrowing rate is 14%.
• Reutzel pays the executory costs (insurance, taxes, and other fees not included in the annual lease pay-
ments) of $485 per year and depreciates all of its trucks using straight-line depreciation.
• The collectibility of the lease payments is reasonably predictable, and there are no important uncertain-
ties surrounding the amount of unreimbursable costs yet to be incurred by Dallas.
• Present value factors for 12% are as follows:

Page 44 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Period Present Value of $1 Present Value of Present Value

Received at The An Ordinary of An Annuity
End of the Period Annuity of $1 due of $1
6 0.51 4.11 4.60
9 0.36 5.33 5.97

Present value factors for 14% are as follows:

Period Present Value of $1 Present Value of Present Value
Received at The End of the An Ordinary of An Annuity
Period Annuity of $1 due of $1
6 0.46 3.89 4.43
9 0.31 4.95 5.64
Required:
a.
1. Explain the four criteria used to determine that a lease should be accounted for as a capital lease by the
lessee.
2. Identify which of these criteria are met by the lease between Dallas and Reutzel.
b. In general, what are the advantages of leasing to Reutzel?
c. Without prejudice to your response to previous requirements, assume that Reutzel recorded the lease on
December 31, 20X4, as a capital lease in the amount of $673,300. Prepare all the journal entries required
to record the capital lease on the books of Reutzel for the fiscal year ended December 31, 20X5.
d. Explain how the lease should be presented on Reutzel's Statement of Financial Position dated December
31, 20X5. (Do not include footnote disclosure.) [CMA adapted]
23.47 Determining whether to make or buy. Jonfran Company manufactures three different models of
paper shredders including the waste container, which serves as the base. Although each model uses a dif-
ferent shredder head, the waste container is the same. The number of waste containers that Jonfran will
need during the next five years is estimated as follows:
20X4 50,000
20X5 50,000
20X6 52,000
20X7 55,000
20X8 55,000

The equipment used to manufacture the waste container must be replaced because it has broken and can-
not be repaired. The new equipment would have a purchase price of $945,000 with terms of 2/10, n/30;
company policy is to take all purchase discounts. The freight on the equipment would be $11,000, and
installation costs would total $22,900. The equipment would be purchased in December 20X3 and be
placed into service on January 1, 20X4. It would have a five-year economic life and would be treated as
three-year property under the modified accelerated cost recovery system (MACRS). This equipment is
expected to have a salvage value of $12,000 at the end of its economic life in 20X8. The new equipment
would be more efficient than the old equipment, resulting in a 25% reduction in both direct materials and
variable overhead. The savings in direct materials would result in an additional onetime decrease in work-
ing capital requirements of $2,500 due to a reduction in direct materials inventories. This working capital
reduction would be recognized at the time of equipment acquisition.
The old equipment is fully depreciated and is not included in the fixed over-head. The old equipment from
the plant can be sold for a salvage amount of $1,500. Jonfran has no alternative use for the manufacturing
space at this time, so if the waste containers are purchased, as discussed next, the old equipment would be
left in place.
Rather than replace the equipment, one of Jonfran's production managers has suggested that the waste
containers be purchased. One supplier has quoted a price of $27 per container. This price is $8 less than
Jonfran's current manufacturing cost, which is as follows:
Jonfran employs a plantwide fixed overhead rate in its operations. If the waste containers are purchased
outside, the salary and benefits of one supervisor, included in the fixed overhead at $45,000, would be

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 45

 Direct materials $10
Direct labor 8
Variable overhead 6
Fixed overhead: Supervision $2
Facilities 5
General 4 11
Total manufacturing cost per unit $35

eliminated. There would be no other changes in the other cash and noncash items included in fixed over-
head, except depreciation on the new equipment.
Jonfran is subject to a 40% income tax rate. Management assumes that all annual cash flows and tax pay-
ments occur at the end of the year. A 12% aftertax discount rate is used. The MACRS depreciation rates
and the present values for 12 and 20% are as follows:
Present Value of Present Value of An
$1 Received at ordinary annuity Of $1
Macrs three- The End of The received At the End Of
year rate Period each Period
YEAR 12% 20% 12% 20%
1 33.3% .89 .83 .89 .83
2 44.5 .80 .69 1.69 1.53
3 14.8 .71 .58 2.40 2.11
4 7.4 .64 .48 3.04 2.59
5 - .57 .40 3.61 2.99

Required:
a. Jonfran Company must decide whether to purchase the waste containers from an outside supplier or to
purchase the equipment to manufacture the waste containers. Calculate the NPV of the estimated aftertax
cash inflows at December 31, 20X3, and determine which of these two options to pursue.
b. Companies often calculate the payback period for an investment. Without prejudice to your response in
Requirement (a), assume that the capital cost is $1,000,000 and the aftertax cash inflows for 20X4-20X8
are as follows:
20X4 $300,000
20X5 350,000
20X6 240,000
20X7 200,000
20X8 200,000

1. Explain why some companies calculate the payback period in addition to determining the NPV.
2. Calculate the payback period for this project using the assumed aftertax cash inflows. [CMA adapted]
23.48 Analyzing three alternative financing decisions. Edwards Corporation is a manufacturing concern
that produces and sells a wide range of products. The company not only mass-produces a number of prod-
ucts and equipment components but also is capable of producing special-purpose manufacturing equip-
ment to customer specifications.
The firm is considering adding a new stapler to one of its product lines. More equipment will be required
to produce the new stapler. Edwards has identified three alternative ways of acquiring the needed equip-
ment:
• Purchase general-purpose equipment
• Lease general-purpose equipment
• Build special-purpose equipment
Purchase of the special-purpose equipment has been ruled out because it would be prohibitively expensive.
The general-purpose equipment can be purchased for $125,000. The equipment has an estimated salvage
value of $15,000 at the end of its useful life of ten years. At the end of five years, the equipment can be
used elsewhere in the plant or be sold for $40,000.

Page 46 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

Alternatively, the general-purpose equipment can be acquired under a five-year lease for $40,000 annu-
ally. The lessor will assume all responsibility for taxes, insurance, and maintenance.
Special-purpose equipment can be constructed by Edwards' Contract Equipment Department. Although
the department is operating at a level that is normal for the time of year, it is below full capacity. The
department could produce the equipment without interfering with its regular revenue-producing activities.
The estimated departmental costs for the construction of the special-purpose equipment are as follows:
Materials and parts $ 75,000
Direct labor 60,000
Variable overhead (50% of DL$) 30,000
Fixed overhead (25% of DL$) 15,000
Total $180,000

Corporation general and administrative costs average 20% of labor dollar content of factory production.
Engineering and management studies provide the following revenue and cost estimates (excluding lease
payments and depreciation) for producing the new stapler, depending on the equipment used:
General-purpose
equipment
Leased Purchased Self-constructed equipment
Unit selling price $5.00 $5.00 $5.00
Unit production costs:Materials 1.80 1.80 1.70
Conversion costs: 1.65 1.65 1.40
Total unit production costs 3.45 3.45 3.10
Unit contribution margin $1.55 $1.55 $1.90
Estimated unit volume 40,000 40,000 40,000
Estimated total contributionmargin $62,000 $62,000 $76,000
Other costs:Supervision $16,000 $16,000 $18,000
Taxes and insurance 3,000 5,000
Maintenance 3,000 2,000
Total $16,000 $22,000 $25,000

Discount Factors For 10% (Rounded)

Period Present value Of $1 Present Value Of $1 Per Period received At
End of Period
1 .91 .91
2 .83 1.74
3 .75 2.49
4 .68 3.17
5 .62 3.79
6 .56 4.36
7 .51 4.87
8 .47 5.34
9 .42 5.76
10 .39 6.15

The company will depreciate the general-purpose machine over ten years using the sum-of-the-years-dig-
its (SYD) method. At the end of five years, the accumulated depreciation will total $80,000. (The present
value of this amount for the first five years is $62,100.) The special-purpose machine will be depreciated
over five years using the SYD method. Its salvage value at the end of that time is estimated to be $30,000.
The company uses an aftertax cost of capital of 10%. Its marginal tax rate is 40%.
Required:
a. Calculate the NPV for each of the three alternatives that Edwards has at its disposal.
b. Should Edwards select any of the three options, and, if so, which one? Explain your answer. [CMA
adapted]

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 47

 23.49 Analyzing the impact of income taxes and inflation. [CMA adapted] Catix Corporation is a divi-
sionalized company, and each division has the authority to make capital expenditures up to $200,000 with-
out approval of the corporate headquarters. The corporate controller has determined that the cost of capital
for Catix Corporation is 12%. This rate does not include an allowance for inflation, which is expected to
average 8% over the next five years. Catix pays income taxes at the rate of 40%.
The Electronics Division of Catix is considering the purchase of an automated assembly and soldering
machine for use in the manufacture of its printed circuit boards. The machine would be placed in service in
early 20X4. The divisional controller estimates that if the machine is purchased, two positions will be
eliminated, yielding a cost savings for wages and employee benefits. However, the machine would require
additional supplies and power. The cost savings and additional costs in current 20X3 prices are as follows:
Wages and employee benefits of the two positions eliminated
($25,000 each) $50,000
Cost of additional supplies 3,000
Cost of additional power 10,000
The new machine would be purchased and installed at the end of 20X3 at a net cost of $80,000. If pur-
chased, the machine would be depreciated on a straight-line basis for both book and tax purposes. The
machine will become technologically obsolete in four years and will have no salvage value at that time.
The Electronics Division compensates for inflation in capital expenditure analyses by adjusting the
expected cash inflows by an estimated price level index. The adjusted aftertax cash inflows are then dis-
counted using the appropriate discount rate. The estimated year-end index values for each of the next five
years are as follows:
Year Year-end Price Index
20X3 1.00
20X4 1.08
20X5 1.17
20X6 1.26
20X7 1.36
20X8 1.47

The Plastics Division of Catix compensates for inflation in capital expenditure analyses by adding the
anticipated inflation rate to the cost of capital and then using the inflation-adjusted cost of capital to dis-
count the project cash inflows. The Plastics Division recently rejected a project with cash inflows and eco-
nomic life similar to those associated with the machine under consideration by the Electronics Division.
The Plastics Division's analysis of the rejected project was as follows:
Net pretax cost savings $37,000
Less incremental depreciation expenses 20,000
Increase in taxable income $17,000
Increase in income taxes (40%) 6,800
Increase in aftertax income $10,200
Add back noncash expense (depreciation) 20,000
Net aftertax annual cash inflow (unadjusted for inflation) $30,200
Present value of net cash inflows using the sum of the cost of capital (12%) $77,916
and the inflation rate (8%) or a minimum required return of 20%
Investment required < 80,000>
Net present value <$ 2,084>

All operating revenues and expenditures occur at the end of the year.
Required:
a. Using the price index provided, prepare a schedule showing the net aftertax annual cash inflows adjusted
for inflation for the automated assembly and soldering machine under consideration by the Elec-
tronics Division.
b. Without prejudice to your answer in Requirement (a), assume that the net aftertax annual cash inflows
adjusted for inflation for the project being considered by the Electronics Division are as follows:
Calculate the NPV for Electronic Division's project.

Page 48 COST AND MANAGEMENT ACCOUNTING

 CHAPTER 23

20X4 20X5 20X6 20X7

Net aftertax annual cash inflow adjusted for inflation $30,000 $35,000 $37,000 $40,000
c. Evaluate the methods used by the Plastics Division and the Electronics Division to compensate for
expected inflation in capital expenditure analyses.
23.50 Capital budgeting and pricing. Wardl Industries is a manufacturer of standard and custom-designed
bottling equipment. Early in December 20X3, Lyan Company asked Wardl to quote a price for a custom-
designed bottling machine to be delivered on April 1, 20X4. Lyan intends to make a decision on the pur-
chase of such a machine by January 1 so Wardl would have the entire first quarter of 20X4 to build the
equipment.
Wardl's standard pricing policy for custom-designed equipment is 50% markup on full cost. Lyan's speci-
fications for the equipment have been reviewed by Wardl's Engineering and Cost Accounting Depart-
ments, and they made the following estimates for raw materials and direct labor:
Raw materials $256,000
Direct labor (11,000 DLhr at 165,000
$15)
Manufacturing overhead is applied on the basis of direct labor hours. Wardl normally plans to run its plant
with 15,000 direct labor hours per month and assigns overhead on the basis of 180,000 direct labor hours
per year. The overhead application rate for 20X4 of $9 per direct labor hour is based on the following bud-
geted manufacturing overhead costs for 20X4:
Variable manufacturing over- $ 972,000
head
Fixed manufacturing overhead 648,000
Total manufacturing overhead $1,620,000

The Wardl production schedule calls for 12,000 direct labor hours per month during the first quarter. If
Wardl is awarded the contract for the Lyan equipment, production of one of its standard products will have
to be reduced. This is necessary because production levels can only be increased to 15,000 direct labor
hours each month on short notice. Furthermore, Wardl's employees are unwilling to work overtime.
Sales of the standard product equal to the reduced production will be lost, but there will be no permanent
loss of future sales or customers. The standard product, whose production schedule will be reduced, has a
unit sales price of $12,000 and the following cost structure:
Raw materials $2,500
Direct labor (250 DLhr at 3,750
$15)
Overhead (250 DLhr at $9) 2,250
Total cost $8,500

Lyan needs the custom-designed equipment to increase its bottle-making capacity so that it will not have
to buy bottles from an outside supplier. Lyan Company requires 5,000,000 bottles annually. Its present
equipment has a maximum capacity of 4,500,000 bottles with a directly traceable cash outlay of $0.15 per
bottle. Thus, Lyan has had to purchase 500,000 bottles from a supplier at $0.40 each. The new equipment
would allow Lyan to manufacture its entire annual demand for bottles at a raw material cost savings of
$0.01 for each bottle manufactured.
Wardl estimates that Lyan's annual bottle demand will continue to be 5,000,000 bottles over the next five
years, the estimated economic life of the special-purpose equipment. Wardl further estimates that Lyan has
an aftertax cost of capital of 15% and is subject to a 40% marginal income tax rate, the same rates as
Wardl.
Required:
a. Wardl Industries plans to submit a bid to Lyan Company for the manufacture of the special-purpose bot-
tling equipment.
1. Calculate the bid Wardl would submit if it follows its standard pricing policy for special-purpose equip-
ment.

CAPITAL BUDGETING METHODOLOGY: THE FINANCIAL ANALYSIS STAGE PAGE 49

 2. Calculate the minimum bid Wardl would be willing to submit on the Lyan equipment that would result
in the same profits as planned for the first quarter of 20X4.
b. Wardl wants to estimate the maximum price Lyan would be willing to pay for the special-purpose bot-
tling equipment.
1. Calculate the present value of the aftertax savings in directly traceable cash outlays that Lyan could
expect to realize from the new special-purpose bottling equipment.
2. Identify the other factors Wardl would have to incorporate in its estimate of the maximum price Lyan
would be willing to pay for the equipment.
3. Describe how the cost savings (Requirement [b] 1) and the other factors (Requirement [b] 2) would be
combined to calculate the estimate of the maximum price Lyan would be willing to pay for the
equipment.
[CMA adapted]
23.51 Monte Carlo sensitivity analysis. NoClip Novelties has discovered a better method for attaching
name badges, like those used at conventions and conferences, without using clips or pins that could dam-
age clothing. By using an adhesive stick-on system, NoClip believes it can capture a significant part of the
badge market. It is planning to use new technology and is somewhat uncertain about development costs.
Development cost estimates are as follows:
Best case $150,000
Worst case 330,000
Most likely case 240,000

Probabilities assigned to estimate cash inflows over four years, the life of the new project, are as follows:

Year1 per- Estimated Year 2 Estimated Year 3 Estimated Year 4 Esti-

centage cash inflows percentage cash inflows percentage cash inflows percentage mated
cash
inflows
0.1 $100,000 0.1 $150,000 0.2 $150,000 0.1 $110,000
0.2 90,000 0.3 110,000 0.4 110,000 0.1 90,000
0.1 85,000 0.5 100,000 0.3 80,000 0.6 70,000
0.2 70,000 0.1 75,000 0.1 55,000 0.2 40,000
0.1 65,000
0.3 50,000

90

70
Cumulative mean NPV [$]

14 000
Number of values

50
10 000
30
6000
10
2000
32500
-22500

2500

0
Monte Carlo iterations
Mid-point values [NPV $]

Required: For the following, make any assumptions you deem necessary:
a. What is the minimum number of iterations required for the Monte Carlo Simulation? Discuss and justify
your answer.
b. Should CleanSweep proceed with the new product line? Discuss and justify your answer.

Page 50 COST AND MANAGEMENT ACCOUNTING