Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd
Upload
188 views

QA Assignment 02

The document contains 5 quantitative analysis questions involving linear programming problems: maximizing daily production and contribution for a bed and pillow company; finding the feasible region and optimal solution for a two variable maximization problem; making the primal and dual problems for a product mix problem across 3 machine shops; converting a given linear programming maximization problem to its dual; and assigning persons to jobs to minimize total cost based on a cost table.

Uploaded by

Markos Melaku
Copyright
© © All Rights Reserved
Available Formats
Download as PDF, TXT or read online on Scribd
188 views

QA Assignment 02

The document contains 5 quantitative analysis questions involving linear programming problems: maximizing daily production and contribution for a bed and pillow company; finding the feasible region and optimal solution for a two variable maximization problem; making the primal and dual problems for a product mix problem across 3 machine shops; converting a given linear programming maximization problem to its dual; and assigning persons to jobs to minimize total cost based on a cost table.

Uploaded by

Markos Melaku
Copyright
© © All Rights Reserved
Available Formats
Download as PDF, TXT or read online on Scribd
You are on page 1/ 2

Quantitative Analysis for Management

Decisions
Assignment 2

Q1. A bed mart company is in the business of manufacturing beds and


pillows. The company has 40 hours for assembly and 32 hours for
finishing work per day. Manufacturing of a bed requires 4 hours for
assembly and 2 hours for finishing. Similarly a pillow requires 2 hours
for assembly and 4 hours for finishing. Profitability analysis indicates
that every bed would contribute Birr 80, while a pillow contribution is
Birr 55 respectively. Find out the daily production of the company to
maximise the contribution (profit). Solve the problem by graphical
method.

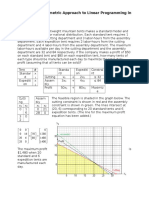
Q2. Maximise 1170x1 + 1110x2

Subject to: 9x1 + 5x2 ≥ 500


7x1 + 9x2 ≥ 300
5x1 + 3x2 ≤ 1500
7x1 + 9x2 ≤ 1900
2x1 + 4x2 ≤ 1000
x1, x2 ≥ 0
-Find graphically the feasible region and the optimal solution.

Q3. Consider the following product mix problem:

Three machine shops A, B, C produces three types of products X, Y, Z


respectively. Each product involves operation of each of the machine
shops. The time required for each operation on various products is
given as follows:
Machine shops

Products A B C Profit per unit


X 10 7 2 Birr 12
Y 2 3 4 Birr 3
Z 1 2 1 Birr 1
Available Hours 100 77 80
Make the Mathematical model of the above primal problem to maximise
the profit and then make the dual problem.

Q4. Convert the following linear programming problem into dual


problem.
Maximise
Z = 22x1 + 25x2 +19x3
Subject to:
18x1 + 26x2 + 22x3 ≤ 350
14x1 + 18x2 + 20x3 ≥180
17x1 + 19x2 + 18x3 = 205
x1, x2, x3 ≥ 0

Q5. A work shop contains four persons available for work on the four
jobs. Only one person can work on any one job. The following table
shows the cost of assigning each person to each job. The objective is to
assign person to jobs such that the total assignment cost is a minimum.

Jobs
1 2 3 4
A 20 25 22 28
Persons B 15 18 23 17
C 19 17 21 24
D 25 23 24 24

You might also like