IP06 Sol S2A E Web

New Effective Learning Mathematics S2A
Integrated Practice VI Solution Guide
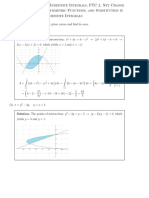
1. 2 x + y = 6
x 0 1 2
y 6 4 2
x + 4 y = −4
x 0 2 4
y −1 −1.5 −2
1A
From the figure, the two straight lines intersect at
(4, −2).
∴ The solution is x = 4 , y = −2 . 1A
(2)
2. x = 3 y
x 0 3 6
y 0 1 2
2x + y = 7
x 0 1 2
y 7 5 3
© 2021 Chung Tai Educational Press Limited |

© 2021 Marshall Cavendish Education (a member of Times Publishing Limited) 1
1A
(3, 1).
∴ The solution is x = 3 , y = 1. 1A
(2)
3. 3x + 2 y = 10
x 0 2 4
y 5 2 −1
2 x − 5 y = −12
x −1 1.5 4
y 2 3 4
1A
(1.4, 2.9) (cor. to 1 d.p.).
∴ The solution is x = 1.4 , y = 2.9 . 1A
(2)

3 x − y = 1............................. (1)
4. 
5 x − y = −1.......................... (2)
From (1), y = 3 x − 1............... (3)
Substitute (3) into (2),
5 x − (3 x − 1) = −1 1M
5 x − 3x + 1 = −1
2 x = −2
x = −1
Substitute x = −1 into (3),
y = 3(−1) − 1
= −4
∴ The solution is x = −1, y = −4 . 1A
(2)
 x − 4 y = 2 ........................... (1)
5. 
3 x − 2 y = 16 ........................ (2)
From (1), x = 4 y + 2 ..............(3)
3(4 y + 2) − 2 y = 16 1M
12 y + 6 − 2 y = 16
10 y = 10
y =1
Substitute y = 1 into (3),
x = 4(1) + 2
=6
(2)
 2 y + 7 x = −15 .....................(1)
6. 
 −4 x − 3 y = 3 ....................... (2)
−7 x − 15
From (1), y = ......... (3)
2
 −7 x − 15 
−4 x − 3  =3 1M
 2 
  −7 x − 15  
2(−4 x) − 2 3    = 2(3)
  2 
−8 x + 21x + 45 = 6
13 x = −39
x = −3
−7(−3) − 15
y=
2
=3
∴ The solution is x = −3, y = 3. 1A
(2)
 x + 4 y = 7 ...............................(1)
7. 
3 x − y = 8 ............................... (2)
(1) × 3: 3 x + 12 y = 21................ (3)

(3) − (2): (3x + 12 y ) − (3x − y ) = 21 − 8 1M

3x + 12 y − 3 x + y = 13
13 y = 13
y =1
x + 4(1) = 7
x=3
(2)
2 x − 3 y = 4 ............................. (1)
8. 
4 x − 2 y = 8 ............................. (2)
(1) × 2: 4 x − 6 y = 8 ................... (3)
(2) − (3): (4 x − 2 y ) − (4 x − 6 y ) = 8 − 8 1M
4x − 2 y − 4x + 6 y = 0
4y = 0
y=0
2 x − 3(0) = 4
2x = 4
x=2
∴ The solution is x = 2 , y = 0 . 1A
(2)
3 x + 8 y = −18 ........................ (1)

9. 
−2 y + 5 x = −7 ........................ (2)
(2) × 4: −8 y + 20 x = −28 .......... (3)
(1) + (3): (3 x + 8 y ) + (−8 y + 20 x) = −18 + (−28) 1M
3 x + 8 y − 8 y + 20 x = −46
23x = −46
x = −2
3(−2) + 8 y = −18
8 y = −12
3
y=−
2
3
∴ The solution is x = −2, y = − . 1A
2
(2)
10. (a) 2 x = ( x + 10°) + 40° (ext. ∠ of ) 1M

2 x = x + 50°
x = 50° 1A
(b)  AC = BC (given)
∴ ∠BAC = x + 33° (base ∠s, isos. )
In ABC,
( x + 33°) + ( x + 33°) = 5 x (ext. ∠ of ) 1M
2 x + 66° = 5 x
3x = 66°
x = 22° 1A
(4)

11. (a) 5 x + 72° + 160° + 78° + 100° = (5 − 2) × 180°

(∠ sum of polygon) 1M
5 x + 410° = 540°
5 x = 130°
x = 26° 1A
(b) x + 4 x + 32° + 90° + 133° + 90° = (6 − 2) × 180°

5 x + 345° = 720°
5 x = 375°
x = 75° 1A
(4)
12. (a) x + 2 x + 131° + 61° = 360° (sum of ext. ∠s of polygon) 1M

3 x + 192° = 360°
3 x = 168°
x = 56° 1A
(b) 4 x + (3 x + 2°) + 90° + 25° + 75° + 70° = 360°

(sum of ext. ∠s of polygon) 1M
7 x + 262° = 360°
7 x = 98°
x = 14° 1A
(4)
13. (a)  DEF ~  XYZ (given)

DE DF
∴ = (corr. sides, ~ s) 1M
XY XZ
x 3
= 1M
4 6
x=2 1A
(b)  LMN ~ IJK (given)

∴ ∠M = ∠J (corr. ∠s, ~ s) 1M
= 60°
∠N = ∠K (corr. ∠s, ~ s)
=x
In LMN,
∠L + ∠M + ∠N = 180° (∠ sum of ) 1M
52° + 60° + x = 180°
x = 68° 1A
(6)
14. (a) Substitute x = 4 into the equation,

3y + 7 = 4 1M
3 y = −3
y = −1
∴ The y-coordinate of A is −1. 1A
(b) Substitute x = 1 and y = 2 into the equation,

L.H.S. = 3(2) + 7 1M
= 13
R.H.S. = 1

 L.H.S. ≠ R.H.S.
∴ (1, 2) does not satisfy the equation.
i.e. (1, 2) does not lie on the graph of 3 y + 7 = x . 1A
(4)
15. (a) Size of each interior angle

= 180° − 15° (adj. ∠s on st. line) 1M
= 165° 1A
(b) Let n be the number of sides of the regular polygon.

360°
= 15° (sum of ext. ∠s of polygon) 1M
n
n = 24
∴ The number of sides of the regular polygon
is 24. 1A
(4)
16. Let x g and y g be the weights of a cupcake and a piece of

cheesecake respectively.
Accordingly,
5 x + 4 y = 900......................(1) 
3 x + 8 y = 1 240....................(2)  1M
 
(1) × 2: 10 x + 8 y = 1 800........(3) 1M
(3) − (2): (10 x + 8 y ) − (3x + 8 y ) = 1 800 − 1 240 1M
10 x + 8 y − 3x − 8 y = 560
7 x = 560
x = 80
∴ The weight of a cupcake is 80 g. 1A
(4)
17. Let x and y be the original numbers of stamps owned by

Luke and Zoe respectively.
Accordingly,
 x + y = 33 ........... (1)

 1 1M
 x + 3 = 2 y .......... (2)
1
(1) − (2): ( x + y ) − ( x + 3) = 33 −y 1M
2
1
x + y − x − 3 = 33 − y
2
3y
= 36
2
y = 24
x + 24 = 33
x=9
∴ The original number of stamps owned by Luke
is 9 and the original number of stamps owned
by Zoe is 24. 1A + 1A
(4)

18. Let x and y be the numbers of apples and pears Kary

bought respectively.
Accordingly,
6 x + 4.5 y = 90...............(1) 
 x = 3 y ............................(2)  1M
 
6(3 y ) + 4.5 y = 90 1M
22.5 y = 90
y=4
x = 3(4)
= 12
∴ Total number of fruits Kary bought
= 12 + 4 1M
= 16 1A
(4)
5 x − y = 11........................(1)
19. 
2 y − 3 x = 7 x − 22..............(2)
From (1), y = 5 x − 11..........(3)
2(5 x − 11) − 3 x = 7 x − 22 1M
10 x − 22 − 3 x = 7 x − 22
7 x − 22 = 7 x − 22
0=0
∴ The equations have infinitely many solutions. 1A
(2)
 x 2 y 19
 − = ..................................... (1)
20.  2 5 10
3
 ( x + 3) = 8 − y ................................ (2)
 2
x 2y 19
(1) × 10: × 10 − × 10 = × 10
2 5 10
5 x − 4 y = 19 ............. (3)
From (2), 3( x + 3) = 2(8 − y )
3 x + 9 = 16 − 2 y
3 x + 2 y = 7 .......................... (4)
(4) × 2: 6 x + 4 y = 14 ............................. (5)
(3) + (5): (5 x − 4 y ) + (6 x + 4 y ) = 19 + 14 1M
5 x − 4 y + 6 x + 4 y = 33
11x = 33
x=3
Substitute x = 3 into (4),
3(3) + 2 y = 7
2 y = −2
y = −1
∴ The solution is x = 3 , y = −1 . 1A
(2)

21. Rewrite the simultaneous equations as follows:

 4 − x y +1
 2 + 3 = 8 .................................. (1)

3 y − 4 − 3 x = 8 .................................... (2)
 4
4− x y +1
(1) × 6: ×6+ × 6 = 8× 6
2 3
3(4 − x) + 2( y + 1) = 48
12 − 3 x + 2 y + 2 = 48
2 y − 3 x = 34 ........... (3)
4 − 3x
(2) × 4: 3 y × 4 − × 4 = 8× 4
4
12 y − (4 − 3 x) = 32
12 y − 4 + 3 x = 32
12 y + 3 x = 36 .............. (4)
(3) + (4): (2 y − 3 x) + (12 y + 3 x) = 34 + 36 1M

2 y − 3x + 12 y + 3 x = 70
14 y = 70
y=5
12(5) + 3x = 36
3x = −24
x = −8
(2)
3 x = −2( y + 2) ........... (1)

22. (a) 
 x = y − 3 .................... (2)
3( y − 3) = −2( y + 2) 1M
3 y − 9 = −2 y − 4
5y = 5
y =1
x =1− 3
= −2
(b) Let x = a + 1 and y = b − 3 . 1M

3 x = −2( y + 2)
The simultaneous equations  can be
x = y − 3
3(a + 1) = −2(b − 1)
written as  .
a + 1 = b − 6
From the result of (a),
x = −2
a + 1 = −2
a = −3
y =1
b −3 =1
b=4
∴ The solution is a = −3, b = 4 . 1A
(4)

23.  ABC ~ PQR (given)

∴ ∠A = ∠P (corr. ∠s, ~ s)
x + y = 70°
∠B = ∠Q (corr. ∠s, ~ s)
y + 20° = 2 x
2 x − y = 20°
 x + y = 70° .................(1) 
∴   1M
2 x − y = 20° ...............(2) 
(1) + (2): ( x + y ) + (2 x − y ) = 70° + 20° 1M
3 x = 90°
x = 30° 1A
Substitute x = 30° into (1),

30° + y = 70°
y = 40° 1A
(4)
24. Each interior angle of a regular pentagon

(5 − 2) × 180°
= (∠ sum of polygon)
5
= 108°
4 x + 3 y = 108°..................(1) 
∴   1M
2 x + 4 y + 4° = 108° ..........(2) 
(2) × 2: 4 x + 8 y + 8° = 216°
4 x + 8 y = 208° .......(3)
(3) − (1): (4 x + 8 y ) − (4 x + 3 y ) = 208° − 108° 1M
5 y = 100°
y = 20° 1A
Substitute y = 20° into (1),

4 x + 3(20°) = 108°
4 x + 60° = 108°
4 x = 48°
x = 12° 1A
(4)
25. Let n be the number of sides of the regular polygon.

∴ Each exterior angle
360°
= (sum of ext. ∠s of polygon) 1M
n
Each interior angle
(n − 2) × 180°
= (∠ sum of polygon) 1M
n
 360°  (n − 2) × 180°
∴ 7 = 1M
 n  n
2 520° = 180°n − 360°
2 880° = 180°n
n = 16
∴ The number of sides of the regular polygon is 16. 1A
(4)

26. (a) In CBA and ABD,

∠BAC = ∠BDA (given)
∠ABC = ∠DBA (common ∠)
∠ACB = 180° − ∠BAC − ∠ABC (∠ sum of ) 1M
= 180° − ∠BDA − ∠DBA (proved)
= ∠DAB (∠ sum of )
∴ CBA ~  ABD (A.A.A.) 1
(b)  CBA ~  ABD (proved)

CB BA
AB BD
8 12
= 1M
12 8 + CD
64 + 8CD = 144
8CD = 80
CD = 10 1A
(5)
27. (a) Sum of interior angles of a regular 12-sided polygon

= (12 − 2) × 180° (∠ sum of polygon) 1M
= 1 800°
∴ Size of each interior angle = 1 800° ÷ 12 1M
= 150° 1A
(b)  PQ = QR
∴ ∠RPQ = ∠PRQ (base ∠s, isos. ) 1M
In PQR,
∠RPQ + ∠PRQ + ∠PQR = 180° (∠ sum of ) 1M
2∠PRQ + 150° = 180°
2∠PRQ = 30°
∠PRQ = 15° 1A
(6)
28. Let x and y be the original numbers of jelly beans Stanley

and Timothy has respectively.
Accordingly,
 x = 7 y .......................(1) 
 x − 54 = y + 54..........(2)  1M
 
7 y − 54 = y + 54 1M
6 y = 108
y = 18
x = 7(18)
= 126
∴ Number of jelly beans they have in total
= 126 + 18 1M
= 144 1A
(4)

29. Let x and y be the numbers of bottles of juice and others

respectively.
Accordingly,
 x + y = 255.....................(1) 
 x = y (1 − 30%)...............(2)  1M
 
y (1 − 30%) + y = 255 1M
0.7 y + y = 255
1.7 y = 255
y = 150
x + 150 = 255
x = 105
∴ Required difference = 150 − 105 1M
= 45 1A
(4)
30. Let x and y be the numbers of boys and girls respectively.

Accordingly,
 x + y = 298 ....................... (1)

3 1M
 5 x + 18 = y ...................... (2)
(1) × (3): 3 x + 3 y = 894 ...... (3)

(2) × 5: 3 x + 90 = 5 y .......... (4)
(3) − (4): (3 x + 3 y ) − (3 x + 90) = 894 − 5 y 1M
3 x + 3 y − 3 x − 90 = 894 − 5 y
8 y = 984
y = 123
∴ There are 123 girls in the Christmas party. 1A
(3)
31. Let $x and $y be the original prices of a lemon tart and a

cup of coffee respectively.
Accordingly,
 x + y = 67 ................................................ (1) 1M
 x(1 + 15%) + y (1 − 8%) = 67 + 2 ...............(2)

From (1), x = 67 − y .................................. (3)
(67 − y ) × (1 + 15%) + y (1 − 8%) = 67 + 2 1M
1.15(67 − y ) + 0.92 y = 69
77.05 − 1.15 y + 0.92 y = 69
−0.23 y = −8.05
y = 35
x = 67 − 35
= 32
∴ The original price of a lemon tart is $32 and the
original price of a cup of coffee is $35. 1A + 1A

(4)

32. Let m be the number of stickers owned by Paul.

Accordingly,
n:m = 7:4
n 7
=
m 4
4n = 7 m ........................... (1)
1M
n − 9 = m + 9 ....................... (2)
From (2), m = n − 18 ...........(3)
4n = 7(n − 18) 1M
4n = 7 n − 126
126 = 3n
n = 42 1A
(3)
33. (a) Let a and b be the original numbers of $2 coins and $5

coins respectively.
Accordingly,
a :b = 2:3
a 2
=
b 3
2b
a= ........................... (1)
3
(a − 3) : (b + 3) = 3 : 7
a −3 3
= 1M
b+3 7
7(a − 3) = 3(b + 3)
7 a − 21 = 3b + 9
7 a − 3b = 30 ............(2)
 2b 
7   − 3b = 30 1M
 3 
  2b  
3 7    − 3(3b) = 3(30)
  3 
14b − 9b = 90
5b = 90
b = 18
Substitute b = 18 into (1),
2(18)
a=
3
= 12
∴ The original number of $2 coins is 12 and
the original number of $5 coins is 18. 1A + 1A
(b) Original amount of money : New amount of money

= $(2 × 12 + 5 × 18) : $ [2 × (12 − 3) + 5 × (18 + 3)] 1M
= $114 : $123
$114 $123
= :
$3 $3
= 38 : 41 1A
(6)
34. (a)   ABC ≅  AED (given)

∴ AC = AD (corr. sides, ≅ s) 1M
∴ ∠ADC = ∠ACD (base ∠s, isos. ) 1M
= 70°

In ACD,
∠ACD + ∠CAD + ∠ADC = 180° (∠ sum of ) 1M
70° + (112° − 2 x) + 70° = 180°
2 x = 72°
x = 36° 1A
(b) Each interior angle of a regular pentagon

(5 − 2) × 180°
5
= 108°
 ∠BCD = 36° + 70°
= 106°
≠ 108° 1M
∴ ABCDE is not a regular pentagon. 1A
(7)
35. (a) In APQ and ACB,

∠PAQ = ∠CAB (common ∠)
∠AQP = ∠ABC (given)
∠APQ = 180° − ∠PAQ − ∠AQP (∠ sum of ) 2M
= 180° − ∠CAB − ∠ABC (proved)
= ∠ACB (∠ sum of )
∴ △ APQ ~  ACB (A.A.A.) 1
(b)  △ APQ ~  ACB (proved)

AQ PQ
AB CB
4 cm 3 cm
= 1M
5 cm + PB 6 cm
4 cm 1
=
5 cm + PB 2
5 cm + PB = 8 cm
PB = 3 cm 1A
(c) In PBC and PQC,

PB = PQ = 3 cm
PC = PC (common side)
∠PQC + 90° = 180° (adj. ∠s on st. line) 1M
∠PQC = 90°
= ∠PBC
∴ PBC ≅ PQC (R.H.S.) 1
(8)
36. (a) In ABC and DEF,

AB = DE
BC = EF 1M
∠ABC = ∠DEF
∴  ABC ≅ DEF (S.A.S.) 1
(b) In ACD and DFA,

  ABC ≅ DEF (proved)
∴ AC = DF (corr. sides, ≅ s) 1M
CD = FA
AD = DA (common side)
∴  ACD ≅ DFA (S.S.S.) 1

(c) Each interior angle of a regular hexagon

(6 − 2) × 180°
6
= 120°
∴ ∠ABC = ∠BCD = 120°
 BA = BC
∴ ∠BAC = ∠BCA (base ∠s, isos. ) 1M
In ABC,
∠ABC + ∠BAC + ∠BCA = 180° (∠ sum of )
120° + 2∠BCA = 180°
2∠BCA = 60°
∠BCA = 30°
∠ACD = ∠BCD − ∠BCA
= 120° − 30°
= 90° 1A
∴ AC ⊥ CD
∴ The claim is agreed. 1A
(8)
37. (a) Each interior angle of a regular pentagon

(5 − 2) × 180°
5
= 108°
∴ ∠PQR = 108°
∠TQP = 180° − 108° (adj. ∠s on st. line)
= 72° 1A
(b) Each interior angle of a regular octagon

(8 − 2) × 180°
8
= 135° 1A
∴ ∠DEF = ∠EFG = ∠FGT = 135°
∠QRS = ∠RSE = 108°
In hexagon EFGTRS,
∠GTR + 108° + 108° + 135° + 135° + 135° = (6 − 2) × 180°
∠GTR + 621° = 720°
∠GTR = 99° 1A
 ∠GTQ + ∠TQP = 99° + 72°
= 171°
≠ 180° 1M
∴ GH and PQ are not parallel to each other. 1A
(8)
38. Extend the straight lines in the figure such that the graphs of the two
equations intersect.
From the figure, the two straight lines intersect at (3, 1).

∴ The solution is x = 3 , y = 1 .
∴ The answer is B.
39. A. Substitute x = 8 and y = −3 into x = − 8 ,

L.H.S. = 8
R.H.S. = −8
 L.H.S. ≠ R.H.S.
∴ (8, −3) does not satisfy the equation x = −8.
i.e. (8, −3) does not satisfy the simultaneous equations
 x = −8
2 x − y = 13 .

B. Substitute x = 8 and y = −3 into x − 5 y = −7 ,

L.H.S. = 8 − 5(−3)
= 23
R.H.S. = −7
 L.H.S. ≠ R.H.S.
∴ (8, −3) does not satisfy the equation x − 5 y = −7 .
 y = −3
 x − 5 y = −7 .

C. Substitute x = 8 and y = −3 into x − 2 y = 2 ,

L.H.S. = 8 − 2(−3)
= 14
R.H.S. = 2
 L.H.S. ≠ R.H.S.
∴ (8, −3) does not satisfy the equation x − 2 y = 2 .
x + y = 5
x − 2 y = 2 .

D. Substitute x = 8 and y = −3 into x − y = 11,

L.H.S. = 8 − (−3)
= 11
R.H.S. = 11
∴ L.H.S. = R.H.S.
Substitute x = 8 and y = −3 into 2 x + 3 y = 7 ,
L.H.S. = 2(8) + 3(−3)
=7
R.H.S. = 7
 L.H.S. = R.H.S.
∴ (8, −3) satisfies the equations x − y = 11 and 2 x + 3 y = 7 .
 x − y = 11
i.e. (8, −3) satisfies the simultaneous equations  .
2 x + 3 y = 7
∴ The answer is D.
40. Rewrite the simultaneous equations as follows:

6 x + 7 y = 45....................(1)
3 x + 11 y = 45...................(2)

(2) × 2: 6 x + 22 y = 90 ....... (3)

(3) − (1): (6 x + 22 y ) − (6 x + 7 y ) = 90 − 45
6 x + 22 y − 6 x − 7 y = 45
15 y = 45
y =3
∴ The answer is A.
41. The answer is D.
42. Reflex ∠BCD = 360° − c (∠s at a pt.)

Reflex ∠AED = 360° − e (∠s at a pt.)
a + b + Reflex ∠ BCD
+ d + Reflex ∠AED = (5 − 2) × 180° (∠ sum of polygon)
a + b + (360° − c ) + d + (360° − e) = 540°
a + b − c + d − e + 720° = 540°
a + b + d = c + e − 180°
43. In regular pentagon ABCDE,

(5 − 2) × 180°
each interior angle = (∠ sum of polygon)
5
= 108°
In quadrilateral CDFG,
p + r + t + v = (4 − 2) × 180° (∠ sum of polygon)
= 360°
∴ p + q + r + s + t + u + v = ( p + r + t + v) + ( q + s + u )
= 360° + 3 × 108°
= 684°
44. In ABE and ADC,

AB 10 2
= =
AD 8 + 7 3
AE 8 2
= =
AC 10 + 2 3
AB AE
∴ =
AD AC
∠EAB = ∠CAD (common ∠)
∴  ABE ~  ADC (ratio of 2 sides, inc. ∠)
AB BE
∴ = (corr. sides, ~ s)
AD DC
2 x
=
3 18
x = 12
∴ The answer is C.
45. In ABC and CDE,

∠ABC = ∠CDE (given)
∠BAC + ∠ABC = ∠ACD (ext. ∠ of )
∠BAC + ∠ABC = ∠ACE + ∠DCE
∠BAC + ∠ABC = ∠ABC + ∠DCE (given)
∠BAC = ∠DCE

∠ACB = 180° − ∠ABC − ∠BAC (∠ sum of )

= 180° − ∠CDE − ∠DCE (proved)
= ∠CED (∠ sum of )
∴ ABC ~ CDE (A.A.A.)
DE CD
BC AB
DE 12
=
6 18
DE = 4
 ∠ABC = ∠ADB (given)
∴ AD = AB (sides opp. equal ∠s)
= 18
AE = AD − DE
= 18 − 4
= 14
46. Let $x and $y be the selling prices of a bag A and a bag B respectively.
Accordingly,
 2 x + 3 y = 3 030 ................................. (1)

3 x + 2 y = 2 970 ................................. (2)
(1) × 2: 4 x + 6 y = 6 060 ....................... (3)
(2) × 3: 9 x + 6 y = 8 910 ........................(4)
(4) − (3): (9 x + 6 y ) − (4 x + 6 y ) = 8 910 − 6 060
9 x + 6 y − 4 x − 6 y = 2 850
5 x = 2 850
x = 570
∴ The selling price of a bag A is $570.
 x + 2 y = 3 ....................... (1)
47. 
 2 x + 4 y = 5 ..................... (2)
(1) × 2: 2 x + 4 y = 6 ........... (3)
(3) − (2): (2 x + 4 y ) − (2 x + 4 y ) = 6 − 5
0 =1
∴ The equations have no solutions.
48. Each interior angle of a regular n-sided polygon

(n − 2) × 180°
n
Each exterior angle of a regular n-sided polygon
360°
= (sum of ext. ∠s of polygon)
n
(n − 2) × 180° 360°
∴ = 4×
n n
180°n − 360° = 1 440°
180°n = 1 800°
n = 10
∴ I is not true.
(10 − 2) × 180°
Each interior angle of the polygon =
10
= 144°
∴ II is true.

360°
Each exterior angle of the polygon =
10
= 36°
∴ III is true.
49. I. Each interior angle of a regular pentagon

(5 − 2) × 180°
5
= 108°
Each interior angle of a regular 10-sided polygon
(10 − 2) × 180°
10
= 144°
∴ I is not correct.
II. Each exterior angle of a regular pentagon

= 180° − 108° (adj. ∠s on st. line)
= 72°
Each exterior angle of a regular 10-sided polygon
= 180° − 144° (adj. ∠s on st. line)
= 36°
∴ II is correct.
50. In ABC and CDE,

AB = CD
BC = DE
∠ABC = ∠CDE
∴  ABC ≅ CDE (S.A.S.)
∴ I is true.
 AF = EF
∴ AEF is an isosceles triangle.
∴ II is true.
Similarly,
 ABC ≅ EFA (S.A.S.)
∴ AC = EA (corr. sides, ≅ s)
  ABC ≅ CDE (proved)
∴ AC = CE (corr. sides, ≅ s)
∴ AC = EA = CE
∴ ACE is an equilateral triangle.
∴ III is true.
51.  ABC is an equilateral triangle.

∴ ∠EBF = ∠FCD = 60°
In BEF and CFD,
∠EBF = ∠FCD
∠EBF + ∠BEF = ∠EFC (ext. ∠ of )
∠EBF + ∠BEF = ∠EFD + ∠CFD
60° + ∠BEF = 60° + ∠CFD
∠BEF = ∠CFD

∠BFE = 180° − ∠EBF − ∠BEF (∠ sum of )

= 180° − ∠FCD − ∠CFD
= ∠CDF (∠ sum of )
∴ BEF ~ CFD (A.A.A.)
52. Each interior angle of a regular pentagon

(5 − 2) × 180°
5
= 108°
 AB = CB
∴ ∠BCA = ∠BAC (base ∠s, isos. )
In ABC,
∠BCA + ∠BAC + ∠ABC = 180° (∠ sum of )
2 ∠BAC + 108° = 180°
2 ∠BAC = 72°
∠BAC = 36°
Similarly,
∠ABE = ∠AEB = ∠DCE = ∠DEC = ∠BCA = 36°
∴ ∠CEF = ∠AED − ∠AEB − ∠DEC
= 108° − 36° − 36°
= 36°
∠FCE = ∠BCD − ∠BCA − ∠DCE
= 108° − 36° − 36°
= 36°
In ABF and CEF,
∠ABF = ∠CEF (proved)
∠FAB = ∠FCE (proved)
∠AFB = ∠CFE (vert. opp. ∠s)
∴  ABF ~ CEF (A.A.A.)
∴ I is true.
In BCF and FEA,
∠CBF = ∠ABC − ∠ABE
= 108° − 36°
= 72°
∠EFA = ∠ABF + ∠FAB (ext. ∠ of )
= 36° + 36°
= 72°
∴ ∠CBF = ∠EFA
 ∠ABF = ∠BAF (proved)
∴ BF = FA (sides opp. equal ∠s)
∠BFC = ∠ABF + ∠FAB (ext. ∠ of )
= 36° + 36°
= 72°
∠FAE = ∠BAE − ∠BAF
= 108° − 36°
= 72°
∴ ∠BFC = ∠FAE
∴ BCF ≅ FEA (A.S.A.)
∴ II is true.
In CFE and CDE,
∠FCE = ∠DCE (proved)
CE = CE (common side)
∠FEC = ∠DEC (proved)
∴ CFE ≅ CDE (A.S.A.)

∴ III is true.
53. x + x + y + x + 80° = 360° (sum of ext. ∠s of polygon)

3 x + y = 280° ............................ (1)
(180° − 70° − 80°) + (180° − x) = y + y (ext. ∠ of )
210° − x = 2 y
x + 2 y = 210° ......... (2)
(1) × 2: 6 x + 2 y = 560° ................................. (3)
(3) − (2): (6 x + 2 y ) − ( x + 2 y ) = 560° − 210°
5 x = 350°
x = 70°
Substitute x = 70° into (1),
3(70°) + y = 280°
y = 70°
54. In AFE and CFB,

∠AFE = ∠CFB (vert. opp. ∠s)
∠FAE = ∠FCB (alt. ∠s, AD // BC)
∠FEA = ∠FBC (alt. ∠s, AD // BC)
∴  AFE ~ CFB (A.A.A.)
AF AE
CF CB
AE
=
AD
1
AD
= 3
AD
1
=
3
∴ AF : FC = 1: 3


IP06 Sol S2A E Web

Uploaded by

Copyright:

Available Formats

IP06 Sol S2A E Web

Uploaded by

Document Information

Original Title

Copyright

Available Formats

Share this document

Share or Embed Document

Sharing Options

Did you find this document useful?

Is this content inappropriate?

Copyright:

Available Formats

IP06 Sol S2A E Web

Uploaded by

Copyright:

Available Formats

New Effective Learning Mathematics S2A

Integrated Practice VI Solution Guide

© 2021 Chung Tai Educational Press Limited |

© 2021 Chung Tai Educational Press Limited |

© 2021 Chung Tai Educational Press Limited |

(3) − (2): (3x + 12 y ) − (3x − y ) = 21 − 8 1M

3 x + 8 y = −18 ........................ (1)

10. (a) 2 x = ( x + 10°) + 40° (ext. ∠ of ) 1M

© 2021 Chung Tai Educational Press Limited |

11. (a) 5 x + 72° + 160° + 78° + 100° = (5 − 2) × 180°

(b) x + 4 x + 32° + 90° + 133° + 90° = (6 − 2) × 180°

12. (a) x + 2 x + 131° + 61° = 360° (sum of ext. ∠s of polygon) 1M

(b) 4 x + (3 x + 2°) + 90° + 25° + 75° + 70° = 360°

13. (a)  DEF ~  XYZ (given)

(b)  LMN ~ IJK (given)

14. (a) Substitute x = 4 into the equation,

(b) Substitute x = 1 and y = 2 into the equation,

© 2021 Chung Tai Educational Press Limited |

15. (a) Size of each interior angle

(b) Let n be the number of sides of the regular polygon.

16. Let x g and y g be the weights of a cupcake and a piece of

17. Let x and y be the original numbers of stamps owned by

© 2021 Chung Tai Educational Press Limited |

18. Let x and y be the numbers of apples and pears Kary

© 2021 Chung Tai Educational Press Limited |

21. Rewrite the simultaneous equations as follows:

(3) + (4): (2 y − 3 x) + (12 y + 3 x) = 34 + 36 1M

3 x = −2( y + 2) ........... (1)

(b) Let x = a + 1 and y = b − 3 . 1M

© 2021 Chung Tai Educational Press Limited |

23.  ABC ~ PQR (given)

Substitute x = 30° into (1),

24. Each interior angle of a regular pentagon

Substitute y = 20° into (1),

25. Let n be the number of sides of the regular polygon.

© 2021 Chung Tai Educational Press Limited |

26. (a) In CBA and ABD,

(b)  CBA ~  ABD (proved)

27. (a) Sum of interior angles of a regular 12-sided polygon

28. Let x and y be the original numbers of jelly beans Stanley

© 2021 Chung Tai Educational Press Limited |

29. Let x and y be the numbers of bottles of juice and others

30. Let x and y be the numbers of boys and girls respectively.

(1) × (3): 3 x + 3 y = 894 ...... (3)

31. Let $x and $y be the original prices of a lemon tart and a

original price of a cup of coffee is $35. 1A + 1A

© 2021 Chung Tai Educational Press Limited |

32. Let m be the number of stickers owned by Paul.

33. (a) Let a and b be the original numbers of $2 coins and $5

(b) Original amount of money : New amount of money

34. (a)   ABC ≅  AED (given)

© 2021 Chung Tai Educational Press Limited |

(b) Each interior angle of a regular pentagon

35. (a) In APQ and ACB,

(b)  △ APQ ~  ACB (proved)

(c) In PBC and PQC,

36. (a) In ABC and DEF,

(b) In ACD and DFA,

© 2021 Chung Tai Educational Press Limited |