中国語
中国語
Uploaded by
happy.world.0230Copyright:
Available Formats
中国語
中国語
Uploaded by
happy.world.0230Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
中国語
中国語
Uploaded by
happy.world.0230Copyright:
Available Formats
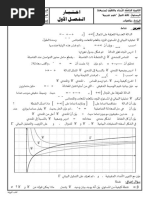
( ) f (x .
y )
= ecosy
e cosy Taylov
fxi ) = ( 1 txt t …) ( + … )
= txt ( ) + ( ”) th . ot
hots 3 (
Gighooderterm)
3 2
2 y
= ha ( xy) =
x
G xy ) &
h( y ) “
y
f (2xc ) = ( l- xt …) ( t * t …
)
=+ xt ) + ( x + )+ ( t )
ha
↑
;( * )
_- xt ( )
th . ot
: )
” 2
f (x ) ÷ ( ) Y
3 ( - Py
{ fe ( az )
C =
2 x
- :
O … 0
fy ( xcg ) = -
2 ( x py =
- 0
. …
Y =
O = .
Y θ 0 .
= 1 9 fxlx ) =
2 0 .
( Y) =
10 o ) u
{
fxx (x % )
{
2
( )
2 =
2 -
6 ( )
x y fxr ( o 0 ).
= 2
fag ( x ) fyx (x % )
.
= = - 6( x - i )y feyl ) = fx ( oo ) = 0
3
fyy ( & ) = -
2 ( x-
) fyy ( 00 ) :
2
D (0 0 )
= fxx ( o 0 ) fyy ( . 0 0
)
-( * ) fyx (0 o
=
) 22 - 0
. = 0 4
.
You might also like
- All Solutions Are Also Done in Excel FileDocument5 pagesAll Solutions Are Also Done in Excel FilemauweberNo ratings yet
- 中国語 2Document1 page中国語 2happy.world.0230No ratings yet
- 中国語 3Document1 page中国語 3happy.world.0230No ratings yet
- ScratchbookDocument7 pagesScratchbookbekhzod5plusNo ratings yet
- Onda de RoyDocument6 pagesOnda de RoyIng. Arnulfo Guerra MadridNo ratings yet
- Cálculo Vectorial 13Document1 pageCálculo Vectorial 132rm2.montesolguinianNo ratings yet
- موضوع اختبار الفصل الأول -الرياضيات -الثالثة ثانوي علوم تجريبيةDocument2 pagesموضوع اختبار الفصل الأول -الرياضيات -الثالثة ثانوي علوم تجريبيةmounia.blz18No ratings yet
- 05 Differential EntropyDocument8 pages05 Differential Entropy林冠廷No ratings yet
- Cal 3Document3 pagesCal 3cardevillossierNo ratings yet
- Clase Integral Semana 2-2Document12 pagesClase Integral Semana 2-2Lucy GuerreroNo ratings yet
- Asig. Cálculo Pp. 262, 272, 282 PDFDocument9 pagesAsig. Cálculo Pp. 262, 272, 282 PDFValeria RodriguezNo ratings yet
- Y Ctrax)Document8 pagesY Ctrax)yongwhan522No ratings yet
- Convolution and Correlation - TutorialspointDocument12 pagesConvolution and Correlation - TutorialspointSavita BhosleNo ratings yet
- Pochodne-wzory_ver2Document1 pagePochodne-wzory_ver2Quevosh 75No ratings yet
- 3 NovDocument2 pages3 Novcamila moreno benitesNo ratings yet
- Doubts +2 24 OctDocument9 pagesDoubts +2 24 Octdhruvverma7580No ratings yet
- I / & P Á Þ o Æ II (MATH162)Document3 pagesI / & P Á Þ o Æ II (MATH162)서정민No ratings yet
- Solución Parcial CD (Inter 2024-1)Document3 pagesSolución Parcial CD (Inter 2024-1)isaacdeclan6No ratings yet
- Green Functions and PropagatorsDocument9 pagesGreen Functions and PropagatorsSilina AmimerNo ratings yet
- dzexams-3as-mathematiques-367395Document5 pagesdzexams-3as-mathematiques-367395bendaifNo ratings yet
- FfffDocument5 pagesFffflolibouaouadjaNo ratings yet
- Capitulo 4Document72 pagesCapitulo 4Wagner Sousa SantosNo ratings yet
- Ecuación de Onda HugoDocument4 pagesEcuación de Onda HugoIng. Arnulfo Guerra MadridNo ratings yet
- Lab1 Intro Lissajous PDFDocument2 pagesLab1 Intro Lissajous PDFmldgmNo ratings yet
- Bloc de notas sin título (432)Document21 pagesBloc de notas sin título (432)ryefastNo ratings yet
- 이름 없는 노트북Document4 pages이름 없는 노트북Sieun ChoNo ratings yet
- Lecture 11Document8 pagesLecture 11hkspider7889No ratings yet
- Ôn HBPDocument5 pagesÔn HBPNgô Thị Hồng AnhNo ratings yet
- 5.3 Fundamental Theorem of CalculusDocument3 pages5.3 Fundamental Theorem of Calculusdamiotukoya06No ratings yet
- Note 4Document1 pageNote 4shuu.ee12No ratings yet
- 신시 정리 노트 - 중간Document10 pages신시 정리 노트 - 중간dlacofls007No ratings yet
- Assignment 2Document2 pagesAssignment 2bedour.alsultanNo ratings yet
- Scan 04 Oct. 24 13 38 11Document10 pagesScan 04 Oct. 24 13 38 11Andres Leonardo Algaranaz RiosNo ratings yet
- TH THDocument8 pagesTH THDuna areny molneNo ratings yet
- Ahmad-Comment On Kung and TraubDocument11 pagesAhmad-Comment On Kung and Traubnalaazana55No ratings yet
- Homework 3 - 1D FDTD Update EquationsDocument2 pagesHomework 3 - 1D FDTD Update Equationspearl301010No ratings yet
- 5.4 The Fundamental Theorem of Calculus HWDocument17 pages5.4 The Fundamental Theorem of Calculus HWkangdw030407No ratings yet
- 31 JanDocument7 pages31 JannhuzoraNo ratings yet
- Laplace PDEDocument15 pagesLaplace PDEhaikalirfan2003No ratings yet
- C. Mate Sup 1Document5 pagesC. Mate Sup 1oliver basurto michueNo ratings yet
- 202 PS2 NotesDocument10 pages202 PS2 Notesİrem Ece BayramNo ratings yet
- Ecuaciones Diferenciales 2Document2 pagesEcuaciones Diferenciales 2paomora2001.22No ratings yet
- T14 2 PDFDocument8 pagesT14 2 PDFJorge ValenzuelaNo ratings yet
- T14 PDFDocument6 pagesT14 PDFJorge ValenzuelaNo ratings yet
- HW 8,10 14Document21 pagesHW 8,10 14wangandy1105No ratings yet
- 02 Log Et Exp1 BacDocument7 pages02 Log Et Exp1 Bacsalmasadouki2003No ratings yet
- Actividad 5Document4 pagesActividad 5gusvorop417No ratings yet
- Cal hw14Document1 pageCal hw14butterfly2434butterNo ratings yet
- 醫學系 2024 02 27 題庫Document16 pages醫學系 2024 02 27 題庫bruce10931052No ratings yet
- Assignment2 1Document9 pagesAssignment2 1chienhsu1222No ratings yet
- Sổ ghi chép chưa đặt tên 16Document9 pagesSổ ghi chép chưa đặt tên 16Nguyên Khôi NguyễnNo ratings yet
- 광주과학고등학교 수학세미나Ⅱ문제Document70 pages광주과학고등학교 수학세미나Ⅱ문제남은우No ratings yet
- Homework OneDocument2 pagesHomework Onejojojoy9091No ratings yet
- Lecture 2Document6 pagesLecture 2jmohamadNo ratings yet
- 12 - MatekDocument7 pages12 - MatekÉdua AmbrusNo ratings yet
- Tarea para El ExamenDocument3 pagesTarea para El ExamenEMILIANO GUILLEN MEDINANo ratings yet
- מבחן ראשון 2Document1 pageמבחן ראשון 2agamshaul2007No ratings yet
- Lecture 04 - Parametric MethodsDocument8 pagesLecture 04 - Parametric MethodsAtaNo ratings yet
- 이름 없는 노트북Document3 pages이름 없는 노트북Sieun ChoNo ratings yet
- 3eme SC Exama s1Document12 pages3eme SC Exama s1Amna DernouniNo ratings yet
- Essay Writing About Radio and TelevisionDocument2 pagesEssay Writing About Radio and TelevisionYoni RibkaNo ratings yet
- Oprah: A Biography 1st Edition Kelley 2024 Scribd DownloadDocument62 pagesOprah: A Biography 1st Edition Kelley 2024 Scribd DownloadkaspaigoneNo ratings yet
- message 4Document7 pagesmessage 42mhmwzsqz6No ratings yet
- Current Role of Stroboscopy in Laryngeal ImagingDocument8 pagesCurrent Role of Stroboscopy in Laryngeal Imagingmajid mirzaeeNo ratings yet
- Satellite JammingDocument76 pagesSatellite JammingSiawasch MohammadiNo ratings yet
- BR Pluto.m3uDocument2 pagesBR Pluto.m3utclt9146No ratings yet
- Click To Edit Master Subtitle StyleDocument4 pagesClick To Edit Master Subtitle Styleayu mauliraNo ratings yet
- Samsung - UN32C5000 - 40C5000 - 46C5000 MAIN BOARD - Schematic PDFDocument16 pagesSamsung - UN32C5000 - 40C5000 - 46C5000 MAIN BOARD - Schematic PDFjose4445No ratings yet
- Agatha Christie Stories Retold For Younger People's Minds Free Download, Borrow, and Streaming Internet Archive 2Document1 pageAgatha Christie Stories Retold For Younger People's Minds Free Download, Borrow, and Streaming Internet Archive 22022carmenamoraNo ratings yet
- đáp án kiểm tra giữa kì 1 khối 11 2023 2024Document2 pagesđáp án kiểm tra giữa kì 1 khối 11 2023 2024Dương HuyềnNo ratings yet
- Friends 'Blind Dates' WorksheetDocument5 pagesFriends 'Blind Dates' WorksheetMaria Lorena100% (4)
- Friends S3E9Document3 pagesFriends S3E9Ver Net25% (4)
- The Walking Dead RPG Starter Set TV CharactersDocument8 pagesThe Walking Dead RPG Starter Set TV CharactersSte Mackenzie-Hawkins100% (2)
- 315 The One Where Ross and Rachel Take A BreakDocument14 pages315 The One Where Ross and Rachel Take A Break이용진No ratings yet
- Series List - LatestDocument11 pagesSeries List - LatestSergio YepesNo ratings yet
- a295 - Ngữ Pháp Tiếng Anh (Mai Lan Hương)Document280 pagesa295 - Ngữ Pháp Tiếng Anh (Mai Lan Hương)Hong Nhung NguyenNo ratings yet
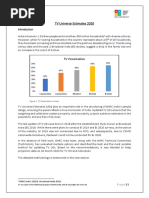
- Barc India TV Universe Estimates 2020Document9 pagesBarc India TV Universe Estimates 2020Sourav_Dhar_7249No ratings yet
- Genres of Viewing Grade 7Document30 pagesGenres of Viewing Grade 7Shiela Mae FloresNo ratings yet
- Sample Questions For Preparation of Test One of TV and Video Tech For OD13T and Beng 15Document2 pagesSample Questions For Preparation of Test One of TV and Video Tech For OD13T and Beng 15Issa BakariNo ratings yet
- Closed Circuit Television (CCTV)Document3 pagesClosed Circuit Television (CCTV)Maria CincoNo ratings yet
- Let S Find PokemonDocument24 pagesLet S Find PokemonShivaprakash Jagalur MuttNo ratings yet
- List of Top Withholding Agents For Inclusion Individual P-46 PDFDocument1 pageList of Top Withholding Agents For Inclusion Individual P-46 PDFQuiar YangaNo ratings yet
- Vdocuments - MX Mohamed Diab Electrical Engineer CVDocument12 pagesVdocuments - MX Mohamed Diab Electrical Engineer CVMelNo ratings yet
- Daftar Nilai Rapor KLS Xii SM1 1718Document13 pagesDaftar Nilai Rapor KLS Xii SM1 1718Yani Febriyani TaryonoNo ratings yet
- Genderbend Hazbin Hotel - Radio Killed The Video StarDocument26 pagesGenderbend Hazbin Hotel - Radio Killed The Video StarAndreea CiocodeicaNo ratings yet
- Speaking Grade 10Document2 pagesSpeaking Grade 10Phương DungNo ratings yet
- Ninye's TLA EssayDocument9 pagesNinye's TLA EssayNinye MugambeNo ratings yet
- Biznet Hospitality (Read-Only)Document1 pageBiznet Hospitality (Read-Only)Idho De' Saouza Al-ghifaryNo ratings yet
- Morning News RankerDocument1 pageMorning News RankerKrystal SwannNo ratings yet