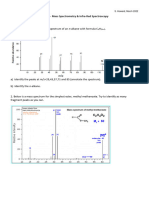
NMR 1
NMR 1
Uploaded by
Jyoti ChaturvediCopyright:
Available Formats
NMR 1
NMR 1
Uploaded by
Jyoti ChaturvediOriginal Title
Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
NMR 1
NMR 1
Uploaded by
Jyoti ChaturvediCopyright:
Available Formats
1
Introductory to NMR Spectroscopy
Ref:
1. NMR Spectroscopy, Basic Principles and Applications, by Roger S. Macomber
2. http://www.cis.rit.edu/htbooks/nmr/ by Joseph P. Hornak
3. Some figures copy from the web page by Guillermo Moyna, University of the
Sciences in Philadelphia
4. Wthrich, K. NMR of Proteins and Nucleic Acids, Wiley, 1986. 1994
5. Cavanagh, J. et al., Protein NMR Spectroscopy-Principles and Practice,
Academic Press, 1996.
6. Van de Ven, F.J. (1995), Multi-dimensional NMR in Liquid-Basic Principles &
Experimental Methods. VCH Publishing
2
1nm
10
10
2
10
3
10
4
10
5
10
6
10
7
(the wave) X-ray UV/VIS Infrared Microwave Radio
Frequency
(the transition) electronic Vibration Rotation Nuclear
(spectrometer) X-ray UV/VIS Infrared/Raman NMR
Fluorescence
NMR Spectroscopy
Where is it?
3
NMR Historic Review
1924 Pauli proposed the presence of nuclear magnetic moment to explain the
hyperfine structure in atomic spectral lines.
1930 Nuclear magnetic moment was detected using refined Stern-Gerlach
experiment by Estermann.
1939 Rabi et al. First detected unclear magnetic resonance phenomenon by
applying r.f. energy to a beam of hydrogen molecules in the Stern-Gerach
set up and observed measurable deflection of the beam.
1946 Purcell et al. at Harvard reported nuclear resonance absorption in paraffin
wax.
Bloch et al. at Stanford found nuclear resonance in liquid water.
1949 Chemical shift phenomenon was observed.
1952 Nobel prize in Physics was awarded to Purcell and Bloch.
1966 Ernst and Anderson first introduce the Fourier Transform technique into
NMR.
Late in the 1960s:
Solid State NMR was revived due to the effort of Waugh.
and associates at MIT.
Biological application become possible due to the introduction
superconducting magnets.
NMR imaging was demonstrated.
1970 2D NMR was introduced.
NMR Historic Review
4
NMR Historic Review
1924 Pauli proposed the presence of nuclear magnetic moment to explain the
hyperfine structure in atomic spectral lines.
1930 Nuclear magnetic moment was detected using refined Stern-Gerlach
experiment by Estermann.
1939 Rabi et al. First detected unclear magnetic resonance phenomenon by
applying r.f. energy to a beam of hydrogen molecules in the Stern-Gerach
set up and observed measurable deflection of the beam.
1946 Purcell et al. at Harvard reported nuclear resonance absorption in paraffin
wax.
Bloch et al. at Stanford found nuclear resonance in liquid water.
1949 Chemical shift phenomenon was observed.
1952 Nobel prize in Physics was awarded to Purcell and Bloch.
1966 Ernst and Anderson first introduce the Fourier Transform technique into
NMR.
Late in the 1960s:
Solid State NMR was revived due to the effort of Waugh.
and associates at MIT.
Biological application become possible due to the introduction
superconducting magnets.
NMR imaging was demonstrated.
1970 2D NMR was introduced.
1980s Macromolecular structure determination in solution by NMR was
achieved.
1991 Nobel prize in Chemistry was awarded to Richard Ernst.
1990s Continuing development of heteronuclear multi-dimensional NMR permit
the determination of protein structure up to 50 KDa.
MRI become a major radiological tool in medical diagnostic.
NMR Applications
(One of themost, if not the most, important analytical spectroscopic tool.)
1. Biomedical applications:
a. Metabolic studies of biological systems.
b. MRI diagnostic imaging, flow imaging, chemical shift imaging, functional
imaging.
c. Macromolecular structure determination in solution.
2. Chemical-related research:
a. Analytical tool.
b. Structural characterization of chemical compounds.
3. Material-related research:
a. Polymer characterization.
b. C60 (Fullerene).
c. High temperature superconductor research.
d. Heterogeneous catalysis (Ziolite).
e. Surface physics.
Basic Properties of NMR
1. A multi-parameter spectroscopic technique.
2. Relatively insensitive.
3. Rather expensive.
2002 Nobel prize in Chemistry was awarded to Kurt Wuthrich
NMR is a versatile tool and it has applications in wide varieties of subjects
in addition to its chemical and biomedical applications, including material
and quantum computing.
5
Felix Bloch
1905-1983
Edward M. Purcell
1912-1997
Kurt Wuthrich
1938-
Richard R. Ernst
1933-
CW NMR 40MHz
1960
6
800 MHz
7
The problem the we want to solve by NMR
What we really
see
What we want to see
NMR
8
Before using NMR
What are N, M, and R ?
Properties of the Nucleus
Nuclear spin
Nuclear magnetic moments
The Nucleus in a Magnetic Field
Precession and the Larmor frequency
Nuclear Zeeman effect & Boltzmann distribution
When the Nucleus Meet the right Magnet and radio wave
Nuclear Magnetic Resonance
9
Nuclear spin
Nuclear spin is the total nuclear angular momentum quantum number.
This is characterized by a quantum number I, which may be integral,
half-integral or 0.
Only nuclei with spin number I = 0 can absorb/emit electromagnetic
radiation. The magnetic quantum number m
I
has values of I, -I+1, ..+I .
( e.g. for I=3/2, m
I
=-3/2, -1/2, 1/2, 3/2 )
1. A nucleus with an even mass A and even charge Z nuclear spin I is
zero
Example:
12
C,
16
O,
32
S No NMR signal
2. A nucleus with an even mass A and odd charge Z integer value I
Example:
2
H,
10
B,
14
N NMR detectable
3. A nucleus with odd mass A I=n/2, where n is an odd integer
Example:
1
H,
13
C,
15
N,
31
P NMR detectable
Properties of the Nucleus
10
Nuclear magnetic moments
Magnetic moment is another important parameter for a nuclei
= I (h/2t)
I: spin number; h: Plank constant;
: gyromagnetic ratio (property of a nuclei)
1
H: I=1/2 , = 267.512 *10
6
rad T
-1
S
-1
13
C: I=1/2 , = 67.264*10
6
15
N: I=1/2 , = 27.107*10
6
11
AT 71000 GAUSS (7.1 TELSLA)
(1T = 10,000G)
W
0
(MHz) 0 30 75 121 280 300 320
Nucleus
15
N
13
C
31
P
19
F
1
H
3
H
Table 1.1 Nuclei of Major Interest to NMR Spectroscopists
Iostope
Abundance
(%)
Spin
2
10
-8b
Relative
c
sensitivity
0
at
1T(MHz)
At 7.04T
1
H 99.9844 1 1/2 2.7927 2.6752 1.000 42.577 300
2
H 0.0156 1 1 0.8574 0.4107 0.00964 6.536 46
10
B
18.83 5 3 1.8006 0.2875 0.0199 4.575
11
B
81.17 5 3/2 2.6880 0.8583 0.165 13.660
13
C
1.108 6 1/2 0.7022 0.6726 0.0159 10.705 75.4
14
N
99.635 7 1 0.4036 0.1933 0.00101 3.076
15
N
0.365 7 1/2 -0.2830 -0.2711 0.00104 4.315 30.4
19
F
100 9 1/2 2.6273 2.5167 0.834 40.055 282.3
29
Si
4.70 14 1/2 -0.5548 -0.5316 0.0785 8.460
31
P
100 15 1/2 1.1305 1.0829 0.0664 17.235 121.4
a Magnetic moment in units of the nuclear magneton, eh/(M
p
c)
b Magnetogyric ratio in SI units
c For equal numbers of nuclei at constant field
12
The Nucleus in a Magnetic Field
Precession and the Larmor frequency
The magnetic moment of a spinning nucleus processes with a characteristic
angular frequency called the Larmor frequency e, which is a function of r and B
0
Remember = I (h/2t) ?
Angular momentum dJ/dt= x B
0
Larmor frequency e=rB
0
Linear precession frequency v=e/2t= rB
0
/2t
Example: At what field strength do
1
H process at a frequency of 600.13MHz? What would be the
process frequency for
13
C at the same field?
J
13
Nuclear Zeeman effect
Zeeman effect: when an atom is placed in an external magnetic field,
the energy levels of the atom are split into several states.
The energy of a give spin sate (E
i
) is directly proportional to the value
of m
I
and the magnetic field strength B
0
Spin State Energy E
I
=- . B
0
=-m
I
B
0
r(h/2p)
Notice that, the difference in energy will always be an integer multiple
of B
0
r(h/2p). For a nucleus with I=1/2, the energy difference between
two states is
E=E
-1/2
-E
+1/2
= B
0
r(h/2p)
m=1/2
m=+1/2
The Zeeman splitting is proportional to the strength of the magnetic
field
14
Boltzmann distribution
Quantum mechanics tells us that, for net absorption of radiation to occur,
there must be more particles in the lower-energy state than in the higher
one. If no net absorption is possible, a condition called saturation.
When its saturated, Boltzmann distribution comes to rescue:
P
m=-1/2
/ P
m=+1/2
= e
-DE/kT
where P is the fraction of the particle population in each state,
T is the absolute temperature,
k is Boltzmann constant 1.381*10
-28
JK
-1
Example: At 298K, what fraction of
1
H nuclei in 2.35 T field are in the upper and
lower states? (m=-1/2 : 0.4999959 ; m=1/2 : 0.5000041 )
The difference in populations of the two states is only on the order of
few parts per million. However, this difference is sufficient to generate
NMR signal.
Anything that increases the population difference will give rise to a more
intense NMR signal.
15
For a particle to absorb a photon of electromagnetic radiation, the particle must
first be in some sort of uniform periodic motion
If the particle uniformly periodic moves (i.e. precession)
at v
precession,
and absorb erengy. The energy is E=hv
precession
For I=1/2 nuclei in B
0
field, the energy gap between two spin states:
AE=rhB
0
/2t
The radiation frequency must exactly match the precession frequency
E
photon
=hv
precession
=hv
photon
=AE=rhB
0
/2t
This is the so called Nuclear Magnetic RESONANCE!!!!!!!!!
v
AE =hv
photon
When the Nucleus Meet the Magnet
Nuclear Magnetic Resonance
16
Nuclear Magnetic Resonance Spectrometer
How to generate signals?
B
0
: magnet
B
1
: applied small energy
17
Magnet B
0
and irradiation energy B
1
B
0
( the magnet of machine)
(1) Provide energy for the nuclei to spin
E
i
=-m
i
B
0
(rh/2t)
Larmor frequency e=rB
0
(2) Induce energy level separation (Boltzmann distribution)
The stronger the magnetic field B
0
, the greater separation
between different nuclei in the spectra
Av =v
1
-v
2
=(r
1
-r
2
)B
0
/2t
(3) The nuclei in both spin states are randomly oriented around the z axis.
M
z
=M, M
xy
=0
( where M is the net
nuclear magnetization)
18
What happen before irradiation
Before irradiation, the nuclei in both spin states are processing with
characteristic frequency, but they are completely out of phase, i.e., randomly
oriented around the z axis. The net nuclear magnetization M is aligned statically
along the z axis (M=M
z
, M
xy
=0)
19
What happen during irradiation
When irradiation begins, all of the individual nuclear magnetic moments become
phase coherent, and this phase coherence forces the net magnetization vector M
to process around the z axis. As such, M has a component in the x, y plan,
M
xy
=Msino. o is the tip angle which is determined by the power and duration of
the electromagnetic irradiation.
o
M
o
z
x
B
1
y
e
o
x
M
xy
y
90 deg pulse o deg pulse
20
What happen after irradiation ceases
After irradiation ceases, not only do the population of the states revert to a
Boltzmann distribution, but also the individual nuclear magnetic moments begin to
lose their phase coherence and return to a random arrangement around the z axis.
(NMR spectroscopy record this process!!)
This process is called relaxation process
There are two types of relaxation process : T1(spin-lattice relaxation) & T2(spin-
spin relaxation)
21
B
1
(the irradiation magnet, current induced)
(1) Induce energy for nuclei to absorb, but still spin at e or v
precession
E
photon
=hv
photon
=AE=rhB
0
/2t=hv
precession
And now, the spin jump to the higher energy ( from m=1/2m= 1/2)
(2) All of the individual nuclear magnetic moments become phase
coherent, and the net M process around the z axis at o angel
M
z
=Mcoso
M
xy
=Msino.
m= 1/2
m= 1/2
22
T1
(the spin lattice relaxation)
How long after immersion in a external field does it take for a collection of nuclei
to reach Boltzmann distribution is controlled by T1, the spin lattice relaxation time.
(major Boltzmann distribution effect)
Lost of energy in system to surrounding (lattice) as heat
( release extra energy)
Its a time dependence exponential decay process of Mz components
dM
z
/dt=-(M
z
-M
z,eq
)/T1
23
T2
(the spin spin relaxation)
This process for nuclei begin to lose their phase coherence and return to a random
arrangement around the z axis is called spin-spin relaxation.
The decay of M
xy
is at a rate controlled by the spin-spin relaxation time T2.
dM
x
/dt=-M
x
/T2
dM
y
/dt=-M
y
/T2
dephasing
24
NMR Relaxation
25
Collecting NMR signals
The detection of NMR signal is on the xy plane. The oscillation of Mxy generate a
current in a coil , which is the NMR signal.
Due to the relaxation process, the time dependent spectrum of nuclei can be
obtained. This time dependent spectrum is called free induction decay (FID)
time
M
xy
time
(if theres no relaxation ) (the real case with T1 &T2)
26
In addition, most molecules examined by NMR have several sets of nuclei, each
with a different precession frequency.
The FID (free induction decay) is then Fourier transform to frequency domain
to obtain each v
pression
( chemical shift) for different nuclei.
Time (sec)
frequency (Hz)
27
Fourier transformation (FT)
FT
FT
28
AT 71000 GAUSS (7.1 TELSLA)
(1T = 10,000G)
W
0
(MHz) 0 30 75 121 280 300 320
Nucleus
15
N
13
C
31
P
19
F
1
H
3
H
Table 1.1 Nuclei of Major Interest to NMR Spectroscopists
Iostope
Abundance
(%)
Spin
2
10
-8b
Relative
c
sensitivity
0
at
1T(MHz)
At 7.04T
1
H 99.9844 1 1/2 2.7927 2.6752 1.000 42.577 300
2
H 0.0156 1 1 0.8574 0.4107 0.00964 6.536 46
10
B
18.83 5 3 1.8006 0.2875 0.0199 4.575
11
B
81.17 5 3/2 2.6880 0.8583 0.165 13.660
13
C
1.108 6 1/2 0.7022 0.6726 0.0159 10.705 75.4
14
N
99.635 7 1 0.4036 0.1933 0.00101 3.076
15
N
0.365 7 1/2 -0.2830 -0.2711 0.00104 4.315 30.4
19
F
100 9 1/2 2.6273 2.5167 0.834 40.055 282.3
29
Si
4.70 14 1/2 -0.5548 -0.5316 0.0785 8.460
31
P
100 15 1/2 1.1305 1.0829 0.0664 17.235 121.4
a Magnetic moment in units of the nuclear magneton, eh/(M
p
c)
b Magnetogyric ratio in SI units
c For equal numbers of nuclei at constant field
29
NMR signals
We have immersed our collection of nuclei in a magnetic field, each is processing with
a characteristic frequency, To observe resonance, all we have to do is irradiate them
with electromagnetic radiation of the appropriate frequency.
Its easy to understand that different nucleus type will give different NMR signal.
(remember v =e/2t= B
0
/2t ? Thus, different cause different v !! )
However, it is very important to know that for same nucleus type, but different
nucleus could generate different signal. This is also what make NMR useful and
interesting.
Depending on the chemical environment, there are variations on the magnetic field
that the nuclei feels, even for the same type of nuclei.
The main reason for this is, each nuclei could be surrounded by different electron
environment, which make the nuclei feel different net magnetic field , B
effect
30
Basic Nuclear Spin Interactions
Nuclear Spin i
Nuclear Spin j
Electrons
Phonons
3
1
Dominant interactions: H = H
Z
+ H
D
+ H
S
+ H
Q
.
H
Z
= Zeeman Interaction H
D
= Dipolar Interactions
H
S
= Chemical Shielding Interaction. H
Q
= Quadrupolar Interaction
6
H
o
H
o
4
5
4
3
1 1
4
31
NMR Parameters
Chemical Shift
The chemical shift of a nucleus is the difference between the resonance frequency
of the nucleus and a standard, relative to the standard. This quantity is reported
in ppm and given the symbol delta,
o = (v - v
REF
) x10
6
/ v
REF
In NMR spectroscopy, this standard is often tetramethylsilane, Si(CH
3
)
4
,
abbreviated TMS, or 2,2-dimethyl-2-silapentane-5-sulfonate, DSS, in
biomolecular NMR.
The good thing is that since it is a relative scale, the o for a sample in a 100 MHz
magnet (2.35 T) is the same as that obtained in a 600 MHz magnet (14.1 T).
0
TMS
ppm
2 10 7 5 15
Aliphatic
Alcohols, protons o
to ketones
Olefins
Aromatics
Amides
Acids
Aldehydes Shielded
(up field)
Deshielded
(low field)
32
Electron surrounding each nucleus in a molecule serves to shield that
nucleus from the applied magnetic field. This shielding effect cause the
DE difference, thus, different v will be obtained in the spectrum
B
eff
=B
0
-B
i
where B
i
induced by cloud electron
B
i
= sB
0
where s is the shielding constant
B
eff
=(1-s) B
0
v
precession
= (rB
0
/2p) (1-s)
s=0 naked nuclei
s >0 nuclei is shielded by electron cloud
s <0 electron around this nuclei is withdraw , i.e.
deshielded
Example: Calculate the chemical shifts of a sample that contains two signals
( 140Hz & 430 Hz using 60MHz instrument; 187Hz & 573 Hz using 80MHz
instrument). (2.33ppm & 7.17ppm)
Example: The 60MHz 1H spectrum of CH3Li shows a signal at 126 Hz upfield of
TMS. What is its chemical shift? (-2.10ppm)
33
HO-CH
2
-CH
3
e
o
low
field
high
field
e
0
=rB
effect
Notice that the intensity of peak is proportional to the number of H
34
1H
13C
Example of 1D : 1H spectra, 13C spectra of Codeine C
18
H
21
NO
3
, MW= 299.4
35
J-coupling
Nuclei which are close to one another could cause an influence on each other's
effective magnetic field. If the distance between non-equivalent nuclei is less than or
equal to three bond lengths, this effect is observable. This is called spin-spin coupling
or J coupling.
1 3
C
1
H
1
H
1
H
one-bond
three-bond
Each spin now seems to has two energy sub-levels depending on the state of the spin it
is coupled to:
The magnitude of the separation is called coupling constant (J) and has units of Hz.
oo
o| |o
||
I S
S
S
I
I
J (Hz)
36
Single spin:
One neighboring spins: - CH CH -
Two neighboring spins: - CH
2
CH -
N neighboring spins: split into N + 1 lines
From coupling constant (J) information, dihedral angles can be derived ( Karplus
equation)
8 . 1 cos 6 . 1 cos 5 . 9
8 . 1 ) 120 cos( 6 . 1 ) 120 ( cos 5 . 9
9 . 1 ) 60 cos( 4 . 1 ) 60 ( cos 4 . 6
1 1
2
2
3
1 1
2
1
3
2 3
+ =
+ =
+ =
_ _
_ _
| |
o|
o|
o
J
J
J
NH
C
C
C
N
2
C
N
37
Nuclear Overhauser Effect (NOE)
The NOE is one of the ways in which the system (a certain spin) can release energy.
Therefore, it is profoundly related to relaxation processes. In particular, the NOE is
related to exchange of energy between two spins that are not scalarly coupled (J
IS
= 0),
but have dipolar coupling.
The NOE is evidenced by enhancement of certain signals in the spectrum when the
equilibrium (or populations) of other nearby are altered. For a two spin system, the
energy diagram is as follwing:
oo
o|
||
W
1S
W
1S
W
1I
W
1I
|o
W
2IS
W
0IS
W represents a transition probability, or the rate at which certain transition can take
place. For example, the system in equilibrium, there would be W
1I
and W
1S
transitions,
which represents single quantum transitions.
38
NOE could provide information of distance between two atoms:
NOE / NOE
std
= r
std
6
/ r
6
Thus, NOE is very important parameter for structure determination of
macromolecules
39
Relaxation Rates
The Bloch Equations:
dM
x
(t) / dt = [ M
y
(t) * B
z
- M
z
(t) * B
y
] - M
x
(t) / T
2
dM
y
(t) / dt = [ M
z
(t) * B
x
- M
x
(t) * B
z
] - M
y
(t) / T
2
dM
z
(t) / dt = [ M
x
(t) * B
y
- M
y
(t) * B
x
] - ( M
z
(t) - M
o
) / T
1
The relaxation rates of the longitudinal magnetization, T1, determine the
length of the recycle delay needed between acquisitions, and the relaxation rates
T2 determine the line width of the signal.
Relaxation could also provide experimental information on the physical
processes governing relaxation, including molecular motions (dynamics).
40
1. Chemical Shift Indices: Determining secondary structure.
2. J-coupling: Determine dihedral angles.
(Karplus equation)
.
3. Nuclear Overhauser Effect (NOE):
Determine inter-atomic distances (NOE R
-6
)
.
4. Residual dipolar coupling:
Determine bond orientations.
.
5. Relaxation rates (T
1
, T
2
etc):
Determine macromolecular dynamics.
NMR Parameters employed for determining protein structure
R
1
H
1
H
15
N
1
H
u
B
O
I
t
41
Steps for NMR Experiment
()
42
1. Sample preparation ()
Which buffer to choose? Isotopic labeling?
Best temperature?
Sample Position ?
Preparation for NMR Experiment
N S
2. Whats the nucleus or prohead? ()
A nucleus with an even mass A and even charge Z nuclear spin I is zero
Example:
12
C,
16
O,
32
S No NMR signal
A nucleus with an even mass A and odd charge Z integer value I
Example:
2
H,
10
B,
14
N NMR detectable
A nucleus with odd mass A I=n/2, where n is an odd integer
Example:
1
H,
13
C,
15
N,
31
P NMR detectable
43
3. The best condition for NMR Spectrometer? ()
Wobble : Tune & Match & Shimming
RCVR
Tune
Match
Absorption
0%
100%
Frequency
4. Whats the goal? Which type of experiment you need? ()
Different experiments will result in different useful information
44
The FID (free induction decay) is then Fourier transform to frequency domain to
obtain v
pression
( chemical shift) for each different nuclei.
frequency (Hz)
5. NMR Data Processing
Time (sec)
45
1D one pulse 1H
Co CO N
R1
H H
Co CO N
R2
H H
Aliphatic Aromatic & Amide
..
Types of NMR Experiments
Homo Nuclear 1D NMR
46
Homo/Hetero Nuclear 2D NMR
Basic 1D Experiment
Basic 2D Experiment
47
48
49
1
H Chemical Shift
1
3
C
C
h
e
m
i
c
a
l
S
h
i
f
t
You might also like
- Chapter12 WorkedExamplesDocument17 pagesChapter12 WorkedExamplesHalil EmreNo ratings yet
- Cap. 1 FriebolinDocument41 pagesCap. 1 FriebolinRuben SuarezNo ratings yet
- Experiment 34: Excited-State Properties of 2-Naphthol Part II: Deprotonation and Protonation Rate Constants ObjectiveDocument6 pagesExperiment 34: Excited-State Properties of 2-Naphthol Part II: Deprotonation and Protonation Rate Constants Objectivediego prado100% (1)
- Ws10 AnswersDocument4 pagesWs10 AnswersKassimNo ratings yet
- 1H NMR Problem SetDocument5 pages1H NMR Problem Setfishfeeder1No ratings yet
- PMR Spectroscopy: Solved Problems Volume : IIFrom EverandPMR Spectroscopy: Solved Problems Volume : IIRating: 5 out of 5 stars5/5 (3)
- Chapter 19 NMRDocument126 pagesChapter 19 NMRMuchammad RofiiNo ratings yet
- NMR Principles and ApplicationsDocument2 pagesNMR Principles and Applicationsahmad054No ratings yet
- NMR-1 BasicDocument52 pagesNMR-1 Basicamit086No ratings yet
- Theoryofirspectroscopy 160622080111Document50 pagesTheoryofirspectroscopy 160622080111ArshNo ratings yet
- 1H NMR SpectrosDocument84 pages1H NMR Spectrosapi-3723327100% (5)
- Molecular Spectroscopy: BackgroundDocument45 pagesMolecular Spectroscopy: Backgroundsavvy_as_98100% (1)
- Spec Prob Set 315 CurrentDocument20 pagesSpec Prob Set 315 CurrentUmang Agarwal57% (7)
- Spin-Spin Coupling in NMRDocument17 pagesSpin-Spin Coupling in NMRBenjamín Marc Ridgway de SassouNo ratings yet
- NMR SpectrosDocument185 pagesNMR SpectrosBathir JafarNo ratings yet
- Photo ChemistryDocument16 pagesPhoto ChemistryArnab BanerjeeNo ratings yet
- Tutorial 3 (Mass Spectrometry & Infra-Red Spectroscopy)Document6 pagesTutorial 3 (Mass Spectrometry & Infra-Red Spectroscopy)Ahmed ZakyNo ratings yet
- Semi-Empirical MethodsDocument3 pagesSemi-Empirical MethodsludihemicarNo ratings yet
- Various Tools and Techniques Used For Structural ElucidationDocument28 pagesVarious Tools and Techniques Used For Structural ElucidationAvinashNo ratings yet
- 1HNMR Lecture NotesDocument53 pages1HNMR Lecture NotesJian Hong Tee100% (1)
- Challenge Problems in David Klein Chap 7-17Document32 pagesChallenge Problems in David Klein Chap 7-17Ling LingNo ratings yet
- Silverstein Chapter 1 Mass SpectrometryDocument71 pagesSilverstein Chapter 1 Mass SpectrometryNikita GroverNo ratings yet
- FTIR Lecture SlidesDocument25 pagesFTIR Lecture Slidesabdul rehman khanNo ratings yet
- NMR CouplingDocument72 pagesNMR CouplingShrutiNo ratings yet
- 13C NMR SpectrosDocument16 pages13C NMR Spectrosapi-3723327100% (4)
- Identification of Organic and Inorganic Compounds by SpectrosDocument79 pagesIdentification of Organic and Inorganic Compounds by SpectrosAin SkNo ratings yet
- LCAO MO Theory Illustrated by Its Application To H2Document8 pagesLCAO MO Theory Illustrated by Its Application To H2maugonzalezsuarezNo ratings yet
- PhotochemistryDocument29 pagesPhotochemistryArangaNo ratings yet
- Isolobal AnalogyDocument4 pagesIsolobal Analogyindu priyaNo ratings yet
- 303 - 11 Final Exam KEY-1 PDFDocument22 pages303 - 11 Final Exam KEY-1 PDFaegaisNo ratings yet
- Infrared Spectrometry: Cua-Narciso-Pilar, Expt 7Document47 pagesInfrared Spectrometry: Cua-Narciso-Pilar, Expt 7Karina NarcisoNo ratings yet
- AromaticityDocument12 pagesAromaticityV G Viju KumarNo ratings yet
- 14Document110 pages14Meyy SarrahNo ratings yet
- Molarity, Molality and NormalityDocument6 pagesMolarity, Molality and NormalitySami FlimbanNo ratings yet
- Vibrational Spectra and Structure Organophosphorous CompoundsDocument8 pagesVibrational Spectra and Structure Organophosphorous CompoundsJack WuNo ratings yet
- CSIR Chemical Sciences Solved December 2012Document57 pagesCSIR Chemical Sciences Solved December 2012Srinidhi A BelieverNo ratings yet
- Cluster Chemistry Chapter 4 PDFDocument48 pagesCluster Chemistry Chapter 4 PDFGax AllebNo ratings yet
- Outline:: Homogenous Catalysis General and Acid/base Catalysis Acidity Function Bronsted Relation and Enzyme ReactionDocument36 pagesOutline:: Homogenous Catalysis General and Acid/base Catalysis Acidity Function Bronsted Relation and Enzyme Reactionuma villashini100% (2)
- GOC1 HybridizationDocument10 pagesGOC1 Hybridizationthe chemistry guru100% (1)
- Unit Photochemistry: StructureDocument22 pagesUnit Photochemistry: StructureSanjay KumarNo ratings yet
- L2 Che101Document16 pagesL2 Che101Musa Ahammed MahinNo ratings yet
- Boger CourseDocument477 pagesBoger CourseharrypoutreurNo ratings yet
- 200 and More NMR Experiments A PracticalDocument8 pages200 and More NMR Experiments A Practicaldelfin000100% (1)
- Molecular RearrangementsDocument9 pagesMolecular RearrangementsDhanaswamy Ilangeswaran67% (3)
- NMR AssignmentsDocument87 pagesNMR AssignmentsahmedkhidryagoubNo ratings yet
- Modern Organic Synthesis An IntroductionDocument37 pagesModern Organic Synthesis An IntroductionHanh DuongNo ratings yet
- NMR HandoutDocument23 pagesNMR HandoutVirendra Singh RajputNo ratings yet
- Atomic Mass SpectrosDocument40 pagesAtomic Mass Spectrosjohnpaul varonaNo ratings yet
- Difficult Questions On Organic ChemistryDocument5 pagesDifficult Questions On Organic Chemistrytarunbirbanga100% (1)
- Buffer SolutionsDocument21 pagesBuffer SolutionsRizka Nur FaridaNo ratings yet
- Thermochemistry: - Petrucci, Herring Madura and BissonnetteDocument49 pagesThermochemistry: - Petrucci, Herring Madura and BissonnetteYousif Khalid100% (1)
- Atkins Exercises and EquationsDocument3 pagesAtkins Exercises and EquationsmaueemalicdemNo ratings yet
- PolymerDocument32 pagesPolymerNo Bi TaNo ratings yet
- Metal Complexes or Coordination Compounds: Kfecn 4K Fe CNDocument90 pagesMetal Complexes or Coordination Compounds: Kfecn 4K Fe CNPavan Boro100% (1)
- Functional GroupsDocument1 pageFunctional GroupsStan SmithNo ratings yet
- CHAPTER 3 Phase Diagram TTT HT - 1stDocument25 pagesCHAPTER 3 Phase Diagram TTT HT - 1stAriff AziziNo ratings yet
- Electrochemical Processes in Biological SystemsFrom EverandElectrochemical Processes in Biological SystemsAndrzej LewenstamNo ratings yet
- Absorption in SemiconductorsDocument12 pagesAbsorption in SemiconductorsKARUTURI AKASH 17BEC0396No ratings yet
- Griffiths QMCH 4 P 19Document6 pagesGriffiths QMCH 4 P 19Anushka Roy100% (1)
- Advanced Practice Test 01 Paper 01 Test Papers PCM Prayas JEE 2Document9 pagesAdvanced Practice Test 01 Paper 01 Test Papers PCM Prayas JEE 2PRITAM SINGHNo ratings yet
- CHEMISTRYDocument70 pagesCHEMISTRYF E R N A NNo ratings yet
- Quantum Number CompleteDocument37 pagesQuantum Number Completeexponential spiralNo ratings yet
- JirakDanielVite 2018 8RADIOACTIVITYANDIONI BasicsOfMedicalPhysicDocument1 pageJirakDanielVite 2018 8RADIOACTIVITYANDIONI BasicsOfMedicalPhysicGeetNo ratings yet
- Frank Herbert Attix (Auth.) Assorbimento Fotoni - Introduction To Radiological Physics and Radiation Dosimetry (1986) - 141-176-12Document1 pageFrank Herbert Attix (Auth.) Assorbimento Fotoni - Introduction To Radiological Physics and Radiation Dosimetry (1986) - 141-176-12EnricoBogoniNo ratings yet
- Chem Quiz PTRDocument3 pagesChem Quiz PTRniloNo ratings yet
- Determination of Atenolol in Pharmaceutical Dosages by Fourier Transform Infrared Spectrometry (FTIR)Document11 pagesDetermination of Atenolol in Pharmaceutical Dosages by Fourier Transform Infrared Spectrometry (FTIR)Geovas GomezNo ratings yet
- QuinineDocument9 pagesQuinineAhmad AlbabNo ratings yet
- For Teachers Only P .S.-CH: Physical Setting/ChemistryDocument10 pagesFor Teachers Only P .S.-CH: Physical Setting/ChemistryAAVANINo ratings yet
- PC Chapter 44Document85 pagesPC Chapter 44ultimuNo ratings yet
- Science8 Q3 Week5Document20 pagesScience8 Q3 Week5Kathrina De SenaNo ratings yet
- Demers 2008 ADocument278 pagesDemers 2008 ADomingo FernándezNo ratings yet
- D and F Block ElementsDocument8 pagesD and F Block ElementsAbhishek SharmaNo ratings yet
- Electron ArrangementDocument31 pagesElectron ArrangementKenix ChanNo ratings yet
- 7.2 Nuclear Reaction MCQsDocument6 pages7.2 Nuclear Reaction MCQsabdullahalmadaniNo ratings yet
- Jablonski DiagramDocument6 pagesJablonski DiagramLipsa PradhanNo ratings yet
- Chemistry 0.1Document3 pagesChemistry 0.1JeremiahNo ratings yet
- APSACS-SECTT Document - No Salma Mubashir Army Public School & College (Hamza Camp) Double Road, RawalpindiDocument14 pagesAPSACS-SECTT Document - No Salma Mubashir Army Public School & College (Hamza Camp) Double Road, RawalpindiWaseem AkramNo ratings yet
- Bonding Ionic CovalentDocument30 pagesBonding Ionic CovalentRaul MejiaNo ratings yet
- Course Code 6457: Assignment No. 1Document10 pagesCourse Code 6457: Assignment No. 1Tahirullah KhanNo ratings yet
- SP01 - Atomic Theory To Bohr - PresentationDocument29 pagesSP01 - Atomic Theory To Bohr - PresentationMarianNo ratings yet
- Electron Spin Resonance SpectrometerDocument8 pagesElectron Spin Resonance SpectrometerSANDEEP TNo ratings yet
- CBSE Class 9 Science Worksheet - Atoms and MoleculesDocument15 pagesCBSE Class 9 Science Worksheet - Atoms and Moleculesaaliya raiyaniNo ratings yet
- Mo TheoryDocument80 pagesMo TheoryMridul Bhaskar0% (1)
- As 3641.1-1999 Recommended Practice For Atomic Emission Spectrometric Analysis Principles and TechniquesDocument7 pagesAs 3641.1-1999 Recommended Practice For Atomic Emission Spectrometric Analysis Principles and TechniquesSAI Global - APACNo ratings yet
- Points To Remember Class: XI Chapter Name: Chemical Bonding and Molecular StructureDocument15 pagesPoints To Remember Class: XI Chapter Name: Chemical Bonding and Molecular Structureaustinfru7No ratings yet
- Chap 3chemistryDocument34 pagesChap 3chemistryLina AnberNo ratings yet
- Atomic Structure - Flash Cards in This CollectionDocument4 pagesAtomic Structure - Flash Cards in This CollectionEman EssaNo ratings yet