Regression Corr
Regression Corr
Uploaded by
Christopher H. ClarkeCopyright:
Available Formats
Regression Corr
Regression Corr
Uploaded by
Christopher H. ClarkeCopyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
Regression Corr
Regression Corr
Uploaded by
Christopher H. ClarkeCopyright:
Available Formats
Simple Linear Regression & Correlation
We now consider bivariate data. Bivariate data is data
connecting two variables:
The independent or explanatory variable, and
The dependent or response variable.
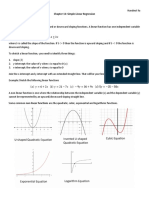
Simple Linear Regression & Correlation
A scatter diagram is used to display bivariate data.
We are interested if there is a linear relationship between
the two sets of data displayed. This can fall in one of 3
classes:
1. POSITIVE LINEAR CORRELATION
2. NEGATIVE LINEAR CORRELATION
3. NO LINEAR CORRELATION
Simple Linear Regression & Correlation
Scatter diagrams show plots of ordered pairs .
Usually, represents the independent variable and
represents the dependent variable. The following
scatter diagrams show different classes of linear
relationships:
x
y
( )
, x y
Simple Linear Regression & Correlation
Graph showing positive correlation
Simple Linear Regression & Correlation
Graph showing negative correlation
Simple Linear Regression & Correlation
Graph showing no linear correlation
Simple Linear Regression & Correlation
A scatter diagram is a good indication as to whether the
linear relationship between the two sets of data can be
represented by a mathematical equation.
This mathematical equation is called a regression function.
We can determine the equation of the regression line
representing the proposed relationship.
Simple Linear Regression & Correlation
The least squares regression line of on :
The general equation of a straight line is
where represents the gradient and represents the
y-intercept. The regression equation is very similar:
The regression equation takes the form
where will represent the gradient and will represent the
y-intercept. These values are called the regression coefficients.
y
y mx c = +
x
c
m
y a bx = +
a
b
Simple Linear Regression & Correlation
These values for and are determined by the following
formulae: and
where and are the mean of and the mean of
respectively. The formula for is based on the fact that
MUST be a point on the regression line.
N.B. and
( )
2
2
n xy x y
b
n x x
a y bx =
a
b
x
y
x
y
( )
, x y
a
n
x
x
=
n
y
y
=
Simple Linear Regression & Correlation
A way to measure the strength of a linear relationship between two
variables is using the product moment correlation coefficient.
This is a number which lies between -1 and +1. Consider 3 cases:
If then there is positive linear correlation.
If then there is negative linear correlation.
If then there is no linear correlation present
r
0 r >
0 r =
0 r <
Simple Linear Regression & Correlation
The correlation coefficient is calculated using the formula:
From this formula we can also determine the coefficient of determination:
This measure represents the ratio of explained variation to total variation.
( ) ( )
2 2
2 2
nxy xy
r
nx xny y
=
( (
( (
( ) ( )
2
2
2 2
2 2
nxy xy
cdr
nx x ny y
(
(
==
(
( ( (
( (
(
*A Practical Example
Tabulated is the maintenance cost/00s$ to the age/mths of 10 X-ray machines.
i. Find the least squares regression line of maintenance cost on age and use it to
predict the maintenance cost for a similar machine 40 mths old.
ii. Calculate the product moment correlation coefficient between the age of the
machine and the cost for maintenance.
iii. Determine the percentage variation in the total maintenance cost that is explained
by the variation in the machine age.
Question adapted from Business Mathematics & Statistics
395 350 300 300 335 310 300 250 240 190 Cost ( y )
60 50 50 30 30 30 20 15 10 5 Age ( x )
10 9 8 7 6 5 4 3 2 1 Machine
156025 3600 23700 395 60
122500 2500 17500 350 50
90000 2500 15000 300 50
90000 900 9000 300 30
112225 900 10050 335 30
96100 900 9300 310 30
90000 400 6000 300 20
62500 225 3750 250 15
57600 100 2400 240 10
36100 25 950 190 5
x
y
xy
2
x
2
y
300 x=
2970 y=
97650 xy=
2
12050 x =
2
913050 y=
From the table on the previous slide we know that:
i. Using the formulae from slide 9
The regression equation is
Estimated cost =
2 2
10;300;2970; 97650;12050; 913050 nxyxy x y ======
( )
2 2
2
10976503002970
2.8
1012050300
2970 300
2.8 212.9
10 10
nxy xy
b
nx x
y x
aybx b
n n
= = =
| |
== = =
|
\ .
212.9 2.8 y x = + y a bx = +
( )
212.92.840$324.90 y= +=
Recall
Using the formulae from slide 11
i. The correlation coefficient =
i. The coefficient of determination =
Thus 77% of the variation in maintenance costs is explained by the variation in
machine ages.
2 2
10;300;2970; 97650;12050; 913050 nxyxy x y ======
( ) ( )
2 2
2 2
2 2
10976503002970
1012050300 109130502970
0.88
n xy x y
r
n x x n y y
r
r
=
( (
( (
=
=
( )
2
2
0.88 0.77 cdr == =
You might also like
- Complete Business Statistics: by Amir D. Aczel & Jayavel Sounderpandian 6 EditionDocument54 pagesComplete Business Statistics: by Amir D. Aczel & Jayavel Sounderpandian 6 EditionMohit VermaNo ratings yet
- Sas Chapter 10 Asda Analysis Examples Replication Winter 2010 SasDocument7 pagesSas Chapter 10 Asda Analysis Examples Replication Winter 2010 SasSarbarup BanerjeeNo ratings yet
- Regression and CorrelationDocument15 pagesRegression and CorrelationsdffsjdfhgNo ratings yet
- Linear CorrelationDocument5 pagesLinear CorrelationTrilochan PanigrahiNo ratings yet
- Regression NotesDocument12 pagesRegression NotesAleciafyNo ratings yet
- 06 Simple Linear Regression Part1Document8 pages06 Simple Linear Regression Part1Rama DulceNo ratings yet
- Forecasting Techniques: Quantitative Techniques in ManagementDocument25 pagesForecasting Techniques: Quantitative Techniques in ManagementvishalNo ratings yet
- BIVARIATE DATA NOTESDocument14 pagesBIVARIATE DATA NOTESchiyanjapeter7No ratings yet
- Unit 6Document8 pagesUnit 6Mahesh GandikotaNo ratings yet
- A A RegressionDocument28 pagesA A RegressionRobin GuptaNo ratings yet
- Stats and MathsDocument29 pagesStats and MathsMystiquemashalNo ratings yet
- RegressionDocument43 pagesRegressionNikhil Aggarwal100% (1)
- X X B X B X B y X X B X B N B Y: QMDS 202 Data Analysis and ModelingDocument6 pagesX X B X B X B y X X B X B N B Y: QMDS 202 Data Analysis and ModelingFaithNo ratings yet
- Chapter 9Document23 pagesChapter 9Ayush ChopraNo ratings yet
- Regression (Autosaved) (Autosaved)Document80 pagesRegression (Autosaved) (Autosaved)DishaNo ratings yet
- Simple Linear RegressionDocument95 pagesSimple Linear RegressionPooja GargNo ratings yet
- A Ybx: Scatter Diagram Correlation CoefficientDocument7 pagesA Ybx: Scatter Diagram Correlation CoefficientNaheed Nazneen TuLiNo ratings yet
- Correlation and Regression - October 25 - 2022Document53 pagesCorrelation and Regression - October 25 - 2022Rezwana SultanaNo ratings yet
- ml-unit-3-notes-1Document58 pagesml-unit-3-notes-1Thil PaNo ratings yet
- Topic 6 Mte3105Document9 pagesTopic 6 Mte3105Siva Piragash Mira BhaiNo ratings yet
- Linear RegressionDocument8 pagesLinear RegressionSailla Raghu rajNo ratings yet
- Simple RegressionDocument50 pagesSimple Regressioniga gemelia100% (1)
- Chapter 15 - Q&A ExtraDocument3 pagesChapter 15 - Q&A Extrakhaai3108No ratings yet
- Regression: 9.1.1 DefinitionDocument20 pagesRegression: 9.1.1 DefinitionBhawna JoshiNo ratings yet
- 09 Inference For Regression Part1Document12 pages09 Inference For Regression Part1Rama DulceNo ratings yet
- Chapter 12 NotesDocument60 pagesChapter 12 Notesmeenahershey52No ratings yet
- Correlation and RegressionDocument23 pagesCorrelation and Regressionreshmii_123No ratings yet
- Chapter 1Document41 pagesChapter 1Daksh ParasharNo ratings yet
- Unit 5 (CORRELATION AND REGRESSION)Document23 pagesUnit 5 (CORRELATION AND REGRESSION)Zara NabilahNo ratings yet
- Topic 2 Part III PDFDocument30 pagesTopic 2 Part III PDFChris MastersNo ratings yet
- Regression AnalysisDocument29 pagesRegression AnalysisRegnold MunuoNo ratings yet
- Regression1Document32 pagesRegression1dosavo8504No ratings yet
- Adequacy of Solutions - : After Reading This Chapter, You Will Be Able ToDocument15 pagesAdequacy of Solutions - : After Reading This Chapter, You Will Be Able TokesslerNo ratings yet
- Chapter 5: Regression and Correlation: Bivariate Data) and Relationship Between The Two VariablesDocument5 pagesChapter 5: Regression and Correlation: Bivariate Data) and Relationship Between The Two VariablesSrinyanavel ஸ்ரீஞானவேல்No ratings yet
- Regression EquationsDocument14 pagesRegression EquationsAswinNo ratings yet
- Linear Regression FormulaDocument5 pagesLinear Regression Formulaapi-140032165No ratings yet
- REGRESSION ANALYSIS STA 221Document10 pagesREGRESSION ANALYSIS STA 221dabini6459No ratings yet
- Linear RegressionDocument12 pagesLinear RegressionDEEPA LAKSHMI RAVINo ratings yet
- Unit 2 Regression AnalysisDocument16 pagesUnit 2 Regression AnalysissbsbxbxahsbxxNo ratings yet
- Assignement On Business ForecastingDocument8 pagesAssignement On Business ForecastingGurbachan SinghNo ratings yet
- Eco 173 Regression Part IDocument4 pagesEco 173 Regression Part INadim IslamNo ratings yet
- Ue21cs352a 20230830120810Document30 pagesUe21cs352a 20230830120810Sonupatel SonupatelNo ratings yet
- Regression AnalysisDocument43 pagesRegression AnalysisRàmèsh RôdálbáñdîNo ratings yet
- Regression EquationDocument56 pagesRegression EquationMuhammad TariqNo ratings yet
- Correlation and RegressionDocument11 pagesCorrelation and RegressionSANIUL ISLAMNo ratings yet
- Econometrics Chapter 3Document24 pagesEconometrics Chapter 3Ibrahin AbdiNo ratings yet
- Linear Regression and Correlation A Level Notes (Precision Academy)Document17 pagesLinear Regression and Correlation A Level Notes (Precision Academy)rudomposiNo ratings yet
- Maths Chapter 1Document18 pagesMaths Chapter 1Abrha636100% (4)
- Chapter OneDocument27 pagesChapter Onetadenardi4No ratings yet
- Stats ch12 PDFDocument28 pagesStats ch12 PDFVivek AnandhanNo ratings yet
- Inferential Sta-WPS OfficeDocument21 pagesInferential Sta-WPS OfficeXtremo ManiacNo ratings yet
- 1 Chapter I Linear EquationsDocument98 pages1 Chapter I Linear Equationsvalenciawinno5No ratings yet
- Statistics-Linear Regression and Correlation AnalysisDocument50 pagesStatistics-Linear Regression and Correlation AnalysisDr Rushen SinghNo ratings yet
- Supplement 5 - Multiple RegressionDocument19 pagesSupplement 5 - Multiple Regressionnm2007kNo ratings yet
- Numerical Methods Chapter Three Curve Fitting: Woldia University Institute of TechnologyDocument22 pagesNumerical Methods Chapter Three Curve Fitting: Woldia University Institute of TechnologyGetachew FentawNo ratings yet
- Sec D CH 12 Regression Part 2Document66 pagesSec D CH 12 Regression Part 2Ranga Sri100% (1)
- MathDocument36 pagesMathRobert EspiñaNo ratings yet
- Statistical Methods for Computer Science IIDocument14 pagesStatistical Methods for Computer Science IIRubeena PathanNo ratings yet
- A-level Maths Revision: Cheeky Revision ShortcutsFrom EverandA-level Maths Revision: Cheeky Revision ShortcutsRating: 3.5 out of 5 stars3.5/5 (8)
- Haramaya University College of Computing and Informatics Department of StatisticsDocument2 pagesHaramaya University College of Computing and Informatics Department of StatisticsSeenaa N DachaasaaNo ratings yet
- Time Series AnalysisDocument3 pagesTime Series AnalysisSamarth VäìşhNo ratings yet
- Mike Zero - Mike EVA Technical DocumentationDocument90 pagesMike Zero - Mike EVA Technical DocumentationAnonymous BZ0l8QylpNo ratings yet
- Structural Equation ModelingDocument23 pagesStructural Equation ModelingYuliana YulianaNo ratings yet
- Information Entropy FundamentalsDocument19 pagesInformation Entropy Fundamentalsmaskon.alienNo ratings yet
- Machine LearningDocument24 pagesMachine LearningHari KrishnanNo ratings yet
- Statistics - G8Document16 pagesStatistics - G8Calm ValleyNo ratings yet
- Math288 Week10Document19 pagesMath288 Week10Nina IsMeNo ratings yet
- Stat. & Prob-Unit-IDocument233 pagesStat. & Prob-Unit-IDivyanshi BajpaiNo ratings yet
- Bloomberg - Term Structure of RiskDocument42 pagesBloomberg - Term Structure of RiskMarkus SchantaNo ratings yet
- Quiz 1 AnswersDocument10 pagesQuiz 1 AnswersAbhijeet singhNo ratings yet
- Analysis of Variance AnovaDocument33 pagesAnalysis of Variance AnovaKanishk BojewarNo ratings yet
- Sppu/Bba (Ca) Syllabus Structure Cbcs/2019 Pattern 11Document2 pagesSppu/Bba (Ca) Syllabus Structure Cbcs/2019 Pattern 11Anish DhobleNo ratings yet
- Chap 4 - Discrete ProbabilityDocument63 pagesChap 4 - Discrete ProbabilityQuizziesZA100% (1)
- tmp4180 TMPDocument14 pagestmp4180 TMPFrontiersNo ratings yet
- Information Theory & Coding: AssignmentDocument6 pagesInformation Theory & Coding: Assignmentsana htpNo ratings yet
- 1-Handout One-An Overview of Descriptive Statistics-Chapter 1&2Document22 pages1-Handout One-An Overview of Descriptive Statistics-Chapter 1&2Ahmed rafiqNo ratings yet
- Bayes TheoremDocument4 pagesBayes TheoremCaptain rebornNo ratings yet
- Introduction To Biostatistics SyllabusDocument8 pagesIntroduction To Biostatistics SyllabusKasparov RepedroNo ratings yet
- Mathematical StatisticsDocument1 pageMathematical StatisticsRaphael SisonNo ratings yet
- Pearson and Spearman CorrelationDocument50 pagesPearson and Spearman CorrelationPartosa Ace Pencer M.No ratings yet
- Mathematical Methods in Risk TheoryDocument217 pagesMathematical Methods in Risk TheoryAnonymous Tph9x741100% (1)
- Statistics For Business and Economics (13e) : John LoucksDocument48 pagesStatistics For Business and Economics (13e) : John LoucksMary Mae Arnado100% (1)
- Arima Modeling With R ListendataDocument12 pagesArima Modeling With R ListendataHarshalKolhatkarNo ratings yet
- Comando Svy StataDocument3 pagesComando Svy StataLays RosalNo ratings yet
- Gerlach Et Al-2018-Nature Human Behaviour - Sup-1Document23 pagesGerlach Et Al-2018-Nature Human Behaviour - Sup-1Yell OwNo ratings yet
- Do UN Interventions Cause Peace Using Matching ToDocument43 pagesDo UN Interventions Cause Peace Using Matching ToYohanes Eki ApriliawanNo ratings yet
- MATH381 Probability and Statistics Summer 2020 Syllabus PDFDocument2 pagesMATH381 Probability and Statistics Summer 2020 Syllabus PDFDima Al KibbiNo ratings yet
- Full Download Principles of Econometrics 4th Edition Hill Test BankDocument35 pagesFull Download Principles of Econometrics 4th Edition Hill Test Bankterleckisunday1514fx100% (54)