- Normal Style
- MLA Style
- APA Style
- Chicago Style
Abstract
In this paper, we further study the quasi-p-convex function. The concepts of strictly quasi-p-convex function and quasi-p-convex cone are given and some new fundamental characterizations and operational properties of quasi-p-convex function are obtained.
1. Introduction
Convex function is an important kind of function in mathematics, which is widely used in mathematical programming, approximation theory, control theory and other fields. But it is found that the mathematical models of many optimization problems in practical application are non-convex, which prompts us to consider the generalized convex function with weak convexity. Generalized convex function can not only retain some good characteristics of convex function but also relax the requirement for convexity appropriately. So its application scope is wider than convex function. In 1949, De Finetti 1 proposed the first generalized convex function, and in 1953, Fenchel 2 named it quasi-convex function. Yang 3, 4, 5 further studied the properties of quasi-convex functions. In 2003, Wang et al. 6 proposed the concept of  -quasi-convex function and studied its related properties. In 2011, Lin et al. 7 established several new Hadamard type inequalities for quasi-convex function. In 2013, Zhang 8 defined harmonic quasi-convex function. In 2021, Bai 9 established Simpson type fractional integral inequality for quasi-convex function. In the same year, Sevda et al. 10 defined p-convex functions by using the concept of epigraph on the basis of p-convex sets. In 2022, Gültekin et al. 11 defined quasi-p-convex functions.
-quasi-convex function and studied its related properties. In 2011, Lin et al. 7 established several new Hadamard type inequalities for quasi-convex function. In 2013, Zhang 8 defined harmonic quasi-convex function. In 2021, Bai 9 established Simpson type fractional integral inequality for quasi-convex function. In the same year, Sevda et al. 10 defined p-convex functions by using the concept of epigraph on the basis of p-convex sets. In 2022, Gültekin et al. 11 defined quasi-p-convex functions.
In this paper, we further study the quasi-p-convex function. The concepts of strictly quasi-p-convex function and quasi-p-convex cone are given and some new fundamental characterizations and operational properties of quasi-p-convex function are obtained.
2. Preliminaries
Definition 2.1 13 Let  and
and  . If for each
. If for each  ,
,  ,
,
where  ,
,  , then
, then  is called a p-convex set in
is called a p-convex set in  .
.
The definition of p-convexity of  can also be given as
can also be given as

for all  and
and 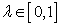 .
.
Definition 2.2 14 Given  , the set
, the set
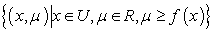
is called epigraph of  , it is denoted by
, it is denoted by  .
.
Definition 2.3 10 Let  and
and  be a function. If the set
be a function. If the set

is p-convex set, then  is called a p-convex function.
is called a p-convex function.
Theorem 2.1 10 Let  ,
,  be a function and
be a function and 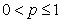 , then
, then  is a p-convex function if and only if
is a p-convex function if and only if  is a p-convex set and for any
is a p-convex set and for any  ,
,  holds, where
holds, where  ,
,  .
.
Definition 2.4 11 Let  .
.
(i) A function  is called quasi-p-convex function if
is called quasi-p-convex function if
 |
for each  ;
; such that
such that  or, equivalently
or, equivalently
 |
for every  and for every
and for every  .
.
(ii) A function  is called quasi-p-concave function if
is called quasi-p-concave function if  is quasi-p-convex, i.e., for each
is quasi-p-convex, i.e., for each 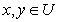 ;
; such that
such that  ,
, or, equivalently
or, equivalently
 |
for every  and for every
and for every 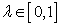 .
.
Example 2.1 Let  be a p-convex set,
be a p-convex set, 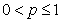 . Define the function
. Define the function  ,
,
 |
where  ,
,  , then
, then  is a quasi-p-convex function.
is a quasi-p-convex function.
Proof. Suppose that  such that
such that  ,
,  , then for each
, then for each  ,
,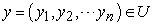 ,
,
 that is,
that is, 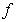 is a quasi-p-convex function.
is a quasi-p-convex function.
Theorem 2.2 11 Let  be a p-convex set,
be a p-convex set,  . If
. If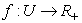 is a p-convex function, then
is a p-convex function, then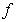 is a quasi-p-convex function.
is a quasi-p-convex function.
Definition 2.5 14 Suppose that  is a subset of
is a subset of  , if for each
, if for each  ,
, such that
such that  , then
, then 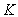 is called a cone.
is called a cone.
Definition 2.6 14 A cone is said to be convex cone if it is also a convex set.
Definition 2.7 12 Let  be a convex cone and
be a convex cone and  be a function. If for each
be a function. If for each  ,
,  ,
,  such that
such that  , then the function
, then the function  is called a positive homogeneous function with respect to degree
is called a positive homogeneous function with respect to degree  .
.
Remark 2.1 12 (1) Let  ,
, 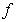 be a positive homogeneous function with respect to degree
be a positive homogeneous function with respect to degree  . If
. If  exists, then
exists, then  .
.
If  ,
,  is called a linear positive homogeneous function.
is called a linear positive homogeneous function.
Theorem 2.3 14 The function is a linear positive homogeneous function if and only if its epigraph
is a linear positive homogeneous function if and only if its epigraph  is a cone in
is a cone in  .
.
Definition 2.8 12 Let  , then
, then  is called a strict local minimum (maximum) of
is called a strict local minimum (maximum) of  , if it exists
, if it exists  such that
such that
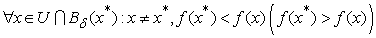 .
.
3. Main Results
Definition 3.1 Let 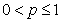 ,
,  be a p-convex set and
be a p-convex set and 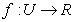 be a function. For each
be a function. For each  , if the inequality
, if the inequality
holds for all  such that
such that  , then
, then  is said to be a strictly quasi-p-convex function.
is said to be a strictly quasi-p-convex function.
Definition 3.1 can also be expressed as follows:
Let  ,
,  be a p-convex set and
be a p-convex set and  be a function. If for any
be a function. If for any  ,
,
where  , then
, then  is said to be a strictly quasi-p-convex function.
is said to be a strictly quasi-p-convex function.
Definition 3.2 Let  ,
,  be a p-concave set and
be a p-concave set and 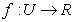 be a function. For each
be a function. For each  , if the inequality
, if the inequality
 (3.3)
(3.3)
holds for all  such that
such that  , then
, then  is said to be a strictly quasi-p-concave function.
is said to be a strictly quasi-p-concave function.
Definition 3.2 can also be expressed as follows:
Let  ,
, 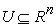 be a p-convex set and
be a p-convex set and  be a function. If for any
be a function. If for any  ,
,
 | (3.4) |
where  , then
, then  is said to be a strictly quasi-p-concave function.
is said to be a strictly quasi-p-concave function.
Theorem 3.1 Let  be a p-convex set,
be a p-convex set,  .
.
(1) If  is a strictly p-convex function, the
is a strictly p-convex function, the is a strictly quasi-p-convex function;
is a strictly quasi-p-convex function;
(2) If  is a strictly quasi-p-convex function, then
is a strictly quasi-p-convex function, then is a quasi-p-convex function.
is a quasi-p-convex function.
Proof. (1) From the strictly p-convexity of  , for each
, for each  ,
,  such that such that
such that such that  ,
,
 |
 |
 |
 ,
,
that is,  is a strictly quasi-p-convex function.
is a strictly quasi-p-convex function.
(2) It comes straight from the definition.
Definition 3.3 A cone is said to be p-convex cone if it is also a p-convex set.
Remark 3.1 If  , p-convex cone is a convex cone.
, p-convex cone is a convex cone.
Lemma 3.1 The set 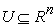 is a p-convex cone if and only if
is a p-convex cone if and only if 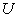 is closed for operations of addition and positive multiplication.
is closed for operations of addition and positive multiplication.
Proof. Suppose that is a p-convex cone,
is a p-convex cone,  , then for each
, then for each ,
,  such that
such that  ,
,
Take  , then
, then
 |
Also  is a cone, so
is a cone, so  is obviously closed for operation of positive multiplication. Therefor
is obviously closed for operation of positive multiplication. Therefor
 |
That is,  is closed for operation of addition.
is closed for operation of addition.
, if  is closed for operations of positive multiplication, then
is closed for operations of positive multiplication, then  is obviously a cone. For each
is obviously a cone. For each 
 such that
such that  , then
, then  . Also
. Also  is closed for operations of addition, so
is closed for operations of addition, so
 ,
,
that is,  is a p-convex set. Thus
is a p-convex set. Thus  is a p-convex cone.
is a p-convex cone.
Lemma 3.2 For  , let
, let  be a p-convex cone,
be a p-convex cone, 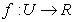 be a positive homogeneous function of degree p. Then
be a positive homogeneous function of degree p. Then is a p-convex function if and only if for any
is a p-convex function if and only if for any  ,
,
 |
Proof. Suppose is a p-convex function, then
is a p-convex function, then  is a p-convex set. Also
is a p-convex set. Also  is a positive homogeneous function of degree p, for each
is a positive homogeneous function of degree p, for each  ,
,  , then
, then
 .
.
Specially, take  , then
, then  , namely
, namely  , so the
, so the  is a cone.
is a cone.
From lemma 3.1, for each  ,
,
 ,
,
namely
 .
.
, for each  , then
, then
 ,
,
namely
 ,
,
so is closed for operation of addition. Also because
is closed for operation of addition. Also because is a positive homogeneous function of degree p, taking
is a positive homogeneous function of degree p, taking , by Theorem 2.3, we known that
, by Theorem 2.3, we known that  is closed for operation of positive multiplication. From Lemma 3.1,
is closed for operation of positive multiplication. From Lemma 3.1,  is a p-convex set, so
is a p-convex set, so  is a p-convex function.
is a p-convex function.
Theorem 3.2 For  , let
, let  be a p-convex cone and
be a p-convex cone and be a positive homogeneous function of degree p. If for all
be a positive homogeneous function of degree p. If for all  ,
,  , then
, then 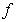 is a quasi-p-convex function if and only if
is a quasi-p-convex function if and only if is a p-convex function.
is a p-convex function.
Proof. For all  , by Theorem 2.2, a p-convex function is obviously is a quasi-p-convex function.
, by Theorem 2.2, a p-convex function is obviously is a quasi-p-convex function.
, if is a quasi-p-convex function, by the positive homogeneity of degree p of
is a quasi-p-convex function, by the positive homogeneity of degree p of  and Lemma 2, it just need to prove that
and Lemma 2, it just need to prove that satisfies the inequality in Lemma 2.
satisfies the inequality in Lemma 2.
Let any  ,
,  . Since
. Since  is a positive homogeneous function of degree p, namely
is a positive homogeneous function of degree p, namely
 |
 | , |
it follows that
 |
Given the quasi-p-convexity of  , we obtain
, we obtain
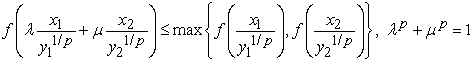 |
 .
.
Let  , then
, then
 |
 |
 |
 .
.
If either or
or is zero, for example
is zero, for example  , remark 1 show that
, remark 1 show that  , then
, then
 .
.
This completes the proof.
Theorem 3.3 Let  be a p-convex set,
be a p-convex set,  and
and 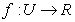 be a quasi-p-convex function. If
be a quasi-p-convex function. If 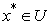 is a strict local minimum of
is a strict local minimum of 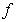 , it is also a strict global minimum of
, it is also a strict global minimum of  , and the set
, and the set  of all minimal points of
of all minimal points of  is p-convex set.
is p-convex set.
Proof. Let 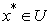 be a strict local minimum of
be a strict local minimum of 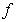 , if
, if  is not a strictly global minimum of
is not a strictly global minimum of 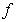 , then it exists
, then it exists  such that
such that  .
.
By using the quasi-p-convexity of  , for
, for  , we have
, we have
 | (3.5) |
It exists small enough  such that
such that
 ,
,
namely 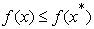 , it contradicts that
, it contradicts that  is a strict local minimum of
is a strict local minimum of  . So
. So  is the strict global minimum of
is the strict global minimum of  .
.
Now assume that 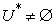 , let
, let  be the minimum value of
be the minimum value of  on
on  , it is noticed that
, it is noticed that
 |
By the quasi-p-convexity of  and Theorem 2.4, the lower level set
and Theorem 2.4, the lower level set  is a p-convex set. Thus
is a p-convex set. Thus  is also a p-convex set.
is also a p-convex set.
Theorem 3.4 Let  be a p-convex set,
be a p-convex set,  , and
, and  be a quasi-p-convex function. Then for each
be a quasi-p-convex function. Then for each  , then
, then  a quasi-p-convex function on
a quasi-p-convex function on  .
.
Proof. For all  such that
such that  , it can be given by conditions
, it can be given by conditions

 .
.
Thus  is a quasi-p-convex function.
is a quasi-p-convex function.
Corollary 3.1 If the function  is a strictly quasi-p-convex function, then
is a strictly quasi-p-convex function, then 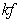 a strictly quasi-p-convex function on
a strictly quasi-p-convex function on  .
.
Proof. It is clear from Theorem 3.4.
Theorem 3.5 Let  be a p-convex set,
be a p-convex set,  . If
. If  is quasi-p-convex functions for
is quasi-p-convex functions for  , then
, then  is a quasi-p-convex function where
is a quasi-p-convex function where  .
.
Proof. Let 
 , so
, so 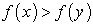 . For
. For 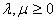 such that
such that  , we have
, we have



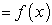 .
.
This is,  is a quasi-p-convex functions.
is a quasi-p-convex functions.
Theorem 3.6 If function  is a quasi-p-concave function. For each
is a quasi-p-concave function. For each 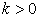 , then
, then  a quasi-p-concave function on
a quasi-p-concave function on  .
.
Proof. For all  such that
such that  , it can be given by conditions
, it can be given by conditions

 .
.
Thus  is a quasi-p-concave function.
is a quasi-p-concave function.
Corollary 3.2 If the function  is a strictly quasi-p-concave function, then
is a strictly quasi-p-concave function, then  a strictly quasi-p-concave function on
a strictly quasi-p-concave function on  .
.
ACKNOWLEDGEMENTS
This work was supported by PhD Research Foundation of Inner Mongolia Minzu University (No. BS402) and Operating expenses for basic scientific research at Universities directly affiliated to Inner Mongolia Autonomous Region (No. GXKY22159).
References
| [1] | Finetti B D. Sulle stratificazioni convesse [J]. Ann Math Pura Appl, 1949, 30(1): 173-183. | ||
| In article | View Article | ||
| [2] | Fenchel W. Convex cones, Sets and Functions [M]. Princeton: Princeton University, 1953. | ||
| In article | |||
| [3] | Yang X M. Quasi-convexity of upper semi-continuous functions [J]. Operations Research Transactions, 1999, 01: 48-51. | ||
| In article | |||
| [4] | Yang X M. Some properties of quasi-convex functions [J]. Chinese Journal of Engineering Mathematics, 1993, 01: 51-56. | ||
| In article | |||
| [5] | Yang X M. A note on criteria of quasi-convex functions [J]. Operations Research Transactions, 2001, 02: 55-56. | ||
| In article | |||
| [6] | Wang J Y, Song Y, Bai X L. E-quasiconvex functions [J]. Journal of Liaocheng University (Natural Science Edition), 2003, 03: 17-19. | ||
| In article | |||
| [7] | Lin Q W, Yu Y H. Quasi-convex functions and Hadamard’s inequality [J]. Mathematics in Practice and Theory, 2011, 41(17): 229-235. | ||
| In article | |||
| [8] | Zhang T Y, Ji A P, Qi F. Integral inequalities of Hermite-Hadamard type for harmonically quasi-convex functions [J]. Proceedings of the Jangjeon Mathematical Society, 2013, 16(3): 399-407. | ||
| In article | |||
| [9] | Bai S P. Simpson type fractional integral inequality for quasi-convex functions [J]. Journal of Inner Mongolia University for Nationalities (Natural Science Edition), 2021, 36(06): 465-470. | ||
| In article | |||
| [10] | Sevda S, Zeynep E, Gültekin T, et al. p-convex functions and some of their properties [J]. Numereical Functional Analysis and Optimization, 2021, 42(4): 443-459. | ||
| In article | View Article | ||
| [11] | Gültekin T, Zeynep E, Sevda S, et al. Quasi p-Convex Functions [J]. Applied Mathematics E-Notes, 2022, 22: 741-750. | ||
| In article | |||
| [12] | Yang X M, Rong W D. Generalized Cconvexity and Its Application [M]. Beijing: Science Press, 2017. | ||
| In article | |||
| [13] | Bayoumi A. Foundations of Complex Analysis in Non Locally Convex Spaces: Function theory Without Convexity Condition [M]. Amsterdam: North-Holland Pub. Co., 2003. | ||
| In article | |||
| [14] | Roekafellar R T. Convex Analysis [M]. Princeton: Princeton University Press, 1970. | ||
| In article | |||
Published with license by Science and Education Publishing, Copyright © 2023 Qian Zheng and Shuhong Wang
![]() This work is licensed under a Creative Commons Attribution 4.0 International License. To view a copy of this license, visit
https://creativecommons.org/licenses/by/4.0/
This work is licensed under a Creative Commons Attribution 4.0 International License. To view a copy of this license, visit
https://creativecommons.org/licenses/by/4.0/
Cite this article:
Normal Style
MLA Style
APA Style
Chicago Style
| [1] | Finetti B D. Sulle stratificazioni convesse [J]. Ann Math Pura Appl, 1949, 30(1): 173-183. | ||
| In article | View Article | ||
| [2] | Fenchel W. Convex cones, Sets and Functions [M]. Princeton: Princeton University, 1953. | ||
| In article | |||
| [3] | Yang X M. Quasi-convexity of upper semi-continuous functions [J]. Operations Research Transactions, 1999, 01: 48-51. | ||
| In article | |||
| [4] | Yang X M. Some properties of quasi-convex functions [J]. Chinese Journal of Engineering Mathematics, 1993, 01: 51-56. | ||
| In article | |||
| [5] | Yang X M. A note on criteria of quasi-convex functions [J]. Operations Research Transactions, 2001, 02: 55-56. | ||
| In article | |||
| [6] | Wang J Y, Song Y, Bai X L. E-quasiconvex functions [J]. Journal of Liaocheng University (Natural Science Edition), 2003, 03: 17-19. | ||
| In article | |||
| [7] | Lin Q W, Yu Y H. Quasi-convex functions and Hadamard’s inequality [J]. Mathematics in Practice and Theory, 2011, 41(17): 229-235. | ||
| In article | |||
| [8] | Zhang T Y, Ji A P, Qi F. Integral inequalities of Hermite-Hadamard type for harmonically quasi-convex functions [J]. Proceedings of the Jangjeon Mathematical Society, 2013, 16(3): 399-407. | ||
| In article | |||
| [9] | Bai S P. Simpson type fractional integral inequality for quasi-convex functions [J]. Journal of Inner Mongolia University for Nationalities (Natural Science Edition), 2021, 36(06): 465-470. | ||
| In article | |||
| [10] | Sevda S, Zeynep E, Gültekin T, et al. p-convex functions and some of their properties [J]. Numereical Functional Analysis and Optimization, 2021, 42(4): 443-459. | ||
| In article | View Article | ||
| [11] | Gültekin T, Zeynep E, Sevda S, et al. Quasi p-Convex Functions [J]. Applied Mathematics E-Notes, 2022, 22: 741-750. | ||
| In article | |||
| [12] | Yang X M, Rong W D. Generalized Cconvexity and Its Application [M]. Beijing: Science Press, 2017. | ||
| In article | |||
| [13] | Bayoumi A. Foundations of Complex Analysis in Non Locally Convex Spaces: Function theory Without Convexity Condition [M]. Amsterdam: North-Holland Pub. Co., 2003. | ||
| In article | |||
| [14] | Roekafellar R T. Convex Analysis [M]. Princeton: Princeton University Press, 1970. | ||
| In article | |||



