Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd Logo
Upload

Welcome to Scribd!

  • 27 views

    D2y dx2 Dy: Mechanics of Materials

    Uploaded by

    vik03223
    This document discusses using the modified Macaulay approach to calculate the deflection of a beam with distributed loads over part of its length. It presents the deflection equation and solves for the constants A and B by setting the deflection to 0 at two points, x=0.6 and x=1.8. It finds A=-3.72 and B=2.952. The deflection equation is then written with these constants substituted in.

    Copyright:

    Attribution Non-Commercial (BY-NC)
    Download as PDF, TXT or read online from Scribd

    D2y dx2 Dy: Mechanics of Materials

    Uploaded by

    vik03223
    27 views1 page
    This document discusses using the modified Macaulay approach to calculate the deflection of a beam with distributed loads over part of its length. It presents the deflection equation and solves for the constants A and B by setting the deflection to 0 at two points, x=0.6 and x=1.8. It finds A=-3.72 and B=2.952. The deflection equation is then written with these constants substituted in.

    Original Title

    12 - Page 146

    Copyright

    © Attribution Non-Commercial (BY-NC)

    Available Formats

    PDF, TXT or read online from Scribd

    Did you find this document useful?

    Is this content inappropriate?

    This document discusses using the modified Macaulay approach to calculate the deflection of a beam with distributed loads over part of its length. It presents the deflection equation and solves for the constants A and B by setting the deflection to 0 at two points, x=0.6 and x=1.8. It finds A=-3.72 and B=2.952. The deflection equation is then written with these constants substituted in.

    Copyright:

    Attribution Non-Commercial (BY-NC)
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    27 views1 page

    D2y dx2 Dy: Mechanics of Materials

    Uploaded by

    vik03223
    This document discusses using the modified Macaulay approach to calculate the deflection of a beam with distributed loads over part of its length. It presents the deflection equation and solves for the constants A and B by setting the deflection to 0 at two points, x=0.6 and x=1.8. It finds A=-3.72 and B=2.952. The deflection equation is then written with these constants substituted in.

    Copyright:

    Attribution Non-Commercial (BY-NC)
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    of 1

    Mechanics of Materials

    128

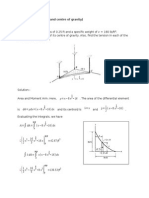
    Using the modified Macaulay approach for distributed loads over part of a beam
    introduced in (j 5.5 (Fig. 5.39b),

    M,,

    E l d2y
    = - 2 0 ~ 62[ (X -0.6)] - 30
    lo3 dx2

    =
    I

    _
    El_
    dy ---20x2 +62[
    103 dx

    (X

    -yl2+ 30[

    - 0.6)2 ]-30[(

    -;.8'

    -2O[(X - 1.8),

    x - 0.6)3]+30[( x - 1.8)3

    ]
    +A

    EI

    - 2oX3 +62[
    = 6

    (X

    - 0.6)3] - ~ O [ ( ~ - O . ~ ) '

    24

    + 30[

    (X

    - 1.8)'

    24
    (X

    - 1.8)3

    -20[
    Now when x = 0.6, y

    o=

    ..

    +AX+B

    = 0,

    20 x 0.63
    + 0.6A + B
    6

    0.72 = 0.6A + B
    and when x = 3, y

    ..

    = 0,

    + 62 x62.43 - 30 x242.4' + 30 x241.2' - 20 x6l.z3 + 3 A + B


    = - 90 + 142.848- 41.472 + 2.592 - 5.76 + 3A + B
    - 8.208 = 3A + B
    o=

    20 x 33
    6

    -___

    (2) - (1)

    .'. A

    - 8.928 = 2.4A

    = -3.72

    Substituting in (l),
    B

    = 0.72 -0.6(

    - 3.72)

    B = 2.952

    Substituting into the Macaulay deflection equation,

    SE l Y = -~
    20x3
    6

    62[ (' 63:)-

    ]-

    30[ (x

    --t6)"] ;-')'I
    +

    30[ (x

    - 3 . 7 2 ~+ 2.952

    - 20 (x -:8' 9'
    At

    x=l
    30 x 0.4'
    20 62
    - 3.72 x 1 + 2.952
    +x 0.43 24
    6
    6

    You might also like