CH18

18-1
CHAPTER 18
ELECTRICAL PROPERTIES
PROBLEM SOLUTIONS
Ohms Law
Electrical Conductivity
18.1 This problem calls for us to compute the electrical conductivity and resistance of a silicon specimen.
(a) We use Equations 18.3 and 18.4 for the conductivity, as
=
Il
1
=
=
VA
Il
d 2
V
2
And, incorporating values for the several parameters provided in the problem statement, leads to
(0.25 A)(45 x 103 m)

7.0 x 103 m 2
(24 V)()
2
= 12.2 ( - m)-1
(b) The resistance, R, may be computed using Equations 18.2 and 18.4, as
R=
l
=
A
l
d 2

2
57 x 103 m
7.0 x 103 m 2
1
12.2 ( m)
()
2
= 121.4
Excerpts from this work may be reproduced by instructors for distribution on a not-for-profit basis for testing or instructional purposes only to
students enrolled in courses for which the textbook has been adopted. Any other reproduction or translation of this work beyond that permitted
by Sections 107 or 108 of the 1976 United States Copyright Act without the permission of the copyright owner is unlawful.
18-2
18.2 For this problem, given that an aluminum wire 10 m long must experience a voltage drop of less than
1.0 V when a current of 5 A passes through it, we are to compute the minimum diameter of the wire. Combining
Equations 18.3 and 18.4 and solving for the cross-sectional area A leads to
A=
Il
V
d 2
From Table 18.1, for aluminum = 3.8 x 107 (-m)-1. Furthermore, inasmuch as A = for a cylindrical
2
wire, then
d 2
Il
=
2
V
or
d=
4 Il
V
When values for the several parameters given in the problem statement are incorporated into this expression, we get
d =
(4)(5 A)(10 m)
()(1.0 V) 3.8 x 10 7 ( m)1
= 1.3 x 10-3 m = 1.3 mm
18-3
18.3 This problem asks that we compute, for a plain carbon steel wire 3 mm in diameter, the maximum
length such that the resistance will not exceed 20 . From Table 18.1 for a plain carbon steel = 0.6 x 107 (-m)1. If d is the diameter then, combining Equations 18.2 and 18.4 leads to
d 2
l = RA = R
2
3 x 103 m
= (20 ) 0.6 x 10 7 ( m)1 ()

2
= 848 m
18-4
18.4 Let us demonstrate, by appropriate substitution and algebraic manipulation, that Equation 18.5 may
be made to take the form of Equation 18.1. Now, Equation 18.5 is just
J = E
(In this equation we represent the electric field with an E.) But, by definition, J is just the current density, the
I
V
current per unit cross-sectional area, or J = . Also, the electric field is defined by E = . And, substituting
l
A
these expressions into Equation 18.5 leads to
I
V
=
l
A
But, from Equations 18.2 and 18.4

=
l
RA
and
l V
I
=
A RA l
Solving for V from this expression gives V = IR, which is just Equation 18.1.
18-5
18.5 (a) In order to compute the resistance of this aluminum wire it is necessary to employ Equations 18.2
and 18.4. Solving for the resistance in terms of the conductivity,
R=
l
l
=
=
A
A
l
d 2

2
From Table 18.1, the conductivity of aluminum is 3.8 x 107 (-m)-1, and
l
R=
d 2
5 m
5 x 103 m 2
3.8 x 10 7 ( m)1 ()
2
= 6.7 x 10-3
(b) If V = 0.04 V then, from Equation 18.1
I =
V
0.04 V
=
= 6.0 A
R 6.7 x 103
(c) The current density is just

J =
I
=
A
I
d 2
6.0 A
5 x 103 m 2
= 3.06 x 105 A/m2
(d) The electric field is just

E=
0.04 V
V
=
= 8.0 x 10-3 V/m
5 m
l
18-6
Electronic and Ionic Conduction

18.6 When a current arises from a flow of electrons, the conduction is termed electronic; for ionic
conduction, the current results from the net motion of charged ions.
18-7
Energy Band Structures in Solids

18.7 For an isolated atom, there exist discrete electron energy states (arranged into shells and subshells);
each state may be occupied by, at most, two electrons, which must have opposite spins. On the other hand, an
electron band structure is found for solid materials; within each band exist closely spaced yet discrete electron
states, each of which may be occupied by, at most, two electrons, having opposite spins. The number of electron
states in each band will equal the total number of corresponding states contributed by all of the atoms in the solid.
18-8
Conduction in Terms of Band and Atomic Bonding Models

18.8 This question asks that we explain the difference in electrical conductivity of metals, semiconductors,
and insulators in terms of their electron energy band structures.
For metallic materials, there are vacant electron energy states adjacent to the highest filled state; thus, very
little energy is required to excite large numbers of electrons into conducting states. These electrons are those that
participate in the conduction process, and, because there are so many of them, metals are good electrical conductors.
There are no empty electron states adjacent to and above filled states for semiconductors and insulators, but
rather, an energy band gap across which electrons must be excited in order to participate in the conduction process.
Thermal excitation of electrons will occur, and the number of electrons excited will be less than for metals, and will
depend on the band gap energy. For semiconductors, the band gap is narrower than for insulators; consequently, at
a specific temperature more electrons will be excited for semiconductors, giving rise to higher conductivities.
18-9
Electron Mobility
18.9 The drift velocity of a free electron is the average electron velocity in the direction of the force
imposed by an electric field.
The mobility is the proportionality constant between the drift velocity and the electric field. It is also a
measure of the frequency of scattering events (and is inversely proportional to the frequency of scattering).
18-10
18.10 (a) The drift velocity of electrons in Si may be determined using Equation 18.7. Since the room
temperature mobility of electrons is 0.14 m2/V-s (Table 18.3), and the electric field is 500 V/m (as stipulated in the
problem statement),
vd = e E
= (0.14 m2 /V - s)(500 V/m) = 70 m/s
(b) The time, t, required to traverse a given length, l (= 25 mm), is just
t =
l
25 x 103 m
=
= 3.6 x 10-4 s
vd
70 m /s
18-11
18.11 (a) The number of free electrons per cubic meter for aluminum at room temperature may be
computed using Equation 18.8 as
n=
| e | e
3.8 x 10 7 ( m)1
(1.602
x 1019 C)(0.0012 m2 / V - s)
= 1.98 x 1029 m-3

(b) In order to calculate the number of free electrons per aluminum atom, we must first determine the
number of copper atoms per cubic meter, NAl. From Equation 4.2 (and using the atomic weight and density values
for Al found inside the front coverviz. 26.98 g/mol and 2.71 g/cm3)
N Al =
(6.023
N A '
AAl
x 10 23 atoms / mol)(2.71 g /cm3)(10 6 cm3 / m3 )

26.98 g / mol
= 6.03 x 1028 m-3

(Note: in the above expression, density is represented by ' in order to avoid confusion with resistivity which is
designated by .) And, finally, the number of free electrons per aluminum atom is just n/NAl
n
1.98 x 10 29 m3
=
= 3.28
N Al
6.03 x 10 28 m3
18-12
18.12 (a) This portion of the problem asks that we calculate, for silver, the number of free electrons per
cubic meter (n) given that there are 1.3 free electrons per silver atom, that the electrical conductivity is 6.8 x 107 (' ) is 10.5 g/cm3. (Note: in this discussion, the density of silver is represented by
m)-1, and that the density (Ag
' in order to avoid confusion with resistivity which is designated by .) Since n = 1.3N , and N
Ag
Ag
Ag is defined in
Equation 4.2 (and using the atomic weight of Ag found inside the front coverviz 107.87 g/mol), then
'
Ag N A
n = 1.3N Ag = 1.3
AAg
(10.5 g /cm3)(6.023 x 10 23 atoms / mol)
= 1.3
107.87 g / mol
= 7.62 x 1022 cm-3 = 7.62 x 1028 m-3

(b) Now we are asked to compute the electron mobility, e. Using Equation 18.8
e =
n | e|
6.8 x 10 7 ( m)1
(7.62
x 10 28 m3 )(1.602 x 1019 C)
= 5.57 x 10-3 m2 /V - s
18-13
Electrical Resistivity of Metals

18.13 We want to solve for the parameter A in Equation 18.11 using the data in Figure 18.37. From
Equation 18.11
A=
ci (1 ci )
However, the data plotted in Figure 18.37 is the total resistivity, total, and includes both impurity (i) and thermal
(t) contributions (Equation 18.9). The value of t is taken as the resistivity at ci = 0 in Figure 18.37, which has a
value of 1.7 x 10-8 (-m); this must be subtracted out. Below are tabulated values of A determined at ci = 0.10,
0.20, and 0.30, including other data that were used in the computations. (Note: the ci values were taken from the
upper horizontal axis of Figure 18.37, since it is graduated in atom percent zinc.)
ci
1 ci
total (-m)
i (-m)
A (-m)
0.10
0.90
4.0 x 10-8
2.3 x 10-8
2.56 x 10-7
0.20
0.80
5.4 x 10-8
3.7 x 10-8
2.31 x 10-7
0.30
0.70
6.15 x 10-8
4.45 x 10-8
2.12 x 10-7
So, there is a slight decrease of A with increasing ci.
18-14
18.14 (a) Perhaps the easiest way to determine the values of 0 and a in Equation 18.10 for pure copper in
Figure 18.8, is to set up two simultaneous equations using two resistivity values (labeled t1 and t2) taken at two
corresponding temperatures (T1 and T2). Thus,
t1 = 0 + aT1
t2 = 0 + aT2
And solving these equations simultaneously lead to the following expressions for a and 0:
a=
t1 t 2
T1 T2

t2
0 = t1 T1 t1
T1 T2

t2
= t T2 t1
2
T1 T2
From Figure 18.8, let us take T1 = 150C, T2 = 50C, which gives t1 = 0.6 x 10-8 (-m), and t2 = 1.25 x 10-8
(-m). Therefore
a=
t1 t 2
T1 T2
[(0.6 x 10-8 ) (1.25 x 10-8 )]( - m)

150C (50C)
6.5 x 10-11 (-m)/C

and

t2
0 = t1 T1 t1
T1 T2
18-15
= (0.6 x 10-8 ) (150)
[(0.6 x 10-8 ) (1.25 x 10-8 )]( - m)

150C (50C)
= 1.58 x 10-8 (-m)

(b) For this part of the problem, we want to calculate A from Equation 18.11
i = Aci (1 ci )
In Figure 18.8, curves are plotted for three ci values (0.0112, 0.0216, and 0.0332). Let us find A for each of these
ci's by taking a total from each curve at some temperature (say 0C) and then subtracting out i for pure copper at
this same temperature (which is 1.7 x 10-8 -m). Below is tabulated values of A determined from these three ci
values, and other data that were used in the computations.
ci
1 ci
total (-m)
i (-m)
A (-m)
0.0112
0.989
3.0 x 10-8
1.3 x 10-8
1.17 x 10-6
0.0216
0.978
4.2 x 10-8
2.5 x 10-8
1.18 x 10-6
0.0332
0.967
5.5 x 10-8
3.8 x 10-8
1.18 x 10-6
The average of these three A values is 1.18 x 10-6 (-m).

(c) We use the results of parts (a) and (b) to estimate the electrical resistivity of copper containing 2.50
at% Ni (ci = 0.025) at120C. The total resistivity is just
total = t + i
Or incorporating the expressions for t and i from Equations 18.10 and 18.11, and the values of 0, a, and A
determined above, leads to
total = ( 0 + aT ) + Aci (1 ci )
{1.58 x 10 -8 ( - m) + [6.5 x 10 -11 ( - m) /C] (120C)}

+ {[1.18 x 10 -6 ( - m) ] (0.0250) (1 0.0250)}
= 5.24 x 10-8 (-m)
18-16
18.15 We are asked to determine the electrical conductivity of a Cu-Ni alloy that has a tensile strength of
275 MPa. From Figure 7.16(a), the composition of an alloy having this tensile strength is about 8 wt% Ni. For this
composition, the resistivity is about 14 x 10-8 -m (Figure 18.9). And since the conductivity is the reciprocal of the
resistivity, Equation 18.4, we have
=
1
1
=
= 7.1 x 10 6 ( - m)-1
14 x 108 m
18-17
18.16 This problem asks for us to compute the room-temperature conductivity of a two-phase Cu-Sn alloy
which composition is 89 wt% Cu-11 wt% Sn. It is first necessary for us to determine the volume fractions of the
and phases, after which the resistivity (and subsequently, the conductivity) may be calculated using Equation
18.12. Weight fractions of the two phases are first calculated using the phase diagram information provided in the
problem.
We may represent a portion of the phase diagram near room temperature as follows:
Applying the lever rule to this situation
W =
C C0
37 11
=
= 0.703
37 0
C C
C C
11 0
=
= 0.297
W = 0
37 0
C C
We must now convert these mass fractions into volume fractions using the phase densities given in the problem
statement. (Note: in the following expressions, density is represented by ' in order to avoid confusion with
resistivity which is designated by .) Utilization of Equations 9.6a and 9.6b leads to
W
V =
'
W W
+
' '
18-18
0.703
=
8.94 g /cm3
0.703
0.297
+
3
8.94 g /cm
8.25 g /cm 3
= 0.686
V =
W
'
W
'
+
W
'
0.297
=
8.25 g /cm3
0.703
0.297
+
3
8.94 g /cm
8.25 g /cm 3
= 0.314
Now, using Equation 18.12
= V + V
= (1.88 x 10-8 - m)(0.686) + (5.32 x 10-7 - m) (0.314)
= 1.80 x 10-7 -m
Finally, for the conductivity (Equation 18.4)
=
1
1
=
= 5.56 x10 6 ( - m)-1
1.80 x 107 m
18-19
18.17 We are asked to select which of several metals may be used for a 3 mm diameter wire to carry 12 A,
and have a voltage drop less than 0.01 V per foot (300 mm). Using Equations 18.3 and 18.4, let us determine the
minimum conductivity required, and then select from Table 18.1, those metals that have conductivities greater than
this value. Combining Equations 18.3 and 18.4, the minimum conductivity is just
=
Il
=
VA
Il
d 2
V
2
(12 A)(300 x 103 m)

3 x 103 m 2
(0.01 V) ()
2
= 5.1 x 10 7 ( - m)-1
Thus, from Table 18.1, only copper, and silver are candidates.
18-20
Intrinsic Semiconduction
18.18 (a) For this part of the problem, we first read, from Figure 18.16, the number of free electrons (i.e.,
the intrinsic carrier concentration) at room temperature (298 K). These values are ni(Ge) = 5 x 1019 m-3 and ni(Si)
= 7 x 1016 m-3.
Now, the number of atoms per cubic meter for Ge and Si (NGe and NSi, respectively) may be determined
' and ' ) and atomic weights (A and A ). (Note: here we
using Equation 4.2 which involves the densities ( Ge
Si
Ge
Si
use ' to represent density in order to avoid confusion with resistivity, which is designated by . Also, the atomic
weights for Ge and Si, 72.59 and 28.09 g/mol, respectively, are found inside the front cover.) Therefore,
N Ge =
(6.023
N A'Ge
AGe
x 10 23 atoms / mol)(5.32 g /cm 3)(10 6 cm3 / m3 )

72.59 g / mol
= 4.4 x 1028 atoms/m3

Similarly, for Si
N Si =
(6.023
N A'Si
ASi

28.09 g / mol
= 5.00 x 1028 atoms/m3

Finally, the ratio of the number of free electrons per atom is calculated by dividing ni by N. For Ge
ni (Ge)
N Ge
5 x 1019 electrons / m3
4.4 x 10 28 atoms / m3
1.1 x 10-9 electron/atom

And, for Si
18-21
ni (Si)
N Si
7 x 1016 electrons / m3
5.00 x 10 28 atoms / m3
= 1.4 x 10-12 electron/atom

(b) The difference is due to the magnitudes of the band gap energies (Table 18.3). The band gap energy at
room temperature for Si (1.11 eV) is larger than for Ge (0.67 eV), and, consequently, the probability of excitation
across the band gap for a valence electron is much smaller for Si.
18-22
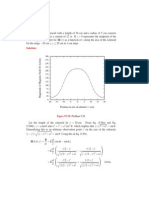
18.19 This problem asks that we make plots of ln ni versus reciprocal temperature for both Si and Ge,
using the data presented in Figure 18.16, and then determine the band gap energy for each material realizing that the
slope of the resulting line is equal to Eg/2k.
Below is shown such a plot for Si.
The slope of the line is equal to
Slope =
ln i
ln 1 ln 2
=
1
1
1

T1
T2
T
Let us take 1/T1 = 0.001 and 1/T2 = 0.007; their corresponding ln values are ln 1 = 54.80 and ln 2 = 16.00.
Incorporating these values into the above expression leads to a slope of
Slope =
16.00
54.80
= 6470
0.001 0.007
This slope leads to an Eg value of

Eg = 2k (Slope)
= 2 (8.62 x 105 eV / K)( 6470 ) = 1.115 eV
18-23
The value cited in Table 18.3 is 1.11 eV.

Now for Ge, an analogous plot is shown below.
We calculate the slope and band gap energy values in the manner outlined above. Let us take 1/T1 = 0.001 and 1/T2
= 0.011; their corresponding ln values are ln 1 = 55.56 and ln 2 = 14.80. Incorporating these values into the
above expression leads to a slope of
Slope =
14.80
55.56
= 4076
0.001 0.011
This slope leads to an Eg value of

Eg = 2k (Slope)
= 2 (8.62 x 105 eV / K)( 4076 ) = 0.70 eV
This value is in good agreement with the 0.67 eV cited in Table 18.3.
18-24
18.20 The factor 2 in Equation 18.35a takes into account the creation of two charge carriers (an electron
and a hole) for each valence-band-to-conduction-band intrinsic excitation; both charge carriers may participate in
the conduction process.
18-25
18.21 In this problem we are asked to compute the intrinsic carrier concentration for PbS at room
temperature. Since the conductivity and both electron and hole mobilities are provided in the problem statement, all
we need do is solve for n and p (i.e., ni) using Equation 18.15. Thus,
ni =
| e | (e + h )
25 ( - m)1
(1.602 x 1019 C)(0.06 + 0.02) m2 / V - s

= 1.95 x 1021 m-3
18-26
18.22 Yes, compound semiconductors can exhibit intrinsic behavior. They will be intrinsic even though
they are composed of two different elements as long as the electrical behavior is not influenced by the presence of
other elements.
18-27
18.23 This problem calls for us to decide for each of several pairs of semiconductors, which will have the
smaller band gap energy and then cite a reason for the choice.
(a) Germanium will have a smaller band gap energy than C (diamond) since Ge is lower in row IVA of the
periodic table (Figure 2.6) than is C. In moving from top to bottom of the periodic table, Eg decreases.
(b) Indium antimonide will have a smaller band gap energy than aluminum phosphide. Both of these
semiconductors are III-V compounds, and the positions of both In and Sb are lower vertically in the periodic table
(Figure 2.6) than Al and P.
(c) Gallium arsenide will have a smaller band gap energy than zinc selenide. All four of these elements
are in the same row of the periodic table, but Zn and Se are more widely separated horizontally than Ga and As; as
the distance of separation increases, so does the band gap.
(d) Cadmium telluride will have a smaller band gap energy than zinc selenide. Both are II-VI compounds,
and Cd and Te are both lower vertically in the periodic table than Zn and Se.
(e) Cadmium sulfide will have a smaller band gap energy than sodium chloride since Na and Cl are much
more widely separated horizontally in the periodic table than are Cd and S.
18-28
Extrinsic Semiconduction
18.24 These semiconductor terms are defined in the Glossary. Examples are as follows: intrinsic--high
purity (undoped) Si, GaAs, CdS, etc.; extrinsic--P-doped Ge, B-doped Si, S-doped GaP, etc.; compound--GaAs,
InP, CdS, etc.; elemental--Ge and Si.
18-29
18.25 For this problem we are to determine the electrical conductivity of and n-type semiconductor, given
that n = 5 x 1017 m-3 and the electron drift velocity is 350 m/s in an electric field of 1000 V/m. The conductivity of
this material may be computed using Equation 18.16. But before this is possible, it is necessary to calculate the
value of e from Equation 18.7. Thus, the electron mobility is equal to
v
e = d
E
=
350 m /s
= 0.35 m2 / V s
1000 V / m
Thus, from Equation 18.16, the conductivity is

= n | e |e
= (5 x 1017 m3 )(1.602 x 1019 C)(0.35 m2 / V s)
= 0.028 (-m)-1
18-30
18.26 The explanations called for are found in Section 18.11.
18-31
18.27 (a) No hole is generated by an electron excitation involving a donor impurity atom because the
excitation comes from a level within the band gap, and thus, no missing electron is created within the normally
filled valence band.
(b) No free electron is generated by an electron excitation involving an acceptor impurity atom because the
electron is excited from the valence band into the impurity level within the band gap; no free electron is introduced
into the conduction band.
18-32
18.28 Nitrogen will act as a donor in Si. Since it (N) is from group VA of the periodic table (Figure 2.6),
and an N atom has one more valence electron than an Si atom.
Boron will act as an acceptor in Ge. Since it (B) is from group IIIA of the periodic table, a B atom has one
less valence electron than a Ge atom.
Sulfur will act as a donor in InSb. Since S is from group VIA of the periodic table, it will substitute for Sb;
also, an S atom has one more valence electron than an Sb atom.
Indium will act as a donor in CdS. Since In is from group IIIA of the periodic table, it will substitute for
Cd; and, an In atom has one more valence electron than a Cd atom.
Arsenic will act as an acceptor in ZnTe. Since As is from group VA of the periodic table, it will substitute
for Te; furthermore, an As atom has one less valence electron than a Te atom.
18-33
18.29 (a) In this problem, for a Si specimen, we are given values for p (2.0 x 1022 m-3) and [500 (m)-1], while values for h and e (0.05 and 0.14 m2/V-s, respectively) are found in Table 18.3. In order to solve
for n we must use Equation 18.13, which, after rearrangement, leads to
n=
p | e | h
| e | e
500 ( m)1 (2.0 x 10 22 m3 )(1.602 x 1019 C)(0.05 m2 / V - s)
(1.602
x 1019 C)(0.14 m2 / V - s)
= 2.97 x 1020 m-3

(b) This material is p-type extrinsic since p (2.0 x 1022 m-3) is greater than n (2.97 x 1020 m-3).
18-34
18.30 (a) This germanium material to which has been added 1024 m-3 As atoms is n-type since As is a
donor in Ge. (Arsenic is from group VA of the periodic table--Ge is from group IVA.)
(b) Since this material is n-type extrinsic, Equation 18.16 is valid. Furthermore, each As atom will donate
a single electron, or the electron concentration is equal to the As concentration since all of the As atoms are ionized
at room temperature; that is n = 1024 m-3, and, as given in the problem statement, e = 0.1 m2/V-s. Thus
= n | e | e
= (10 24 m-3)(1.602 x 10-19 C)(0.1 m2 /V - s)

= 1.6 x 104 (-m)-1
18-35
18.31 In order to solve for the electron and hole mobilities for GaSb, we must write conductivity
expressions for the two materials, of the form of Equation 18.13i.e.,
= n | e | e + p | e | h
For the intrinsic material

8.9 x 10 4 ( - m)-1 = (8.7 x 10 23 m-3)(1.602 x 10-19 C) e
+ (8.7 x 10 23 m-3 )(1.602 x 10-19 C) h
which reduces to
0.639 = e + h
Whereas, for the extrinsic GaSb

2.3 x 105 ( - m)-1 = (7.6 x 10 22 m-3)(1.602 x 10-19 C) e
+ (1.0 x 10 25 m-3 )(1.602 x 10-19 C) h
which may be simplified to

0.1436 = 7.6 x 10-3 e + h
Thus, we have two independent expressions with two unknown mobilities. Upon solving these equations
simultaneously, we get e = 0.50 m2/V-s and h = 0.14 m2/V-s.
18-36
The Temperature Dependence of Carrier Concentration

18.32 In order to estimate the electrical conductivity of intrinsic silicon at 80C, we must employ Equation
18.15. However, before this is possible, it is necessary to determine values for ni, e, and h. According to Figure
18.16, at 80C (353 K), ni = 1.5 x 1018 m-3, whereas from the "<1020 m-3" curves of Figures 18.19a and 18.19b, at
80C (353 K), e = 0.10 m2/V-s and h = 0.035 m2/V-s (realizing that the mobility axes of these two plot are scaled
logarithmically). Thus, the conductivity at 80C is
= ni | e | (e + h )
= (1.5 x 1018 m3)(1.602 x 1019 C)(0.10 m2 /V - s + 0.035 m2 / V s)

= 0.032 ( - m)-1
18-37
18.33
This problem asks for us to assume that electron and hole mobilities for intrinsic Ge are
temperature-dependent, and proportional to T -3/2 for temperature in K. It first becomes necessary to solve for C in
Equation 18.36 using the room-temperature (298 K) conductivity [2.2 (-m)-1] (Table 18.3). This is accomplished
by taking natural logarithms of both sides of Equation 18.36 as
ln = ln C
Eg
3
lnT
2 kT
2
and after rearranging and substitution of values for Eg (0.67 eV, Table 18.3), and the room-temperature
conductivity, we get
ln C = ln +
= ln (2.2) +
Eg
3
lnT +
2 kT
2
0.67 eV
3
ln (298) +
2
(2)(8.62 x 105 eV / K)(298 K)
= 22.38
Now, again using Equation 18.36, we are able to compute the conductivity at 448 K (175C)
ln = ln C
= 22.38
Eg
3
ln T
2 kT
2
0.67 eV
3
ln (448 K)
2
(2)(8.62 x 105 eV / K)(448 K)
= 4.548
which leads to
= e4.548 = 94.4 (-m)-1.
18-38
18.34 This problem asks that we determine the temperature at which the electrical conductivity of intrinsic
Ge is 40 (-m)-1, using Equation 18.36 and the results of Problem 18.33. First of all, taking logarithms of Equation
18.36
ln = ln C
Eg
3
ln T
2 kT
2
And, from Problem 18.33 the value of ln C was determined to be 22.38. Using this and = 40 (-m)-1, the above
equation takes the form
ln 40 = 22.38
3
0.67 eV
ln T
2
(2)(8.62 x 105 eV / K)(T)
In order to solve for T from the above expression it is necessary to use an equation solver. For some solvers, the
following set of instructions may be used:
ln(40) = 22.38 1.5*ln(T) 0.67/(2*8.62*10^-5*T)
The resulting solution is T = 400, which value is the temperature in K; this corresponds to T(C) = 400 273 =
127C.
18-39
18.35 This problem asks that we estimate the temperature at which GaAs has an electrical conductivity of
1.6 x 10-3 (-m)-1 assuming that the conductivity has a temperature dependence as shown in Equation 18.36. From
the room temperature (298 K) conductivity [10-6 (-m)-1] and band gap energy (1.42 eV) of Table 18.3 we
determine the value of C (Equation 18.36) by taking natural logarithms of both sides of the equation, and after
rearrangement as follows:
ln C = ln +
= ln 106 ( m)1 +
Eg
3
ln T +
2 kT
2
3
1.42 eV
ln (298 K) +
2
(2)(8.62 x 105 eV / K)(298 K)
= 22.37
Now we substitute this value into Equation 18.36 in order to determine the value of T for which = 1.6 x 10-3 (m)-1, thus
ln = ln C
ln 1.6 x 10-3 ( - m)-1 = 22.37
Eg
3
ln T
2
2 kT
3
1.42 eV
lnT
2
(2)(8.62 105 eV / K) (T)
This equation may be solved for T using an equation solver. For some solvers, the following set of instructions may
be used:
ln(1.6*10^3) = 22.37 1.5*ln(T) 1.42/(2*8.62*10^5*T)
The resulting solution is T = 417; this value is the temperature in K which corresponds to T(C) = 417 K 273 =
144C.
18-40
18.36 This question asks that we compare and then explain the difference in temperature dependence of
the electrical conductivity for metals and intrinsic semiconductors.
For metals, the temperature dependence is described by Equation 18.10 (and converting from resistivity to
conductivity using Equation 18.4), as
=
1
0 + aT
That is, the electrical conductivity decreases with increasing temperature.

Alternatively, from Equation 18.8, the conductivity of metals is equal to
= n | e | e
As the temperature rises, n will remain virtually constant, whereas the mobility (e) will decrease, because the
thermal scattering of free electrons will become more efficient. Since |e| is independent of temperature, the net
result will be diminishment in the magnitude of .
For intrinsic semiconductors, the temperature-dependence of conductivity is just the opposite of that for
metalsi.e, conductivity increases with rising temperature.
One explanation is as follows:
Equation 18.15
describes the conductivity; i.e.,

= n | e | (e + h ) = p | e | (e + h )
= ni | e | (e + h )
Both n and p increase dramatically with rising temperature (Figure 18.16), since more thermal energy becomes
available for valence band-conduction band electron excitations. The magnitudes of e and h will diminish
somewhat with increasing temperature (per the upper curves of Figures 18.19a and 18.19b), as a consequence of the
thermal scattering of electrons and holes. However, this reduction of e and h will be overwhelmed by the
increase in n and p, with the net result is that increases with temperature.
An alternative explanation is as follows: for an intrinsic semiconductor the temperature dependence is
represented by an equation of the form of Equation 18.36. This expression contains two terms that involve
temperaturea preexponential one (in this case T -3/2) and the other in the exponential. With rising temperature the
preexponential term decreases, while the exp (Eg/2kT) parameter increases. With regard to relative magnitudes,
the exponential term increases much more rapidly than the preexponential one, such that the electrical conductivity
of an intrinsic semiconductor increases with rising temperature.
18-41
Factors That Affect Carrier Mobility

18.37 This problems asks that we determine the room-temperature electrical conductivity of silicon that
has been doped with 1023 m-3 of arsenic atoms. Inasmuch as As is a group VA element in the periodic table
(Figure 2.6) it acts as a donor in silicon. Thus, this material is n-type extrinsic, and it is necessary to use Equation
18.16), with n = 1023 m-3 since at room temperature all of the As donor impurities are ionized. The electron
mobility, from Figure 18.18 at an impurity concentration of 1023 m-3, is 0.065 m2/V-s. Therefore, the conductivity
is equal to
= n | e | e = (10 23 m3 )(1.602 x 1019 C)(0.065 m 2 / V s) = 1040 ( m)1
18-42
18.38 Here we are asked to calculate the room-temperature electrical conductivity of silicon that has been
doped with 2 x 1024 m-3 of boron atoms. Inasmuch as B is a group IIIA element in the periodic table (Figure 2.6) it
acts as an acceptor in silicon. Thus, this material is p-type extrinsic, and it is necessary to use Equation 18.17, with
p = 2 x 1024 m-3 since at room temperature all of the B acceptor impurities are ionized. The hole mobility, from
Figure 18.18 at an impurity concentration of 2 x 1024 m-3, is 0.0065 m2/V-s. Therefore, the conductivity is equal to
= p | e | e = (2 10 24 m3 )(1.602 1019 C)(0.0065 m2 / V s) = 2080 ( m)1
18-43
18.39 In this problem we are to estimate the electrical conductivity, at 75C, of silicon that has been doped
with 1022 m-3 of phosphorous atoms. Inasmuch as P is a group VA element in the periodic table (Figure 2.6) it acts
as a donor in silicon. Thus, this material is n-type extrinsic, and it is necessary to use Equation 18.16; n in this
expression is 1022 m-3 since at 75C all of the P donor impurities are ionized. The electron mobility is determined
using Figure 18.19a. From the 1022 m-3 impurity concentration curve and at 75C (348 K), e = 0.08 m2/V-s.
Therefore, the conductivity is equal to
= n | e | e = (10 22 m3 )(1.602 x 1019 C)(0.08 m2 / V s) = 128 ( m)1
18-44
18.40 In this problem we are to estimate the electrical conductivity, at 135C, of silicon that has been
doped with 1024 m-3 of aluminum atoms. Inasmuch as Al is a group IIIA element in the periodic table (Figure 2.6)
it acts as an acceptor in silicon. Thus, this material is p-type extrinsic, and it is necessary to use Equation 18.17; p
in this expression is 1024 m-3 since at 135C all of the Al acceptor impurities are ionized. The hole mobility is
determined using Figure 18.19b. From the 1024 m-3 impurity concentration curve and at 135C (408 K,) h = 0.007
m2/V-s. Therefore, the conductivity is equal to
= p | e | h = (10 24 m3 )(1.602 1019 C)(0.007 m2 / V s) = 1120 ( m)1
18-45
The Hall Effect

18.41 (a) This portion of the problem calls for us to determine the electron mobility for some hypothetical
metal using the Hall effect. This metal has an electrical resistivity of 3.3 x 10-8 (-m), while the specimen
thickness is 15 mm, Ix = 25 A and Bz = 0.95 tesla; under these circumstances a Hall voltage of 2.4 x 10-7 V is
measured. It is first necessary to convert resistivity to conductivity (Equation 18.4). Thus
=
1
1
=
= 3.0 x 10 7 ( - m)-1
8
3.3 x10 ( m)
The electron mobility may be determined using Equation 18.20b; and upon incorporation of Equation 18.18, we
have
e = RH
VH d
I x Bz
2.4 x 107 V (15 x 103 m) 3.0 x 10 7 ( m)1
=
(25 A)(0.95 tesla)
= 0.0045 m2 /V - s
(b) Now we are to calculate the number of free electrons per cubic meter. From Equation 18.8 we have
n =
| e | e
3.0 x 10 7 ( - m)1
(1.602
x 1019 C)(0.0045 m2 / V - s)
= 4.17 x 10 28 m-3
18-46
18.42 In this problem we are asked to determine the magnetic field required to produce a Hall voltage of
-3.5 x 10-7 V, given that = 1.2 x 107 (-m)-1, e = 0.0050 m2/V-s, Ix = 40 A, and d = 35 mm. Combining
Equations 18.18 and 18.20b, and after solving for Bz, we get
Bz =
VH d
I x e
3.5 x 107 V 1.2 x 10 7 ( m)1 (35 x 103 m)
(40 A)(0.0050 m2 / V - s)
= 0.74 tesla
18-47
Semiconducting Devices
18.43 The explanations called for are found in Section 18.15.
18-48
18.44 The energy generated by the electron-hole annihilation reaction, Equation 18.21, is dissipated as
heat.
18-49
18.45 In an electronic circuit, a transistor may be used to (1) amplify an electrical signal, and (2) act as a
switching device in computers.
18-50
18.46 The differences in operation and application for junction transistors and MOSFETs are described in
Section 18.15.
18-51
Conduction in Ionic Materials

18.47 We are asked in this problem to determine the electrical conductivity for the nonstoichiometric
Fe(1 - x)O, given x = 0.040 and that the hole mobility is 1.0 x 10-5 m2/V-s. It is first necessary to compute the
number of vacancies per cubic meter for this material. For this determination let us use as our basis 10 unit cells.
For the sodium chloride crystal structure there are four cations and four anions per unit cell. Thus, in ten unit cells
of FeO there will normally be forty O2- and forty Fe2+ ions. However, when x = 0.04, (0.04)(40) = 1.6 of the Fe2+
sites will be vacant. (Furthermore, there will be 3.2 Fe3+ ions in these ten unit cells inasmuch as two Fe3+ ions are
created for every vacancy). Therefore, each unit cell will, on the average contain 0.16 vacancies. Now, the number
of vacancies per cubic meter is just the number of vacancies per unit cell divided by the unit cell volume; this
volume is just the unit cell edge length (0.437 nm) cubed. Thus
# vacancies
m3
0.16 vacancies / unit cell
(0.437 109 m) 3
= 1.92 x 1027 vacancies/m3

Inasmuch as it is assumed that the vacancies are saturated, the number of holes (p) is also 1.92 x 1027 m-3. It is
now possible, using Equation 18.17, to compute the electrical conductivity of this material as
= p | e | h
= (1.92 10 27 m-3)(1.602 10-19 C)(1.0 10-5 m2 /V - s) = 3076 ( - m)-1
18-52
18.48 For this problem, we are given, for NaCl, the activation energy (173,000 J/mol) and preexponential
(4.0 x 10-4 m2/s) for the diffusion coefficient of Na+ and are asked to compute the mobility for a Na+ ion at 873 K.
The mobility, Na+, may be computed using Equation 18.23; however, this expression also includes the diffusion
coefficient DNa+, which is determined using Equation 5.8 as
Na +
Q
= D0 exp d
RT
173,000 J / mol
= (4.0 x 10-4 m2 /s) exp
(8.31 J / mol - K)(873 K)

= 1.76 x 10-14 m2 /s
Now solving for Na+ yields
Na +
Na +
eD
Na +
kT
(1)(1.602 x 1019 C /atom)(1.76 x 1014 m2 /s)
(1.38
x 1023 J /atom - K) (873 K)
= 2.34 x 10-13 m2 /V - s
(Note: the value of nNa+ is unity, since the valence for sodium is one.)
18-53
Capacitance
18.49 We want to compute the plate spacing of a parallel-plate capacitor as the dielectric constant is
increased form 2.2 to 3.7, while maintaining the capacitance constant. Combining Equations 18.26 and 18.27 yields
A
C= r 0
l
Now, let us use the subscripts 1 and 2 to denote the initial and final states, respectively. Since C1 = C2, then
r10 A
l1
r 2 0 A
l2
And, solving for l2
l2 =
r 2 l1
r1
(3.7)(2 mm)
= 3.36 mm
2.2
18-54
18.50 This problem asks for us to ascertain which of the materials listed in Table 18.5 are candidates for a
parallel-plate capacitor that has dimensions of 38 mm by 65 mm, a plate separation of 1.3 mm so as to have a
minimum capacitance of 7 x 10-11 F, when an ac potential of 1000 V is applied at 1 MHz. Upon combining
Equations 18.26 and 18.27 and solving for the dielectric constant r we get
r =
lC
0 A
(1.3 x 103 m)(7 x 1011 F)

(8.85 x 1012 F / m)(38 x 103 m)(65 x 103 m)
= 4.16
Thus, the minimum value of r to achieve the desired capacitance is 4.16 at 1 MHz. Of those materials listed in the
table, titanate ceramics, mica, steatite, soda-lime glass, porcelain, and phenol-formaldehyde are candidates.
18-55
18.51 In this problem we are given, for a parallel-plate capacitor, its area (3225 mm2), the plate separation
(1 mm), and that a material having an r of 3.5 is positioned between the plates.
(a) We are first asked to compute the capacitance. Combining Equations 18.26 and 18.27, and solving for
C yields
C =
r 0 A
l
(3.5)(8.85 x 1012 F / m)(3225 mm2 )(1 m2 /10 6 mm2 )

103 m
= 10-10 F = 100 pF
(b) Now we are asked to compute the electric field that must be applied in order that 2 x 10-8 C be stored
on each plate. First we need to solve for V in Equation 18.24 as
V =
Q
2 x 108 C
=
= 200 V
C
1010 F
The electric field E may now be determined using Equation 18.6; thus
E =
200 V
V
=
= 2.0 x 105 V/m
l
103 m
18-56
18.52 This explanation is found in Section 18.19.
18-57
Field Vectors and Polarization

Types of Polarization
18.53 Shown below are the relative positions of Ca2+ and O2- ions, without and with an electric field
present.
Now,
d = r 2+ + r 2- = 0.100 nm + 0.140 nm = 0.240 nm
Ca
O
and
d = 0.05 d = (0.05)(0.240 nm) = 0.0120 nm = 1.20 x 10 -11 m
From Equation 18.28, the dipole moment, p, is just

p = q d
= (1.602 x 10-19 C)(1.20 x 10-11 m)

= 1.92 x 10-30 C-m
18-58
18.54 (a) In order to solve for the dielectric constant in this problem, we must employ Equation 18.32, in
which the polarization and the electric field are given. Solving for r from this expression gives
r =
P
+ 1
0 E
4.0 x 106 C / m2
(8.85
x 1012 F / m)(1 x 105 V / m)
+ 1
= 5.52
(b) The dielectric displacement may be determined using Equation 18.31, as
D = 0 E + P
(8.85
x 10-12 F/m)(1 x 105 V/m) + 4.0 x 10-6 C/m2
= 4.89 x 10-6 C/m2
18-59
18.55 (a) We want to solve for the voltage when Q = 2.0 x 10-10 C, A = 650 mm2, l = 4.0 mm, and r =
3.5. Combining Equations 18.24, 18.26, and 18.27 yields
C =
Q
A
A
= = r 0
V
l
l
Or
Q
A
= r 0
V
l
And, solving for V, and incorporating values provided in the problem statement, leads to
V =
(2.0
Ql
r 0 A
x 1010 C)(4.0 x 103 m)
(3.5)(8.85 x 1012 F / m)(650 mm2 )(1 m2 /10 6 mm2 )
= 39.7 V
(b) For this same capacitor, if a vacuum is used
V =
(2.0
(8.85
Ql
0 A
x 1010 C)(4.0 x 103 m)
x 1012 F / m)(650 x 106 m2 )
= 139 V
(c) The capacitance for part (a) is just
C =
Q
2.0 x 1010 C
=
= 5.04 x 10-12 F
39.7 V
V
While for part (b)
18-60
C =
Q
2.0 x 1010 C
=
= 1.44 x 10-12 F
139 V
V
(d) The dielectric displacement may be computed by combining Equations 18.31, 18.32 and 18.6, as
V
D = 0 E + P = 0 E + 0 (r 1)E = 0r E = 0 r
l
And incorporating values for r and l provided in the problem statement, as well as the value of V computed in part
(a)
D=
(8.85 x 1012 F / m) (3.5)(39.7 V)

4.0 x 103 m
= 3.07 x 10-7 C/m2
(e) The polarization is determined using Equations 18.32 and 18.6 as

V
P = 0 (r 1)E = 0 (r 1)
l
(8.85 x 1012 F / m) (3.5 1)(39.7 V)

4.0 x 103 m
= 2.20 x 10-7 C/m2
18-61
18.56 (a) For electronic polarization, the electric field causes a net displacement of the center of the
negatively charged electron cloud relative to the positive nucleus. With ionic polarization, the cations and anions
are displaced in opposite directions as a result of the application of an electric field. Orientation polarization is
found in substances that possess permanent dipole moments; these dipole moments become aligned in the direction
of the electric field.
(b) Only electronic polarization is to be found in gaseous argon; being an inert gas, its atoms will not be
ionized nor possess permanent dipole moments.
Both electronic and ionic polarizations will be found in solid LiF, since it is strongly ionic. In all
probability, no permanent dipole moments will be found in this material.
Both electronic and orientation polarizations are found in liquid H2O. The H2O molecules have permanent
dipole moments that are easily oriented in the liquid state.
Only electronic polarization is to be found in solid Si; this material does not have molecules with
permanent dipole moments, nor is it an ionic material.
18-62
18.57 (a) This portion of the problem asks that we compute the magnitude of the dipole moment
associated with each unit cell of BaTiO3, which is illustrated in Figure 18.35. The dipole moment p is defined by
Equation 18.28 as p = qd in which q is the magnitude of each dipole charge, and d is the distance of separation
between the charges. Each Ti4+ ion has four units of charge associated with it, and thus q = (4)(1.602 x 10-19 C) =
6.41 x 10-19 C. Furthermore, d is the distance the Ti4+ ion has been displaced from the center of the unit cell,
which is just 0.006 nm + 0.006 nm = 0.012 nm [Figure 18.35(b)]. Hence
p = qd = (6.41 x 10-19 C)(0.012 x 10 -9 m)
= 7.69 x 10-30 C-m

(b) Now it becomes necessary to compute the maximum polarization that is possible for this material. The
maximum polarization will exist when the dipole moments of all unit cells are aligned in the same direction.
Furthermore, it is computed by dividing the above value of p by the volume of each unit cell, which is equal to the
product of three unit cell edge lengths, as shown in Figure 18.35. Thus
P =
p
VC
7.69 x 1030 C m
(0.403
x 109 m)(0.398 x 109 m)(0.398 x 109 m)
= 0.121 C/m2
18-63
Frequency Dependence of the Dielectric Constant

18.58 For this soda-lime glass, in order to compute the fraction of the dielectric constant at low
frequencies that is attributed to ionic polarization, we must determine the r within this low-frequency regime; such
is tabulated in Table 18.5, and at 1 MHz its value is 6.9. Thus, this fraction is just
fraction =
r (low) r (high)
r (low)
6.9 2.3
= 0.67
6.9
18-64
Ferroelectricity
18.59 The ferroelectric behavior of BaTiO3 ceases above its ferroelectric Curie temperature because the
unit cell transforms from tetragonal geometry to cubic; thus, the Ti4+ is situated at the center of the cubic unit cell,
there is no charge separation, and no net dipole moment.
18-65
DESIGN PROBLEMS
Electrical Resistivity of Metals

18.D1 This problem asks that we calculate the composition of a copper-nickel alloy that has a room
temperature resistivity of 2.5 x 10-7 -m. The first thing to do is, using the 90 Cu-10 Ni resistivity data, determine
the impurity contribution, and, from this result, calculate the constant A in Equation 18.11. Thus,
total = 1.90 x 10-7 ( - m) = i + t
From Table 18.1, for pure copper, and using Equation 18.4
1
1
=
= 1.67 x 10-8 ( - m)
7
6.0 x 10 ( m)1
t =
Thus, for the 90 Cu-10 Ni alloy

i = total t = 1.90 x 10-7 1.67 x 10-8
= 1.73 x 10-7 (-m)

In the problem statement, the impurity (i.e., nickel) concentration is expressed in weight percent. However,
Equation 18.11 calls for concentration in atom fraction (i.e., atom percent divided by 100).
Consequently,
conversion from weight percent to atom fraction is necessary. (Note: we now choose to denote the atom fraction of
' , and the weight percents of Ni and Cu by C and C , respectively.) Using these notations, this
nickel as cNi
Ni
Cu
conversion may be accomplished by using a modified form of Equation 4.6a as
' =
cNi
'
C Ni
100
C Ni ACu
C Ni ACu + CCu ANi
Here ANi and ACu denote the atomic weights of nickel and copper (which values are 58.69 and 63.55 g/mol,
respectively). Thus
' =
cNi
(10 wt%)(63.55 g / mol)

(10 wt%)(63.55 g / mol) + (90 wt%)(58.69 g / mol)
18-66
= 0.107
Now, solving for A in Equation 18.11
A =
' 1 c '
cNi
Ni
1.73 x 107 ( m)
= 1.81 x 10-6 ( - m)
(0.107 )(1 0.107 )
' to give a room temperature resistivity of 2.5 x 10-7 -m. Again, we must
Now it is possible to compute the cNi
determine i as
i = total t
= 2.5 x 10-7 1.67 x 10-8 = 2.33 x 10-7 ( - m)
If Equation 18.11 is expanded, then

' A c' 2
i = A cNi
Ni
Or, rearranging this equation, we have

' 2 Ac' + = 0
A cNi
i
Ni
' (using the quadratic equation solution)

Now, solving for cNi
' =
cNi
A 2 4 Ai
2A
Again, from the above

A = 1.81 x 10-6 (-m)
i = 2.33 x 10-7 (-m)
18-67
which leads to
1.81 x 106
' =
cNi
(1.81 x 106 ) 2 (4)(1.81 x 106 )(2.33 x 107 )

(2)(1.81 x 106 )
And, taking the negative root,

' = 0.152
cNi
Or, in terms of atom percent,

' = 100c ' = (100)(0.152) = 15.2 at%
C Ni
Ni
While the concentration of copper is

' = 100 C ' = 100 15.2 = 84.8 at%
CCu
Ni
Now, converting this composition to weight percent Ni, requires that we use Equation 4.7a as
C Ni =
' A
CNi
Ni
x 100
' A + C' A
C Ni
Ni
Cu Cu
(15.2 at%)(58.69 g / mol)

x 100
(15.2 at%)(58.69 g / mol) + (84.8 at%)(63.55 g / mol)
= 14.2 wt%
18-68
18.D2 This problem asks that we determine the electrical conductivity of an 85 wt% Cu-15 wt% Zn alloy
at 100C using information contained in Figures 18.8 and 18.37. In order to solve this problem it is necessary to
employ Equation 18.9 which is of the form
total = t + i
since it is assumed that the alloy is undeformed. Let us first determine the value of i at room temperature (25C)
which value will be independent of temperature. From Figure 18.8, at 25C and for pure Cu, t(25) = 1.75 x 10-8
-m. Now, since it is assumed that the curve in Figure 18.37 was generated also at room temperature, we may take
as total(25) at 85 wt% Cu-15 wt% Zn which has a value of 4.7 x 10-8 -m. Thus
i = total (25) t (25)
= 4.7 x 10-8 - m - 1.75 x 10-8 - m = 2.95 x 10-8 - m
Finally, we may determine the resistivity at 100C, total(100), by taking the resistivity of pure Cu at 100C
from Figure 18.8, which gives us t(100) = 0.90 x 10-8 -m. Therefore
total (100) = i + t (100)
= 2.95 x 10-8 - m + 0.90 x 10-8 - m = 3.85 x 10-8 - m
And, using Equation 18.4 the conductivity is calculated as

=
1
1
=
= 2.60 x 10 7 ( - m)-1
3.85 x 108 m
18-69
18.D3 To solve this problem, we want to consult Figures 7.16(b) and 18.9 in order to determine the Ni
concentration ranges over which the yield strength is greater than 130 MPa (19,000 psi) and the conductivity
exceeds 4.0 x 106 (-m)-1.
From Figure 7.16(b), a Ni concentration greater than about 23 wt% is necessary for a yield strength in
excess of 130 MPa. In Figure 18.9 is plotted the resistivity versus the Ni content. Since conductivity is the
1
reciprocal of resistivity, the resistivity must be less than 25 x 10-8 -m--i.e.,
. According to
4.0 x 10 6 ( m)1
the figure, this will be the case for Ni concentrations less than 17 wt%.
Hence, it is not possible to prepare an alloy meeting the criteria; for the stipulated yield strength the
required Ni content must be greater than 23 wt%, whereas for the required conductivity, less than 17 wt% Ni is
necessary.
18-70
Extrinsic Semiconduction
Factors That Affect Carrier Mobility
18.D4 First of all, those elements which, when added to silicon render it n-type, lie one group to the right
of silicon in the periodic table; these include the group VA elements (Figure 2.6)--i.e., nitrogen, phosphorus,
arsenic, and antimony.
Since this material is extrinsic and n-type, n >> p, and the electrical conductivity is a function of the hole
concentration according to Equation 18.16. Also, the number of free electrons is about equal to the number of
donor impurities, Nd. That is
n ~ Nd
From Equation 18.16, the conductivity is a function of both the electron concentration (n) and the electron mobility
(e). Furthermore, the room-temperature electron mobility is dependent on impurity concentration (Figure 18.18).
One way to solve this problem is to use an iterative approachi.e., assume some donor impurity concentration
(which will also equal the value of n), then determine a "calculated" electron mobility from Equation 18.16i.e.,
e =
n |e|
and, finally, compare this mobility with the "measured" value from Figure 18.18, taken at the assumed n (i.e., Nd)
value.
Let us begin by assuming that Nd = 1022 m-3. Thus, the "calculated" mobility value is
e =
200 ( m)1
=
= 0.125 m2 / V s
n | e | (10 22 m3 )(1.602 x 1019 C)
From Figure 18.18, at an impurity concentration of 1022 m-3 the "measured" electron mobility is 0.10 m2/V-s,
which is slightly lower than the "calculated" value.
For our next choice, let us assume a higher impurity concentration, say 1023 m-3.
At this higher
concentration there will be a reduction of both "calculated" and "measured" electron mobilities. The "calculated"
value is just
e =
200 ( m)1
=
= 0.0125 m2 / V s
n | e | (10 23 m3 )(1.602 x 1019 C)
18-71
Whereas, Figure 18.18 yields a "measured" e of 0.06 m2/V-s, which is higher than the "calculated" value.
Therefore, the correct impurity concentration will lie somewhere between 1022 and 1023 m-3 probably closer to the
lower of these two values. At 1.3 x 1022 m-3, both "measured" and "calculated" e values are about equal (0.095
m2/V-s).
It next becomes necessary to calculate the concentration of donor impurities in atom percent. This
computation first requires the determination of the number of silicon atoms per cubic meter, NSi, using Equation
4.2, which is as follows
N Si =
(6.023
'
N A Si
ASi

28.09 g / mol
= 5 x 1028 m-3
' in order to avoid confusion with
(Note: in the above discussion, the density of silicon is represented by Si
resistivity, which is designated by .)
The concentration of donor impurities in atom percent (C d' ) is just the ratio of Nd and (Nd + NSi)
multiplied by 100 as
C d' =
Nd
N d + N Si
x 100
1.3 x 10 22 m3
(1.3 x 10 22 m3) + (5 x 10 28 m3 )
x 100 = 2.6 x 10-5 at%
Now, conversion to weight percent (Cd) is possible using Equation 4.7a as
Cd =
C d' Ad
x 100
' A
C d' Ad + CSi
Si
where Ad and ASi are the atomic weights of the donor and silicon, respectively. Thus, the concentration in weight
percent will depend on the particular donor type. For example, for nitrogen
18-72
CN =
' A
CN
N
x 100
' A + C' A
CN
N
Si Si
(2.6 x 105 at%) (14.01 g / mol)

(2.6 x 105 at%) (14.01 g / mol) + (99.999974 at%)(28.09 g / mol)
x 100
= 1.3 x 10-5 wt%

Similar calculations may be carried out for the other possible donor impurities which yield
C P = 2.87 x 10-5 wt%
C As = 6.93 x 10-5 wt%
CSb = 1.127 x 10-4 wt%
18-73
18.D5 This problem asks for us to determine the temperature at which boron is to be diffused into highpurity silicon in order to achieve a room-temperature electrical conductivity of 1000 (-m)-1 at a distance 0.2 m
from the surface if the B concentration at the surface is maintained at 1.0 x 1025 m-3. It is first necessary for us to
compute the hole concentration (since B is an acceptor in Si) at this 0.2 m location.
From Equation 18.17, the conductivity is a function of both the hole concentration (p) and the hole
mobility (h). Furthermore, the room-temperature hole mobility is dependent on impurity concentration (Figure
18.18). One way to solve this problem is to use an iterative approachi.e., assume some boron concentration, NB
(which will also equal the value of p), then determine a "calculated" hole mobility from Equation 18.17i.e.,
h =
p| e |
and then compare this mobility with the "measured" value from Figure 18.18, taken at the assumed p (i.e., NB).
Let us begin by assuming that NB = 1024 m-3. Thus, the "calculated" mobility value is
h =
1000 ( m)1
=
= 0.0062 m2 / V s
p | e | (10 24 m3 )(1.602 x 1019 C)
From Figure 18.18, at an impurity concentration of 1024 m-3 the "measured" hole mobility is 0.01 m2/V-s, which is
higher than the "calculated" value.
For our next choice, let us assume a lower boron concentration, say 1023 m-3. At this lower concentration
there will be an increase of both "calculated" and "measured" hole mobilities. The "calculated" value is just
h =
1000 ( m)1
=
= 0.062 m2 / V s
p | e | (10 23 m3 )(1.602 x 1019 C)
Whereas, Figure 18.18 yields a "measured" h of 0.024 m2/V-s, which is lower than the "calculated" value.
Therefore, the correct impurity concentration will lie somewhere between 1023 and 1024 m-3. At 4.0 x 1023 m-3,
"measured" and "calculated" values are about equal (0.015 m2/V-s).
With regard to diffusion, the problem is one involving the nonsteady-state diffusion of B into the Si,
wherein we have to solve for temperature. Temperature is incorporated into the diffusion coefficient expression
given in the problem. But we must first employ the solution to Fick's second law for constant surface composition
boundary conditions, Equation 5.5; in this expression C0 is taken to be zero inasmuch as the problem stipulates that
the initial boron concentration may be neglected. Thus,
18-74
x
= 1 erf
Cs C0
2 Dt
C x C0
4.0 x 10 23 m3 0
1.0 x 10 25 m3 0
x
= 1 erf
2 Dt
which reduces to
x
0.9600 = erf
2 Dt
In order to solve this expression for a value
x
of it is necessary to interpolate using data in Table 5.1. Thus
2 Dt
erf(z)
1.4
0.9523
0.9600
1.5
0.9661
z 1.4
0.9600 0.9523
=
1.5 1.4
0.9661 0.9523
From which, z = 1.4558; which is to say

1.4558 =
x
2 Dt
Inasmuch as there are 3600 s/h (= t) and x = 0.2 m (= 2 x 10-7 m) the above equation becomes
1.4558 =
2 x 107 m
2 (D)(3600 s)
which, when solving for the value of D, leads to

2
1 2 107 m
= 1.31 1018 m2 /s
D=
3600 s (2)(1.4558)
18-75
Now, equating this value to the expression for D given in the problem gives
347,000 J / mol
D = 1.31 x 10-18 m2 /s = (2.4 x 10-4 ) exp
(8.31 J / mol - K)(T)
To solve for T, let us take the natural logarithms of both sides of the above equation; this leads to
ln (1.31 x 1018 ) = ln (2.4 x 104 )
41.176 = 8.335
347,000
8.31T
4.176 x 10 4
T
which yields a value for T of 1271 K (998C).
18-76
Conduction in Ionic Materials

18.D6 This problem asks, for the nonstoichiometric Fe(1 - x)O, given the electrical conductivity [1200 (m)-1] and hole mobility (1.0 x 10-5 m2/V-s) that we determine the value of x. It is first necessary to compute the
number of holes per unit volume (p) using Equation 18.17. Thus
p =
| e | h
1200 ( m)-1
(1.602 1019 C)(1.0 105 m2 / V - s)
= 7.49 10 26 holes/m3
Inasmuch as it is assumed that the acceptor states are saturated, the number of vacancies is also 7.49 x 1026 m-3.
Next, it is possible to compute the number of vacancies per unit cell by taking the product of the number of
vacancies per cubic meter times the volume of a unit cell. This volume is just the unit cell edge length (0.437 nm)
cubed:
# vacancies
= (7.49 10 26 m3)(0.437 x 109 m) 3 = 0.0625
unit cell
A unit cell for the sodium chloride structure contains the equivalence of four cations and four anions. Thus, if we
take as a basis for this problem 10 unit cells, there will be 0.625 vacancies, 40 O2- ions, and 39.375 iron ions (since
0.625 of the iron sites is vacant). (It should also be noted that since two Fe3+ ions are created for each vacancy, that
of the 39.375 iron ions, 38.125 of them are Fe2+ and 1.25 of them are Fe3+). In order to find the value of (1 x) in
the chemical formula, we just take the ratio of the number of total Fe ions (39.375) and the number of total Fe ion
sites (40). Thus
(1 x ) =
39.375
= 0.984
40
Or the formula for this nonstoichiometric material is Fe0.984O.
18-77
Semiconductor Devices
18.D7 (a) In this portion of the problem we are asked to determine the time required to grow a layer of
SiO2 that is 100 nm (i.e., 0.100 m) thick on the surface of a silicon chip at 1000C, in an atmosphere of O2
(oxygen pressure = 1 atm). Thus, using Equation 18.37, it is necessary to solve for the time t. However, before this
is possible, we must calculate the value of B from Equation 18.38a as follows:
1.24 eV
1.24 eV
B = 800 exp
= (800) exp
kT
(8.62 10-5 eV/atom - K)(1000 + 273 K)
= 0.00990 m2/h
Now, solving for t from Equation 18.37 using the above value for B and that x = 0.100 m, we have
t =
(0.100 m) 2
x2
=
B
0.00990 m2 / h
= 1.01 h
Repeating the computation for B at 700C:
1.24 eV
B = (800) exp
(8.62 10-5 eV/atom - K)(700 + 273 K)
= 3.04 10-4 m2/h

And solving for the oxidation time as above
t =
(0.100 m) 2
3.04 10-4 m2 / h
= 32.9 h
(b) This part of the problem asks for us to compute the heating times to form an oxide layer 100 nm thick at
the same two temperatures (1000C and 700C) when the atmosphere is water vapor (1 atm pressure). At 1000C,
the value of B is determined using Equation 18.38b, as follows:
18-78
0.70 eV
0.70 eV
B = 215 exp
= (215) exp
kT
(8.62 10-5 eV/atom - K)(1000 + 273 K)
= 0.365 m2/h
And computation of the time t from the rearranged form of Equation 18.37, leads to
t =
x2
(0.100 m) 2
=
B
0.365 m2 / h
= 0.0274 h = 98.6 s
And at 700C, the value of B is
0.70 eV
B = (215) exp
= 0.0510 m2 / h
(8.62 10-5 eV/atom - K)(700 + 273 K)
Whereas the time required to grow the 100 nm oxide layer is
t =
x2
(0.100 m) 2
=
B
0.0510 m2 / h
= 0.196 h = 706 s
From the above computations, it is very apparent (1) that the 100 nm oxide layer forms more rapidly at
1000C (than at 700C) in both O2 and H2O gaseous atmospheres, and (2) that the oxide layer formation is more
rapid in water vapor than in oxygen.
18-79
18.D8 We are asked to compare silicon and gallium arsenide semiconductors relative to properties and
applications.
The following are the characteristics and applications for Si: (1) being an elemental semiconductor, it is
cheaper to grow in single-crystalline form; (2) because of its electron band structure, it is best used in transistors;
(3) electronic processes are relatively slow due to the low mobilities for electrons and holes (Table 18.3).
For GaAs: (1) it is much more expensive to produce inasmuch as it is a compound semiconductor; (2)
because of its electron band structure it is best used in light-emitting diodes and semiconducting lasers; (3) its band
gap may be altered by alloying; (4) electronic processes are more rapid than in Si due to the greater mobilities for
electrons and holes; (5) absorption of electromagnetic radiation is greater in GaAs, and therefore, thinner layers are
required for solar cells.

CH18

Uploaded by

Copyright:

Available Formats

CH18

Uploaded by

Document Information

Copyright

Available Formats

Share this document

Share or Embed Document

Sharing Options

Did you find this document useful?

Is this content inappropriate?

Copyright:

Available Formats

CH18

Uploaded by

Copyright:

Available Formats

What are some properties and applications of silicon semiconductors?

What are some properties and applications of silicon semiconductors?

What are some properties and applications of gallium arsenide semiconductors?

What are some properties and applications of gallium arsenide semiconductors?

18-1

(0.25 A)(45 x 103 m)

()(1.0 V) 3.8 x 10 7 ( m)1

= 1.3 x 10-3 m = 1.3 mm

= (20 ) 0.6 x 10 7 ( m)1 ()

But, from Equations 18.2 and 18.4

(c) The current density is just

= 3.06 x 105 A/m2

(d) The electric field is just

Electronic and Ionic Conduction

Energy Band Structures in Solids

Conduction in Terms of Band and Atomic Bonding Models

= (0.14 m2 /V - s)(500 V/m) = 70 m/s

(b) The time, t, required to traverse a given length, l (= 25 mm), is just

= 1.98 x 1029 m-3

x 10 23 atoms / mol)(2.71 g /cm3)(10 6 cm3 / m3 )

= 6.03 x 1028 m-3

(10.5 g /cm3)(6.023 x 10 23 atoms / mol)

= 7.62 x 1022 cm-3 = 7.62 x 1028 m-3

Electrical Resistivity of Metals

So, there is a slight decrease of A with increasing ci.

[(0.6 x 10-8 ) (1.25 x 10-8 )]( - m)

6.5 x 10-11 (-m)/C

= (0.6 x 10-8 ) (150)

[(0.6 x 10-8 ) (1.25 x 10-8 )]( - m)

= 1.58 x 10-8 (-m)

The average of these three A values is 1.18 x 10-6 (-m).

{1.58 x 10 -8 ( - m) + [6.5 x 10 -11 ( - m) /C] (120C)}

Applying the lever rule to this situation

(12 A)(300 x 103 m)

x 10 23 atoms / mol)(5.32 g /cm 3)(10 6 cm3 / m3 )

= 4.4 x 1028 atoms/m3

x 10 23 atoms / mol)(2.33 g /cm3)(10 6 cm3 / m3 )

= 5.00 x 1028 atoms/m3

1.1 x 10-9 electron/atom

= 1.4 x 10-12 electron/atom

The slope of the line is equal to

This slope leads to an Eg value of

The value cited in Table 18.3 is 1.11 eV.

This slope leads to an Eg value of

(1.602 x 1019 C)(0.06 + 0.02) m2 / V - s

Thus, from Equation 18.16, the conductivity is

= (5 x 1017 m3 )(1.602 x 1019 C)(0.35 m2 / V s)

18.26 The explanations called for are found in Section 18.11.

500 ( m)1 (2.0 x 10 22 m3 )(1.602 x 1019 C)(0.05 m2 / V - s)

= 2.97 x 1020 m-3

= (10 24 m-3)(1.602 x 10-19 C)(0.1 m2 /V - s)

For the intrinsic material

+ (8.7 x 10 23 m-3 )(1.602 x 10-19 C) h

Whereas, for the extrinsic GaSb

which may be simplified to

The Temperature Dependence of Carrier Concentration

= (1.5 x 1018 m3)(1.602 x 1019 C)(0.10 m2 /V - s + 0.035 m2 / V s)

ln 1.6 x 10-3 ( - m)-1 = 22.37

That is, the electrical conductivity decreases with increasing temperature.

One explanation is as follows: