Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd Logo
Upload

Welcome to Scribd!

  • 44 views

    Ap Questions Chapter 2.1-2.4

    Uploaded by

    quangdungta10
    The document contains practice questions related to calculus concepts such as derivatives, limits, and the intermediate value theorem. There are multiple choice questions testing skills like finding derivatives of functions, limits, and applying theorems. The document would be useful for practicing calculus skills and concepts.

    Copyright:

    © All Rights Reserved
    Download as DOCX, PDF, TXT or read online from Scribd

    Ap Questions Chapter 2.1-2.4

    Uploaded by

    quangdungta10
    44 views4 pages
    The document contains practice questions related to calculus concepts such as derivatives, limits, and the intermediate value theorem. There are multiple choice questions testing skills like finding derivatives of functions, limits, and applying theorems. The document would be useful for practicing calculus skills and concepts.

    Original Title

    ap_questions_chapter_2.1-2.4

    Copyright

    © © All Rights Reserved

    Available Formats

    DOCX, PDF, TXT or read online from Scribd

    Did you find this document useful?

    Is this content inappropriate?

    The document contains practice questions related to calculus concepts such as derivatives, limits, and the intermediate value theorem. There are multiple choice questions testing skills like finding derivatives of functions, limits, and applying theorems. The document would be useful for practicing calculus skills and concepts.

    Copyright:

    © All Rights Reserved
    Download as DOCX, PDF, TXT or read online from Scribd
    Download as docx, pdf, or txt
    44 views4 pages

    Ap Questions Chapter 2.1-2.4

    Uploaded by

    quangdungta10
    The document contains practice questions related to calculus concepts such as derivatives, limits, and the intermediate value theorem. There are multiple choice questions testing skills like finding derivatives of functions, limits, and applying theorems. The document would be useful for practicing calculus skills and concepts.

    Copyright:

    © All Rights Reserved
    Download as DOCX, PDF, TXT or read online from Scribd
    Download as docx, pdf, or txt
    of 4

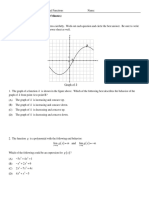
    Practice AP Questions 2.1-2.

    4 Name:___________________________________

    1) If f(x) = , then f’(0) =

    (A) -2 (B) 0 (C) 1 (D) (E)

    2) A particle moves along the x-axis in such a way that its position at time t is given by . What is the
    acceleration of the particle at time t=0?

    (A) -4

    (B) -2

    (C) -3/5

    (D) 2

    (E) 4

    3) Let f be the function given by f(x) = tanx and let g be the function given by g(x) = x 2. At what value of x in the interval
    0 ≤ x ≤ π do the graphs of f and g have parallel tangent lines?

    (A) 0

    (B) 0.660

    (C) 2.083

    (D) 2.194

    (E) 2.207

    4) Two functions f(x) and g(x) are differentiable. If h(x) = x2g(x) – f(3x + 1), determine the value of h’(x).

    (A) 2xg’(x) – 3f’(3x + 1)

    (B) 2xg’(x) – f’(3)

    (C) x2g’(x) + 2xg(x) – f’(3x + 1)


    (D) x2g’(x) + 2xg(x) – 3f’(3x + 1)

    (E) x2g’(x) – f’(3x + 1)

    5) Let f and g be differentiable functions such that

    If h(x) = f(g(x)), then h’(1) =

    (A)
    -9 (B) 15 (C) 0 (D) -5 (E) -12

    6) If f(x) = (2 + 3x)4, then the fourth derivative of f is

    (A) 0 (B) 4!(3) (C) 4!(34) (D) 4!(35) (E) 4!(2 + 3x)

    7) The at x = 3 is

    (A) -1 (B) 0 (C) 1 (D) 3 (E) nonexistent

    8) If y = 7 is a horizontal asymptote of a rational function f, then which of the following must be true?

    (A) (B) (C) (D) (E)


    9) Let f(x) be a continuous and differentiable function. The table below gives the values of f(x) and f’(x), the derivative of

    f(x), at several values. If g(x) = , what is the value of g’(2)?

    (A) -1/8

    (B) 0 x 1 2 3 4
    f(x) -3 -8 -9 0
    (C) 1/16 f’(x) -5 -4 3 16

    (D) 1/64

    (E) 16

    10) If f(x) = cos2(x), then f ’’ (π) =

    (A) -2 (B) 0 (C) 1 (D) 2 (E) 2π

    11) Two particles leave the origin at the same time and move along the y-axis with their respective positions determined
    by the functions y1 = cos2t and y2 = 4sint for 0 < x < 6. For how many values of t do the particles have the same
    acceleration?

    (A) 0 (B) 1 (C) 2 (D) 3 (E) 4

    12) Evaluate .

    (A) 5/2 (B) 5/16 (C) 40 (D) 160 (E) The limit DNE

    13) If f is continuous on [2, 6], with f(2) = 20 and f(6) = 10, then the Intermediate Value Theorem says which of the
    following is true?

    I. f(x) = 25 does not have a solution on [2, 6]

    II. f(x) = 17 has a solution on [2, 6]

    III. f(x) = 0 has a solution on [2, 6]


    (A) I only (B) II only (C) III only (D) I and II only (E) I, II, and III

    You might also like