T P
- 1. Sunil Patil
- 2. The transportation problem is a special type of linear programming problem, where the objective is to minimize the cost of distributing a product from a number of sources to a number of destinations.
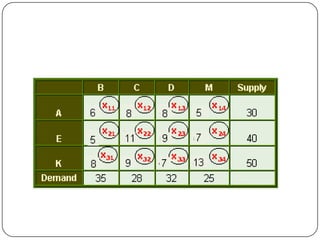
- 3. Example firm has 3 factories - A, E, and K. There are four major warehouses situated at B, C, D, and M. Average daily product at A, E, K is 30, 40, and 50 units respectively. The average daily requirement of this product at B, C, D, and M is 35, 28, 32, 25 units respectively.
- 6. The problem can be formulated mathematically in the linear programming form asMinimize = 6x11 + 8x12 + 8x13 + 5x14 + 5x21 + 11x22 + 9x23 + 7x24+ 8x31 + 9x32 + 7x33 + 13x34subject toCapacity constraintsx11 + x12 + x13 + x14 = 30x21 + x22 + x23 + x24 = 40x31 + x32 + x33 + x34 = 50Requirement constraintsx11 + x21 + x31 = 35x12 + x22 + x32 = 28x13 + x23 + x33 = 32x14 + x24 + x34 = 25xij ³ 0
- 8. For a feasible solution to exist, it is necessary that total capacity equals total requirements. Total supply = total demand.If total supply = total demand then it is a balanced transportation problem.There will be (m + n – 1) basic independent variables out of (m x n) variables.
- 9. OriginIt is the location from which shipments are dispatched.DestinationIt is the location to which shipments are transported.Unit Transportation costIt is the cost of transporting one unit of the consignment from an origin to a destination.Feasible SolutionBasic Feasible SolutionOptimal Solution
- 10. Feasible SolutionA solution that satisfies the row and column sum restrictions and also the non-negativity restrictions is a feasible solution.Basic Feasible SolutionA feasible solution of (m X n) transportation problem is said to be basic feasible solution, when the total number of allocations is equal to (m + n – 1).Optimal SolutionA feasible solution is said to be optimal solution when the total transportation cost will be the minimum cost.
- 11. Methods for finding an initial basic feasible solution:North West Corner RuleMatrix Minimum MethodVogel Approximation Method
- 12. North West Corner RuleThe North West corner rule is a method for computing a basic feasible solution of a transportation problem, where the basic variables are selected from the North – West corner ( i.e., top left corner ).
- 13. Steps : North West Corner RuleSelect the north west (upper left-hand) corner cell of the transportation table and allocate as many units as possible equal to the minimum between available supply and demand, i.e., min(s1, d1).Adjust the supply and demand numbers in the respective rows and columns.If the demand for the first cell is satisfied, then move horizontally to the next cell in the second column.If the supply for the first row is exhausted, then move down to the first cell in the second row.If for any cell, supply equals demand, then the next allocation can be made in cell either in the next row or column.Continue the process until all supply and demand values are exhausted.
- 15. Matrix Minimum MethodMatrix minimum (Least cost) method is a method for computing a basic feasible solution of a transportation problem, where the basic variables are chosen according to the unit cost of transportation. This method is very useful because it reduces the computation and the time required to determine the optimal solution. The following steps summarize the approach.
- 16. Steps : Matrix Minimum MethodIdentify the box having minimum unit transportation costIf the minimum cost is not unique, then you are arbitrary to choose any cell.Choose the value of the corresponding as much as possible subject to the capacity and requirement constraints.Repeat steps 1-3 until all restrictions are satisfied.
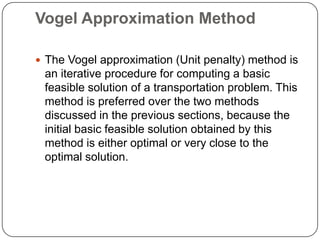
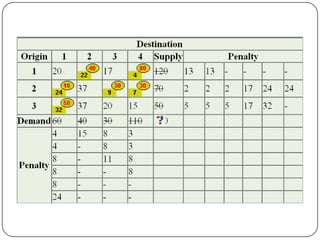
- 18. Vogel Approximation MethodThe Vogel approximation (Unit penalty) method is an iterative procedure for computing a basic feasible solution of a transportation problem. This method is preferred over the two methods discussed in the previous sections, because the initial basic feasible solution obtained by this method is either optimal or very close to the optimal solution.
- 19. Steps Vogel Approximation MethodIdentify the boxes having minimum and next to minimum transportation cost in each row and write the difference (penalty) along the side of the table against the corresponding row.Identify the boxes having minimum and next to minimum transportation cost in each column and write the difference (penalty) against the corresponding columnIdentify the maximum penalty. If it is along the side of the table, make maximum allotment to the box having minimum cost of transportation in that row. If it is below the table, make maximum allotment to the box having minimum cost of transportation in that column.If the penalties corresponding to two or more rows or columns are equal, you are at liberty to break the tie arbitrarily.Repeat the above steps until all restrictions are satisfied.
- 24. Maximization In A Transportation ProblemSolution.Maximization transportation problem can be converted into minimization transportation problem by subtracting each transportation cost from maximum transportation cost.
- 25. Maximization In A Transportation ProblemSurya Roshni Ltd. has three factories - X, Y, and Z. It supplies goods to four dealers spread all over the country. The production capacities of these factories are 200, 500 and 300 per month respectively.Determine a suitable allocation to maximize the total net return.
- 26. Unbalanced Transportation ProblemCertain situations, the total supply is not equal to the total demand. Thus, the transportation problem with unequal supply and demand is said to be unbalanced transportation problem.
- 27. Solution:If the total supply is more than the total demand, we introduce an additional column, which will indicate the surplus supply with transportation cost zero. Similarly, if the total demand is more than the total supply, an additional row is introduced in the table, which represents unsatisfied demand with transportation cost zero. The balancing of an unbalanced transportation problem is illustrated in the following example
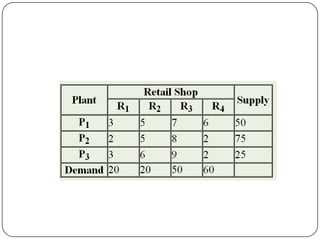
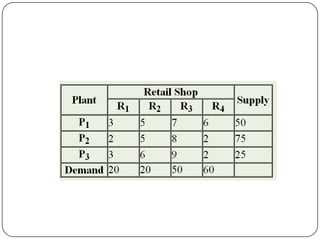
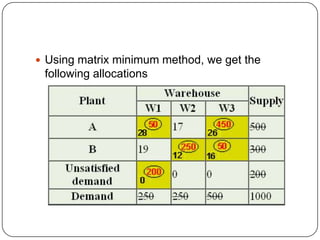
- 29. Using matrix minimum method, we get the following allocations
- 30. Prohibited RoutesSometimes there may be situations, where it is not possible to use certain routes in a transportation problem.For example, road construction, bad road conditions, strike, unexpected floods, local traffic rules, etc. We can handle such type of problems in different ways:A very large cost represented by M or ∞ is assigned to each of such routes, which are not available.
- 32. Thank You…!