(6) Hyperbola (Theory).Module-3pdf

Total no.of questions in Hyperbola are-
Inchapter examples..................................................10
Solved Examples............................................... ......14
Total no. of questions.............................................. 24
HYPERBOLA

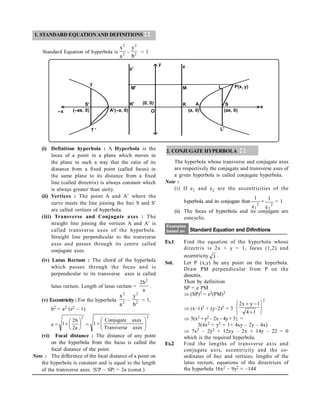
(i) Definition hyperbola : A Hyperbola is the
locus of a point in a plane which moves in
the plane in such a way that the ratio of its
distance from a fixed point (called focus) in
the same plane to its distance from a fixed
line (called directrix) is always constant which
is always greater than unity.
(ii) Vertices : The point A and A where the
curve meets the line joining the foci S and S
are called vertices of hyperbola.
(iii) Transverse and Conjugate axes : The
straight line joining the vertices A and A is
called transverse axes of the hyperbola.
Straight line perpendicular to the transverse
axes and passes through its centre called
conjugate axes.
(iv) Latus Rectum : The chord of the hyperbola
which passes through the focus and is
perpendicular to its transverse axes is called
latus rectum. Length of latus rectum =
a
b
2 2
.
(v) Eccentricity : For the hyperbola 2
2
a
x
– 2
2
b
y
= 1,
b2 = a2 (e2 – 1)
e =
2
a
2
b
2
1 





 =
2
axes
Transverse
axes
Conjugate
1 








(vi) Focal distance : The distance of any point
on the hyperbola from the focus is called the
focal distance of the point.
Note : The difference of the focal distance of a point on
the hyperbola is constant and is equal to the length
of the transverse axes. |SP – SP| = 2a (const.)
z'
y z
P(x, y)
S
(ae, 0)
x
A
L'
L
M
M'
K' K
A'(–a, 0)
T '
–x
T
(a, 0)
(0, 0)
O
(–ae, 0)
S'
2. CONJUGATE HYPERBOLA
The hyperbola whose transverse and conjugate axes
are respectively the conjugate and transverse axes of
a given hyperbola is called conjugate hyperbola.
Note :
(i) If e1 and e2 are the eccentricities of the
hyperbola and its conjugate then 2
1
e
1
+
2
2
e
1
= 1
(ii) The focus of hyperbola and its conjugate are
concyclic.
Examples
based on Standard Equation and Difinitions
Ex.1 Find the equation of the hyperbola whose
directrix is 2x + y = 1, focus (1,2) and
eccentricity 3 .
Sol. Let P (x,y) be any point on the hyperbola.
Draw PM perpendicular from P on the
directrix.
Then by definition
SP = e PM
 (SP)2 = e2(PM)2
(x–1)2 + (y–2)2 = 3
2
1
4
1
y
x
2









5(x2 + y2 – 2x – 4y+ 5} =
3(4x2 + y2 + 1+ 4xy – 2y – 4x)
 7x2 – 2y2 + 12xy – 2x + 14y – 22 = 0
which is the required hyperbola.
Ex.2 Find the lengths of transverse axis and
conjugate axis, eccentricity and the co-
ordinates of foci and vertices; lengths of the
latus rectum, equations of the directrices of
the hyperbola 16x2 – 9y2 = –144
1. STANDARD EQUATION AND DEFINITIONS
Standard Equation of hyperbola is 2
2
a
x
– 2
2
b
y
= 1

Sol. The equation 16x2 – 9y2 = – 144 can be
written as
9
x2
–
16
y2
= – 1. This is of the form
2
2
a
x
– 2
2
b
y
= – 1
 a2 = 9, b2 = 16  a = 3, b = 4
Length of transverse axis :
The length of transverse axis = 2b = 8
Length of conjugate axis :
The length of conjugate axis = 2a = 6
Eccentricity : e = 2
2
b
a
1 =
16
9
1 =
4
5
Foci : the coordinates of the foci are (0,± be), i.e.,
(0, ± 4)
Length of Latus rectum :
The length of latus rectum =
b
a
2 2
=
4
)
3
(
2 2
=
2
9
Equation of directrices :
The equation of directrices are y = ±
e
b
y = ±
 
4
/
5
4
= ±
5
16
Ex.3 Find the coordinates of foci, the eccentricity
and latus- rectum, equations of directrices
for the hyperbola 9x2–16y2–72x+96y–144=0
Sol. Equation can be rewritten as
2
2
4
)
4
x
( 
– 2
2
3
)
3
y
( 
= 1 so a = 4, b = 3
b2 = a2 (e2 – 1) gives e = 5/4
Foci : X = ± ae, Y = 0 gives the foci as
(9,3), (– 1,3)
Centre : X = 0, Y = 0 i.e. (4,3)
Directrices : X = ± a/e i.e. x – 4 = ± 16/5
 directrices are 5x – 36 = 0; 5x – 4 = 0
Latus- rectum = 2b2/ a = 2 x 9/4= 9/2
Ex.4 Two straight lines pass through the fixed
points (± a,0) and have gradients whose
product is k. Show that the locus of the
points of inter-section of the lines is a
hyperbola.
Sol. y= m1(x –a),y= m2(x+a) wherem1m2 =k, given
In order to find the locus of their point of
intersection we have to eliminate the unknown
m1 and m2. Multiplying, we get
y2 = m1m2 (x2 – a2) or y2 = k(x2–a2)
or
1
x2
–
k
y2
= a2 which represents a hyperbola.
Ex.5 The eccentricity of the conic represented by
x2 – y2 – 4x + 4y + 16 = 0 is
(A) 1 (B) 2 (C) 2 (D)1/2
Sol.[B] We have x2 – y2 – 4x +4y+16 = 0
or, (x2 – 4x)– (y2 – 4y) = 16
or, (x2 – 4x + 4) – (y2 – 4y+ 4) = – 16
or, (x–2)2 – (y – 2)2 = – 16
or, 2
2
4
)
2
x
( 
– 2
2
4
)
2
y
( 
= 1
Shifting the origin at (2,2) , we obtain
2
2
4
X
– 2
2
4
Y
= – 1, where x = X + 2, y = Y + 2
This is a rectangular hyperbola, whose
eccentricity is always 2 .
Ex.6 The equation 16x2 = 3y2 – 32x + 12y – 44= 0
represents a hyperbola
(A) the length of whose transverse axis is 3
4
(B) the length of whose conjugate axis is 4
(C) whose centre is (– 1,2)
(D) whose eccentricity is
3
19
Sol.[D] We have, 16(x2 – 2x) – 3 (y2 – 4y) = 44
 16 (x –1)2 – 3 (y– 2)2 = 48

3
)
1
x
( 2

–
16
)
2
y
( 2

= 1
This equation represents a hyperbola with
eccentricity given
e =
2
axis
Transverse
axis
Conjugate
1 







 =
2
3
4
1 







 =
3
19
3. Parametric equation of the Hyperbola
Let the equation of ellipse in standard form will be
given by 2
2
a
x
– 2
2
b
y
= 1

Then the equation of ellipse in the parametric form
will be given by x= a sec , y = b tan  where  is
the eccentric angle whose value vary from 0   <
2. Therefore coordinate of any point P on the ellipse
will be given by (a sec, b tan ).
4. POSITION OFAPOINT P(x1, y1) WITH RESPECT
TO HYPERBOLA
The quantity 2
2
1
a
x
– 2
2
1
b
y
= 1 is positive, zero or
negative according as the point (x1, y1) lies inside on
or outside the hyperbola 2
2
a
x
– 2
2
b
y
= 1.
5. LINE & HYPERBOLA
‘’The straight line y = mx + c is a sacant, a tangent or
passes outside the hyperbola 2
2
a
x
– 2
2
b
y
= 1 according
as c2 > = < a2m2 – b2
Examples
based on Line and Hyperbola
Ex.7 Find the position of the point (5, – 4) relative
to the hyperbola 9x2 – y2 = 1.
Sol. Since 9(5)2 –(–4)2 –1=225–16 –1 =208 > 0
so the point ( 5,–4) lies inside the hyperbola
9x2– y2 = 1
S.No. Particulars Hyperbola Conjugate Hyperbola
2
2
a
x
– 2
2
b
y
= 1 – 2
2
a
x
+ 2
2
b
y
= 1
1. Co-ordinate of the centre (0, 0) (0, 0)
2. Co-ordinate of the vertices (a, 0) & (–a, 0) (0, b) & (0, –b)
3. Co-ordinate of foci (+ ae, 0) (0, + be)
4. Length of the transverse axes 2a 2b
5. Length of the conjugate axes 2b 2a
6. Equation of directrix x = + a/e y = + b/e
7. Eccentricity e = 2
2
a
b
1 e = 2
2
b
a
1
8. Length of latus rectum
a
b
2 2
b
a
2 2
9. Equation of transverse axes y = 0 x = 0
10. Equation of conjugate axes x = 0 y = 0
Ex.8 Show that the line x cos  + y sin  = p
touches the hyperbola 2
2
a
x
– 2
2
b
y
= 1 if
a2 cos2  – b2 sin2  = p2.
Sol. The given line is
x cos  + y sin  = p
 y sin  = – x cos  + p
y = – x cot  + p cosec 
Comparing this line with y = mx + c
m = – cot , c = p cosec 
Since the given line touches the hyperbola
2
2
a
x
– 2
2
b
y
= 1 then
c2 = a2m2 – b2
p2 cosec2  = a2 cot2 – b2
or p2 = a2 cos2  – b2 sin2 
Ex.9 The line 5x + 12y = 9 touches the hyperbola
x2 – 9y2 = 9 at the point
(A) (– 5,4/3) (B) (5, – 4/3)
(C) (3, – 1/2) (D) None of these
Sol.[B] We have : m = Slope of the tangent = –
12
5
If a line of slope m is tangent to the hyperbola
2
2
a
x
– 2
2
b
y
= 1, then the coordinates of the point
of contact are

l
2
x
x
a
+
l
2
y
y
b
= a2 + b2 = a2 e2.
(ii) The equation of normal at (a sec , b tan )
to the ellipse 2
2
a
x
– 2
2
b
y
= 1 is
ax cos  + by cot  = a2 + b2.
(iii) The equation of the normal to the hyperbola
2
2
a
x
– 2
2
b
y
= 1 in terms of the slope m of the
normal is given by y = mx – 2
2
2
2
2
m
b
a
)
b
a
(
m


Note : In general four normals can be drawn from a
point (x1, y1) to the hyperbola 2
2
a
x
– 2
2
b
y
= 1
8. EQUATION OF PAIR OF TANGENTS
If P (x1, y1) be any point out side the hyperbola
2
2
a
x
– 2
2
b
y
= 1 and a pair of tangents PA, PB can be
drawn to it from P.
then the equation of pair of tangents of PA and PB
is SS1 = T2
where S  2
2
a
x
– 2
2
b
y
– 1 = 0
S1 = 2
2
1
a
x
– 2
2
1
b
y
– 1 = 0
T = 2
1
a
x
x
– 2
1
b
y
y
– 1 = 0












2
2
2
2
2
2
2
2
b
m
a
b
,
b
m
a
m
a
Here, a2 = 9, b2 = 1 and m = – 5/12
So, points of contact are 







3
4
,
5
i.e. 






3
4
,
5 and 






3
4
,
5 .
Out of these two points 






3
4
,
5 lies on the
line 5x + 12y = 9. Hence, 






3
4
,
5 is the
required point.
6. EQUATION OFTANGENT
(i) The equation of tangents of slope m to the
hyperbola 2
2
a
x
– 2
2
b
y
= 1 are
y = mx + 2
2
2
b
m
a  and the co-ordinates
of the point of contacts are












2
2
2
2
2
2
2
2
b
m
a
b
,
b
m
a
m
a
(ii) Equation of tangent to the hyperbola
2
2
a
x
– 2
2
b
y
= 1 at the point (x1, y1) is
2
1
a
xx
– 2
1
b
yy
= 1
(iii) Equation of tangent to the hyperbola
2
2
a
x
– 2
2
b
y
= 1 at the point
(a sec, b tan) is
a
x
sec –
b
y
tan = 1
Note : In general two tangents can be drawn from
an external point (x1, y1) to the hyperbola
and they are y – y1 = m1 (x – x1) and
y – y1 = m2 (x – x1), where m1 and m2 are
roots of (x1
2 – a2) m2 – 2x1y1 + y1
2 + b2 = 0
7. EQUATION OF NORMAL
(i) The equation of normal to the hyperbola
2
2
a
x
– 2
2
b
y
= 1 at (x1, y1) is

9. CHORD OF CONTACT
If PA and PB be the tangents through point
P (x1, y1) to the hyperbola 2
2
a
x
– 2
2
b
y
= 1, then
the equation of the chord of contact AB is
2
1
a
x
x
– 2
1
b
y
y
= 1 or T = 0 (at x1, y1)
10. EQUATION OFACHORDWHOSE MIDDLE POINT
IS GIVEN
Equation of the chord of the hyperbola
2
2
a
x
– 2
2
b
y
= 1, bisected at the given point (x1, y1) is
T = S1.
2
1
a
xx
– 2
1
b
yy
– 1 = 2
2
1
a
x
– 2
2
1
b
y
– 1
11. DIRECTOR CIRCLE
The locus of the intersection of tangents which are
at right angles is known as director circle of the
hyperbola. The equation of the director circle is x2 +
y2 = a2 – b2.
Note: The circle described on transverse axis of the
hyperbola as diameter is called auxiliary circle and so
its equation is x2 + y2 = a2
Examples
based on Director Circle
Ex.10 Find the locus of the mid points of the chords
of the circle x2 + y2 = 16, which are tangent
to the hyperbola 9x2 – 16y2 = 144.
Sol. Any tangent to hyperbola
16
x2
–
9
y2
= 1 is
y = mx + (16m2 – 9) ...(1)
Let (x1,y1) be the mid- point of the chord of
the circle x2 +y2 = 16, then equation of the
chord is (T= S1)
xx1 + yy1 – (x1
2 + y1
2) = 0 ...(2)
Since (1) and (2) are same, comparing,
we get
1
x
m
= –
1
y
1
=
)
y
x
(
9
m
16
2
1
2
1
2



 m = –
1
1
y
x
, ( 2
1
x + 2
1
y )2 = 2
1
y (15m2 – 9)
Eliminating m and generalizing (x1, y1),
required locus is (x2 + y2)2 = 16x2 – 9y2

Ex.1 If e and e be the eccentricities of a hyperbola
and its conjugate then the value of 2
e
1
+ 2
'
e
1
=
(A) 0 (B) 1 (C) 2 (D) 4
Sol.[B] Hyperbola 2
2
a
x
– 2
2
b
y
= 1
b2
= a2
(e2
– 1)
or e2
= 1 + 2
2
a
b
= 2
2
2
a
b
a 
Conjugate hyperbola 2
2
a
x
– 2
2
b
y
= –1
i.e.Transverse axis is along y-axis and conjugate
along x-axis.
a2
= b2
(e'2
– 1)
e'2
= 2
2
2
b
b
a 
 2
e
1
+ 2
'
e
1
= 2
2
2
2
b
a
b
a


= 1
Ex. 2. If the foci of the ellipse
16
x2
+ 2
2
b
y
= 1 and hyperbola
144
x 2
–
81
y2
=
25
1
coincide, then the value of b2
is-
(A) 1 (B) 5 (C) 7 (D) 9
Sol.[C] For hyperbola
144
x 2
–
81
y2
=
25
1
e2
= 1 + 2
2
a
b
= 1 +
144
81
=
144
225
e =
12
15
=
4
5
i.e., e > 1
Hence the foci are
(±ae, 0) 





 0
,
4
5
.
5
12
= (±3, 0)
Now the foci coincide therefore for ellipse
ae = 3 or a2
e2
= 9
or a2








 2
2
a
b
1 = 9
a2
– b2
= 9
or 16 – b2
= 9
 b2
= 7
Ex. 3. The equation of the common tangents to the
parabola y2
= 8x and the hyperbola 3x2
– y2
= 3 is-
(A) 2x± y+1 = 0 (B) x ± y + 1 = 0
(C) x ± 2y+1 = 0 (D) x ± y + 2 = 0
Sol.[A] Parabola y2
= 8x
 4a = 8  a = 2
Any tangent to the parabola is
y = mx +
m
2
...(i)
If it is also tangent to the hyperbola
1
x2
–
3
y2
= 1 i.e. a2
= 1, b2
= 3 then
c2
= a2
m2
– b2

2
m
2






= 1.m2
– 3
or m4
– 3m2
– 4 = 0  (m2
–4) (m2
+ 1) = 0
 m = ±2 puting for m in (i), we get the tangents
as 2x ± y + 1 = 0
Ex. 4. The locus of the point of intersection of the
lines 3 x–y–4 3 k=0 and
3 kx +ky – 4 3 = 0 for different values of k
is-
(A) Ellipse (B) Parabola
(C) Circle (D) Hyperbola
Sol.[D] 3 x – y = 4 3 k ...(i)
K( 3 x + y) = 4 3 ...(ii)
To find the locus of their point of intersection
eliminate the variable K between the equations
from (i) K =
3
4
y
x
3 
and putting in (ii), we get
( 3 x – y) ( 3 x + y) = 3 (4)2
3x2
– y2
= 48
or
16
x2
–
48
y2
= 1
Hence the locus is hyperbola
Ex. 5. The area of a triangle formed by the lines
x – y = 0, x + y = 0 and any tangent to the
hyperbola x2
– y2
= a2
is-
(A) a2
(B) 2a2
(C) 3a2
(D) 4a2
Sol.[A] Any tangent to the hyperbola at
P(a sec , a tan ) is
x sec  – y tan  = a ...(i)
Also x – y = 0 ...(ii)
x + y = 0 ...(iii)
Solving the above three lines in pairs, we get the
point A, B, C as











 tan
sec
a
,
tan
sec
a
,
SOLVED EXAMPLES













 tan
sec
a
,
tan
sec
a
and (0, 0)
Since the one vertex is the origin therefore the
area of the triangle ABC is
2
1
(x1
y2
– x2
y1
)
=
2
a 2














2
2
2
2
tan
sec
1
tan
sec
1
=
2
a 2
(–2) = –a2
= a2
Ex.6. The locus of the mid point ofthe chords of the
circle x2
+ y2
= 16, which are tangent to the
hyperbola 9x2
– 16y2
= 144 is-
(A) x2
+ y2
= a2
– b2
(B) (x2
+ y2
)2
= a2
– b2
(C) (x2
+ y2
)2
= a2
x2
– b2
y2
(D) (x2
+ y2
)2
= a2
+ b2
Sol.[C] Let (h, k) be the mid point of the chord of the
circle x2
+ y2
= a2
, so that its equation by
T = S1
is
hx + ky = h2
+ k2
or y = –
k
h
x +
k
k
h 2
2

i.e.
the form y = mx + c
It will touch the hyperbola if c2
= a2
m2
– b2

2
2
2
k
k
h







 
= a2
2
k
h






 – b2
(h2
+ k2
)2
= a2
h2
– b2
k2
Generalising, the locus of the mid-point
(h, k) is (x2
+ y2
)2
= a2
x2
– b2
y2
Ex.7 The eccentricity of the conic represented by
x2
– y2
– 4x + 4y + 16 = 0 is-
(A) 1 (B) 2 (C) 2 (D)
2
1
Sol.[B] We have x2
– y2
– 4x + 4y + 16 = 0
or (x2
– 4x) – (y2
– 4y) = – 16
or (x2
– 4x + 4) – (y2
– 4y + 4) = – 16
or (x – 2)2
– (y – 2)2
= – 16
or 2
2
4
)
2
x
( 
– 2
2
4
)
2
y
( 
= – 1
Shifting the origin at (2, 2), we obtain
2
2
4
x
– 2
2
4
y
= –1,
where x = X + 2, y = Y + 2
This is rectangular hyperbola, whose eccentricity
is always 2 .
i.e. e2
= 1+ 2
2
a
b
= 1 + 2
2
4
4
; e = 2
Ex.8 The equation 9x2
– 16y2
– 18x + 32y – 151 = 0
represent a hyperbola -
(A) The length of the transverse axes is 4
(B) Length of latus rectum is 9
(C) Equation of directrix is x=
5
21
and x = –
5
11
(D) None of these
Sol.[C] We have 9x2
– 16y2
– 18x + 32y – 151 = 0
9(x2
– 2x) – 16(y2
– 2y) = 151
9(x2
–2x+1) – 16(y2
– 2y + 1) = 144
9(x – 1)2
– 16(y – 1)2
= 144
16
)
1
x
( 2

–
9
)
1
y
( 2

= 1
Shifting the origin at (1, 1) without rotating the
axes
16
x2
–
9
y2
= 1
where x = X + 1 and y = Y + 1
This is of the form 2
2
a
x
– 2
2
b
y
= 1
where a2
= 16 and b2
= 9 so
The length of the transverse axes = 2a = 8
The length of the letus rectum =
a
b
2 2
=
2
a
The equaiton of the directrix x = ±
a
e
x – 1 = ±
5
16
 x = ±
5
16
+ 1
x =
5
21
; x = –
5
11
Ex.9 The equations to the common tangents to the
two hyperbola 2
2
a
x
– 2
2
b
y
= 1 & 2
2
a
y
– 2
2
b
x
= 1 are
(A) y = ± x ± 2
2
a
b 
(B) y = ± x ± 2
2
b
a 
(C) y = ± x ± (a2
– b2
)
(D) y = ± x ± 2
2
b
a 
Sol.[B] Any tangent to the hyperbola 2
2
a
x
– 2
2
b
y
= 1 is
y = mx ± 2
2
2
b
m
a 
or y = mx + c
where c = ± 2
2
2
b
m
a 
This will touch the hyperbola 2
2
a
y
– 2
2
b
x
= 1
if the equation 2
2
a
)
c
mx
( 
– 2
2
b
x
= 1 has equal
roots or x2
(b2
m2
– a2
) + 2b2
mcx + (c2
– a2
)b2
= 0 is an
quadratic equation have equal roots

4b4
m2
c2
= 4(b2
m2
– a2
) (c2
– a2
)b2
c2
= a2
– b2
m2
a2
m2
– b2
= a2
– b2
m2
m2
(a2
+ b2
) = a2
+ b2
 m = ±1
Hence, the equations of the common tangents
are y = ±x ± 2
2
b
a 
Ex.10 For what value of  does the line y = 2x +  touches
the hyperbola 16x2
– 9y2
= 144?
Sol.  Equation of hyperbola is 16x2
– 9y2
= 144
or
9
x2
–
16
y2
= 1 comparing this with
2
2
a
x
– 2
2
b
y
= 1, we get a2
= 9, b2
= 16 and comparing
this line y = 2x +  with m = mx + c ;
m = 2 & c = 
If the line y = 2x +  touches the hyperbola
16x2
– 9y2
= 144
then c2
= a2
m2
– b2
  = 9(2)2
– 16
= 36 – 16 = 20;   = ±25
Ex.11 Find the equation of the tangent to the hyperbola
x2
– 4y2
= 36 which is perpendicular to the line
x – y + 4 = 0.
Sol. Let m be the slope of the tangent since the
tangent is perpendicular to the line
x – y + 4 = 0.
 m x 1 = –1  m = –1
since x2
– 4y2
= 36
or
36
x2
–
9
y2
= 1
Comparing this with 2
2
a
x
+ 2
2
b
y
= 1;
 a2
= 36 & b2
= 9 so the equation of tangents
are
y = (– 1) x ± 9
)
1
(
x
36 2


 y = –x ±27 or x + y ± 33 = 0
EX.12 Find the locus of the point of intersection of
tangents to the hyperbola 4x2
– 9y2
= 36 which
meet at a constant angle /4.
Sol. Let the point of intersection of tangents be
P (x1
,y1
). Then the equation of pair of tangents
from P(x1
,y1
) to the given hyperbola is
(4x2
– 9y2
– 36) (4x1
2
– 9y1
2
– 36)
= [4x1
x – 9y1
y – 36]2
. ...(1)
[SS1
= T2
]
or x2
(y1
2
+4) +2x1
y1
xy+y2
(x1
2
– 9)+..= 0
...(2)
Since angle between the tangent is /4.
tan 




 
4
=
  
 
9
x
4
y
9
x
4
y
y
x
2
2
1
2
1
2
1
2
1
2
1
2
1






Hence locus of P (x1
, y1
) is (x2
+ y2
– 5)2
= 4(9y2
– 4x2
+ 36)
Ex.13 Prove that the locus of the middle points of
chords of hyperbola 2
2
a
x
– 2
2
b
y
=1, passing
through the fixed point (h,k) is a hyperbola whose
centre is the point (
2
1
h,
2
1
k)
Sol. Using T= S1
, equation of chord having (x1
,y1
) as
mid point is 2
1
a
x
x
– 2
1
b
y
y
=
2
2
1
a
x
– 2
2
1
b
y
It passes through (h,k) so 2
1
a
h
x
– 2
1
b
k
y
=
2
2
1
a
x
– 2
2
1
b
y
 Locus of (x, y) is
2
2
a
h
2
1
x 






– 2
2
b
k
2
1
y 






=
4
1








 2
2
2
2
b
k
a
h
which is a hyperbola whose centre is 





k
2
1
,
h
2
1
.
Ex.14 From the points on the circle x2
+ y2
= a2
, tangents
are drawn to the hyperbola
x2
– y2
= a2
; prove that the locus of the middle
points of the chords of contact is the curve (x2
– y2
)2
= a2
(x2
+ y2
).
Sol. Since any point on the circle x2
+ y2
= a2
is
(a cos, a sin) chord of contact of this point
w.r.t hyperbola x2
– y2
= a2
is
x (a cos) – y(a sin) = a2
or x cos – y sin = a ...(1)
If its mid point be ( h,k) , then it is same as
T = S1
i.e. hx – ky– a2
= h2
–k2
– a2
or hx – ky = h2
– k2
...(2)
Comparing (1) & (2) , we get
h
cos 
=
k
sin 
=
)
k
h
(
a
2
2

or (h2
– k2
) cos = ah ...(3)
and (h2
– k2
) sin= ak ...(4)
Squaring and adding (3) and (4), we get
(h2
– k2
)2
= a2
h2
+ a2
k2
 (h2
– k2
)2
= a2
(h2
+ k2
)
Hence the required locus is
(x2
– y2
)2
= a2
(x2
+ y2
)

(6) Hyperbola (Theory).Module-3pdf

More Related Content

(6) Hyperbola (Theory).Module-3pdf