Algebra

Radicals and
Rational
Exponents

19

RADICALS
A perfect square is the square of a natural number. 1, 4,
9, 16, 25, and 36 are the first six perfect squares.

A perfect cube is the cube of a natural number. 1, 8, 27,
64, 125, and 216 are the first six perfect cubes.

Perfect Powers
This idea can be expanded to perfect powers of a variable
for any radicand.
The radicand xn is a perfect power when n is a multiple of
the index of the radicand.

A quick way to determine if a radicand xn is a perfect
power for an index is to determine if the exponent n is
divisible by the index of the radical.

Since the exponent, 20, is divisible by the index, 5,
Example: 5
x 20
x20 is a perfect fifth power.

For all real numbers a and b and all rational numbers m For all real numbers a and b and all rational numbers m
and n, and n,

Product rule: am • an = am + n Zero exponent rule: a0 = 1, a 0

a m
Raising a power to a power:
a   a
m n m n

 a mn , a  0
an
Quotient rule:
Raising a product to a power : abm  a m b m
m
m 1 a am
a  m, a0    m , b0
Negative exponent rule: a Raising a quotient to a power : b b

Rational

Product Rule for Radicals
For nonnegative real numbers a and b,
n
a  n b  n ab

Example 1:
3
32  3 8 3 4  23 4
3
 250  3  125 3 2  53 2
4
48  4
16 4 3  24 3

Quotient Rule for Radicals
For nonnegative real numbers a and b,
n
a n a
n
 , b0
b b

Example 2:
81 81 9
 
100 100 10
75 25
 Simplify radicand, if possible.  25  5
3 1

Quotient Rule for Radicals
Example 3:

64 x 6 3
64 x 6 4x2
3
12
  4
y 3
y12 y

64 x 5 64 x 5 32 x 2 16 x 2 2
 3
   4x 2
2x 3 2x 1 1

3a 6b5 3a 8 4
3a 8 4
a8 4 3 a2 4 3
4
 2 13
4 8
  
16a b 16b 4
16b8 4
16b8 2b 2

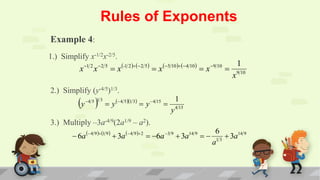
Rules of Exponents
Example 4:
1.) Simplify x-1/2x-2/5.
1 2 2 5 -1 2   2 5  5 10   4 10  9 10 1
x x x x x 
x 9 10
2.) Simplify (y-4/5)1/3.
y  4 5 1 3
 y  4 5 1 3  y  4 15 
1
y 4 15
3.) Multiply –3a-4/9(2a1/9 – a2).
6
 6a 4 9 1 9   3a 4 9  2  6a 3 9  3a14 9    3a14 9
a1 3

Factoring Expressions
Example 5:
1.) Factor x1/4 – x5/4. The smallest of the two exponents is 1/4.
x1/4 – x5/4 = x1/4 (1 – x5/4-(1/4)) = x1/4 (1 – x4/4) = x1/4 (1 – x)
Original Exponent
exponent factored out

2.) Factor x-1/2 + x1/2. The smallest of the two exponents is -1/2.
-1/2 + x1/2 = x -1/2 (1– x1/2-(-1/2) ) = x -1/2 (1– x) =
1 x
x
x1 2
Original Exponent
exponent factored out

SEATWORK HOMEWORK
1 1
2 2
3 3
4 4
5 5

SEATWORK #1 :
Like radicals are radicals having the same
radicands. They are added the same way
like terms are added.

5 2 4 2 9 2
4 4 4

Answer:
54 2  44 2  94 2
3 xyz  10 xyz  5 xyz  8 xyz
2 2 2 2

65 7  75 6 Cannot be simplified further.

SEATWORK #2 : Adding & Subtracting
To Add or Subtract Radicals
1. Simplify each radical expression.
2. Combine like radicals (if there are any).

3 250  5 160 3 y 4 48x5  x4 3x5 y 4

Answer:
3 250  5 160  3  25 10  5  16 10 
3  5 10  5  4 10  15 10  20 10  35 10
3 y 4 48x5  x4 3x5 y 4  3 y 4 16x 4 4 3x  x4 x 4 y 4 4 3x 
3 y  2 x 4 3 x  x  xy 4 3 x  6 xy 4 3 x  x 2 y 4 3x 
 4 3 x (6 xy  x 2 y )

SEATWORK #3 : Multiplying Radicals

Multiply: 3( 5  x )
Answer:
3 5 3 x
(8  5 )( 6  2 )  Use the FOIL method.
48  8 2  6 5  10

( 3  6)( 3  6)   3  6
2
3  6 3  36 
3  36  33 Notice that the inner and outer terms cancel.

SEATWORK #4 : Rationalizing Denominators
To Rationalize a Denominator
Multiply both the numerator and the denominator of the fraction
by a radical that will result in the radicand in the denominator
becoming a perfect power.

x2 5 pq 4
y3 2r

Answer :
x2 x2 y3 x 2 y 3 xy y x y
3
   3
 3
 2
y y 3
y 3 y y y

5 pq 4 5 pq 4 2r 10 pq 4 r 10 pq 4 r q 2 10 pr
    
2r 2r 2r 2r 2r 2r

SEATWORK #5 : Simplifying Radicals
Simplify by rationalizing the denominator:
5 c  2d
2 1 c d

Answer :
5 5 2  1 5( 2  1)
  
2 1 2  1 2 1 2 1

c  2d c  2d c d
  
c d c d c d
( c  2d )( c  d ) c  cd  2cd  d 2

( c  d )( c  d ) cd

HOMEWORK #1 : Simplifying Radicals

1 3 8 100 6
( r  3) 5
Simplify:  2 4
3 3 3 6 3
( r  3)5
Answer:
1 3 1 3 3 3 3 2 3
      
3 3 3 3 3 3 3 3

8 100 8 3 100 6 24 600 4 6 100 6
2 4 2  4   2 4  4 4 
3 6 3 3 6 6 3 6 6 6
2 6 10 6 8 6 40 6 32 6 16 6
4 4    
6 6 6 6 6 3

6
( r  3)5 ( r  3)5 6 ( 5 6 ) ( 5 3 )
  ( r  3)  ( r  3)( 5 6 )(10 6 )  ( r  3) 5 6 
( r  3)5 ( r  3)
53
3
1
(r  3) 5 6

HOMEWORK #2 :

8
2
Evaluating in Either Order
3

 8
Answer:

8  2   4
2 2
 3 2
3

or

8
2
3  8  64  4
3 2 3
16

HOMEWORK #3 :

8
2
Evaluating a-m/n 
3

Answer:

8 1 1 1 1
2

   2 
 8 2 4
3

8
2 2
3
3

HOMEWORK #4 :

y   
1 1 1

Simplify: 6 6  a b ab2 3
 
 
Answer:
y 
1
6 6  6
y6  y
 1 1   1 1  1 1
 a 2b 3

ab  

 a 2b 3
 
 a b
 
   
1 1
1  1
a 2
b 3

3 2
a b 2 3 18

HOMEWORK #5 :
Exponent 1/n When n Is Odd
1
1
 1 
 27
1 5

27 3 3  
 32 

Answer: 1
27 3
 3
27  3

 27 
1
3  3
 27  3
1
 1  5 1 1
   5 
 32  32 2 19

Submitted
by:
John Marion G. De Guzman
2nd year BTTE AUTOMOTIVE
ALGEBRA 111

Algebra

More Related Content

Algebra