Calc 2.6
- 1. 2.6 Related Rates Solving Real-Life Problems
- 2. You pump air at a steady rate into a deflated balloon until the balloon bursts. Does the radius of the balloon change faster when you first start pumping the air, or just before the balloon bursts? Why?
- 3. Ex 1 p. 149 Two rates that are related Suppose x and y are two differentiable functions of t and are related by equation y = x3 – 2 Find dy/dt , given that dx/dt = 2 when x = 1 Implicitly derive x and y with respect to t y = x3 − 2 dy 2 dx = 3x Now substitute in what you know dt dt dy = 3(1) 2 (2) =6 dt In this problem you were given the equation that relates y and x. Most times you have to create the equation that relates variables. Luckily we know geometry and trig!
- 4. Ex 2 p. 150 Ripples in a pond A pebble is dropped in a calm pond, causing ripples in the form of concentric circles. The radius r of the outer ripple is increasing at a constant rate of 1 foot per second. When the radius is 5 feet, at what rate is the total area A of the disturbed water changing? Equation: A = π r 2 r dr Given rate: = 1 ft/sec dt A = π r2 Find dA/dt when r = 5 ft dA dr Substitute what you know! = π (2r ) dA dt dt = 2π (5)(1) = 10π dt dA dr = 2π r When radius is 5 ft, the Area is changing dt dt at rate of 10π ft2/sec
- 5. Ex 3 p. 151 An Inflating Balloon Air is being pumped into a spherical balloon at a rate of 5 cubic feet per minute. Find the rate of change of the radius when the radius is 1.5 feet. Volume is changing with time, and so is the radius Equation: 4 3 V = πr 3 Given dV/dt = 5 ft3/min, Find dr/dt when r = 1.5 ft dV 4 2 dr dV 2 dr = π ÷3r = 4π r dt 3 dt dt dt Now plug in the given info after a cleanup dr dr 5 5 = 4π (1.5) 2 = ft / min ≈ 0.177 ft / min dt dt 9π
- 6. Ex 5 p.152 Changing Angle of Elevation Find the rate of change in the angle of elevation of the camera 10 seconds after lift-off 2 2 hyp = s + 2000 2 Solution: Let θ be angle of elevation. Equations: tan θ = s 2000 s = 50t 2 Given t = 10 so s(10) = 5000 ft ds = 100t = velocity dt dθ 1 ds dθ (100t ) sec 2 θ = = cos 2 θ dt 2000 dt dt 2000 2000 cos θ = 2 s 2 + 20002 dθ 2000 (100t ) 2000 ( 100 ) ( 10 ) 2 = ÷ = = rad/sec dt s 2 + 20002 2000 50002 + 20002 29
- 7. Ex 6 Conical tanks and water going into or out of a tank When water goes into a conical tank, the volume, the radius, and the height of the water are all a function of time. We need to find an equation that relates volume, radius and height, namely π r 2h V= 3 (You might recall that a cylinder with same height and radius is 3 times as much volume as a cone.)
- 8. A conical tank is 8 inches high and 8inches across the top. If water is flowing into the tank at rate of 5 in3/min, find the rate of change of the depth when the water is 6 inches deep. dV = 5in3 / min Given: dt Implicitly derive with respect r 4 to time: π r 2h What you can observe: = V= h 8 3 or r = 1 h dr 1 dh = 2 dt 2 dt dV π 2 dh dr What you are solving for: dh = r + h(2r ) ÷ dt dt 3 dt dt when h = 6 in. Replace all r’s with expressions dV π 2 dh dr = r + 2rh ÷ in h, and all dr/dt’s with dt 3 dt dt expressions in dh/dt Yuck!!!!
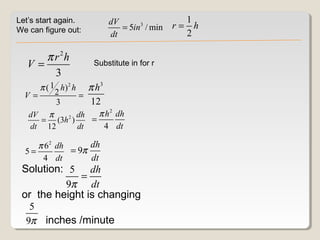
- 9. Let’s start again. dV 1 We can figure out: = 5in3 / min r= h dt 2 π r 2h V= Substitute in for r 3 π ( 1 2 h) 2 h π h3 V= = 3 12 dV π 2 dh π h 2 dh = (3h ) = dt 12 dt 4 dt π 62 dh dh 5= = 9π 4 dt dt Solution: 5 dh = 9π dt or the height is changing 5 9π inches /minute
- 10. •Related rate problems often involve a situation in which you are asked to calculate the rate at which one quantity changes with respect to time from the rate at which a second quantity changes with respect to time. •Related rate problems can be recognized because the rate of change of one or more quantities with respect to time is given and the rate of change with respect to time of another quantity is required. http://www.mathdemos.org/mathdemos/relatedrates/relatedrates.html
- 12. DO NOT MAKE ANY SUBSTITUTIONS before you differentiate! That creates a problem where things can’t change with time. If a rate is decreasing or getting smaller over time, it is a negative rate! Geometry formulas are in the back of your book. 2.6 p. 154 #1-8, 13-27 odd, 35, 36, 45, 46