deformations of smooth functions on 2-torus whose kronrod-reeb graph is a tree

deformations of smooth functions on
2-torus whose kronrod-reeb graph is a tree
Bohdan Feshchenko
June 5, 2015
Topology department, Institute of Mathematics of NAS of Ukraine, Kyiv, Ukraine

introduction
Let M be a smooth compact surface, X ⊂ M be a closed (possibly
empty) subset, and D(M, X) be the group of diffeomorphisms of M
ﬁxed on some neighborhood of X. Then D(M, X) acts from the right
on C∞
(M) by following rule:
γ : C∞
(M) × D(M, X) → C∞
(M), γ(f, h) = f ◦ h. (1)
Given f ∈ C∞
(M) let
S(f, X) = {f ∈ D(M, X) | f ◦ h = f}, O(f, X) = {f ◦ h | h ∈ D(M, X)}
be respectively the stabilizer and the orbit of f under the action (1).
2

introduction
If X is empty, then put D(M) = D(M, ∅), S(f) = S(f, ∅), O(f) = O(f, ∅),
and so on. We will also endow the spaces D(M, X), C∞
(M), S(f, X),
and O(f, X) with the corresponding Whitney C∞
-topologies.
Denote by Sid(f, X) and Did(M, X) the identity path components
S(f, X) and D(M, X) respectively, and Of(f, X) be the path component
of f in O(f, X). Let also
S′
(f, X) = S(f) ∩ Did(M, X).
3

theorem (maksymenko, 2006, 2010, 2012)
Let f ∈ F(M) and X be a finite (possibly empty) union of regular
components of certain level sets of function f. Then the following
statements hold true.
(1) Of(f, X) = Of(f, X ∪ ∂M), and so
πkO(f, X) ∼= πkO(f, X ∪ ∂M), k ≥ 1.
(2) The following map
p : D(M, X) −→ O(f, X), p(h) = f ◦ h.
is a Serre fibration with fiber S(f, X), i.e. it has homotopy lifting
property for CW-complexes. This implies that
4

(a) p(Did(M, X)) = Of(f, X);
(b) the restriction map
p|Did(M,X) : Did(M, X) −→ Of(f, X) (2)
is also a Serre fibration with fiber S′
(f, X);
(c) for each k ≥ 0 we have an isomorphism
λk : πk
(
D(M, X), S(f, X)
)
−→ πkO(f, X) defined by λk[ω] = [f ◦ ω]
for a continuous map ω : (Ik
, ∂Ik
, 0) →
(
D(M), S(f), idM
)
, and
making commutative the following diagram
· · · // πkD(M, X)
q
//
p
((QQQQQQQQQQQQQ
πk
(
D(M, X), S(f, X)
)
λk
∼=

∂ // πk−1S(f, X) // · ·
πkO(f, X),
∂◦λ−1
k
66mmmmmmmmmmmmm
5

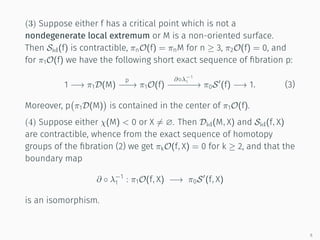
(3) Suppose either f has a critical point which is not a
nondegenerate local extremum or M is a non-oriented surface.
Then Sid(f) is contractible, πnO(f) = πnM for n ≥ 3, π2O(f) = 0, and
for π1O(f) we have the following short exact sequence of ﬁbration p:
1 −→ π1D(M)
p
−−−→ π1O(f)
∂◦λ−1
1
−−−−−−→ π0S′
(f) −→ 1. (3)
Moreover, p
(
π1D(M)
)
is contained in the center of π1O(f).
(4) Suppose either χ(M) 0 or X ̸= ∅. Then Did(M, X) and Sid(f, X)
are contractible, whence from the exact sequence of homotopy
groups of the ﬁbration (2) we get πkO(f, X) = 0 for k ≥ 2, and that the
boundary map
∂ ◦ λ−1
1 : π1O(f, X) −→ π0S′
(f, X)
is an isomorphism.
6

publications
(with Maksymenko), Homotopy properties of spaces of smooth
functions on 2–torus, Ukrainian Mathematical Journal, vol. 66,
no. 9 (2014) 1205-1212 arXiv:1401.2296
(with Maksymenko) Orbits of smooth functions on 2–torus and
their homotopy types, arXiv:1409.0502 (submited to Mat. Studii),
2014.
(with Maksymenko), Functions on 2-torus whose Kronrod-Reeb
graph contains a cycle, Methods of Functional Analysis and
Topology, no. 1 (2015) 22-40, arXiv:1411.6863.
7

Let B be a set, S be a group, and H be a subgroup of the group of
permutations Σ(B) of B. The unit of H we denote by e.
Denote by Map(B, S) the group of all maps B → S, with respect to
the point-wise multiplication. Then H acts on Map(B, S) by the
following rule:
α · h = α ◦ h : B
h
−→ B
α
−→ S,
α ∈ Map(B, S) and h ∈ H.
8

The wreath product of S and H over B is a semi-direct product
S ≀
B
H := Map(B, S) ⋊ H,
i.e. it is the Cartesian product Map(B, S) × H with respect to the
multiplication, which is given by the following formula:
(α1, h1) · (α2, h2) = ((α1 ◦ h2)α2, h1h2),
for (α1, h1), (α2, h2) ∈ Map(B, S) × H.
9

Obviously, S ≀
B
H is a group: the pair (ε, idB) is the unit of S ≀
B
H, where
ε : B → S is the constant map to the unit of S, and (α ◦ h−1
, h−1
) is
the inverse for (α, h), where α ∈ Map(B, S) is a point-wise inverse of
α. Then the following exact sequence holds
1 // Map(B, S)
j
// S ≀
B
H
p
// H // 1,
where j(α) = (α, e) and p(α, h) = h. The map p, by deﬁnition, admits
a section s : H → S ≀
B
H, s(h) = (ε, h).
10

theorem
Let f ∈ F(T2
) be such that Γ(f) is a tree. Then there is an
isomorphism
ξ : π1Of(f) ∼=
∏
i
π0S′
(f|Di
, ∂Di) ≀
Zn×Zm
Z2
,
for certain 2-disks {Di}i∈I, and n, m ∈ Z+.
12

deformations of smooth functions on 2-torus whose kronrod-reeb graph is a tree

More Related Content

deformations of smooth functions on 2-torus whose kronrod-reeb graph is a tree