Lesson 9: The Product and Quotient Rules (Section 41 handout)
- 1. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Notes Section 2.4 The Product and Quotient Rules V63.0121.041, Calculus I New York University October 3, 2010 Announcements Quiz 2 next week on §§1.5, 1.6, 2.1, 2.2 Midterm in class (covers all sections up to 2.5) Announcements Notes Quiz 2 next week on §§1.5, 1.6, 2.1, 2.2 Midterm in class (covers all sections up to 2.5) V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 2 / 40 Help! Notes Free resources: Math Tutoring Center (CIWW 524) College Learning Center (schedule on Blackboard) TAs’ office hours my office hours each other! V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 3 / 40 1
- 2. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Objectives Notes Understand and be able to use the Product Rule for the derivative of the product of two functions. Understand and be able to use the Quotient Rule for the derivative of the quotient of two functions. V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 4 / 40 Outline Notes Derivative of a Product Derivation Examples The Quotient Rule Derivation Examples More derivatives of trigonometric functions Derivative of Tangent and Cotangent Derivative of Secant and Cosecant More on the Power Rule Power Rule for Negative Integers V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 5 / 40 Recollection and extension Notes We have shown that if u and v are functions, that (u + v ) = u + v (u − v ) = u − v What about uv ? V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 6 / 40 2
- 3. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Is the derivative of a product the product of the derivatives? Notes (uv ) = u v ! Try this with u = x and v = x 2 . Then uv = x 3 =⇒ (uv ) = 3x 2 . But u v = 1 · 2x = 2x. So we have to be more careful. V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 7 / 40 Mmm...burgers Notes Say you work in a fast-food joint. You want to make more money. What are your choices? Work longer hours. Get a raise. Say you get a 25 cent raise in your hourly wages and work 5 hours more per week. How much extra money do you make? V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 8 / 40 Money money money money Notes The answer depends on how much you work already and your current wage. Suppose you work h hours and are paid w . You get a time increase of ∆h and a wage increase of ∆w . Income is wages times hours, so ∆I = (w + ∆w )(h + ∆h) − wh FOIL = w · h + w · ∆h + ∆w · h + ∆w · ∆h − wh = w · ∆h + ∆w · h + ∆w · ∆h V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 9 / 40 3
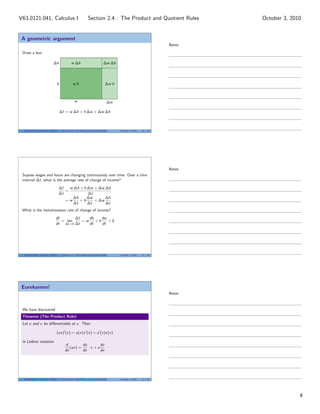
- 4. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 A geometric argument Notes Draw a box: ∆h w ∆h ∆w ∆h h wh ∆w h w ∆w ∆I = w ∆h + h ∆w + ∆w ∆h V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 10 / 40 Notes Supose wages and hours are changing continuously over time. Over a time interval ∆t, what is the average rate of change of income? ∆I w ∆h + h ∆w + ∆w ∆h = ∆t ∆t ∆h ∆w ∆h =w +h + ∆w ∆t ∆t ∆t What is the instantaneous rate of change of income? dI ∆I dh dw = lim =w +h +0 dt ∆t→0 ∆t dt dt V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 11 / 40 Eurekamen! Notes We have discovered Theorem (The Product Rule) Let u and v be differentiable at x. Then (uv ) (x) = u(x)v (x) + u (x)v (x) in Leibniz notation d du dv (uv ) = ·v +u dx dx dx V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 12 / 40 4
- 5. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Sanity Check Notes Example Apply the product rule to u = x and v = x 2 . Solution (uv ) (x) = u(x)v (x) + u (x)v (x) = x · (2x) + 1 · x 2 = 3x 2 This is what we get the “normal” way. V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 13 / 40 Which is better? Notes Example Find this derivative two ways: first by direct multiplication and then by the product rule: d (3 − x 2 )(x 3 − x + 1) dx Solution by the product rule: dy d d 3 = (3 − x 2 ) (x 3 − x + 1) + (3 − x 2 ) (x − x + 1) dx dx dx = (−2x)(x 3 − x + 1) + (3 − x 2 )(3x 2 − 1) = −5x 4 + 12x 2 − 2x − 3 V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 14 / 40 One more Notes Example d Find x sin x. dx Solution d d d x sin x = x sin x + x sin x dx dx dx = 1 · sin x + x · cos x = sin x + x cos x V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 15 / 40 5
- 6. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Mnemonic Notes Let u = “hi” and v = “ho”. Then (uv ) = vu + uv = “ho dee hi plus hi dee ho” V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 16 / 40 Iterating the Product Rule Notes Example Use the product rule to find the derivative of a three-fold product uvw . Apply the product rule Apply the product rule Solution to uv and w to u and v (uvw ) = ((uv )w ) = (uv ) w + (uv )w = (u v + uv )w + (uv )w = u vw + uv w + uvw So we write down the product three times, taking the derivative of each factor once. V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 18 / 40 Outline Notes Derivative of a Product Derivation Examples The Quotient Rule Derivation Examples More derivatives of trigonometric functions Derivative of Tangent and Cotangent Derivative of Secant and Cosecant More on the Power Rule Power Rule for Negative Integers V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 19 / 40 6
- 7. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 The Quotient Rule Notes What about the derivative of a quotient? u Let u and v be differentiable functions and let Q = . Then v u = Qv If Q is differentiable, we have u = (Qv ) = Q v + Qv u − Qv u u v =⇒ Q = = − · v v v v u u v − uv =⇒ Q = = v v2 This is called the Quotient Rule. V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 20 / 40 The Quotient Rule Notes We have discovered Theorem (The Quotient Rule) u Let u and v be differentiable at x, and v (x) = 0. Then is differentiable v at x, and u u (x)v (x) − u(x)v (x) (x) = v v (x)2 V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 21 / 40 Verifying Example Notes Example d x2 Verify the quotient rule by computing and comparing it to dx x d (x). dx Solution d d d x2 x dx x 2 − x 2 dx (x) = dx x x2 x · 2x − x 2 · 1 = x2 x2 d = 2 =1= (x) x dx V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 22 / 40 7
- 8. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Mnemonic Notes Let u = “hi” and v = “lo”. Then u vu − uv = = “lo dee hi minus hi dee lo over lo lo” v v2 V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 23 / 40 Examples Notes Example Answers d 2x + 5 1. dx 3x − 2 d sin x 2. dx x 2 d 1 3. dt t 2 + t + 2 V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 24 / 40 Solution to first example Notes Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 25 / 40 8
- 9. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Solution to second example Notes Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 27 / 40 Another way to do it Notes Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 28 / 40 Solution to third example Notes Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 30 / 40 9
- 10. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Outline Notes Derivative of a Product Derivation Examples The Quotient Rule Derivation Examples More derivatives of trigonometric functions Derivative of Tangent and Cotangent Derivative of Secant and Cosecant More on the Power Rule Power Rule for Negative Integers V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 32 / 40 Derivative of Tangent Notes Example d Find tan x dx Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 33 / 40 Derivative of Cotangent Notes Example d Find cot x dx Answer d 1 cot x = − 2 = − csc2 x dx sin x Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 34 / 40 10
- 11. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Derivative of Secant Notes Example d Find sec x dx Solution d d 1 cos x · 0 − 1 · (− sin x) sec x = = dx dx cos x cos2 x sin x 1 sin x = = · = sec x tan x cos2 x cos x cos x V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 35 / 40 Derivative of Cosecant Notes Example d Find csc x dx Answer d csc x = − csc x cot x dx Solution V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 36 / 40 Recap: Derivatives of trigonometric functions Notes y y sin x cos x Functions come in pairs cos x − sin x (sin/cos, tan/cot, sec/csc) tan x sec2 x Derivatives of pairs follow similar patterns, with cot x − csc2 x functions and co-functions sec x sec x tan x switched and an extra sign. csc x − csc x cot x V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 37 / 40 11
- 12. V63.0121.041, Calculus I Section 2.4 : The Product and Quotient Rules October 3, 2010 Outline Notes Derivative of a Product Derivation Examples The Quotient Rule Derivation Examples More derivatives of trigonometric functions Derivative of Tangent and Cotangent Derivative of Secant and Cosecant More on the Power Rule Power Rule for Negative Integers V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 38 / 40 Power Rule for Negative Integers Notes Use the quotient rule to prove Theorem d −n x = (−n)x −n−1 dx for positive integers n. Proof. d −n d 1 x = dx dx x n d d x n · dx 1 − 1 · dx x n = x 2n 0 − nx n−1 = = −nx −n−1 x 2n V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 39 / 40 Summary Notes The Product Rule: (uv ) = u v + uv u vu − uv The Quotient Rule: = v v2 Derivatives of tangent/cotangent, secant/cosecant d d tan x = sec2 x sec x = sec x tan x dx dx d d cot x = − csc2 x csc x = − csc x cot x dx dx The Power Rule is true for all whole number powers, including negative powers: d n x = nx n−1 dx V63.0121.041, Calculus I (NYU) Section 2.4 The Product and Quotient Rules October 3, 2010 40 / 40 12