Maths group ppt
- 1. Subject:- BUSINESS MATHEMATICS GROUP PRESENTATION – 02 B.Com(Hons.) IVth sem SUBMITTED TO: SUBMITTED BY: Mr. SUNIL BHARDWAJ SIR K.Devika Jaishree chauhan
- 2. PARTIAL DIFFERENTIATION • When we write u = f(x , y), we are saying that we have a function, u, which depends on two independent variables: x and y. We can consider the change in u with respect to either of these two independent variables by using the partial derivative.
- 3. The partial derivative of u with respect to x is written as: the partial of u with respect to y. It is written as: * The rule for partial derivatives is that we differentiate with respect to one variable while keeping all the other variables constant. = f(x , y) = f(x , y)
- 4. Taking the Partial Derivative of a Partial Derivative • So far we have defined and given examples for first-order partial derivatives. Second-order partial derivatives are simply the partial derivative of a first-order partial derivative. We can have four second-order partial derivatives:
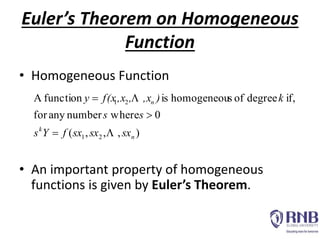
- 7. • Homogeneous Function • An important property of homogeneous functions is given by Euler’s Theorem. ),,,( 0wherenumberanyfor if,degreeofshomogeneouisfunctionA 21 21 n k n sxsxsxfYs ss k),x,,xf(xy Euler’s Theorem on Homogeneous Function
- 8. If z = F (x,y) be a homogenious function of x,y of degree n then x + y = nz for all x,y z z x y Proof: We have z is a homogenious function of degree n. 1 2 1 2 ( ) '( ) ( ) '( ) n n n n z y y y nx f x f x x x x y y nx f yx f x x so that z = xn y x f EXAMPLE
- 9. 11 , '( ) '( )n nz y y Similarly x f x f y x x x 1 1 Thus ,we have x + y = ( ) '( ) '( )n n nz z y y y nx f yx f yx f x y x x x x + y = ( ) =nz hence the result. nz z ynx f xx y
- 10. Maxima and Minima of Functions of Two Variables Let f be a function with two variables with continuous second order partial derivatives fxx, fyy and fxy at a critical point (a,b). Let D = fxx(a,b) fyy(a,b) - fxy 2(a,b) • If D > 0 and fxx(a,b) > 0, then f has a relative minimum at (a,b). • If D > 0 and fxx(a,b) < 0, then f has a relative maximum at (a,b). • If D < 0, then f has a saddle point at (a,b). • If D = 0, then no conclusion can be drawn.