Rational numbers
- 1. RATIONAL NUMBERS By: Ms. Prerna Sharma
- 2. A rational number is a number that can be represented in the form p/q where p and q are integers and q is not equal to zero. So, a rational number can be: p q Rational Numbers……
- 3. Real numbers (R) include all the rational numbers (Q). Real numbers include the integers (Z). Integers involves the natural numbers(N). Every whole number is a rational number because every whole number can be expressed as a fraction. Types of Numbers……
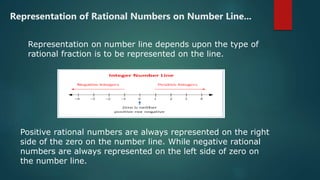
- 4. Representation of Rational Numbers on Number Line... Representation on number line depends upon the type of rational fraction is to be represented on the line. Positive rational numbers are always represented on the right side of the zero on the number line. While negative rational numbers are always represented on the left side of zero on the number line.
- 5. Representation of Rational Numbers on Number Line... Below are some of the types of rational numbers and ways to represent them on the number line: Proper fractions are those in which numerator is less than the denominator. Such fractions exist between only zero and on. Proper fractions are less than one and greater than zero. So, proper fractions always exist between zero and one on number line. Improper fractions are those in which numerator of the fraction will be greater than its denominator. Since, the numerator is greater than the denominator the number will be greater than one. To, represent such rational fractions on the number line first we convert the improper fraction into the mixed fraction so as to know between which integers will the fraction lie.
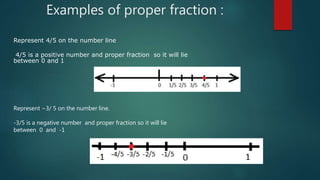
- 6. Examples of proper fraction : Represent 4/5 on the number line 4/5 is a positive number and proper fraction so it will lie between 0 and 1 Represent −3/ 5 on the number line. -3/5 is a negative number and proper fraction so it will lie between 0 and -1
- 7. Examples of Improper fraction : Represent 9/5 on the number line. 9/5 = 1 + 4/5 , so it will lie between 1 and 2 Represent −4/3 on the number line. -4/3 = (-)1 +1/3 so it will lie between -1 and -2
- 8. Arithmetic Operations on Rational Numbers Addition We add p/q and s/t, we need to make the denominator same. we get (pt+qs)/qt. Example: 1/2 + 3/4 = (2+3)/4 = 5/4 Subtraction If we subtract p/q and s/t, then also, we need to make the denominator same, first, and then do the subtraction. Example: 1/2 – 3/4 = (2-3)/4 = -1/4 Multiplication If p/q is multiplied by s/t, then we get (p × s)/(q x t). Example: 1/2 × 3/4 = (1×3)/(2×4) = 3/8 Division If p/q is divided by s/t, then it is represented as: (p/q)÷(s/t) = pt/qs Example: 1/2 ÷ 3/4 =(1×4)/(2×3) = 4/6 = 2/3
- 9. Properties of Rational Numbers Closure Property Commutativity Property Associative Property Distributive Property Additive Property Additive Inverse Multiplicative Identity Multiplicative Inverse or Reciprocal Multiplication by 0
- 10. Closure Property The sum of any two rational numbers is always a rational number. This is called ‘Closure property of addition’ of rational numbers. Thus, Q is closed under addition If a/b and c/d are any two rational numbers, then (a/b) + (c/d) is also a rational number. Addition The difference between any two rational numbers is always a rational number. Hence Q is closed under subtraction. If a/b and c/d are any two rational numbers, then (a/b) - (c/d) is also a rational number. Subtraction The product of two rational numbers is always a rational number. Hence Q is closed under multiplication. If a/b and c/d are any two rational numbers, then (a/b)x (c/d) = ac/bd is also a rational number. Multiplication The collection of non-zero rational numbers is closed under division. If a/b and c/d are two rational numbers, such that c/d ≠ 0, then a/b ÷ c/d is always a rational number. Division
- 11. Closure Property Examples Addition (7/6)+(2/5) = 47/30 Subtraction (5/6) – (1/3) = ½ Multiplication (2/5) * (3/7) = 6/35 Key Points Rational numbers are closed under addition, subtraction and multiplication. Division is not under closure property The division is not under closure property because division by zero is not defined. We can also say that except ‘0’ all numbers are closed under division.
- 12. Commutativity Property Addition 1.Addition of two rational numbers is commutative. 2.If a/b and c/d are any two rational numbers, 3.then 4.(a/b)+(c/d) 5. = (c/d) + (a/b) Subtraction Subtraction of two rational numbers is not commutative. If a/b and c/d are any two rational numbers, then (a/b) - (c/d) ≠ (c/d) - (a/b) Multiplication Multiplication of rational numbers is commutative. If a/b and c/d are any two rational numbers, then (a/b)x (c/d) = (c/d)x(a/b) Division Division of rational numbers is not commutative. If a/b and c/d are two rational numbers, then a/b ÷ c/d ≠ c/d ÷ a/b
- 13. Commutativity Property Examples Addition 2/9 + 4/9 = 6/9 = 2/3 4/9 + 2/9 = 6/9 = 2/3 Hence, 2/9 + 4/9 = 4/9 + 2/9 Subtraction 5/9 - 2/9 = 3/9 = 1/3 2/9 - 5/9 = -3/9 = -1/3 Hence, 5/9 - 2/9 ≠ 2/9 - 5/9 Therefore, Commutative property is not true for division. Multiplication 5/9 x 2/9 = 10/81 2/9 x 5/9 = 10/81 Hence, 5/9 x 2/9 = 2/9 x 5/9 Division 2/3 ÷ 1/3 = 2/3 x 3/1 = 2 1/3 ÷ 2/3 = 1/3 x 3/2 = 1/2 Hence, 2/3 ÷ 1/3 ≠ 1/3 ÷ 2/3 Therefore, Commutative property is not true for division.
- 14. Associative Property Addition • Addition of rational numbers is associative. • If a/b, c/d and e/f are any three rational numbers, then • a/b + (c/d + e/f) = (a/b + c/d) + e/f Subtraction • Subtraction of rational numbers is not associative. • If a/b, c/d and e/f are any three rational numbers, then • a/b - (c/d -e/f) ≠ (a/b - c/d) - e/f Multiplication • Multiplication of rational numbers is associative. • If a/b, c/d and e/f are any three rational numbers, then • a/b x (c/d x e/f) = (a/b x c/d) x e/f Division • Division of rational numbers is not associative. • If a/b, c/d and e/f are any three rational numbers, then • a/b ÷ (c/d ÷ e/f) ≠ (a/b ÷ c/d) ÷ e/f
- 15. Examples Addition 2/9 + (4/9 + 1/9) = 2/9 + 5/9 = 7/9 (2/9 + 4/9) + 1/9 = 6/9 + 1/9 = 7/9 Hence, 2/9 + (4/9 + 1/9) = (2/9 + 4/9) + 1/9 x+(y + z ) = (x + y)+z Subtraction 2/9 - (4/9 - 1/9) = 2/9 - 3/9 = -1/9 (2/9 - 4/9) - 1/9 = -2/9 - 1/9 = -3/9 Hence, 2/9 - (4/9 - 1/9) ≠ (2/9 - 4/9) - 1/9 Therefore, Associative property is not true for subtraction. Multiplication 2/9 x (4/9 x 1/9) = 2/9 x 4/81 = 8/729 (2/9 x 4/9) x 1/9 = 8/81 x 1/9 = 8/729 Hence, 2/9 x (4/9 x 1/9) = (2/9 x 4/9) x 1/9 Therefore, Associative property is true for multiplication. x(y z) = (x y)z Division 2/9 ÷ (4/9 ÷ 1/9) = 2/9 ÷ 4 = 1/18 (2/9 ÷ 4/9) ÷ 1/9 = 1/2 - 1/9 = 7/18 Hence, 2/9 ÷ (4/9 ÷ 1/9) ≠ (2/9 ÷ 4/9) ÷ 1/9 Therefore, Associative property is not true for division. Associative Property
- 16. Distributive Property Distributive Property of Multiplication over Addition • Multiplication of rational numbers is distributive over addition. • If a/b, c/d and e/f are any three rational numbers, then • a/b x (c/d + e/f) = a/b x c/d + a/b x e/f Distributive Property of Multiplication over Subtraction • Multiplication of rational numbers is distributive over subtraction. • If a/b, c/d and e/f are any three rational numbers, then • a/b x (c/d - e/f) = a/b x c/d - a/b x e/f
- 17. Examples Multiplication is distributive over addition. 1/3 x (2/5 + 1/5) = 1/3 x 3/5 = 1/5 1/3 x (2/5 + 1/5) = 1/3 x 2/5 + 1/3 x 1/5 = (2 + 1) / 15 = 1/5 Hence, 1/3 x (2/5 + 1/5) = 1/3 x 2/5 + 1/3 x 1/5 Therefore, Multiplication is distributive over addition. Multiplication is distributive over subtraction. 1/3 x (2/5 - 1/5) = 1/3 x 1/5 = 1/15 1/3 x (2/5 - 1/5) = 1/3 x 2/5 - 1/3 x 1/5 = (2 - 1) / 15 = 1/15 Hence, 1/3 x (2/5 - 1/5) = 1/3 x 2/5 - 1/3 x 1/5 Therefore, Multiplication is distributive over subtraction. Distributive Property….
- 18. Additive inverse (- a/b) is the negative or additive inverse of (a/b) If a/b is a rational number , then there exists a rational number (-a/b) such that a/b + (-a/b) = (-a/b) + a/b = 0
- 19. Examples Additive inverse of 3/5 is (-3/5) Additive inverse of (-3/5) is 3/5 Additive inverse of 0 is 0 itself.
- 20. Additive Identity The sum of any rational number and zero is the rational number itself. • If a/b is any rational number, then a/b + 0 = 0 + a/b = a/b Zero is the additive identity for rational numbers. Example : 2/7 + 0 = 0 + 2/7 = 2/7
- 21. Example : 2/7 + 0 = 0 + 2/7 = 2/7
- 22. Multiplicative Identity The product of any rational number and 1 is the rational number itself. ‘One’ is the multiplicative identity for rational numbers. If a/b is any rational number, then a/b x 1 = 1 x a/b = a/b
- 23. Example 5/7 x 1 = 1x 5/7 = 5/7
- 24. Multiplication By 0 Every rational number multiplied with 0 gives 0. If a/b is any rational number, then a/b x 0 = 0 x a/b = 0
- 25. Example 5/7 x 0 = 0x 5/7 = 0
- 26. Multiplicative Inverse Or Reciprocal For every rational number a/b, a≠0, there exists a rational number c/d such that a/b x c/d = 1. Then c/d is the multiplicative inverse of a/b. If b/a is a rational number, then a/b is the multiplicative inverse or reciprocal of it.
- 27. Example : The reciprocal of 2/3 is 3/2 The reciprocal of 1/3 is 3 The reciprocal of 3 is 1/3 The reciprocal of 1 is 1 The reciprocal of 0 is undefined.
- 28. Key Notes- The result of two rational is always a rational number is we multiply, add or subtract them. A rational number remains the same is we divide or multiply both numerator and denominator with the same number. Sum of zero and a rational number revert the same number itself. 0 is an additive identity and 1 is a multiplicative identity for rational numbers. For a rational number x/y, the additive inverse is -x/y and y/x is the multiplicative inverse.
- 29. Thank you