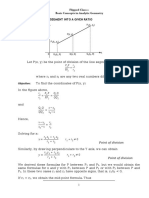
1 Functions, Limits and Continuity
Uploaded by
Reign dropping1 Functions, Limits and Continuity
Uploaded by
Reign droppingDIFFERENTIAL CALCULUS (MATH 111)
VII.3. Rectilinear motion
DIFFERENTIAL CALCULUS VII.4. Arc length
VIII.Differentiation of trigonometric functions
COURSE DESCRIPTION IX. Differentiation of Inverse trigonometric functions
The course provides topics in functions, limits, and continuity, the derivative and X. Differentiation of logarithmic and exponential functions
differentiation, the basic theorems of calculus and their applications, derivatives from XI. Differentiation of hyperbolic and inverse hyperbolic functions
parametric equations and partial derivatives. XII. Partial differentiation
This is a first course in calculus that contains topics that are important in the field of XIII. Indeterminate forms and L’hospital’s rules, Rolle’s theorem and Mean value theorem
mathematical analysis and a powerful tool in the applied fields. It contains subjects involving
higher mathematics, and therefore implies that the students taking has a working familiarity Textbooks and References
with the basic concepts acquired in Algebra, Geometry, Trigonometry, and Analytic 1. Differential & Integral Calculus. Feliciano and Uy. Merriam Webster Bookstore, Inc.,
Geometry. 1983.
2. Leithold, Louis, The calculus, with Analytic Geometry. 6 th edition. Harper and Row
Course Contents: publisher, New York,1992.
I. Variables, Functions, Continuity and Limits 3. Rainville, Earl D. & Clyde E. Love. Differential and integral Calculus. McGraw-Hill
I.1. Functions: Domains and Range Publishing Co.,Inc.,1981.
I.2. Theorems on limits and functions
I.3. One-sided limits
I.4. Limits and infinity
I.5. Infinite limits
I.6. Continuity of functions/limits
II. Differentiation and Applications
II.1. Functions as a rate of change
II.1.a.Increments of a function
II.1.b. Ratio of increments
III. .
III.1. Rules for differentiating algebraic functions
III.2. Chain rule
IV. Applications of derivatives
IV.1. Tangent lines to plane curves and their slopes
IV.2. Derivative as a rate of change
V. Implicit and Parametric differentiation
VI. Higher order derivatives and their applications
VI.1. Increasing and Decreasing functions
VI.2. Concavity and point of inflection
VII. Applications on:
VII.1. Maxima-Minima
VII.2. Time rates
ENGR. IAN C. PAGULAYAN
1
DIFFERENTIAL CALCULUS (MATH 111)
Introduction Two types of Variables
Definition of terms 1. Independent Variable – is one who can stand alone without depending on any other
1. Constants – a quantity that has a fixed value in the problem functions
Example. Integers – 1,2,3,…
- π (3.1415 ) , g ( 9.81 ) , e(2.718) 2. Dependent Variable – is one who depends on another function for its value
2. Variable – a quantity whose value may change in the problem Example: Area of a circle
Ac =π r 2
3. Functions A = _________________
π = _________________
- Set of ordered pairs on numbers (x,y) in which no two distinct ordered pairs r = _________________
have the same first number. The set of all possible values of x is called the
domain of the function, and the collection of resulting values of y is called The function concept may be extended to relations between more than two variables.
the range of the function. The numbers x and y variables. A function like |x| z = f(x,y)
is said to be defined in pieces. Example:
- maybe single-valued or multi-valued depending on how many variables will 1. The volume of a cylinder
have for a given value of the independent variables. The first variable is said 2. Evaluate the values of the functions given
to be the function of the second variable when the first depends on the a. f ( x )=x 3 +2 x−1
value on the second variable. Required: a) f(-1)
General notation b) f(2)
If y and x are variables 2
b. f ( y ) = y + 5 y −4
Then, y=f(x) Required: a) f(t+1)
- A function determines a correspondence between the domain and the 3. The area of a rectangle is six in2. Express the perimeter P of the rectangle as a
range. For each number x in the domain, there corresponds one and only function of the length x of one side.
one number in y in the range.
Seatwork:
Example: Identify whether the equation is a function and give the domain or if 1. If f ( x )=x 2−4 x , find ( a ) f (−5 ) , ( b ) f ( y 2+ 1 ) , ( c ) f ( x+ Δ x ) , ( d ) f ( x +1 )−f ( x −1 )
not give the reason.
1. 2. If y=tan ( x−π ), find x as a function of y
x 2+ 3
2. 3. If y= , find x as a function of y
y
4. A right circular cylinder, a radius of base x, height y, in inscribed in a right
circular cone, radius of base r and height h. Express y as a function of x (r and
h are constants).
ENGR. IAN C. PAGULAYAN
2
DIFFERENTIAL CALCULUS (MATH 111)
OPERATIONS ON FUNCTIONS
Given two functions f and g:
i. Their sum, denoted by f+g, is the function defined by Table 1
( f + g )( x )=f ( x )+ g ( x)
ii. Their difference, denoted by f-g, is the function defined by
( f −g ) ( x )=f ( x )−g(x )
iii. Their product, denoted by f ∙ g, is the function defined by
( f ∙ g ) ( x ) =f ( x ) ∙ g(x)
iv. Their difference, denoted by f/g, is the function defined by
( fg ) ( x )= gf (x)
(x)
where g(x) ≠ 0 Table 2
The table 1 shows that when x is near 3, whether a little less or little greater than 3,
COMPOSITE FUNCTION
Given two functions f and g, the composite function, denoted by f ° g and read as f of g, is f ( x )=x 2 is nearer 9. In other words, “x2 approaches the number 9 as a limit when x
defined by ( f ° g ) ( x ) =f (g ( x)) and the domain of f ° g is the set of all numbers x in the approaches 3”. The abbreviated symbolic form for this statement is
domain of g such that g(c) is in the domain of f. x 2 → 9 as x →3
The composite function is associative. We may also say that “the limit of x2 as x approaches 3 is 9.” In symbol, we write this as
lim x 2=9
x →3
Objectives:
Use the definition of limit to estimate the limits.
From the discussion above, we may formulate the following definition of limit of a function.
Determine whether limits of function exist.
Use properties of limits and direct substitution to evaluate limits. Definition
Concept:
Let f(x) be any function and let a and L be numbers. If we can make f(x) as close to L
The notion of a limit is a fundamental concept of calculus.
as we please by choosing x sufficiently close to a then we say that the limit of f(x) as
In this chapter, you will learn how to evaluate limits and how to use them in the two basic x approaches a is L or symbolically
problems of calculus: The Tangent line and the area problem lim f ( x )=L
x→ a
LIMITS (Lim) – Is a variable to which a function approaches as close to it as possible.
Theorems of Limits
Consider a function f(x) = x2
1. Limit of a constant function
lim c=c c = any constant
The function is well-defined for all real values of x x→ a
The table shows some of the values:
ENGR. IAN C. PAGULAYAN
3
DIFFERENTIAL CALCULUS (MATH 111)
3 2
2. Limit of the identity function 1. lim (5 x −2 x +1)
lim x =a x →1
x→ a
3. Limit of scalar multiple 3 3 2
2. lim (t + 1) √ t +11
lim cf (x )=¿ c lim f (x) ¿ t→4
x→ a x →a
4. Limit of algebraic sum or difference
z 2−2 z+1
lim [f ( x ) ± g(x )]=¿ lim f ( x ) ± lim g(x )¿ 3. lim
x→ a x→ a x→ a z→1 z 2 +2
5. Limit of product Seatwork:
lim [f ( x )∗g( x )]=¿ lim f ( x )∗¿ lim g ( x)¿ ¿ x 2−4 x+ 4
x→ a x →a x →a 1. lim
6. Limit of quotient x →2 x−2
lim f ( x )
f (x) y 3− y 2− y −2
lim
[ ]
x→ a g ( x )
= x→ a
lim g(x )
x →a
2. lim
x →1 2 y 3−5 y 2 +5 y−6
Where: lim
x→ a
g ( x ) ≠0
Limits that fail to exist
Show that the limit does not exist
7. Limit of a radical function |x|
n n lim
lim [ √ f ( x )]=¿ lim f ( x ) ¿
√ n = any positive integer and f (x) ≥ 0 if n is even x →0 x
x→ a x→ a
This means that no matter how close x gets to 0, there will be both positive and negative x-
8. Limit of a power function
values that yield f (x) = 1 and f (x) = –1.
lim ¿ ¿
x→ a This implies that the limit does not exist.
We assume that f(x) and g(x) are defined for all values in some interval containing “a”, Assignment: (Functions and Limits)
except possibly as “a” itself. Feliciano and Uy. Differential & Integral Calculus
Page 3 (numbers 4,6 & 9)
Note: Page 8 (numbers 3, 4 & 5)
lim ¿ : right side of “a”
+¿
x→ a f (x)¿
Read:
lim ¿ : left side of “a” 1. One-sided limits
−¿
x→ a f (x)¿
2. Limits and infinity
Example: Evaluate the limit of the following functions and simplify 3. Infinite limits
4. Continuity of functions/limits
ENGR. IAN C. PAGULAYAN
4
DIFFERENTIAL CALCULUS (MATH 111)
Graph
ENGR. IAN C. PAGULAYAN
5
DIFFERENTIAL CALCULUS (MATH 111)
ENGR. IAN C. PAGULAYAN
6
You might also like
- Tribute To Teacher Retirees 2017 With The Theme: "Precious Gems Stored in The Treasure Chest of Memories"100% (17)Tribute To Teacher Retirees 2017 With The Theme: "Precious Gems Stored in The Treasure Chest of Memories"5 pages
- Student Handbook - Undergraduate Programme 2023-2024No ratings yetStudent Handbook - Undergraduate Programme 2023-2024299 pages
- Applications of Maxima and Minima (Reviewer)No ratings yetApplications of Maxima and Minima (Reviewer)10 pages
- Math 7 Classroom Observation Lesson Plan 1 Final100% (1)Math 7 Classroom Observation Lesson Plan 1 Final9 pages
- Grace Mission College: "Honesty Can Keep You Safe, But If You Can't Be Trusted, You Trap YourselfNo ratings yetGrace Mission College: "Honesty Can Keep You Safe, But If You Can't Be Trusted, You Trap Yourself2 pages
- LAB MANUAL. EXPERIMENT 1. Viscosity of Fluids (Ball Drop Method)33% (3)LAB MANUAL. EXPERIMENT 1. Viscosity of Fluids (Ball Drop Method)6 pages
- Math 203: Number Theory: Alberto D. Yazon, PHDNo ratings yetMath 203: Number Theory: Alberto D. Yazon, PHD56 pages
- Module 3: Problem Solving and Reasoning: What Are The Skills Developed Through Problem Solving?No ratings yetModule 3: Problem Solving and Reasoning: What Are The Skills Developed Through Problem Solving?2 pages
- MODULE 4 Part 2-Integral Calculus - Updated100% (1)MODULE 4 Part 2-Integral Calculus - Updated13 pages
- Calculus 1 (Differential Calculus) Lecture Notes: Engr. Jason Anthony B. ConsultaNo ratings yetCalculus 1 (Differential Calculus) Lecture Notes: Engr. Jason Anthony B. Consulta5 pages
- MAT051 Lesson 1.1 THE INTUITIVE IDEA OF A LIMITNo ratings yetMAT051 Lesson 1.1 THE INTUITIVE IDEA OF A LIMIT9 pages
- Royal Colleges of Science and Management Inc.: College Algebra (MATH 1) First Semester AY: 2022-2023100% (1)Royal Colleges of Science and Management Inc.: College Algebra (MATH 1) First Semester AY: 2022-20236 pages
- Plane Areas in Rectangular Coordinates: Prepared By: Keren TangarorangNo ratings yetPlane Areas in Rectangular Coordinates: Prepared By: Keren Tangarorang38 pages
- Lesson 1 Derivative of Trigonometric FunctionsNo ratings yetLesson 1 Derivative of Trigonometric Functions17 pages
- Rules of Differentiation of Functions in CalculusNo ratings yetRules of Differentiation of Functions in Calculus7 pages
- Calculus 2 A Simplified Text in Integral CalculusNo ratings yetCalculus 2 A Simplified Text in Integral Calculus150 pages
- College of Teacher Education and Human Sciences Preliminary Exam Math in The Modern World Second Semester S.Y. 2019-2020No ratings yetCollege of Teacher Education and Human Sciences Preliminary Exam Math in The Modern World Second Semester S.Y. 2019-20201 page
- Math 311-Differential Equations SyllabusNo ratings yetMath 311-Differential Equations Syllabus2 pages
- Inverse Trigonometric Functions (Trigonometry) Mathematics Question BankFrom EverandInverse Trigonometric Functions (Trigonometry) Mathematics Question BankNo ratings yet
- Flipped-Class 3 - Graphs of Quadratic FunctionsNo ratings yetFlipped-Class 3 - Graphs of Quadratic Functions11 pages
- Flipped-Class 2 - Basic Concepts in Analytic GeometryNo ratings yetFlipped-Class 2 - Basic Concepts in Analytic Geometry22 pages
- Lecture Notes 1 Real Numbers and Real LineNo ratings yetLecture Notes 1 Real Numbers and Real Line16 pages
- Flipped-Class 2 - Basic Concepts in Analytic GeometryNo ratings yetFlipped-Class 2 - Basic Concepts in Analytic Geometry22 pages
- Lecture Notes 1 Real Numbers and Real LineNo ratings yetLecture Notes 1 Real Numbers and Real Line16 pages
- Test Bank Management System Applying Rasch Model and Data Encryption Standard (DES) AlgorithmNo ratings yetTest Bank Management System Applying Rasch Model and Data Encryption Standard (DES) Algorithm9 pages
- Wake County Schools IEP Document: C: EC File, Parent/Guardian Student UID#: 9294965, Page: 1No ratings yetWake County Schools IEP Document: C: EC File, Parent/Guardian Student UID#: 9294965, Page: 110 pages
- Peter M. Mcisaac Museums of The Mind German Modernity and The Dynamics of Collecting 2007No ratings yetPeter M. Mcisaac Museums of The Mind German Modernity and The Dynamics of Collecting 2007338 pages
- Ricardo Padilla Perez v. Secretary of Health & Human Services, 985 F.2d 552, 1st Cir. (1993)No ratings yetRicardo Padilla Perez v. Secretary of Health & Human Services, 985 F.2d 552, 1st Cir. (1993)10 pages
- Germs, Malaria and The Invention of Mansonian Tropical Medicine: From 'Diseases in The Tropics' To 'Tropical Diseases'No ratings yetGerms, Malaria and The Invention of Mansonian Tropical Medicine: From 'Diseases in The Tropics' To 'Tropical Diseases'27 pages
- Concourse Tech330 Economic Analysis For TechnologistsNo ratings yetConcourse Tech330 Economic Analysis For Technologists10 pages
- Simple Past Tense Grammar Drills Grammar Guides - 1928No ratings yetSimple Past Tense Grammar Drills Grammar Guides - 192810 pages
- The Squeezing Potential of Rocks Around Tunnels Theory and PredictionNo ratings yetThe Squeezing Potential of Rocks Around Tunnels Theory and Prediction27 pages
- Tender No: Indian Institute of Technology Madras Chennai 600 036No ratings yetTender No: Indian Institute of Technology Madras Chennai 600 0369 pages
- Images of The Soul in The Shaar Ha GilguNo ratings yetImages of The Soul in The Shaar Ha Gilgu4 pages
- Tribute To Teacher Retirees 2017 With The Theme: "Precious Gems Stored in The Treasure Chest of Memories"Tribute To Teacher Retirees 2017 With The Theme: "Precious Gems Stored in The Treasure Chest of Memories"
- Student Handbook - Undergraduate Programme 2023-2024Student Handbook - Undergraduate Programme 2023-2024
- Grace Mission College: "Honesty Can Keep You Safe, But If You Can't Be Trusted, You Trap YourselfGrace Mission College: "Honesty Can Keep You Safe, But If You Can't Be Trusted, You Trap Yourself
- LAB MANUAL. EXPERIMENT 1. Viscosity of Fluids (Ball Drop Method)LAB MANUAL. EXPERIMENT 1. Viscosity of Fluids (Ball Drop Method)
- Module 3: Problem Solving and Reasoning: What Are The Skills Developed Through Problem Solving?Module 3: Problem Solving and Reasoning: What Are The Skills Developed Through Problem Solving?
- Calculus 1 (Differential Calculus) Lecture Notes: Engr. Jason Anthony B. ConsultaCalculus 1 (Differential Calculus) Lecture Notes: Engr. Jason Anthony B. Consulta
- Royal Colleges of Science and Management Inc.: College Algebra (MATH 1) First Semester AY: 2022-2023Royal Colleges of Science and Management Inc.: College Algebra (MATH 1) First Semester AY: 2022-2023
- Plane Areas in Rectangular Coordinates: Prepared By: Keren TangarorangPlane Areas in Rectangular Coordinates: Prepared By: Keren Tangarorang
- College of Teacher Education and Human Sciences Preliminary Exam Math in The Modern World Second Semester S.Y. 2019-2020College of Teacher Education and Human Sciences Preliminary Exam Math in The Modern World Second Semester S.Y. 2019-2020
- Inverse Trigonometric Functions (Trigonometry) Mathematics Question BankFrom EverandInverse Trigonometric Functions (Trigonometry) Mathematics Question Bank
- Flipped-Class 2 - Basic Concepts in Analytic GeometryFlipped-Class 2 - Basic Concepts in Analytic Geometry
- Flipped-Class 2 - Basic Concepts in Analytic GeometryFlipped-Class 2 - Basic Concepts in Analytic Geometry
- Test Bank Management System Applying Rasch Model and Data Encryption Standard (DES) AlgorithmTest Bank Management System Applying Rasch Model and Data Encryption Standard (DES) Algorithm
- Wake County Schools IEP Document: C: EC File, Parent/Guardian Student UID#: 9294965, Page: 1Wake County Schools IEP Document: C: EC File, Parent/Guardian Student UID#: 9294965, Page: 1
- Peter M. Mcisaac Museums of The Mind German Modernity and The Dynamics of Collecting 2007Peter M. Mcisaac Museums of The Mind German Modernity and The Dynamics of Collecting 2007
- Ricardo Padilla Perez v. Secretary of Health & Human Services, 985 F.2d 552, 1st Cir. (1993)Ricardo Padilla Perez v. Secretary of Health & Human Services, 985 F.2d 552, 1st Cir. (1993)
- Germs, Malaria and The Invention of Mansonian Tropical Medicine: From 'Diseases in The Tropics' To 'Tropical Diseases'Germs, Malaria and The Invention of Mansonian Tropical Medicine: From 'Diseases in The Tropics' To 'Tropical Diseases'
- Concourse Tech330 Economic Analysis For TechnologistsConcourse Tech330 Economic Analysis For Technologists
- Simple Past Tense Grammar Drills Grammar Guides - 1928Simple Past Tense Grammar Drills Grammar Guides - 1928
- The Squeezing Potential of Rocks Around Tunnels Theory and PredictionThe Squeezing Potential of Rocks Around Tunnels Theory and Prediction
- Tender No: Indian Institute of Technology Madras Chennai 600 036Tender No: Indian Institute of Technology Madras Chennai 600 036