Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd Logo
Upload

Welcome to Scribd!

  • 5 views

    Chapter 2-Kinematics

    Uploaded by

    antonstefanbiehler
    This document provides definitions and equations related to kinematics, including: 1) It defines displacement, velocity, acceleration, and speed, and distinguishes between scalars and vectors. 2) It presents three equations of motion that apply to uniform acceleration in a straight line. 3) It describes the motion of an object falling with air resistance, noting how acceleration decreases from g as drag force increases over time.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd

    Chapter 2-Kinematics

    Uploaded by

    antonstefanbiehler
    5 views1 page
    This document provides definitions and equations related to kinematics, including: 1) It defines displacement, velocity, acceleration, and speed, and distinguishes between scalars and vectors. 2) It presents three equations of motion that apply to uniform acceleration in a straight line. 3) It describes the motion of an object falling with air resistance, noting how acceleration decreases from g as drag force increases over time.

    Original Description:

    AS physics notes

    Copyright

    © © All Rights Reserved

    Available Formats

    PDF, TXT or read online from Scribd

    Did you find this document useful?

    Is this content inappropriate?

    This document provides definitions and equations related to kinematics, including: 1) It defines displacement, velocity, acceleration, and speed, and distinguishes between scalars and vectors. 2) It presents three equations of motion that apply to uniform acceleration in a straight line. 3) It describes the motion of an object falling with air resistance, noting how acceleration decreases from g as drag force increases over time.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    5 views1 page

    Chapter 2-Kinematics

    Uploaded by

    antonstefanbiehler
    This document provides definitions and equations related to kinematics, including: 1) It defines displacement, velocity, acceleration, and speed, and distinguishes between scalars and vectors. 2) It presents three equations of motion that apply to uniform acceleration in a straight line. 3) It describes the motion of an object falling with air resistance, noting how acceleration decreases from g as drag force increases over time.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    You are on page 1of 1

    Chapter 2

    Kinematics
    _____
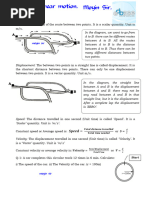
    Rectilinear Motion (1-D motion) Graphs: Definitions:
    Remember to assign a reference positive direction and be constant throughout. Displacement: is the distance travelled in a stated direction from a reference
    Waves
    point. (Vector)
    Types of Graphs Gradient represents Area Under Graph
    Velocity: is the rate of change of displacement with respect to time. (Vector)
    represents
    Displacement – Time 𝑑𝑠 - Acceleration: is the rate of change of velocity with respect to time. (Vector)
    𝑣𝑒𝑙𝑜𝑐𝑖𝑡𝑦 =
    𝑑𝑡 Speed: is the rate of change of distance travelled with respect to time. (Scalar)
    Velocity – Time 𝑑𝑣 Displacement
    𝑎𝑐𝑐𝑒𝑙𝑒𝑟𝑎𝑡𝑖𝑜𝑛 =
    𝑑𝑡 Equations of Motion: only apply for uniform acceleration in a straight line.
    Acceleration - Time - Change in velocity
    1) 𝑣 = 𝑢 + 𝑎𝑡
    1 Derived using area under Velocity-
    Motion of object falling in a uniform g-field with air resistance: 2) 𝑠 = 𝑢𝑡 + 𝑎𝑡 2 Time graph and Equation (1)
    2
    Time Forces acting on object Motion of object
    3) 𝑣 2 = 𝑢2 + 2𝑎𝑠 Derived using Equation (1) and (2)
    𝒕 = 𝟎𝒔  Initial velocity is zero
    Above equations are used in a uniform gravitational field without air resistance.
     Only force acting on object is its
    weight
    Projectile Motion (Non-linear motion):
     𝑨𝒄𝒄𝒆𝒍𝒆𝒓𝒂𝒕𝒊𝒐𝒏 = 𝒈
    Horizontal Motion: Vertical Motion:
     Velocity constant, Acceleration is 0  Acceleration is constant
    𝒕 = 𝒕𝟏  As object velocity increases, the
    drag force (due to air resistance
    increases from zero.
     𝐹𝑛𝑒𝑡 = 𝑚𝑔 − 𝐹𝐷
     𝑨𝒄𝒄𝒆𝒍𝒆𝒓𝒂𝒕𝒊𝒐𝒏 < 𝒈
    𝒕 = 𝒕𝟐  When 𝐹𝐷 = 𝑚𝑔, resultant force on
    object is zero.
     𝑨𝒄𝒄𝒆𝒍𝒆𝒓𝒂𝒕𝒊𝒐𝒏 = 𝟎
     Object falls with max. constant
    velocity, called Terminal Velocity

    Superphysics.sg

    You might also like