Basic Integral Formula
Basic Integral Formula
Uploaded by
kotekatbasil0000Copyright:
Available Formats
Basic Integral Formula
Basic Integral Formula
Uploaded by
kotekatbasil0000Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
Basic Integral Formula
Basic Integral Formula
Uploaded by
kotekatbasil0000Copyright:
Available Formats
Basic Integral Formula
xn+1 dx 1 a−x
∫ x n dx = n+1
+c ∫ a2 −x2 = 2a log |a+x| + c.
dx
∫ dx = x + c. ∫ √x2 +a2 = log|x + √x 2 + a2 | + c.
dx
∫ = log|x| + c. x√x2 +a2 a2
x ∫ √x 2 + a2 dx = 2
+ 2
log|x + √x 2 + a2 | + c.
dx 1
∫ ax+b = a log|ax + b| + c. dx
∫ √x2 −a2 = log|x + √x 2 − a2 | + c.
ax
∫ ax dx = log a + c x√x2 −a2 a2
∫ √x 2 − a2 dx = 2
− 2
log|x + √x 2 − a2 | + c.
∫ ex dx = ex + c. `
x√a2 −x2 a2 x
∫ √a2 − x 2 dx = 2
+ 2
sin−1 a + c.
∫ sin x dx = − cos x + c
𝑒 𝑎𝑥
∫ cos x dx = sin x + c. ∫ 𝑒 𝑎𝑥 sin 𝑏𝑥 𝑑𝑥 = (𝑎 sin 𝑏𝑥 − 𝑏 cos 𝑏𝑥) + 𝑐
𝑎2 + 𝑏 2
∫ tan x dx = − log|cos x| + c = log|sec x| + c. 𝑒 𝑎𝑥
𝑎𝑥 (𝑎 cos 𝑏𝑥 + 𝑏 sin 𝑏𝑥) + 𝑐
∫𝑒 cos 𝑏𝑥 𝑑𝑥 = 2
𝑎 + 𝑏2
∫ cot x dx = log|sin x| + c.
∫ sec x tan x dx = sec x + c. PARTIAL FRACTION
∫ cosec x cot x dx = − cosec x + c. 𝑝𝑥 + 𝑞 𝐴 𝐵
= +
(𝑥 − 𝑎)(𝑥 − 𝑏) 𝑥 − 𝑎 𝑥 − 𝑏
∫ sec 2 x dx = tan x + c
𝑝𝑥 + 𝑞 𝐴 𝐵
2
= +
2
∫ cosec x dx = − cot x + c. (𝑥 − 𝑎) 𝑥 − 𝑎 (𝑥 − 𝑎)2
∫ sec x dx = log|sec x + tan x| + c 𝑝𝑥 2 + 𝑞𝑥 + 𝑟 𝐴 𝐵 𝐶
= + +
(𝑥 − 𝑎)(𝑥 − 𝑏)(𝑥 − 𝑐) 𝑥 − 𝑎 𝑥 − 𝑏 𝑥 − 𝑐
∫ cosec x dx = log|cosec x − cot x| + c
𝑝𝑥 2 + 𝑞𝑥 + 𝑟 𝐴 𝐵 𝐶
dx = + +
∫ √1−x2 = sin−1 x + c or − cos −1 x + c. (𝑥 − 𝑎)2 (𝑥 − 𝑏) 𝑥 − 𝑎 (𝑥 − 𝑎)2 𝑥 − 𝑏
dx 𝑝𝑥 2 + 𝑞𝑥 + 𝑟 𝐴 𝐵𝑥 + 𝐶
∫ 1+x2 = tan−1 x + c or − cot −1 x + c. 2
= + 2
(𝑥 − 𝑎)(𝑥 + 𝑏𝑥 + 𝑐) 𝑥 − 𝑎 𝑥 + 𝑏𝑥 + 𝑐
dx
∫ x√x2 −1 = sec −1 x + c or − cosec −1 x + c. INTEGRATION BY PARTS
dx x
∫ √a2 −x2 = sin−1 a + c. ∫ 𝑓(𝑥)𝑔(𝑥)𝑑𝑥 = 𝑓(𝑥) ∫ 𝑔(𝑥) 𝑑𝑥
dx 1 x 𝑑
∫ a2 +x2 = a tan−1 a + c. − ∫[ (𝑓(𝑥)) ∫ 𝑔(𝑥)𝑑𝑥] 𝑑𝑥
𝑑𝑥
dx 1 x
∫ x√x2 −a2 = a sec −1 a + c. SPECIAL TYPE INTEGRALS
dx 1 x−a
∫ x2 −a2 = 2a log |x+a| + c. ∫ 𝑒 𝑥 [𝑓(𝑥) + 𝑓 ′ (𝑥)] 𝑑𝑥 = 𝑒 𝑥 𝑓(𝑥) + 𝑐
1|Page
You might also like
- Advanced Engineering Mathematics 3rd Edition PDFDocument775 pagesAdvanced Engineering Mathematics 3rd Edition PDFLeangHeySey100% (2)
- Maths Extension 2 - IntegrationDocument14 pagesMaths Extension 2 - IntegrationTommybilwijNo ratings yet
- Integration FormulaDocument2 pagesIntegration FormulaAMIN BUHARI ABDUL KHADERNo ratings yet
- IntegrationDocument123 pagesIntegrationHari KrushnaNo ratings yet
- Integration: Antiderivative of F or A Primitive of FDocument6 pagesIntegration: Antiderivative of F or A Primitive of Fvanesh wildNo ratings yet
- Practico IntegralesDocument27 pagesPractico IntegralesDon BarredoraNo ratings yet
- INT 1: If: FXDX FX CDocument30 pagesINT 1: If: FXDX FX Cmukesh_49679852No ratings yet
- Class XII - Math Chapter: Integral CalculusDocument5 pagesClass XII - Math Chapter: Integral CalculusSunita MauryaNo ratings yet
- Form (Integration3)Document9 pagesForm (Integration3)api-3724082No ratings yet
- 1PC CALCULO DIFERENCIAÑLDeI 2018Document3 pages1PC CALCULO DIFERENCIAÑLDeI 2018Jimena Alfaro LoyolaNo ratings yet
- Integrarea Functiilor RationaleDocument4 pagesIntegrarea Functiilor RationaleAdriana Pelea BrandasNo ratings yet
- Metode de Integrare. Integrarea Functiilor Rationale: 1 2 P p+1 N 1 2 1 1 1 2 1 1 M 2 M M m+1 2 m+1 m+1 KDocument4 pagesMetode de Integrare. Integrarea Functiilor Rationale: 1 2 P p+1 N 1 2 1 1 1 2 1 1 M 2 M M m+1 2 m+1 m+1 KVlad IsacNo ratings yet
- Integration 101 Examples With Full Solutions Not MineDocument34 pagesIntegration 101 Examples With Full Solutions Not MineHyojinNo ratings yet
- DRT 11 Neet SolDocument2 pagesDRT 11 Neet SolPRADEEP CNo ratings yet
- 23 - PDFsam - 01 رياضيات 1-بDocument2 pages23 - PDFsam - 01 رياضيات 1-بMina AlbertNo ratings yet
- Chap 2 Calculus ECDocument28 pagesChap 2 Calculus ECMidhun BabuNo ratings yet
- Formula Sheet 07 _ Integration __ Lakshya MHTCET 2025Document5 pagesFormula Sheet 07 _ Integration __ Lakshya MHTCET 2025sameerpinjarkarNo ratings yet
- Formulae,,key Points,, Algorithm (For Class 12 TH Maths)Document23 pagesFormulae,,key Points,, Algorithm (For Class 12 TH Maths)angusingh60No ratings yet
- Formula de Integrare Prin PărțiDocument3 pagesFormula de Integrare Prin PărțiBiancaPascuNo ratings yet
- Integration by PartsDocument1 pageIntegration by PartsKim RobynNo ratings yet
- Integrales Inmediatas: LN LN LN LN LNDocument1 pageIntegrales Inmediatas: LN LN LN LN LNJuan Cruz EmmaNo ratings yet
- E (1 X) DX e (1 X) DX Edx Xedx: Exercise - 8 (F)Document14 pagesE (1 X) DX e (1 X) DX Edx Xedx: Exercise - 8 (F)Javaji SowmyaNo ratings yet
- Integration FormulaeDocument7 pagesIntegration Formulaeommayekar19No ratings yet
- DRT 10 Xii Neet SolDocument2 pagesDRT 10 Xii Neet SolPRADEEP CNo ratings yet
- Tavola TaylorDocument2 pagesTavola TaylorRiccardo PalumboNo ratings yet
- X Integrals 30Document1 pageX Integrals 30MasudRanaNo ratings yet
- Integration FormulasDocument5 pagesIntegration Formulasa79c5.pvtNo ratings yet
- Exercise SheetsDocument34 pagesExercise Sheetssyz1103174840No ratings yet
- Intergration Unit Sol-7Document8 pagesIntergration Unit Sol-7ishaanrox64No ratings yet
- MAST10006 Calculus 2 Exercise Sheets 2023s1Document34 pagesMAST10006 Calculus 2 Exercise Sheets 2023s1Shyam MahendraNo ratings yet
- Tabel de Integrale NedefiniteDocument1 pageTabel de Integrale Nedefinitealamanca100% (6)
- CH 7.integration 1 Ak 2024-25Document24 pagesCH 7.integration 1 Ak 2024-25latoy53115No ratings yet
- Improper Integral FormulasDocument15 pagesImproper Integral FormulassaiganeshvetrivelanNo ratings yet
- 7 IntegralsDocument5 pages7 Integralspatilnagraj5622No ratings yet
- Samakalan FormulaDocument1 pageSamakalan Formulaksanjay01064No ratings yet
- 2da PC CÁLCULO DIFERENCIALDocument9 pages2da PC CÁLCULO DIFERENCIALJimena Alfaro LoyolaNo ratings yet
- 4.integrals Yielding To Trigo & Inv Trigo FNDocument28 pages4.integrals Yielding To Trigo & Inv Trigo FNUnesca TeeNo ratings yet
- Practica #3 SolucionarioDocument3 pagesPractica #3 SolucionarioAbel Alejandro Pinto QuintanaNo ratings yet
- Diff & Integrals FormulaeDocument2 pagesDiff & Integrals FormulaeExodusNo ratings yet
- Integrals: Elementary FormsDocument32 pagesIntegrals: Elementary FormsndourNo ratings yet
- Tarea CalculoDocument4 pagesTarea Calculoearteaga0975No ratings yet
- Integration Partial FractionsDocument11 pagesIntegration Partial Fractionsnyakallotoons939No ratings yet
- IntegralesDocument6 pagesIntegralesFer CLNo ratings yet
- Unidad 1 Integrales Calculo Integral Valeria Casandra Rios Tellez ES1922027238 Raymundo Irineo Zanabria Salcedo LT-LCIN-2002-B1-001 24-07-2020Document6 pagesUnidad 1 Integrales Calculo Integral Valeria Casandra Rios Tellez ES1922027238 Raymundo Irineo Zanabria Salcedo LT-LCIN-2002-B1-001 24-07-2020Valeria CasandraNo ratings yet
- Formula Paper For Calculus120 and Pre Calculus BDocument12 pagesFormula Paper For Calculus120 and Pre Calculus BShreya PodderNo ratings yet
- Integration Formulas-1 PDFDocument2 pagesIntegration Formulas-1 PDFYashvardhan AgarwalNo ratings yet
- Integral FormulasDocument2 pagesIntegral Formulasakku jainNo ratings yet
- Integration FormulasDocument5 pagesIntegration FormulasAhmad NaeemNo ratings yet
- FormulasDocument3 pagesFormulasيوسف العامري100% (1)
- MATH 259 - Review Sheet (Prerequisite Topics) : (This Is NOT A Comprehensive List!)Document4 pagesMATH 259 - Review Sheet (Prerequisite Topics) : (This Is NOT A Comprehensive List!)Celal KermangilNo ratings yet
- Apni KakshaDocument22 pagesApni Kakshajayaburman05No ratings yet
- DerivadasDocument1 pageDerivadasItan EscandonNo ratings yet
- Unknown 15Document27 pagesUnknown 15Pragya PanwarNo ratings yet
- Tavole PDFDocument3 pagesTavole PDFIvan TomaNo ratings yet
- TaylorDocument3 pagesTaylorBernardo GalvanoNo ratings yet
- Tavole PDFDocument3 pagesTavole PDFMarco TestaNo ratings yet
- Tavole PDFDocument3 pagesTavole PDFRenato Di MasoNo ratings yet
- Tavole PDFDocument3 pagesTavole PDFMarco TestaNo ratings yet
- DX X dxn1Document3 pagesDX X dxn1jadhavadi5354No ratings yet
- Trigonometric Ratios to Transformations (Trigonometry) Mathematics E-Book For Public ExamsFrom EverandTrigonometric Ratios to Transformations (Trigonometry) Mathematics E-Book For Public ExamsRating: 5 out of 5 stars5/5 (1)
- Multiple Chose QuestionsDocument8 pagesMultiple Chose Questionsvinod kumarNo ratings yet
- Introduction To MatricesDocument2 pagesIntroduction To MatricesMiss GurlNo ratings yet
- Chapter 1 - Groups and FieldsDocument33 pagesChapter 1 - Groups and FieldsChristopherNo ratings yet
- Math8 - q1 - Mod12 - Finding The Equations of A Line - 08092020Document17 pagesMath8 - q1 - Mod12 - Finding The Equations of A Line - 08092020Cassandra Nicole Francisco100% (1)
- Chapter 3 OTSMDocument74 pagesChapter 3 OTSMayush5699.ajNo ratings yet
- 2nd Preboard 23 - 24Document7 pages2nd Preboard 23 - 24notcute02No ratings yet
- Warm-Up #2 - 1: Write The Following Solutions in Interval Notation Then Graph On A Number Line: 1) 2) 3)Document4 pagesWarm-Up #2 - 1: Write The Following Solutions in Interval Notation Then Graph On A Number Line: 1) 2) 3)Samuel AdamsNo ratings yet
- Matlab Random Walk Solution (Code)Document7 pagesMatlab Random Walk Solution (Code)YussefRikliNo ratings yet
- Exercise 4bDocument10 pagesExercise 4bDR KOOKGACH LIFENo ratings yet
- 2ndWEEK 3Document13 pages2ndWEEK 3Vanessa Pangan KellerNo ratings yet
- Two Variable Linear EquationDocument3 pagesTwo Variable Linear EquationEdelNo ratings yet
- Harvard University - Linear Algebra & Differential EquationDocument70 pagesHarvard University - Linear Algebra & Differential Equationeverlbs100% (3)
- 7 Slope Linear Functions Practice FULLDocument25 pages7 Slope Linear Functions Practice FULLAbdelrazig KamalNo ratings yet
- Numerical Methods NotesDocument553 pagesNumerical Methods Notesfuwad84100% (1)
- Assurance ExamDocument4 pagesAssurance Examegberongbetunde9No ratings yet
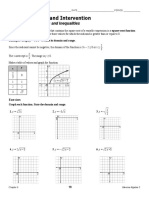
- 7 6 Study Guide and Intervention Worksheet - Tier 2Document3 pages7 6 Study Guide and Intervention Worksheet - Tier 2api-292698981No ratings yet
- 9709 w14 QP 33Document4 pages9709 w14 QP 33Nina AmaniNo ratings yet
- Determinants MCQSDocument5 pagesDeterminants MCQSNoo EntryNo ratings yet
- Abdi ValentinDocument13 pagesAbdi ValentingiancarlomoronNo ratings yet
- A-Level Mathematics - 9164 Syllabus PDFDocument37 pagesA-Level Mathematics - 9164 Syllabus PDFChris PunungweNo ratings yet
- CC301B - Discrete MathematicsDocument4 pagesCC301B - Discrete MathematicspraveenNo ratings yet
- 3DDocument10 pages3DAnonymous RuJRdfL9HINo ratings yet
- 6-3 Study Guide and Intervention: Square Root Functions and InequalitiesDocument2 pages6-3 Study Guide and Intervention: Square Root Functions and InequalitiesGLoOmItONo ratings yet
- 2ND - Math TosDocument3 pages2ND - Math TosAnalyn LuzonNo ratings yet
- Polynomial Math 8Document33 pagesPolynomial Math 8Lea Jane RafalesNo ratings yet
- Integral Calculus NotesDocument43 pagesIntegral Calculus NotesArjay S. Bazar100% (1)
- Symbols: Department of Mathematics, Iit KharagpurDocument8 pagesSymbols: Department of Mathematics, Iit Kharagpurjeremysarfer12No ratings yet
- G 1 1 P - C M (3 0 S) : Final Practice ExamDocument32 pagesG 1 1 P - C M (3 0 S) : Final Practice ExamIan Jeff DimaunahanNo ratings yet
- Nonlinear Continuum Mechanics Nonlinear Continuum Mechanics: 2. MotionDocument68 pagesNonlinear Continuum Mechanics Nonlinear Continuum Mechanics: 2. Motionahmadomar89No ratings yet