MI H2 2021 Prelim P1 Solution
MI H2 2021 Prelim P1 Solution
Uploaded by
zuyun2004Copyright:
Available Formats
MI H2 2021 Prelim P1 Solution
MI H2 2021 Prelim P1 Solution
Uploaded by
zuyun2004Original Description:
Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
MI H2 2021 Prelim P1 Solution
MI H2 2021 Prelim P1 Solution
Uploaded by
zuyun2004Copyright:
Available Formats
1
2021 Preliminary Examination
PU3 MATHEMATICS 9758/01
Solutions
Qn Solution
1(i) d 1 d
( 4 − x2 )
−1
[1] = 2
dx 4 − x dx
2x
=
( 4 − x2 )
2
1(ii) ⌠ x2
[4] dx 1 dv 2x
⌡ (4 − x )
2 2 Let u = x, =
2 dx ( 4 − x 2 )2
⌠
1 2x du 1 1
= x dx = , v=
2 (4 − x )
2
2 dx 2 4 − x2
⌡
1 1 ⌠ 1 1
= x 2
− 2
dx
2 4 − x ⌡ 2 4 − x
x 1 1
= − ⌠ dx
2(4 − x ) 2 ⌡ 4 − x
2 2
x 1 1 2+ x
=2 − ln +c
2 ( 4 − x ) 2 2 ( 2 ) 2 − x
x 1 2+ x
= − ln +c
2 ( x + 4) 8 2 − x
2
Qn Solution
2(a) ( 0, b ) → ( 0, 0 )
translate b units in negaive y direction
(i)
[1]
Note: ( a, 0 ) → ( a, −b ) ( not required )
2(ii) scale parallel to x axis by scale factor
1
[1] ( a, 0 ) →
a
(1, 0 )
1
scale parallel to x axis by scale factor
( 0, b ) → a
( 0, b )
2(b) Range of g = ( −∞,3) ∪ ( 3, ∞ ) or \ {3}
(i)
[2] Domain of h = ( −∞, ∞ )
Since range of g ⊆ domain of h
⇒ hg exists
2(b) 1 1
(ii) hg ( x ) =2 − 3 − =−1 +
x −1 x −1
[3]
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
2
Qn Solution
Method 1
( hg ) ( 3) = a
−1
hg ( ( hg ) ( 3) ) = hg ( a )
−1
1
3 =−1 +
a −1
1
=4
a −1
5
a=
4
-------------------------------------------------------
Method 2
To find ( hg )
−1
Let
1
y =−1 +
x −1
1
y +1 =
x −1
1
x −1 =
y +1
1
=x +1
y +1
1
( hg ) = ( x)
−1
+1
x +1
1 5
( hg ) ( 3=)
−1
1
+=
3 +1 4
Qn Solution
3(i) dy du
[3] y = ux3 ⇒ = x3 + 3ux 2
dx dx
du
x 2 x3 + 3ux 2 − 3 x ( ux 3 ) + 4 =0
dx
du
x5 + 3ux 4 − 3ux 4 + 4 = 0
dx
du
x5 +4= 0
dx
du 4
= − 5
dx x
3(ii) du 4
[4] = − 5
dx x
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
3
Qn Solution
Integrating with respect to x on both sides of the equation,
4
u= ∫ − 5 dx
x
∫ −4 x
−5
= dx
= x −4 + c
y
y= ux3 ⇒ u =
x3
y
= 3
x −4 + c
x
y x −1 + cx3
=
When x = 1 , y = 3,
c=2
∴ y = x −1 + 2 x 3
Qn Solution
4(i) ac is the length of projection of c onto a
[1]
OR
ac is the length of projection of OC onto OA .
4(ii) ( 2a − b )( 2a − b ) = 4(aa) − 2(ab) − 2(ba) + (bb)
[3] 2 2
=4 a − 4(ab) + b ( ba =ab)
4(1) − 4 a b cos 60 + (2) 2
= 2 o
1
= 4 − 4(1)(2) + 4 = 4.
2
( 2a − b )( 2a − b ) =
2
2a − b = 4 ⇒ 2a − b = 2.
4(iii) By Ratio Theorem,
[1] b + 2a
c= .
3
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
4
4(iv) ac
cos ∠AOC =
[3] a c
b + 2a
a
3
= [from (iii)]
a c
1 ab + 2(12 ) 2
= [since a=
a a= 12 ]
3 c
1 ab + 2
= .
3 c
bc
cos ∠COB =
b c
b + 2a
b
3
= [from (iii)]
b c
1 22 + 2(ab) 2
= [since b=
b b= 22 ]
3 2c
1 2 + ab
= = cos ∠AOC.
3 c
Therefore line OC bisects the angle AOB.
Qn Solution
5(i) dx
x = tan θ ⇒ = sec 2 θ
[4] dθ
1 1
∫ x 2 + 1 dx = ∫ tan 2 θ + 1 ( sec θ ) dθ
2
1
=∫
sec θ
( sec2 θ ) dθ
= ∫ sec θ dθ
= ln sec θ + tan θ + c
= ln x2 + 1 + x + c
Alternatively,
1 + tan 2 θ =
sec 2 θ ⇒ sec θ =1 + x 2 .
5(ii) 1
=
[4] y −1
( x − 1)
2
1
=y2 −1
( x − 1)
2
1
( x − 1)
2
= 2
y +1
1
x − 1 =±
y2 +1
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
5
1
1−
x= ( x < 1)
y2 +1
Volume of solid
1
2 1
⌠ 3 1 ⌠ 3 2 1
=π 1 − dy =π 1 − + 2 dy
y 2
+ 1 ⌡ y 2
+ 1 y + 1
⌡0 0
= π y − 2 ln y 2 + 1 + y + tan −1 y [ from part (i)]
3
0
1 2
1 1 −1 1
= π − 2 ln +1 + + tan −0
3 3 3 3
1 2 1 π
= π − 2 ln + +
3 3 3 6
1 π 1 π
= π − 2 ln 3 + OR π − ln 3 + units3 .
3 6 3 6
Qn Solution
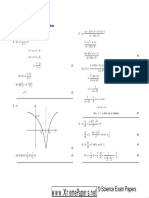
6(i)
[4] y
O x
6(ii) Method 1: Simplifying the modulus using the graph
[3] 1
From the graph, the root of = b ( x − a ) is equal to the root of
a−x
1 1 1
b ( x − a) ⇒ = ( x − a) ⇒ x = a ±
2
− = .
a−x b b
1
Since x > a, x= a + .
b
--------------------------------------------------------------------------
Method 2: Solve by squaring both sides
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
6
2
1 1
= b ( x − a) ⇒ b2 ( x − a )
2
=
a−x a−x
2
1
⇒ =( x − a ) ( a − x ) =( x − a )
2 2 4
b
1
⇒ = ( x − a ) (since b > 0)
2
b
1
⇒ x =a± .
b
1
Since x > a, x= a + .
b
6(iii) From the sketch in part (i),
[2] 1
x < a or a < x < a +
b
OR
1
x<a+ , x≠a
b
Qn Solution
7(i) un = a n +1
− ( n + 1)
a
[2]
u1 =0 ⇒ a 2 − 2a =0 ⇒ a 2 =2a … (*)
Method 1:
u3 = a 4 − 4a = ( a 2 ) − 4a
2
= (2 ) a 2
− 4a [from (*)]
= (2 ) 2 a
− 4a = 4a − 4a = 0.
Method 2:
u3 = a 4 − 4a = ( a 2 ) − ( 22 )
2 a
= (a ) − (2 )
2 2 a 2
( a − 2 )( a + 2 )
= 2 a 2 a
= 0. [since = a 2 − 2a 0 from (*)]
7(ii) u = 2n +1 − ( n + 1)2
n
[4]
Method 1: Expansion
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
7
Qn Solution
n n
∑=
u ∑ 2 − ( r + 1)
r +1 2
=
r
r 1= r 1
n n
∑ 2r +1 − ∑ ( r + 1)
2
=
=r 1=r 1
n n
= ∑2 r +1
− ∑ ( r 2 + 2r + 1)
=r 1=r 1
n
n n n
=r 1
= ∑2 r +1
− ∑ r 2 + 2∑ r + ∑ 1
= r 1 =r 1 =r 1
4 ( 2n − 1) n n
= − ( n + 1)( 2n + 1) + 2 ( n + 1) + n
2 −1 6 2
n
= 4 ( 2n − 1) − ( n + 1)( 2n + 1) − n(n + 1) − n
6
n
OR 4 ( 2n − 1) − ( n + 1)( 2n + 1) − n 2 − 2n
6
n
OR 4 ( 2n − 1) − ( n + 1)( 2n + 1) − n(n + 2)
6
Method 2: Change of index
n n
∑= ∑ 2 − ( r + 1)
r +1 2
ur
=r 1=r 1
n n
∑2 − ∑ ( r + 1)
r +1 2
=
=r 1=r 1
n n +1
=r 1=
=
r 2
∑ 2r × 2 − ∑ r 2
n
n +1
=2∑ 2r − ∑ r 2 − 12
= r 1= r 1
2 ( 2n − 1) n + 1
= 2 − ( n + 1 + 1) ( 2 ( n + 1) + 1) − 1
2 − 1 6
1
= 4 ( 2n − 1) − ( n + 1)( n + 2 )( 2n + 3) + 1
6
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
8
Qn Solution
7(iii) 9
[2] ∑ (v
r =1
r +1 − vr ) =v2 − v1
+ v3 − v2
+ ...
+ v9 − v8
+ v10 − v9
= v10 − v1
10
∑u
r =1
r = 3587. [from (ii) or from graphing calculator]
3587
Hence, v10 − v1 =
⇒ v10= 3587 + v=
1 3587 + u1 [since v=
1 u1 (given)]
= 3587 + [21+1 − (1 + 1) 2 ] (since =
⇒ v10 a 2)
⇒ v=
10 3587 +=
0 3587.
Qn Solution
8(i) y
[2]
y =1
O x
x=2
8(ii) x =+cot t 2 y= sec t
[3] dx dy
= − cos ec 2t =sec t tan t
dt dt
dy sec t tan t
=
dx − cos ec 2t
1 sin t
cos t cos t
=
1
− 2
sin t
sin 3 t
= −
cos 2 t
π
Since − < t < 0 , sin 3 t < 0, cos 2 t > 0
2
dy
Therefore > 0 , C is increasing
dx
8(iii) Method 1
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
9
Qn Solution
[2] Using GC,
π dy
At t = − , x= 1, y =1.4142, =0.70711 ,
4 dx
−1
Gradient of normal = −1.4142
0.70711
Equation of normal
y − 1.4142 = −1.4142 ( x − 1)
−1.4142 x + 2.8284
y=
−1.41x + 2.83 (3s.f.)
y=
-
Method 2
π
sin 3 −
π π dy 4 =1
At t =− , x= 1, y = sec = 2, =
− ,
4 4 dx 2 π 2
cos −
4
1
Gradient of normal − − 2
=
1
2
Equation of normal
y− 2 = − 2 ( x − 1)
y= − 2x + 2 2
8(iv) Midpoint R
[3] ( cot p + 2 ) − 2 sec p
= ,
2 2
cot p sec p
= ,
2 2
cot p sec p
= x = y
2 2
1
=tan p = sec p 2 y
2x
Method 1
Using trigonometric identity,
tan 2 p + 1 =sec 2 p
2
1
(2y)
2
+1 =
2x
1
4=y2 +1
4x2
-------------------------------------------------------------------------
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
10
Qn Solution
Method 2
1
tan p =
2x
Using the right angle triangle,
1
= 2y
2x
2
4x +1
4x2 + 1
y=
4x
-------------------------------------------------------------------------
Method 3
1
cos p =
2y
Using the right angle triangle,
1
tan p =
2x
1
= 2x
4 y2 −1
1
2
= 4x2
4 y −1
Qn Solution
9(a) For w = 1 − 3 i :
(i)
( 3)
2
[4] 1 − 3 i =12 +
w= 2
=
( w ) arg 1 −
arg= ( 3 i)
3 1
=− tan −1 =− π.
1 3
3 3
For z
= 2 cos π − i sin π :
4 4
3 3 3 3
z = 2 cos π − i sin π = 2 cos − π +i sin − π
4 4 4 4
3
Therefore z = 2 and arg ( z ) = − π .
4
Method 1
2
w= z* w =
2
z 22 = 2 4 2 ( )
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
11
Qn Solution
( w z *) arg ( w ) + arg ( z *)
arg = 2 2
= 2 arg ( w ) − arg ( z )
1 3 1
= 2 − π − − π = π.
3 4 12
1 1
Therefore,
= w2 z* 4 2 cos π + i sin π .
12 12
Method 2
π 3π 3π
− i − i i
w = 2e 3
, z
= 2e 4
z*
⇒= 2e 4
2
2
−π i 3π
i
w z* = 2e 3 2e 4
2π 3π
− i i
= 4e 3
2e 4
π
i
= 4 2 e12
1 1
= w2 z* 4 2 cos π + i sin π
12 12
9(a) Method 1
(ii) n nπ nπ
[2] w= 2n cos − + i sin −
3 3
( )
For Re wn = 0 ,
nπ
Re ( w =
) 2n n
cos − = 0
3
nπ
cos − = 0
3
nπ 3π π π 3π
− = ..., − , − , , ,...
3 2 2 2 2
nπ ( 2k + 1) π
=− , where k ∈
3 2
3 ( 2k + 1)
n= −
2
3 ( 2k + 1)
Since 3 ( 2k + 1) is odd for all k ∈ , is never an integer. Thus there is
2
no integer value of n such that the real part of wn is zero.
---------------------------------------------------------------------
Method 2
For the real part of wn to be zero, this means wn is purely imaginary.
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
12
Qn Solution
5π 3π π π 3π 5π
arg ( wn ) =..., − , − , − , , , ,
2 2 2 2 2 2
π
= ( 2k + 1) , where k ∈
2
n
Since arg ( wn ) = n arg ( w ) = − π ,
3
n π
− π = ( 2k + 1)
3 2
n 2k + 1
− =
3 2
3 ( 2k + 1)
n= −
2
3 ( 2k + 1)
Since 3 ( 2k + 1) is odd for all k ∈ , is never an integer. Thus there is
2
no integer value of n such that the real part of wn is zero.
9(b) 3 z 3 + 13 z 2 + 20 z + 14 =
0
(i) Since all coefficients of the equation are real and −1 + i is a root, −1 − i is another
[4] root.
A quadratic factor:
z − ( −1 + i ) z − ( −1 − i ) = [ z + 1 − i ][ z + 1 + i ]
= ( z + 1) − i ( z + 1) + i
=( z + 1) − i 2
2
= z 2 + 2 z + 1 − ( −1)
= z2 + 2z + 2
⇒ 3 z 3 + 13 z 2 + 20 z + 14 = (z 2
+ 2 z + 2 ) ( az + b )
Method 1 Method 2
Comparing z 3 terms: 3z + 7
2 3 2
a=3 z + 2 z + 2 3 z + 13 z + 20 z + 14
− ( 3z 3 + 6 z 2 + 6 z )
Comparing constant terms:
7z 2 + 14 z + 14
2b = 14
− ( 7z 2 + 14 z + 14 )
⇒b= 7
0
7
We have, 3 z 3 + 13 z 2 + 20 z + 14 = (z 2
+ 2 z + 2 ) ( 3 z + 7 ) . 3 z + 7 =0 ⇒ z =− .
3
7
Therefore, the other roots are −1 − i and − .
3
9(b) 3 2
Given w + 13w + 60 w + 126 = 0
(ii) Divide 9 throughout:
[2]
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
13
Qn Solution
1 3 13 2 60 126
w + w + w+ = 0
9 9 9 9
1 3 13 2 20
w + w + w + 14 =0
9 9 3
3 2
1 1 1
3 w + 13 w + 20 w + 14 =
0
3 3 3
1
So, z in 3 z 3 + 13 z 2 + 20 z + 14 has been replaced with w.
3
7
z =−1 + i, z =−1 − i, z =−
3
1 1 1 7
w =−1 + i, w =−1 − i, w =−
3 3 3 3
Therefore, w =−3 + 3i, w =−3 − 3i, w =−7 .
Qn Solution
10(i) dq
[2] To find maximum q, = 0 , i.e. I = 0 .
dt
q
0+ = V
C
q = VC
10(ii) q
[2] RI + = V
C
Differentiate with respect to t,
dI 1 d q d V
R + =
dt C dt dt
dV
If V is a constant, i.e. =0
dt
dI 1
R + (I ) = 0
dt C
dI I dq
R = + 0, since
= I
dt C dt
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
14
Qn Solution
10(iii) dI I
[5] R + = 0
dt C
dI I
= −
dt RC
1 1
∫ I dI = ∫ − RC dt
1
ln I = − t+d
RC
1 1
− t +d − t
=I e= e RC
e
RC + d
1
− t
I = Ae RC
V
When
= t 0,=I
R
V
A=
R
1
V − RC t
∴I = e
R
10(iv) I
[2]
O t
10(v) As t → ∞ , I → 0 .
[1]
In the long run, the current in the circuit tends to/approaches 0 amp.
Qn Solution
11( Amount of money Ali paid at the end of 3 years
a) 36
= 2 ( 200 ) + ( 36 − 1)(10 )
[2] 2
= 13500
Amount Ali owes the bank at the end of 3 years
= 50000 − 13500
= 36500
11( At the end of 1 month, amount owed
=b) 1.003 ( 36500 ) − 900
(i)
= 35709.50
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
15
Qn Solution
[2]
At the end of 2 months, amount owed
= 1.003 ( 35709.50 ) − 900
= 34916.6285
At the end of 3 months, amount owed
= 1.003 ( 34916.6285 ) − 900
= 34121.38
= 34121 (nearest dollar)
11 Month Amount owed at the Amount owed at the
(b) start of month end of month
(ii) 1 1.003 ( 36500 ) 1.003 ( 36500 ) − 900
[5]
2 1.003 1.003 ( 36500 ) − 900 1.0032 ( 36500 ) − 1.003 ( 900 )
= 1.0032 ( 436500 ) − 1.003 ( 900 ) −900
3 1.003 1.0032 ( 36500 ) − 1.003 ( 900 ) − 900 1.0033 ( 36500 ) − 1.0032 ( 900 ) − 1.003 ( 900 )
= 1.0033 ( 36500 ) − 1.0032 ( 900 ) − 1.003 ( 900 ) −900
… … …
n 1.003n ( 36500 ) − 1.003n −1 ( 900 )
−1.003n − 2 ( 900 ) − ... − 900
On the last day of the nth month, Ali owed
1.003n ( 36500 ) − 1.003n −1 ( 900 ) − 1.003n − 2 ( 900 ) − ... − 900
= 1.003n ( 36500 ) − 900 + 1.003 ( 900 ) ... + 1.003n − 2 ( 900 ) + 1.003n −1 ( 900 )
= 1.003n ( 36500 ) − 900 (1 + 1.003 + ... + 1.003n − 2 + 1.003n −1 )
1.003n − 1
= 1.003n ( 36500 ) − 900
1.003 − 1
= 1.003n ( 36500 ) − 300000 (1.003n − 1)
When Ali pays off his study loan,
1.003n ( 36500 ) − 300000 (1.003n − 1) ≤ 0
Using GC,
n 1.003n ( 36500 ) − 300000 (1.003n − 1)
43 276.54
44 −622.6
45 −1524
Ali takes 44 months to pay off his study loan, i.e. he pays off his study loan in August
2027.
11( Method 1:
b) Since Ali pays $ (1.003 × 276.54 ) in the last month, total interest Ali paid
(iii)
=43 × 900 + (1.003 × 276.54 ) − 36500
[2]
= 2477.37 (nearest cent)
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
16
Qn Solution
Method 2:
Since Ali pays $276.54 in the last month, total amount he paid
= 13500 + 43 × 900 + (1.003 × 276.54 )
= 52477.37
Total amount of interest Ali paid
= 52477.37 − 50000
= 2477.37 (nearest cent)
11( Let the amount that Ali pays per month upon graduation be $x.
v) 1.00336 − 1
[3] 1.00336 ( 36500 ) − x ≤0
1.003 − 1
40656.16902 − 37.956 x ≤ 0
x ≥ 1071.14
Ali needs to pay $1072 per month.
© Millennia Institute 9758/01/PU3/Prelim/21 Solution
You might also like
- Qdoc - Tips Mathematics T For New STPM SyallabusDocument51 pagesQdoc - Tips Mathematics T For New STPM SyallabusFun Fun Tik TokNo ratings yet
- NYJC 2017 EOY Exam Paper Solutions 2Document12 pagesNYJC 2017 EOY Exam Paper Solutions 2Low Jia YingNo ratings yet
- KIS - KBAT 2016 - Form 4 KISDocument33 pagesKIS - KBAT 2016 - Form 4 KISHalipah AyetNo ratings yet
- 1 (A) Suppose The Following Facts Are Known About The Function G and Its DerivativeDocument5 pages1 (A) Suppose The Following Facts Are Known About The Function G and Its DerivativeShereen LimNo ratings yet
- QA20091 Em2 26 - 2Document4 pagesQA20091 Em2 26 - 2api-25895802No ratings yet
- Aporte 3 Tarea 3 DerivadasDocument3 pagesAporte 3 Tarea 3 DerivadasLaura Gómez CardonaNo ratings yet
- Aporte 3 Tarea 3 DerivadasDocument3 pagesAporte 3 Tarea 3 DerivadasLaura Gómez CardonaNo ratings yet
- Correccion Act 1 202351Document4 pagesCorreccion Act 1 202351MAYRA ALEJANDRA ELIZALDE RODRIGUEZNo ratings yet
- ListaS6 CalculoA 2020-2Document2 pagesListaS6 CalculoA 2020-2Yasmin SeloisNo ratings yet
- NewProgress - AMaths TB (Sol) - ch13Document1 pageNewProgress - AMaths TB (Sol) - ch13kkakilaiNo ratings yet
- Basic Mathematics-Theory-04 - BM Exercise SolDocument19 pagesBasic Mathematics-Theory-04 - BM Exercise SolRaju SinghNo ratings yet
- K Cet KeyDocument7 pagesK Cet KeypcmbacademicsNo ratings yet
- Mathematics 1B Semester Test 1 (V2) MemoDocument8 pagesMathematics 1B Semester Test 1 (V2) MemoLwando MankwaliNo ratings yet
- Solu H.M 2Document5 pagesSolu H.M 2Amro BaradiyyhaNo ratings yet
- Exercise 2.3 (Solutions) : y X X XDocument6 pagesExercise 2.3 (Solutions) : y X X XSpell MasterTMNo ratings yet
- © Science Exam Papers: Worked Solutions Edexcel C3 Paper IDocument3 pages© Science Exam Papers: Worked Solutions Edexcel C3 Paper I유리No ratings yet
- A-Level H2 Maths 2017 - Paper 1: A A Ax A X Aa X ADocument17 pagesA-Level H2 Maths 2017 - Paper 1: A A Ax A X Aa X AterrygohNo ratings yet
- Aod Sheet 4 MCQ (BMDP) With Answers - 072741Document10 pagesAod Sheet 4 MCQ (BMDP) With Answers - 072741arnavnagar1608No ratings yet
- Chapter 40 - By-KamalDocument8 pagesChapter 40 - By-Kamalقاسم علاوNo ratings yet
- 2020Document24 pages2020vipin yadavNo ratings yet
- MAT 1300 Midterm 2 Booklet Solutions PDFDocument41 pagesMAT 1300 Midterm 2 Booklet Solutions PDFdongmianjunNo ratings yet
- 2701 EVE JEE-Main January 2024 MPC G5jripDocument24 pages2701 EVE JEE-Main January 2024 MPC G5jripkiran091950No ratings yet
- Tut 5Document2 pagesTut 5Harshvardhan SinghNo ratings yet
- Mt129 Name IdDocument16 pagesMt129 Name IdEe BNo ratings yet
- Revisão de Funções e ComposiçõesDocument2 pagesRevisão de Funções e Composiçõesmarinaalmondes16No ratings yet
- Ace Analysis Additional Mathematics SPM - SPM Practice (Form 4, Chapter 1, Pg. 1-4) IDocument4 pagesAce Analysis Additional Mathematics SPM - SPM Practice (Form 4, Chapter 1, Pg. 1-4) I钰颖No ratings yet
- TJC 9758 2023 Prelim P1 SolutionDocument20 pagesTJC 9758 2023 Prelim P1 SolutionSierra KohNo ratings yet
- Quiz3 140Document1 pageQuiz3 140Franchezca WestermannNo ratings yet
- January 2022 ExamDocument6 pagesJanuary 2022 ExamjidsjijsdwdNo ratings yet
- Methods of Differentiation - DPP 01 (Lec of 03) - Lakshya JEE 2024Document2 pagesMethods of Differentiation - DPP 01 (Lec of 03) - Lakshya JEE 2024sahil 1909No ratings yet
- Worksheet - 1 Newton-Leibnitz FormulaDocument10 pagesWorksheet - 1 Newton-Leibnitz FormulaChetanNo ratings yet
- Cal1Mid 1112 Sem2Document4 pagesCal1Mid 1112 Sem2Khánh My Đỗ BùiNo ratings yet
- 37 - PDFsam - 01 رياضيات 1-بDocument2 pages37 - PDFsam - 01 رياضيات 1-بMina Albert100% (1)
- Ans and Sol Jee Main 2022 Phase 1-24-06 2022 e Math FinalDocument8 pagesAns and Sol Jee Main 2022 Phase 1-24-06 2022 e Math FinalVadivel KmNo ratings yet
- Exercise 1.2 ComplexDocument3 pagesExercise 1.2 ComplexDana Rashed.No ratings yet
- Review 3Document2 pagesReview 3mgs911No ratings yet
- 2023 MAV MathsMethods Trial Exam 1 SolutionsDocument10 pages2023 MAV MathsMethods Trial Exam 1 Solutionsmiddoka101No ratings yet
- 63 - PDFsam - 01 رياضيات 1-بDocument2 pages63 - PDFsam - 01 رياضيات 1-بMina AlbertNo ratings yet
- NSGHS 2010 2U Trial SolutionsDocument11 pagesNSGHS 2010 2U Trial SolutionsYon Seo YooNo ratings yet
- Calculo 1 Semana 4 IaccDocument6 pagesCalculo 1 Semana 4 Iaccangel navarroNo ratings yet
- Original Problem Series Week-2: WWM MathsDocument8 pagesOriginal Problem Series Week-2: WWM MathspiyushNo ratings yet
- Tut 2Document3 pagesTut 2Harshvardhan SinghNo ratings yet
- JEE-MAIN-MATHS MODULE-1(Solution)Document17 pagesJEE-MAIN-MATHS MODULE-1(Solution)emogirlslovewineNo ratings yet
- T324 Test 1 Mathematics N5 Memo 2024Document6 pagesT324 Test 1 Mathematics N5 Memo 2024johannesthibakhoaneNo ratings yet
- M1M2搶分技巧班2022-2023 ANS_v2Document28 pagesM1M2搶分技巧班2022-2023 ANS_v2conniechoympNo ratings yet
- CW1020_6_2024_solDocument5 pagesCW1020_6_2024_sollintaoliu114No ratings yet
- 12 CHAP8-KEY2,3,5 IMP QUESDocument13 pages12 CHAP8-KEY2,3,5 IMP QUESvasanthsm83No ratings yet
- EMTH 201-Tutorial 3-4-9-2023Document2 pagesEMTH 201-Tutorial 3-4-9-2023oatsmartinNo ratings yet
- Notes Important Questions Answers 12th Math Chapter 3 Exercise 3.7Document5 pagesNotes Important Questions Answers 12th Math Chapter 3 Exercise 3.7shahidNo ratings yet
- Niversidad Acional DE Ngenieria: F I MecanicaDocument9 pagesNiversidad Acional DE Ngenieria: F I MecanicaDAVIS106No ratings yet
- 1 Minor Adv Answer KeyDocument6 pages1 Minor Adv Answer Keyparideshmukh2300No ratings yet
- Integral Calculus: Indefinite Integral Page No. 730Document7 pagesIntegral Calculus: Indefinite Integral Page No. 730Dipon AminNo ratings yet
- 微乙上末 99Document7 pages微乙上末 99Bryan WLNo ratings yet
- Math 1013 (1B) REVIEW 2024Document12 pagesMath 1013 (1B) REVIEW 2024abhilakshay2002No ratings yet
- Resolver Los 5 EjerciciosDocument1 pageResolver Los 5 EjerciciosIvan VegaNo ratings yet
- Zadatak 6.: Izra Cunaj Derivacije Sljede Cih Funkcija U To Cki X:, XDocument1 pageZadatak 6.: Izra Cunaj Derivacije Sljede Cih Funkcija U To Cki X:, XMayaNo ratings yet
- Ex7.5 Que6,12,18Document2 pagesEx7.5 Que6,12,18bdjsjdnxnsjNo ratings yet
- Calculus Test12 Sol 20191015Document7 pagesCalculus Test12 Sol 20191015Mạc Hải LongNo ratings yet
- Factoring and Algebra - A Selection of Classic Mathematical Articles Containing Examples and Exercises on the Subject of Algebra (Mathematics Series)From EverandFactoring and Algebra - A Selection of Classic Mathematical Articles Containing Examples and Exercises on the Subject of Algebra (Mathematics Series)No ratings yet
- Dolphin TrainerDocument3 pagesDolphin TrainerCláudia PinheiroNo ratings yet
- Chapter 5 Worked SolutionsDocument279 pagesChapter 5 Worked Solutionscormac molloyNo ratings yet
- Class 11 Inequalities, Modulus and LogarithmDocument3 pagesClass 11 Inequalities, Modulus and Logarithmkwonswara100% (1)
- Current ResumeDocument2 pagesCurrent Resumeapi-357130373No ratings yet
- 3d Pythagoras pdf2Document2 pages3d Pythagoras pdf2Manikandan GeethaNo ratings yet
- IIT JEE (Maths Test Paper) : Dhruv ClassesDocument4 pagesIIT JEE (Maths Test Paper) : Dhruv ClassesAmit GuptaNo ratings yet
- IIT JEE Coordinate Geometry - Preparation Tips To Practical Applications! - askIITiansDocument19 pagesIIT JEE Coordinate Geometry - Preparation Tips To Practical Applications! - askIITiansaskiitian67% (3)
- Sims 2 Private School HomeworkDocument7 pagesSims 2 Private School Homeworkg663q32m100% (1)
- Homework: Vampires Don T Wear Polka DotsDocument4 pagesHomework: Vampires Don T Wear Polka DotsAlejandro ParraNo ratings yet
- NEWS81Document3 pagesNEWS81aliscribd46No ratings yet
- Craig Lichtenberg - Resume ONLINE - 2010Document2 pagesCraig Lichtenberg - Resume ONLINE - 2010CraigLichtenbergNo ratings yet
- 3.2 Angles and TrianglesDocument9 pages3.2 Angles and TrianglesAlbert Thaw TunNo ratings yet
- Unit 5 Properties of Triangles AnswersDocument14 pagesUnit 5 Properties of Triangles AnswersGehan FaroukNo ratings yet
- Q WGRR Gwfoj 1 W 8 B O8 AHfzDocument198 pagesQ WGRR Gwfoj 1 W 8 B O8 AHfzdrjbjpNo ratings yet
- Proof OneDocument15 pagesProof Oneapi-253901565No ratings yet
- 8 Closed Compass V2docDocument8 pages8 Closed Compass V2docCarlo Miguel MagalitNo ratings yet
- Term 2 Revision Set 2: What Is The Name of Line Segment AB On The Circle Below? A. Diameter B. Radius C. Chord D. ArcDocument6 pagesTerm 2 Revision Set 2: What Is The Name of Line Segment AB On The Circle Below? A. Diameter B. Radius C. Chord D. ArcPLASMAASIDNo ratings yet
- Jab Saara India Vedantu Pe Online Padhega: Scholarship Admission TestDocument32 pagesJab Saara India Vedantu Pe Online Padhega: Scholarship Admission Testsudheer shakyaNo ratings yet
- Topic01 Real NumbersDocument11 pagesTopic01 Real NumbersAlexandra Regina MoralesNo ratings yet
- AJ Sadler Specialist Unit 3 CH 5 Worked SolutionsDocument44 pagesAJ Sadler Specialist Unit 3 CH 5 Worked SolutionsjamieharrisonbusinessNo ratings yet
- Concept Context e ContentDocument0 pagesConcept Context e ContentOvidiu MunteanuNo ratings yet
- Complete Pet Unit 2 TestDocument6 pagesComplete Pet Unit 2 TestThu Hằng TrầnNo ratings yet
- A Liouville-Type Theorem For The Equation of Special Lagrangian ManifoldsDocument4 pagesA Liouville-Type Theorem For The Equation of Special Lagrangian ManifoldsmyminumishNo ratings yet
- Accomplishment Report in CRDocument2 pagesAccomplishment Report in CRNorma DoinogNo ratings yet
- How To Calculate Volumes of Partially Full TanksDocument8 pagesHow To Calculate Volumes of Partially Full TanksAnonymous uCYIu1No ratings yet
- Screenshot 2025-01-07 at 11.34.54 PMDocument7 pagesScreenshot 2025-01-07 at 11.34.54 PMthindshubhneet98No ratings yet
- Kathryn Ming Resume 2016 1Document2 pagesKathryn Ming Resume 2016 1api-278731011No ratings yet
- Year 9 MATHEMATICSDocument153 pagesYear 9 MATHEMATICSCindy InternetNo ratings yet
- GEC 210 Lecture Note 6 Series-1Document32 pagesGEC 210 Lecture Note 6 Series-1Ifiok UsoroNo ratings yet