Matrices

Matrix is a rectangular array of numbers, symbols, points, or characters each belonging to a specific row and column. A matrix is identified by its order which is given in the form of rows ⨯ and columns. The numbers, symbols, points, or characters present inside a matrix are called the elements of a matrix. The location of each element is given by the row and column it belongs to.

Matrices are important for students of class 12 and are also of great importance in engineering mathematics. In this introductory article on matrices, we will learn about the types of matrices, the transpose of matrices, the rank of matrices, the adjoint and inverse of matrices, the determinants of matrices, and many more in detail.

What are Matrices?

Matrices are rectangular arrays of numbers, symbols, or characters where all of these elements are arranged in each row and column. An array is a collection of items arranged at different locations.

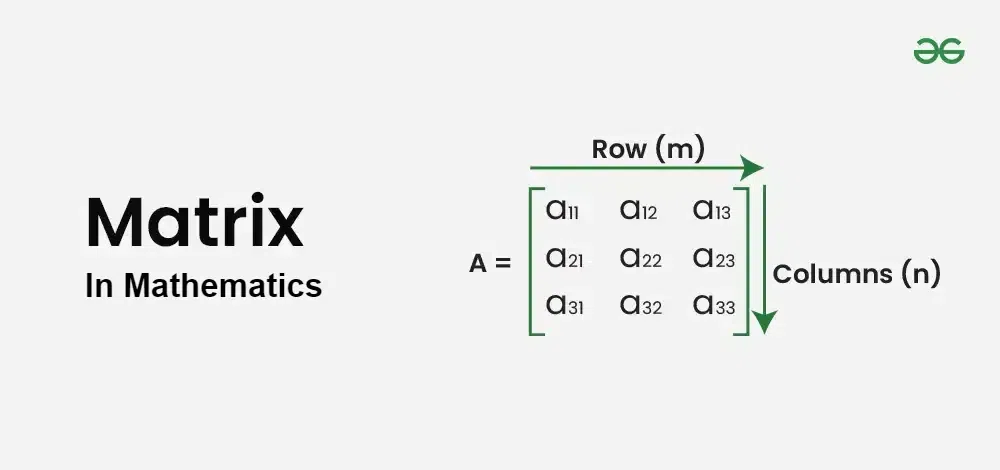
Let’s assume points are arranged in space each belonging to a specific location then an array of points is formed. This array of points is called a matrix. The items contained in a matrix are called Elements of the Matrix. Each matrix has a finite number of rows and columns and each element belongs to these rows and columns only. The number of rows and columns present in a matrix determines the order of the matrix. Let’s say a matrix has 3 rows and 2 columns then the order of the matrix is given as 3⨯2.

Matrices Definition

A rectangular array of numbers, symbols, or characters is called a Matrix. Matrices are identified by their order. The order of the matrices is given in the form of a number of rows ⨯ number of columns. A matrix is represented as [P]_m⨯n where P is the matrix, m is the number of rows and n is the number of columns. Matrices in maths are useful in solving numerous problems of linear equations and many more.

Order of Matrix

Order of a Matrix tells about the number of rows and columns present in a matrix. Order of a matrix is represented as the number of rows times the number of columns. Let’s say if a matrix has 4 rows and 5 columns then the order of the matrix will be 4⨯5. Always remember that the first number in the order signifies the number of rows present in the matrix and the second number signifies the number of columns in the matrix.

Matrices Examples

Examples of matrices are mentioned below:

Example: [Tex]\begin{bmatrix} 1 & 2 \\ 3 &4 \\ \end{bmatrix}_{2\times 2}[/Tex], [Tex]\begin{bmatrix} 1 & -1 & 2 \\ 3 & 2 & 6 \\ 4 & -2& 5\\\end{bmatrix}_{3 \times3}[/Tex]

Operation on Matrices

Matrices undergo various mathematical operations such as addition, subtraction, scalar multiplication, and multiplication. These operations are performed between the elements of two matrices to give an equivalent matrix that contains the elements which are obtained as a result of the operation between elements of two matrices. Let’s learn the operation of matrices.

Addition of Matrices

In addition of matrices, the elements of two matrices are added to yield a matrix that contains elements obtained as the sum of two matrices. The addition of matrices is performed between two matrices of the same order.

Example: Find the sum of [Tex]\bold{\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}}[/Tex]and [Tex]\bold{\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix}}[/Tex]

Solution:

Here, we have A = [Tex]\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}[/Tex]and B = [Tex]\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix} [/Tex]

A + B = [Tex]\begin{bmatrix} 1& 2\\ 4& 5\\ \end{bmatrix}[/Tex]+ [Tex]\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix} [/Tex]

⇒ A + B = [Tex]\begin{bmatrix} 1 + 2 & 2 + 3\\ 4 + 6& 5 + 7\\ \end{bmatrix}[/Tex]= [Tex]\begin{bmatrix} 3 & 5\\ 10& 12\\ \end{bmatrix} [/Tex]

Subtraction of Matrices

Subtraction of Matrices is the difference between the elements of two matrices of the same order to give an equivalent matrix of the same order whose elements are equal to the difference of elements of two matrices. The subtraction of two matrices can be represented in terms of the addition of two matrices. Let’s say we have to subtract matrix B from matrix A then we can write A – B. We can also rewrite it as A + (-B). Let’s solve an example

Example: Subtract [Tex]\bold{\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}}[/Tex]from [Tex]\bold{\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix} }[/Tex].

Let us assume A = [Tex]\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix}[/Tex]and B = [Tex]\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix} [/Tex]

A – B = [Tex]\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix}[/Tex]– [Tex]\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix} [/Tex]

⇒ A – B = [Tex]\begin{bmatrix} 2 – 1 & 3 – 2 \\ 6 – 4 & 7 – 5 \\ \end{bmatrix}[/Tex]= [Tex]\begin{bmatrix} 1 & 1 \\ 2 & 2 \\ \end{bmatrix}[/Tex]

Scalar Multiplication of Matrices

Scalar Multiplication of matrices refers to the multiplication of each term of a matrix with a scalar term. If a scalar let’s ‘k’ is multiplied by a matrix then the equivalent matrix will contain elements equal to the product of the scalar and the element of the original matrix. Let’s see an example:

Example: Multiply 3 with [Tex]\bold{\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}}[/Tex].

3[A] = [Tex]\begin{bmatrix} 3\times1 & 3\times 2\\ 3\times4& 3\times5 \\ \end{bmatrix}[/Tex]

⇒ 3[A] = [Tex]\begin{bmatrix} 3 & 6\\ 12& 15 \\ \end{bmatrix}[/Tex]

Multiplication of Matrices

In the multiplication of matrices, two matrices are multiplied to yield a single equivalent matrix. The multiplication is performed in the manner that the elements of the row of the first matrix multiply with the elements of the columns of the second matrix and the product of elements are added to yield a single element of the equivalent matrix. If a matrix [A]_i⨯j is multiplied with matrix [B]_j⨯k then the product is given as [AB]_i⨯k.

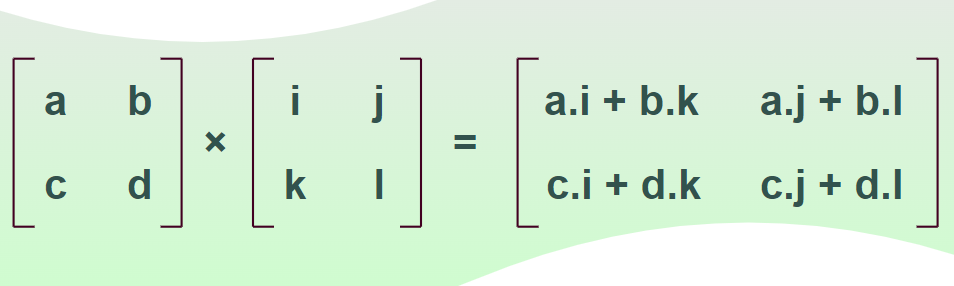
Let’s see an example.

Example: Find the product of [Tex]\bold{\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}}[/Tex]and [Tex]\bold{\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix}}[/Tex]

Solution:

Let A = [Tex]\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}[/Tex]and B = [Tex]\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix}[/Tex]

⇒ AB = [Tex]\begin{bmatrix} 1 & 2\\ 4& 5 \\ \end{bmatrix}[/Tex][Tex]\begin{bmatrix} 2 & 3 \\ 6 & 7 \\ \end{bmatrix}[/Tex]

⇒ AB = [Tex]\begin{bmatrix} 1\times2+2\times6 & 1\times3+2\times7\\ 4\times2+5\times6& 4\times3+5\times7 \\ \end{bmatrix}[/Tex]

⇒ [Tex]AB = \begin{bmatrix} 14 & 17\\ 38& 47 \\ \end{bmatrix}[/Tex]

Properties of Matrix Addition and Multiplication

Properties followed by Multiplication and Addition of Matrices is listed below:

• A + B = B + A (Commutative)
• (A + B) + C = A + (B + C) (Associative)
• AB ≠ BA (Not Commutative)
• (AB) C = A (BC) (Associative)
• A (B+C) = AB + AC (Distributive)

Transpose of Matrix

Transpose of Matrix is basically the rearrangement of row elements in column and column elements in a row to yield an equivalent matrix. A matrix in which the elements of the row of the original matrix are arranged in columns or vice versa is called Transpose Matrix. The transpose matrix is represented as A^T. if A = [a_ij]_mxn , then A^T = [b_ij]_nxm where b_ij = a_ji.

Let’s see an example:

Example: Find the transpose of [Tex]\begin{bmatrix} 18 & 17\\ 38& 47 \\ \end{bmatrix}  [/Tex].

Solution:

Let A = [Tex]\begin{bmatrix} 18 & 17\\ 38& 47 \\ \end{bmatrix}[/Tex]

⇒ A^T = [Tex]\begin{bmatrix} 18 & 38\\ 17& 47 \\ \end{bmatrix}[/Tex]

Properties of the Transpose of a Matrix

Properties of the transpose of a matrix are mentioned below:

• (A^T)^T = A
• (A+B)^T = A^T + B^T
• (AB)^T = B^TA^T

Trace of Matrix

Trace of a Matrix is the sum of the principal diagonal elements of a square matrix. Trace of a matrix is only found in the case of a square matrix because diagonal elements exist only in square matrices. Let’s see an example.

Example: Find the trace of the matrix [Tex]\begin{bmatrix} 1 & 2 & 3\\ 4 & 5 & 6\\ 7 & 8 & 9 \end{bmatrix}        [/Tex]

Solution:

Let us assume A = [Tex]\begin{bmatrix} 1 & 2 & 3\\ 4 & 5 & 6\\ 7 & 8 & 9 \end{bmatrix} [/Tex]

Trace(A) = 1 + 5 + 9 = 15

Types of Matrices

Based on the number of rows and columns present and the special characteristics shown, matrices are classified into various types.

• Row Matrix: A Matrix in which there is only one row and no column is called Row Matrix.
• Column Matrix: A Matrix in which there is only one column and now row is called a Column Matrix.
• Horizontal Matrix: A Matrix in which the number of rows is less than the number of columns is called a Horizontal Matrix.
• Vertical Matrix: A Matrix in which the number of columns is less than the number of rows is called a Vertical Matrix.
• Rectangular Matrix: A Matrix in which the number of rows and columns are unequal is called a Rectangular Matrix.
• Square Matrix: A matrix in which the number of rows and columns are the same is called a Square Matrix.
• Diagonal Matrix: A square matrix in which the non-diagonal elements are zero is called a Diagonal Matrix.
• Zero or Null Matrix: A matrix whose all elements are zero is called a Zero Matrix. A zero matrix is also called as Null Matrix.
• Unit or Identity Matrix: A diagonal matrix whose all diagonal elements are 1 is called a Unit Matrix. A unit matrix is also called an Identity matrix. An identity matrix is represented by I. 
• Symmetric matrix: A square matrix is said to be symmetric if the transpose of the original matrix is equal to its original matrix. i.e. (A^T) = A. 
• Skew-symmetric Matrix: A skew-symmetric (or antisymmetric or antimetric[1]) matrix is a square matrix whose transpose equals its negative i.e. (A^T) = -A. 
• Orthogonal Matrix: A matrix is said to be orthogonal if AA^T = A^TA = I 
• Idempotent Matrix: A matrix is said to be idempotent if A² = A 
• Involutory Matrix: A matrix is said to be Involutory if A² = I. 
• Upper Triangular Matrix: A square matrix in which all the elements below the diagonal are zero is known as the upper triangular matrix
• Lower Triangular Matrix: A square matrix in which all the elements above the diagonal are zero is known as the lower triangular matrix
• Singular Matrix: A square matrix is said to be a singular matrix if its determinant is zero i.e. |A|=0
• Nonsingular Matrix: A square matrix is said to be a non-singular matrix if its determinant is non-zero.

Note: Every Square Matrix can uniquely be expressed as the sum of a symmetric matrix and a skew-symmetric matrix. A = 1/2 (A^T + A) + 1/2 (A – A^T). 

Learn More, Types of Matrices

Determinant of a Matrix

Determinant of a matrix is a number associated with that square matrix. The determinant of a matrix can only be calculated for a square matrix. It is represented by |A|. The determinant of a matrix is calculated by adding the product of the elements of a matrix with their cofactors.

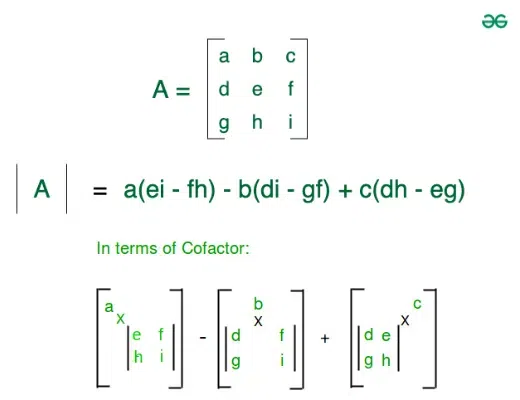
Determinant of a Matrix

Let’s see how to find the determinant of a square matrix.

Example 1: How to find the determinant of a 2⨯2 square matrix?

Let say we have matrix A = [Tex]\begin{bmatrix} a & b \\ c & d \end{bmatrix}[/Tex]

Then, determinant is of A is |A| = ad – bc

Example 2: How to find the determinant of a 3⨯3 square matrix?

Let’s say we have a 3⨯3 matrix A = [Tex]\begin{bmatrix} a & b& c \\ d & e & f \\ g & h &i \\ \end{bmatrix}[/Tex]

Then |A| = a(-1)¹⁺¹[Tex]\begin{vmatrix} e& f \\ h & i\\ \end{vmatrix}[/Tex]+ b(-1)¹⁺²[Tex]\begin{vmatrix} d& f \\ g & i\\ \end{vmatrix}[/Tex] + c(-1)¹⁺³[Tex]\begin{vmatrix} d& e \\ g & h\\ \end{vmatrix}[/Tex]

Minor of a Matrix

Minor of a matrix for an element is given by the determinant of a matrix obtained after deleting the row and column to which the particular element belongs to. Minor of Matrix is represented by Mij. Let’s see an example.

Example: Find the minor of the matrix [Tex]\begin{bmatrix} a & b& c \\ d & e & f \\ g & h &i \\ \end{bmatrix}[/Tex]for the element ‘a’.

Minor of element ‘a’ is given as M₁₁ = [Tex]\begin{vmatrix} e& f \\ h & i\\ \end{vmatrix}[/Tex]

Cofactor of Matrix

Cofactor of a matrix is found by multiplying the minor of the matrix for a given element by (-1)i+j. Cofactor of a Matrix is represented as Cij. Hence, the relation between the minor and cofactor of a matrix is given as Mij = (-1)^i+jMij. If we arrange all the cofactor obtained for an element then we get a cofactor matrix given as C = [Tex]\begin{bmatrix} c_{11} & c_{12}& c_{13} \\ c_{21} & c_{22} & c_{23} \\ c_{31} & c_{32} &c_{33} \\ \end{bmatrix}[/Tex]

Learn More, Minors and Cofactors

Adjoint of a Matrix

Adjoint is calculated for a square matrix. Adjoint of a matrix is the transpose of the cofactor of the matrix. The Adjoint of a Matrix is thus expressed as adj(A) = C_T where C is the Cofactor Matrix.

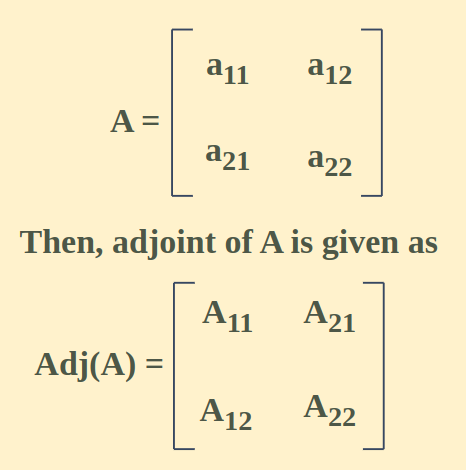
Let’s say for example we have matrix
[Tex]A = \begin{bmatrix} a_1 & b_1 & c_1\\ a_2 & b_2 & c_2\\ a_3 & b_3 & c_3 \end{bmatrix}[/Tex]
 then 
[Tex]\mathrm{adj(A)} = \begin{bmatrix} A_1 & B_1 & C_1\\ A_2 & B_2 & C_2\\ A_3 & B_3 & C_3 \end{bmatrix}^T \\ \Rightarrow \mathrm{adj(A)} =\begin{bmatrix} A_1 & A_2 & A_3\\ B_1 & B_2 & B_3\\ C_1 & C_2 & C_3 \end{bmatrix}[/Tex]
where, 
[Tex]\begin{bmatrix} A_1 & B_1 & C_1\\ A_2 & B_2 & C_2\\ A_3 & B_3 & C_3 \end{bmatrix}[/Tex]is cofactor of Matrix A.

Properties of Adjoint of Matrix

Properties of the Adjoint of a matrix are mentioned below:

• A(Adj A) = (Adj A) A = |A| I_n
• Adj(AB) = (Adj B) . (Adj A)
• |Adj A| = |A|^n-1
• Adj(kA) = k^n-1 Adj(A)
• |adj(adj(A))| = [Tex]|A| ^ (n-1) ^ 2 [/Tex]
• adj(adj(A)) = |A|^(n-2) × A
• If A = [L,M,N] then adj(A) = [MN, LN, LM]
• adj(I) = I {where I is Identity Matrix}

Where, “n = number of rows = number of columns”

Inverse of a Matrix

A matrix is said to be an inverse of matrix ‘A’ if the matrix is raised to power -1 i.e. A-1. The inverse is only calculated for a square matrix whose determinant is non-zero. The formula for the inverse of a matrix is given as:

A^-1 = adj(A)/det(A) = (1/|A|)(Adj A), where |A| should not be equal to zero, which means matrix A should be non-singular. 

Properties Inverse of Matrix

• (A^-1)^-1 = A 
• (AB)^-1 = B^-1A^-1 
• only a non-singular square matrix can have an inverse. 

Elementary Operation on Matrices

Elementary Operations on Matrices are performed to solve the linear equation and to find the inverse of a matrix. Elementary operations are between rows and between columns. There are three types of elementary operations performed for rows and columns. These operations are mentioned below:

Elementary operations on rows include:

• Interchanging two rows
• Multiplying a row by a non-zero number
• Adding two rows

Elementary operations on columns include:

• Interchanging two columns
• Multiplying a column by a non-zero number
• Adding two columns

Augmented Matrix

A matrix formed by combining columns of two matrices is called Augmented Matrix. An augmented matrix is used to perform elementary row operations, solve a linear equation, and find the inverse of a matrix. Let us understand through an example.

Let’s say we have a matrix A = [Tex]\begin{bmatrix} a_1 & b_1 & c_1\\ a_2 & b_2 & c_2\\ a_3 & b_3 & c_3 \end{bmatrix}[/Tex], X = [Tex]\begin{bmatrix} x \\ y \\z \end{bmatrix}[/Tex]and B = [Tex]\begin{bmatrix} p_{1} \\ p_{2} \\p_{3} \end{bmatrix}[/Tex]then augmented matrix is formed between A and B. The augmented matrix for A and B is given as

[A|B] = [Tex]\left[\begin{array}{lll|l} a_1 & b_1 & c_1&p_1\\ a_2 & b_2 & c_2&p_2\\ a_3 & b_3 & c_3 &p_3\end{array}\right][/Tex]

Solving Linear Equation Using Matrices

Matrices are used to solve linear equations. To solve linear equations we need to make three matrices. The first matrix is of coefficients, the second matrix is of variables and the third matrix is of constants. Let’s understand it through an example.

Let’s say we have two equations given as a₁x + b₁y = c₁ and a₂x + b₂y = c₂. In this case, we will form the first matrix of coefficient let’s say A = [Tex]\begin{bmatrix}a_{1} & b_{1}\\a_{2} & b_{2}\end{bmatrix}[/Tex], the second matrix is of variables let’s say X = [Tex]\begin{bmatrix}x\\y\end{bmatrix}[/Tex]and the third matrix is of coefficient B = [Tex]\begin{bmatrix}c_{1}\\c_{2}\end{bmatrix}[/Tex]then the matrix equation is given as

AX = B

⇒ X = A^-1B

where,

• A is Coefficient Matrix
• X is Variable Matrix
• B is Constant Matrix

Hence we can see that the value of variable X can be calculated by multiplying the inverse of matrix A with B and then equalizing the equivalent product of two matrices with matrix X.

Rank of a Matrix

Rank of Matrix is given by the maximum number of linearly independent rows or columns of a matrix. The rank of a matrix is always less than or equal to the total number of rows or columns present in a matrix. A square matrix has linearly independent rows or columns if the matrix is non-singular i.e. determinant is not equal to zero. Since a zero matrix has no linearly independent rows or columns its rank is zero.

Rank of a matrix can be calculated by converting the matrix into Row-Echelon Form. In row echelon form we try to convert all the elements belonging to a row to be zero using Elementary Opeartion on Row. After the operation, the total number of rows which has at least one non-zero element is the rank of the matrix. The rank of the matrix A is represented by ρ(A).

Eigen Value and Eigen Vectors of Matrices

Eigen Values are the set of scalar associated with the linear equation in matrix form. Eigenvalues are also called characteristic roots of the matrices. The vectors that are formed by using the eigenvalue to tell the direction at that points are called Eigenvectors. Eigenvalues change the magnitude of eigenvectors. Like any vector, Eigenvector doesn’t change with linear transformation.

For a Square Matrix A of order ‘n’ another square matrix A – λI is formed of the same order, where I is the Identity Matrix and λ is the eigenvalue. The eigenvalue λ satisfies an equation Av = λv where v is a non-zero vector.

Learn more about Eigenvalues and Eigenvectors at our website.

Matrices Formulas

The basic formula for the matrices has been discussed below:

• A^-1 = adj(A)/|A|
• A(adj A) = (adj A)A = I, where I is an Identity Matrix
• |adj A| = |A|n-1 where n is the order of matrix A
• adj(adj A) = |A|n-2A where n is the order of the matrix
• |adj(adj A)| = |A|^{(n-1)^2}
• adj(AB) = (adj B)(adj A)
• adj(A^p) = (adj A)^p
• adj(kA) = kn-1(adj A) where k is any real number
• adj(I) = I
• adj 0 = 0
• If A is symmetric then adj(A) is also symmetric
• If A is a diagonal Matrix then adj(A) is also a diagonal matrix
• If A is a triangular matrix then adj(A) is also a triangular matrix
• If A is a singular Matrix then |adj A| = 0
• (AB)^-1 = B^-1A^-1

Read More:

• Set Theory
• Calculus
• Trigonometry

Unsolved Problems

Problem 1: Find the sum of matrices A and B where A = [[3, 4], [1, 2]] and B = [[5, 6], [7, 8]].

Problem 2: Subtract matrix B from matrix A where A = [[6, 5], [4, 3]] and B = [[2, 1], [0, 9]].

Problem 3: Multiply matrices A and B where A = [[1, 0], [0, 1]] and B = [[2, 3], [4, 5]].

Problem 4: Find the determinant of matrix A where A = [[7, 5], [3, 2]].

Problem 5: Calculate the transpose of matrix A where A = [[1, 2, 3], [4, 5, 6], [7, 8, 9]].

Problem 6: Find the inverse of matrix A where A = [[2, 5], [1, 3]].

Problem 7: Determine if matrix A is symmetric where A = [[2, 3, 4], [3, 5, 6], [4, 6, 8]].

Problem 8: Find the eigenvalues of matrix A where A = [[3, 1], [0, 2]].

Problem 9: Find the rank of matrix A where A = [[2, 4, 6], [1, 2, 3], [0, 0, 0]].

Problem 10: Find the trace of matrix A where A = [[4, 2, 1], [0, 3, 5], [6, 7, 8]].

Summary

These 10 unsolved matrix problems cover fundamental operations such as matrix addition, subtraction, multiplication, determinant calculation, transpose, inversion, symmetry check, eigenvalue determination, rank calculation, and trace calculation. The problems are designed to reinforce understanding of key matrix concepts, providing practice in matrix manipulation and analysis.

Matrices – FAQs

What is Matrix in Math?

Matrices in maths are rectangular array arrangements of numbers or variables which are located in specific rows and columns and undergo various operations.

How to solve Matrices?

We solve matrices for different operations such as addition, subtraction, multiplication, transpose etc. These methods are discussed under the title Operations on Matrices.

What are the different Types of Matrices?

The different types of matrices are, row matrix, column matrix, horizontal matrix, vertical matrix, square matrix, diagonal matrix, null matrix, identity matrix, triangular matrices, symmetric and skew symmetric matrices, hermitian and skew hermitian matrices etc. These types have been discussed under the title ‘Types of Matrices’

What is Rank of a Matrix?

The rank of a matrix is the number of linearly independent rows or columns present in a matrix.

What is the Transpose of a Matrix?

The Transpose of a matrix is the rearrangement of elements of rows into columns and vice versa.

What is the Formula to find the Inverse of a Matrix?

The inverse of the matrix can be find out using the formula A^-1 = (1/|A|)(adj A)

What is the Condition to Multiply two Matrices?

Two matrices can be multiplied only if the number of columns of the first matrix is equal to the number of rows of the second matrix.

How to Find Determinant of 2⨯2 Matrix?

The determinant of a 2⨯2 Matrix can be find by subtracting the product of diagonal elements of the matrix.

What is the principal diagonal of a matrix?

The diagonal of a square matrix running from the upper left entities to the lower right entities are principal diagonal of a matrix.

GeeksforGeeks

Improve