Raft Design
Raft Design
Uploaded by
ramesh_madkatte1082Copyright:
Available Formats
Raft Design
Raft Design
Uploaded by
ramesh_madkatte1082Original Description:
Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
Raft Design
Raft Design
Uploaded by
ramesh_madkatte1082Copyright:
Available Formats
Example 12.
7 Raft Foundation
Design a raft foundation for the layout of columns shown in Figure 12.C.19(a). All columns are
of square shape of size 40 40 cm. ADSP80 kN/m
3
. Use M 15 concrete and Fe 415 steel.
Assume 10% as the load of raft and soil above.
A. Design of Raft Slab
Total vertical column load 600 1600 2000 600 800 1800 2000
1000 800 1000 1200 600 14000
Eccentricity along the x direction is obtained by taking moment of column loads about the
grid 11
x
61600 1800 1000 122000 2000 1200 18600 1000 600
14000
9:1714 m
e
x
9:17146 3 0:1714 m
Eccentricity along the y direction is obtained by taking moment of column loads about the
grid CC
7000 6000 5000
(c)
(d)
4000 3000 2000 1000 0
750
500
250
0
-250
-500
-750
S
h
e
a
r
f
o
r
c
e
(
i
n
k
N
)
7000 6000 5000 4000 3000 2000 1000 0
250
200
150
100
50
0
B
e
a
r
i
n
g
p
r
e
s
s
u
r
e
(
i
n
k
N
/
m
2
)
Figure 12.C.18 (Continued )
570 Foundation Design
T
a
b
l
e
1
2
.
C
.
7
C
o
m
p
a
r
a
t
i
v
e
s
t
u
d
y
(
s
e
e
c
o
m
m
e
n
t
s
i
n
S
e
c
t
i
o
n
1
2
.
C
.
2
)
.
M
a
x
i
m
u
m
b
e
a
r
i
n
g
p
r
e
s
s
u
r
e
(
k
N
/
m
2
)
M
a
x
i
m
u
m
b
e
n
d
i
n
g
m
o
m
e
n
t
(
k
N
m
)
M
a
x
i
m
u
m
s
h
e
a
r
f
o
r
c
e
(
k
N
)
M
a
x
i
m
u
m
d
e
e
c
t
i
o
n
(
m
m
)
C
o
n
v
e
n
t
i
o
n
a
l
d
e
s
i
g
n
W
i
t
h
f
a
c
t
o
r
e
d
l
o
a
d
6
2
4
.
3
2
3
9
8
7
.
3
2
3
1
0
3
2
.
2
0
6
2
4
.
3
2
3
/
2
8
1
8
.
0
8
W
i
t
h
d
e
s
i
g
n
l
o
a
d
6
2
4
.
3
2
3
/
1
.
5
4
1
6
.
2
2
9
8
7
.
3
2
3
/
1
.
5
6
5
8
.
2
2
1
0
3
2
.
2
0
/
1
.
5
6
8
8
.
1
3
1
8
.
0
8
/
1
.
5
1
2
.
0
5
D
e
s
i
g
n
u
s
i
n
g
B
E
F
(
w
i
t
h
d
e
s
i
g
n
l
o
a
d
o
n
l
y
)
2
0
5
.
0
5
0
k
N
/
m
2
a
t
x
0
m
m
4
4
0
.
5
7
6
k
N
m
a
t
x
6
6
8
0
m
m
;
2
9
9
.
9
3
7
k
N
m
a
t
x
2
2
2
7
m
m
6
3
1
.
6
2
8
k
N
a
t
x
6
6
8
0
m
m
;
5
6
4
.
4
0
6
k
N
a
t
x
7
2
4
m
m
5
.
8
6
m
m
a
t
x
0
m
m
Structural Design of Foundations 571
7
0
0
m
m
0.5d
(
d
/
2
+
9
0
0
) 0
.
5
d
1600 kN
700 mm
Corner
(d/2+900)
7
0
0
(d+400)
0.5d
(
d
/
2
+
9
0
0
)
0.5d
400
800 kN
Side
Critical sections in shear
(b1) (b2)
(c)
6000 mm 6000 mm 6000 mm 700 700
4 3 2 1
19400 mm
7
5
0
m
m
8
0
0
m
m
4 -20 mm dia bars @ 260 mm c/c
per meter length of the slab
4 legged shear stirrups
(d+400)
400 mm
Figure 12.C.19 Raft layout and column loads; (b1) critical section in shear; (b2) critical section in
shear; and (c) details of reinforcement along the longitudinal section (Example 12.7).
572 Foundation Design
y
5800 1800 2000 1000 10600 1600 2000 600
14000
5:4285 m
e
y
5:42855 0:4285 m
I
x
19:4 11:4
3
12
2395:16 m
4
I
y
11:4 19:4
3
12
6936:31 m
4
A 19:4 11:4 221:16 m
2
M
x
pe
y
14000 0:4285 6000 kNm 12:C:65
M
y
pe
x
14000 0:1714 2400 kNm 12:C:66
P
A
14000
221:16
63:302 kN=m
2
Soil pressure at different points is as follows
s
P
A
M
y
I
y
x
M
x
I
x
y 12:C:67
s 63:302
2400
6936:31
x
6000
2395:16
y 63:158 1:5269x 0:4745y
At corner A4
s
A4
63:158 0:346 9:7 2:505 5:7 80:93 BC of soil 80 kN=m
2
At corner C4
s
C4
63:158 0:346 9:72:505 5:7 74:225 kN=m
2
At corner A1
s
A1
63:1580:346 9:7 2:505 5:7 52:38 kN=m
2
At corner C1
s
C1
63:1580:346 9:72:505 5:7 45:67 kN=m
2
At corner B4
Structural Design of Foundations 573
s
B4
63:158 0:346 9:7 66:658 kN=m
2
At corner B1
s
B1
63:1580:346 9:7 59:943 kN=m
2
In the x direction, the raft is divided in three strips, that is three equivalent beams:
1. Beam AA with 3.2 m width and soil pressure of 80 kN=m
2
2. Beam BB with 5.0 m width and soil pressure of
80 66:65
2
73:32 kN=m
2
3. Beam CC with 5.0 m width and soil pressure of
66:65 52:38
2
59:52 kN=m
2
.
The bending moment is obtained by using a coefcient 1/10 and L as the center to center of
column distance
M M
wL
2
10
12:C:68
For strip AA
Maximum moment
80 6
2
10
288 kNm=m
For strip BB
Maximum moment
73:32 6
2
10
263:95 kNm=m
For strip CC
Maximum moment
59:52 6
2
10
214:272 kNm=m
For any strip in the y direction, take M
wL
2
8
since there is only a two-span equivalent beam.
For strip 44
Maximum moment
80 5
2
8
250 kNm=m
The depth of the raft is governed by two-way shear at one of the exterior columns. If the location
of critical shear is not obvious, it may be necessary to check all possible locations.
Shear strength of concrete, t
c
0:25
f
ck
p
0:25
15
p
0:97N=mm
2
For a corner column (say C1)
Perimeter b
o
2
d
2
900
d 1800 mm (Figure 12.C.19(b))
t
v
V
u
b
o
d
1:5 800 1000
d 1800d
0:97
1200000
d 1800d
0:97
)d
2
1800d1237113:40 0
574 Foundation Design
d
1800
1800
2
4 1 1237113:40
q
2 1
530:773 mm
For a corner column (say A2)
Perimeter b
o
2
d
2
900
d 400 2d 2200 mm
t
v
V
u
b
o
d
1:5 1600 1000
2d 2200d
0:97
d
2
1100d1237113:40 0 12:C:69
)d
1100
1100
2
4 1 1237113:40
q
2 1
690:811 mm
However, adopt an effective depth of 750 mm and overall depth of 800 mm
Reinforcement in the longitudinal direction is given by (considering a 1 m wide strip)
A
t
0:5
15
415
1
1
4:6 288 10
6
15 1000 750
2
s 2
4
3
5
1000 750 1109:51 mm
2
Use 20 mm bars, A
f
314:151 mm
2
Number of bars
1109:51
p
4
20
2
3:531 4 bars
Spacing of long bars
1000
p
4
20
2
1109:51
283:152 mm
Provide 420 mmFbars for reinforcement @260 mmc/c at top and bottomin both directions.
Minimum reinforcement in the slabs 0:12%
0:12
100
800 1000
960 mm
2
=m < 1109:51 mm
2
=m
Minimum steel governs in the remaining raft. Critical sections in shear.
WINBEF Solution:
WINBEF Input
Youngs modulus of soil, E
s
10
5
kN=m
2
, unit weight of soil, g
soil
20 kN=m
3
.
Structural Design of Foundations 575
Poissons ratio of soil, n
s
0:3.
Youngs modulus of concrete
E
f
5000
s
ck
p
5000
15
p
19364:92 N=mm
2
1:9364 10
7
kN=m
2
Bbreadth of foundation 1.0 m (considering a 1 m wide strip for design).
Moment of inertia of the concrete beam I
f
Bd
3
12
1:00:8
3
12
0:04267 m
4
.
Modulus of subgrade reaction, k
s
1
Bmm
0:65
E
s
B
4
E
f
I
f
12
q h i
E
s
1n
2
s
.
k
s
0:65
1000
10
5
1 0:3
2
10
5
1:0
4
1:9364 10
7
0:04267
12
s
59902
1000
59:902 kN=m
2
=mm:
Loading Data for WINBEF and Results
The loading data is shown in Figure 12.C.20.
Note: In the loading diagram, the load coming on to the beam is taken as maximum of A, B
and C strip loads as explained below (see Figure 12.C.19(a)).
The maximum load on strip 11: maximum of A1 (600 kN), B1 (800 kN) and C1 (800 kN),
which is 800 kN.
The maximum load on strip 22: maximum of A2 (1600 kN), B2 (1800 kN) and C2
(1000 kN), which is 1800 kN.
The maximum load on strip 33: maximum of A3 (2000 kN), B3 (2000 kN) and C3
(1200 kN), which is 2000 kN.
The maximumload on strip 44: maximumof A4 (600 kN), B4 (1000 kN) and C4 (1000 kN),
which is 1000 kN.
The details of deection, bending moment, shear force and bearing pressure are shown in
Figure 12.C.21. Please see also comments given in Section 12.C.2.
Figure 12.C.20 Loading data for WINBEF solution (Example 12.7).
576 Foundation Design
Example 12.8 Annular Raft Foundation
Design an annular (circular) raft for a circular tank whose outer diameter is 12 m and is
supported by a ring beam of 9 m diameter. The inner diameter of the annulus is 8 m. The
factored soil pressure under the raft is 80 kN/m
2
and a linearly varying soil pressure due to
18000 16000 14000 12000 10000 8000 6000 4000 2000 0
8.0
7.5
7.0
6.5
6.0
5.5
5.0
4.5
4.0
3.5
3.0
D
e
f
l
e
c
t
i
o
n
(
i
n
m
m
)
Length of the beam (in mm)
(a)
18000 16000 14000 12000 10000 8000 6000 4000 2000 0
1000
800
600
400
200
0
-200
-400
-600
B
e
n
d
i
n
g
m
o
m
e
n
t
(
i
n
k
N
-
m
)
Length of the beam (in mm)
(b)
(c)
18000 16000 14000 12000 10000 8000 6000 4000 2000 0
1000
800
600
400
200
0
-200
-400
-600
-800
-1000
S
h
e
a
r
f
o
r
c
e
(
i
n
k
N
)
Length of the beam (in mm)
Figure 12.C.21 (a) BEF deection diagram; (b) BEF bending moment diagram; (c) BEF shear force
diagram; and (d) BEF bearing pressure diagram (Example 12.7).
Structural Design of Foundations 577
You might also like
- CE199 2L 1Q1819 DC 1st TakeDocument10 pagesCE199 2L 1Q1819 DC 1st TakeJohn Michael Ramos100% (1)
- Gantry Girder DesignDocument5 pagesGantry Girder DesignHitesh ParmarNo ratings yet
- Designs Design of Deck Slab (S1) : 1) Data: Overall Thickness 600 MMDocument11 pagesDesigns Design of Deck Slab (S1) : 1) Data: Overall Thickness 600 MMskumaraneeeNo ratings yet
- Materials: Slab Bridge DesignDocument13 pagesMaterials: Slab Bridge DesignephremNo ratings yet
- Computation of Wind Force On Superstructure For PierDocument2 pagesComputation of Wind Force On Superstructure For Piervasu7900No ratings yet
- Biaxial Column DesignDocument4 pagesBiaxial Column DesignKwan Hau LeeNo ratings yet
- Jhansi-Khajuraho Toll Plaza Load CalculationDocument3 pagesJhansi-Khajuraho Toll Plaza Load CalculationVarun Tyagi100% (1)
- LECTURE2 Shear ConnectionDocument36 pagesLECTURE2 Shear ConnectionMahmoud A SalamaNo ratings yet
- ATRUCTDocument75 pagesATRUCTRadu StratilaNo ratings yet
- Design of Isolated Footing With Vertical Load Only - According To ACI 318M-99Document1 pageDesign of Isolated Footing With Vertical Load Only - According To ACI 318M-99mahmoud IbrahemNo ratings yet
- Calculation of Force and Bending Moment Due To Earth Pressure On A Cantilevering WallDocument1 pageCalculation of Force and Bending Moment Due To Earth Pressure On A Cantilevering Wallvasu7900No ratings yet
- Load Combination To Euro CodeDocument5 pagesLoad Combination To Euro CodeAbdul Hameed HatemNo ratings yet
- Sleeper Rev1Document23 pagesSleeper Rev1Yatendra TyagiNo ratings yet
- Etabs Steel Column Design Compair EtabsDocument10 pagesEtabs Steel Column Design Compair EtabsSumedha MayadunnaNo ratings yet
- Equivalent Static Force Procedure: - Example 1Document8 pagesEquivalent Static Force Procedure: - Example 1Omar Marghani SalmaNo ratings yet
- 14.Eng-Analysis of Sloshing Impact - MuthuvijayDocument16 pages14.Eng-Analysis of Sloshing Impact - MuthuvijayImpact JournalsNo ratings yet
- Design of Steel StructuresDocument8 pagesDesign of Steel StructuresSaiRamNo ratings yet
- Etabs Analysis ResultDocument2 pagesEtabs Analysis Resultmat_11410% (1)
- Summary of IS 1893 - Part 1Document6 pagesSummary of IS 1893 - Part 1prabhat_iitdNo ratings yet
- Openstaad - Reference ManualDocument206 pagesOpenstaad - Reference ManualAnay KarambelkarNo ratings yet
- Drop Panel CalculationDocument2 pagesDrop Panel Calculationsandiok86No ratings yet
- Composite BeamDocument7 pagesComposite Beamsaif.mullaNo ratings yet
- Combined Footing Design PDFDocument2 pagesCombined Footing Design PDFRenee100% (1)
- British Problem 5 PDFDocument5 pagesBritish Problem 5 PDFelixnzNo ratings yet
- Wall Footing DesignDocument102 pagesWall Footing DesignAUNGPS100% (1)
- Connection DesignDocument43 pagesConnection DesignAmul AmnajeNo ratings yet
- Effective Length of Stepped ColumnDocument1 pageEffective Length of Stepped ColumnSunil PulikkalNo ratings yet
- Design of PilecapDocument16 pagesDesign of PilecapMariappan .PNo ratings yet
- Design KN/M.: 388 RelnforcedDocument6 pagesDesign KN/M.: 388 RelnforcedSubhajyoti DasNo ratings yet
- Dynamic Wind ResponseDocument7 pagesDynamic Wind Responsejatin kalraNo ratings yet
- Shear Strength CalculationDocument4 pagesShear Strength CalculationAnil kumar RNo ratings yet
- Gantry Girders - Sections and Design - Construction - Civil Engineering PDFDocument12 pagesGantry Girders - Sections and Design - Construction - Civil Engineering PDFDinesh VermaNo ratings yet
- Design of Strip Footing at Grid C-C: U U, LimDocument3 pagesDesign of Strip Footing at Grid C-C: U U, LimSujal MaharjanNo ratings yet
- Design of Biaxial ColumnDocument5 pagesDesign of Biaxial ColumndineshNo ratings yet
- Wind LoadDocument6 pagesWind LoadRafaArdiSyahrezaNo ratings yet
- Arce306 Matrix STR Analy - +++Document66 pagesArce306 Matrix STR Analy - +++Vijay KumarNo ratings yet
- Loads and Forces Acting On Retaining Wall and Their CalculationsDocument6 pagesLoads and Forces Acting On Retaining Wall and Their CalculationsPrantik Adhar SamantaNo ratings yet
- STAAD Input File For BracketDocument2 pagesSTAAD Input File For BracketSaurabh Pandey0% (1)
- Staircase NewDocument10 pagesStaircase NewUmesh ChamaraNo ratings yet
- Gust Load Calc2Document36 pagesGust Load Calc2Anand.5No ratings yet
- Concrete Lectures Slab.2 Direct Design Method - PPT ExamplesDocument58 pagesConcrete Lectures Slab.2 Direct Design Method - PPT ExamplesUmer WaheedNo ratings yet
- Composite Slab Deck ACI318Document4 pagesComposite Slab Deck ACI318jflttwNo ratings yet
- Substructure Final PDFDocument34 pagesSubstructure Final PDFSushmit Sharma100% (1)
- Live Load CalculationDocument1 pageLive Load CalculationSuman NakarmiNo ratings yet
- Design of RC Building - ExerciseDocument6 pagesDesign of RC Building - Exercisesajeerala100% (1)
- Strap: 6.5.5.7 National Building Code of CanadaDocument32 pagesStrap: 6.5.5.7 National Building Code of CanadakanchanabalajiNo ratings yet
- IS875 (Part3) - Wind Loads On Buildings and Structures - IIT Kanpur-Part 1Document7 pagesIS875 (Part3) - Wind Loads On Buildings and Structures - IIT Kanpur-Part 1Adam Michael GreenNo ratings yet
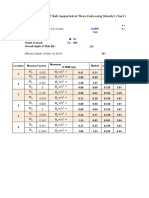
- Design of Slab Using Moody's ChartDocument2 pagesDesign of Slab Using Moody's ChartProlay MannaNo ratings yet
- Calculation of Pile Bearing CapacityDocument3 pagesCalculation of Pile Bearing Capacityhayder1974No ratings yet
- Circular Column CalculationDocument6 pagesCircular Column CalculationKwan Hau LeeNo ratings yet
- 00calculation Sheet For MMII Compressor Shed1Document31 pages00calculation Sheet For MMII Compressor Shed1clarkgaguiNo ratings yet
- Analysis of Cylindrical Water Tanks - Wind or EqDocument5 pagesAnalysis of Cylindrical Water Tanks - Wind or EqGiurcanas AndreiNo ratings yet
- Bar Bending Schedule For Pile Foundation With CalculationsDocument5 pagesBar Bending Schedule For Pile Foundation With CalculationsSuresh DevarajanNo ratings yet
- Steel DesignDocument8 pagesSteel DesignMUMANo ratings yet
- Rafter Foundation CalculationsDocument8 pagesRafter Foundation CalculationstsapoutshisNo ratings yet
- nov_2015_designDocument24 pagesnov_2015_designphearl keeri doyayagNo ratings yet
- Strap Footing PDFDocument7 pagesStrap Footing PDFMohammed Sumer100% (2)
- CL CL CL CL Class ASS ASS ASS ASS TEST - 2014 TEST - 2014 TEST - 2014 TEST - 2014 TEST - 2014Document6 pagesCL CL CL CL Class ASS ASS ASS ASS TEST - 2014 TEST - 2014 TEST - 2014 TEST - 2014 TEST - 2014Sri DNo ratings yet
- 507 39 Solutions-Instructor-manual Ch15 DRCSDocument38 pages507 39 Solutions-Instructor-manual Ch15 DRCSArun Goyal100% (2)
- 603 CompreDocument15 pages603 CompreCj SuarezNo ratings yet
- 7.2-ULS SUMMARYDocument1 page7.2-ULS SUMMARYramesh_madkatte1082No ratings yet
- 7.4 BASE SLAB DESIGNDocument8 pages7.4 BASE SLAB DESIGNramesh_madkatte1082No ratings yet
- 67651-6690-SHT 5 of 49Document1 page67651-6690-SHT 5 of 49ramesh_madkatte1082No ratings yet
- 2.1 Appendix 2.0 Wind LoadDocument2 pages2.1 Appendix 2.0 Wind Loadramesh_madkatte1082No ratings yet
- East 4Document31 pagesEast 4ramesh_madkatte1082No ratings yet
- Load CombinationsDocument10 pagesLoad Combinationsramesh_madkatte1082No ratings yet
- g.58 44ac9100-0000-L01-2116 - Pipe Span and Unit WeightDocument1 pageg.58 44ac9100-0000-L01-2116 - Pipe Span and Unit Weightramesh_madkatte1082No ratings yet
- TP 1U61A CV 1800 CAL 0030 R0 - Trestle Supp ReportDocument224 pagesTP 1U61A CV 1800 CAL 0030 R0 - Trestle Supp Reportramesh_madkatte1082No ratings yet
- Plan BracingDocument15 pagesPlan Bracingramesh_madkatte1082No ratings yet
- Is 456 - 2000Document114 pagesIs 456 - 2000ramesh_madkatte1082No ratings yet
- Job Information: KarmaDocument3 pagesJob Information: Karmaramesh_madkatte1082No ratings yet
- 4.6 Detailing Requirements For Flexure: 4.6.1 Tendon ProfileDocument10 pages4.6 Detailing Requirements For Flexure: 4.6.1 Tendon Profileramesh_madkatte1082No ratings yet
- Release 1Document1 pageRelease 1ramesh_madkatte1082No ratings yet
- Monorail 13Document26 pagesMonorail 13ramesh_madkatte1082No ratings yet
- Sr. No. Structure Remarks Squad Deliverab Le Input To Magcons Final StatusDocument1 pageSr. No. Structure Remarks Squad Deliverab Le Input To Magcons Final Statusramesh_madkatte1082No ratings yet
- TUBE 7 of BRANCH /011-DOH-UFWC-660105-001/B1 Line No Atta To Match Civil Pipe SupportDocument1 pageTUBE 7 of BRANCH /011-DOH-UFWC-660105-001/B1 Line No Atta To Match Civil Pipe Supportramesh_madkatte1082No ratings yet
- Comb WalkayDocument37 pagesComb Walkayramesh_madkatte1082No ratings yet
- Gopal PipingDocument17 pagesGopal Pipingramesh_madkatte1082No ratings yet
- 1.0 Remove The Intermediate Nodes From Staad File. 2.0 Change Bracing Pattern in Elevation On Grid A & BDocument5 pages1.0 Remove The Intermediate Nodes From Staad File. 2.0 Change Bracing Pattern in Elevation On Grid A & Bramesh_madkatte1082No ratings yet
- Analysis and Design of Slabs 1Document19 pagesAnalysis and Design of Slabs 1Adil Javed Chaudhary100% (5)