Distribution of Shear Stresses in Circular Shafts
Distribution of Shear Stresses in Circular Shafts
Uploaded by
Snehasish IsharCopyright:
Available Formats
Distribution of Shear Stresses in Circular Shafts
Distribution of Shear Stresses in Circular Shafts
Uploaded by
Snehasish IsharOriginal Description:
Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
Distribution of Shear Stresses in Circular Shafts
Distribution of Shear Stresses in Circular Shafts
Uploaded by
Snehasish IsharCopyright:
Available Formats
6/14/2014
NPTEL-IITR
LECTURE 19
Distribution of shear stresses in circular Shafts subjected to torsion :
The simple torsion equation is written as
This states that the shearing stress varies directly as the distance r' from the axis of the shaft and the following is the
stress distribution in the plane of cross section and also the complementary shearing stresses in an axial plane.
Hence the maximum strear stress occurs on the outer surface of the shaft where r = R
The value of maximum shearing stress in the solid circular shaft can be determined as
From the above relation, following conclusion can be drawn
(i) t maxm T
(ii) t maxm 1/d 3
Power Transmitted by a shaft:
In practical application, the diameter of the shaft must sometimes be calculated from the power which it is required to
transmit.
Given the power required to be transmitted, speed in rpm N' Torque T, the formula connecting
These quantities can be derived as follows
http://nptel.ac.in/courses/Webcourse-contents/IIT-ROORKEE/strength%20of%20materials/homepage.htm
1/5
6/14/2014
NPTEL-IITR
Torsional stiffness: The torsional stiffness k is defined as the torque per radian twist .
For a ductile material, the plastic flow begins first in the outer surface. For a material which is weaker in shear
longitudinally than transversely for instance a wooden shaft, with the fibres parallel to axis the first cracks will be
produced by the shearing stresses acting in the axial section and they will upper on the surface of the shaft in the
longitudinal direction.
In the case of a material which is weaker in tension than in shear. For instance a, circular shaft of cast iron or a
cylindrical piece of chalk a crack along a helix inclined at 450 to the axis of shaft often occurs.
Explanation: This is because of the fact that the state of pure shear is equivalent to a state of stress tension in one
direction and equal compression in perpendicular direction.
A rectangular element cut from the outer layer of a twisted shaft with sides at 450 to the axis will be subjected to such
stresses, the tensile stresses shown will produce a helical crack mentioned.
TORSION OF HOLLOW SHAFTS:
From the torsion of solid shafts of circular x section , it is seen that only the material at the outer surface of the shaft can
be stressed to the limit assigned as an allowable working stresses. All of the material within the shaft will work at a
lower stress and is not being used to full capacity. Thus, in these cases where the weight reduction is important, it is
advantageous to use hollow shafts. In discussing the torsion of hollow shafts the same assumptions will be made as in
the case of a solid shaft. The general torsion equation as we have applied in the case of torsion of solid shaft will hold
good
http://nptel.ac.in/courses/Webcourse-contents/IIT-ROORKEE/strength%20of%20materials/homepage.htm
2/5
6/14/2014
NPTEL-IITR
Hence by examining the equation (1) and (2) it may be seen that the t maxm in the case of hollow shaft is 6.6% larger then
in the case of a solid shaft having the same outside diameter.
Reduction in weight:
Considering a solid and hollow shafts of the same length 'l' and density 'r' with di = 1/2 Do
http://nptel.ac.in/courses/Webcourse-contents/IIT-ROORKEE/strength%20of%20materials/homepage.htm
3/5
6/14/2014
NPTEL-IITR
Hence the reduction in weight would be just 25%.
Illustrative Examples :
Problem 1
A stepped solid circular shaft is built in at its ends and subjected to an externally applied torque. T0 at the shoulder as
shown in the figure. Determine the angle of rotation q0 of the shoulder section where T0 is applied ?
Solution: This is a statically indeterminate system because the shaft is built in at both ends. All that we can find from the
statics is that the sum of two reactive torque TA and TB at the built in ends of the shafts must be equal to the applied
torque T0
Thus
TA+ TB = T0
------ (1)
[from static principles]
Where TA ,TB are the reactive torque at the built in ends A and B. wheeras T0 is the applied torque
From consideration of consistent deformation, we see that the angle of twist in each portion of the shaft must be same.
i.e
qa = q b = q 0
using the relation for angle of twist
N.B: Assuming modulus of rigidity G to be same for the two portions
So the defines the ratio of TA and TB
So by solving (1) & (2) we get
Non Uniform Torsion: The pure torsion refers to a torsion of a prismatic bar subjected to torques acting only at the ends.
While the non uniform torsion differs from pure torsion in a sense that the bar / shaft need not to be prismatic and the
http://nptel.ac.in/courses/Webcourse-contents/IIT-ROORKEE/strength%20of%20materials/homepage.htm
4/5
6/14/2014
NPTEL-IITR
applied torques may vary along the length.
Here the shaft is made up of two different segments of different diameters and having torques applied at several cross
sections. Each region of the bar between the applied loads betwe changes in cross section is in pure torsion, hence the
formula's derived earlier may be applied. Then form the internal torque, maximum shear stress and angle of rotation for
each region can be calculated from the relation
The total angle to twist of one end of the bar with respect to the other is obtained by summation using the formula
If either the torque or the cross section changes continuously along the axis of the bar, then the (summation can be
replaced by an integral sign ( ). i.e We will have to consider a differential element.
After considering the differential element, we can write
Substituting the expressions for Tx and Jx at a distance x from the end of the bar, and then integrating between the limits
0 to L, find the value of angle of twist may be determined.
Goto Home
http://nptel.ac.in/courses/Webcourse-contents/IIT-ROORKEE/strength%20of%20materials/homepage.htm
5/5
You might also like
- Third Periodical Test Science 8Document3 pagesThird Periodical Test Science 8Eileen Joy Noceda86% (69)
- Study of a reluctance magnetic gearbox for energy storage system applicationFrom EverandStudy of a reluctance magnetic gearbox for energy storage system applicationRating: 1 out of 5 stars1/5 (1)
- TorsionDocument18 pagesTorsionBhupesh GoyalNo ratings yet
- Design of ShaftsDocument5 pagesDesign of ShaftsDamianNo ratings yet
- TORSIONDocument34 pagesTORSIONisheanopamushongwiNo ratings yet
- Prof TVKB SOM Lecture 05 TorsionDocument57 pagesProf TVKB SOM Lecture 05 TorsiontvkbhanuprakashNo ratings yet
- Strength of MaterialDocument163 pagesStrength of MaterialJackKiaNo ratings yet
- Torsion in Circular Shafts and Thin-Walled TubesDocument6 pagesTorsion in Circular Shafts and Thin-Walled TubesRonald obasieNo ratings yet
- Derivation of Torsion TheoryDocument9 pagesDerivation of Torsion TheorysayerzNo ratings yet
- MET 214 - Lecture 4Document18 pagesMET 214 - Lecture 4zetseatNo ratings yet
- Lecture 6 - Torsion - 2015Document38 pagesLecture 6 - Torsion - 2015imranjamiNo ratings yet
- Lecture 3 TorsionDocument24 pagesLecture 3 TorsionJacob BaltazarNo ratings yet
- Members Subjected To Torsional LoadsDocument8 pagesMembers Subjected To Torsional LoadsRahulkumarchauhanNo ratings yet
- Som 200323142910Document9 pagesSom 200323142910Asante MandioperaNo ratings yet
- Topic 1 ShaftsDocument16 pagesTopic 1 Shaftsemmanuelkitich423No ratings yet
- BFC 20903 (Mechanics of Materials) Chapter 6: TorsionDocument26 pagesBFC 20903 (Mechanics of Materials) Chapter 6: TorsionHamierul MohamadNo ratings yet
- Torsion of Circular and Non-Circular ShaftsDocument13 pagesTorsion of Circular and Non-Circular ShaftsJohn CenaNo ratings yet
- ME374P1C2 - Exercise 5 - GuditoDocument49 pagesME374P1C2 - Exercise 5 - GuditoDessa GuditoNo ratings yet
- Chapter One Shaft DesignDocument71 pagesChapter One Shaft DesignhaymanotNo ratings yet
- Torsion SummaryDocument8 pagesTorsion SummaryVeronica AlvaradoNo ratings yet
- TORSIONDocument10 pagesTORSIONDarius MegamindNo ratings yet
- Ch.4 Torsion - NOTESDocument9 pagesCh.4 Torsion - NOTESDpt HtegnNo ratings yet
- Shaft N Coupling CP 10.Document29 pagesShaft N Coupling CP 10.Ashraf HaikalNo ratings yet
- Lecture 18 TorsionDocument6 pagesLecture 18 TorsionYash PaulNo ratings yet
- Beam TorsionDocument48 pagesBeam TorsionKory EstesNo ratings yet
- Torsion - Notes PDFDocument20 pagesTorsion - Notes PDFRichy Rahul AdithyaNo ratings yet
- ShaftDocument8 pagesShaftshaafiqueNo ratings yet
- Chapter 1 Shaft (Cont'd)Document47 pagesChapter 1 Shaft (Cont'd)Rayuth KEATNo ratings yet
- Torsion 1Document41 pagesTorsion 1Innah Rahman PutryNo ratings yet
- Torsion of Circular ShaftDocument12 pagesTorsion of Circular ShaftTare Er KshitijNo ratings yet
- SOM 2 Lecture NotesDocument163 pagesSOM 2 Lecture NotesKarthikKaru100% (1)
- TorsionDocument5 pagesTorsionbrightimefor223No ratings yet
- TorsionDocument20 pagesTorsionSathish SelvaNo ratings yet
- Chapter 7 - Torsion - Civil PDFDocument50 pagesChapter 7 - Torsion - Civil PDFcoded coderNo ratings yet
- SM II 1Document128 pagesSM II 1lokesh.ecb2658No ratings yet
- Combinedbending+Twisting of ShaftsDocument6 pagesCombinedbending+Twisting of ShaftsjoemonjacobNo ratings yet
- Dom 2022 PyqDocument12 pagesDom 2022 Pyqabhiram.mceNo ratings yet
- Torsion Civil EngineeringDocument43 pagesTorsion Civil Engineeringhaseebtkhan100% (1)
- Design of ShaftsDocument26 pagesDesign of ShaftsKrishna VamsiNo ratings yet
- ME403 Unit4 Pivots-and-CollarsDocument9 pagesME403 Unit4 Pivots-and-CollarsAaditya BhatnagarNo ratings yet
- TorsionDocument11 pagesTorsionayubNo ratings yet
- Deformable - Chapter 5Document21 pagesDeformable - Chapter 5Charlotte FerriolNo ratings yet
- ME 457 Experimental Solid Mechanics (Lab) Torsion Test: Solid and Hollow ShaftsDocument5 pagesME 457 Experimental Solid Mechanics (Lab) Torsion Test: Solid and Hollow Shaftsanon-735529100% (2)
- Torsional and Bending StressesDocument6 pagesTorsional and Bending StressesSajjadNo ratings yet
- Module 3 - TorsionDocument20 pagesModule 3 - TorsionArin ParkNo ratings yet
- Shear - Torsion - HandoutDocument10 pagesShear - Torsion - HandoutDirajen PMNo ratings yet
- Strength of Materials: Torsion of Circular ShaftDocument54 pagesStrength of Materials: Torsion of Circular ShaftsushilkumarNo ratings yet
- Shaft DesignDocument16 pagesShaft DesignOgunsina AdebayoNo ratings yet
- Chapter Four Torsion of Circular Shafts & Power TransmissionDocument48 pagesChapter Four Torsion of Circular Shafts & Power TransmissionYalem MesfinNo ratings yet
- Chapter3 Torsion FinalDocument78 pagesChapter3 Torsion FinalNaveen KumarNo ratings yet
- CH6 PDFDocument45 pagesCH6 PDFAguero AgueroNo ratings yet
- MODULE-2 Torsion and Bending StressesDocument14 pagesMODULE-2 Torsion and Bending Stressesrhea lee enriquezNo ratings yet
- Torsion LectureDocument91 pagesTorsion LectureY o oNo ratings yet
- Review On Design of AgitatorDocument4 pagesReview On Design of AgitatorAnonymous YWS7ndsiNo ratings yet
- Cylindrical Compression Helix Springs For Suspension SystemsFrom EverandCylindrical Compression Helix Springs For Suspension SystemsNo ratings yet
- Influence of System Parameters Using Fuse Protection of Regenerative DC DrivesFrom EverandInfluence of System Parameters Using Fuse Protection of Regenerative DC DrivesNo ratings yet
- A New System of Alternating Current Motors and Transformers and Other EssaysFrom EverandA New System of Alternating Current Motors and Transformers and Other EssaysRating: 5 out of 5 stars5/5 (1)
- Pd9211-Quality Concepts in Design PDFDocument2 pagesPd9211-Quality Concepts in Design PDFSnehasish IsharNo ratings yet
- Overview Contact in LS DYNADocument3 pagesOverview Contact in LS DYNASnehasish IsharNo ratings yet
- DoeDocument37 pagesDoeSnehasish IsharNo ratings yet
- Pd9211-Quality Concepts in Design-R8 PDFDocument2 pagesPd9211-Quality Concepts in Design-R8 PDFSnehasish IsharNo ratings yet
- Issues in Practical Problems, Part B: Turbulent FlowsDocument18 pagesIssues in Practical Problems, Part B: Turbulent FlowsSnehasish IsharNo ratings yet
- Closed Coiled Helical Springs Subjected To Axial LoadsDocument6 pagesClosed Coiled Helical Springs Subjected To Axial LoadsSnehasish Ishar100% (1)
- Catia Interview Questions and AnswersDocument110 pagesCatia Interview Questions and AnswersSnehasish IsharNo ratings yet
- Plasma Arc Welding and MachiningDocument8 pagesPlasma Arc Welding and MachiningSnehasish IsharNo ratings yet
- Catia Interview Questions and AnswersDocument110 pagesCatia Interview Questions and AnswersSnehasish IsharNo ratings yet
- KSPDocument23 pagesKSPNadia RamadaniNo ratings yet
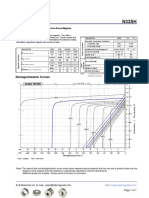
- N33SH Grade Neodymium Magnets DataDocument1 pageN33SH Grade Neodymium Magnets DataSteve HsuNo ratings yet
- Instant Ebooks Textbook Analytical Instrumentation Handbook Third Edition Jack Cazes Download All ChaptersDocument84 pagesInstant Ebooks Textbook Analytical Instrumentation Handbook Third Edition Jack Cazes Download All Chaptersbesmararamma100% (4)
- Linear Algebra ReviewDocument13 pagesLinear Algebra ReviewYoumna ShatilaNo ratings yet
- Assignment 1Document9 pagesAssignment 1Darling RajNo ratings yet
- Adas-Eu09 Notes CX BeamDocument33 pagesAdas-Eu09 Notes CX BeamMarius ConstantinNo ratings yet
- 2 Mark 16 MarkedcDocument18 pages2 Mark 16 MarkedccoolrajeeeNo ratings yet
- Grade 9 Test ItemDocument169 pagesGrade 9 Test ItemZhave RoncalesNo ratings yet
- 9 12 CosmologyDocument16 pages9 12 CosmologyMa. Soledad LedesmaNo ratings yet
- Sem QuestionsDocument11 pagesSem QuestionsRojaNo ratings yet
- Magnetism and Matter DPPDocument5 pagesMagnetism and Matter DPPChristopher NolanNo ratings yet
- Lecture # 2 Cement HydrationDocument142 pagesLecture # 2 Cement HydrationmsbNo ratings yet
- AMME3500 2013 Assignment 1Document5 pagesAMME3500 2013 Assignment 1JinDownloaderFree92No ratings yet
- Chemical Bond 1Document4 pagesChemical Bond 1NARENDRAN SNo ratings yet
- P1 Energy TransfersDocument12 pagesP1 Energy TransfersChris HarrisNo ratings yet
- 2-6 Phases of MatterDocument3 pages2-6 Phases of MatterRon Keanu BaronggoNo ratings yet
- BmeDocument36 pagesBmeOmkar ShetyeNo ratings yet
- N FSJPQ 3 K 16 Ut Ur Hja Dy UDocument14 pagesN FSJPQ 3 K 16 Ut Ur Hja Dy UVikas KumarNo ratings yet
- Physics DefinitionsDocument2 pagesPhysics DefinitionsElaine Neo Kai WenNo ratings yet
- Assignment On Straight LinesDocument7 pagesAssignment On Straight LinesSAMEER.SHARMANo ratings yet
- Measuring Techniques in Gas-Liquid and Gas-Liquid-Solid ReactorsDocument33 pagesMeasuring Techniques in Gas-Liquid and Gas-Liquid-Solid ReactorsEsteem 2021No ratings yet
- Aldehyde Ketone Carboxylic Acid Solved QuestionsDocument42 pagesAldehyde Ketone Carboxylic Acid Solved QuestionsTreasure SeekerNo ratings yet
- Phys Math PracticeDocument6 pagesPhys Math PracticeDenaiya Watton LeehNo ratings yet
- Title Pages ThesisDocument32 pagesTitle Pages ThesisQasim MuhammadNo ratings yet
- Mathematics B3D Dr. Helen J. Wilson Spring 2006Document77 pagesMathematics B3D Dr. Helen J. Wilson Spring 2006Roy VeseyNo ratings yet
- Main Fans: by Daniel Brassel (Cts - TPT)Document41 pagesMain Fans: by Daniel Brassel (Cts - TPT)Mahmoud MohammadNo ratings yet
- Complex Numbers For Planar Kinematics: R J B I A R B A R 1 JDocument15 pagesComplex Numbers For Planar Kinematics: R J B I A R B A R 1 JUmesh AhirraoNo ratings yet
- Questions On Unit I (Basics of Electromagnetics)Document2 pagesQuestions On Unit I (Basics of Electromagnetics)Thota Venkata Lakshmi Manasa 22120487101No ratings yet
- Rotary Screw CompressorsDocument22 pagesRotary Screw Compressorsganeshdhage100% (2)