Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd Logo
Upload

Welcome to Scribd!

  • 128 views

    Math Calculus Worksheet Chap 1: Limits and Their Properties Section

    Uploaded by

    Brille Adrian Fernando
    (1) This document provides strategies and techniques for evaluating limits, including direct substitution, recognizing indeterminate forms, using functions that agree except at one point, and using rationalizing and dividing out techniques. (2) It also presents the theorem that if two functions agree everywhere except at one point, and the limit of one of the functions exists as the variable approaches that point, then the limit of the other function is the same. (3) Examples are given to show calculating limits using these strategies, like rationalizing the numerator and applying the limit laws for fractions.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd

    Math Calculus Worksheet Chap 1: Limits and Their Properties Section

    Uploaded by

    Brille Adrian Fernando
    128 views1 page
    (1) This document provides strategies and techniques for evaluating limits, including direct substitution, recognizing indeterminate forms, using functions that agree except at one point, and using rationalizing and dividing out techniques. (2) It also presents the theorem that if two functions agree everywhere except at one point, and the limit of one of the functions exists as the variable approaches that point, then the limit of the other function is the same. (3) Examples are given to show calculating limits using these strategies, like rationalizing the numerator and applying the limit laws for fractions.

    Original Title

    Calculus Worksheet 1-6

    Copyright

    © © All Rights Reserved

    Available Formats

    PDF, TXT or read online from Scribd

    Did you find this document useful?

    Is this content inappropriate?

    (1) This document provides strategies and techniques for evaluating limits, including direct substitution, recognizing indeterminate forms, using functions that agree except at one point, and using rationalizing and dividing out techniques. (2) It also presents the theorem that if two functions agree everywhere except at one point, and the limit of one of the functions exists as the variable approaches that point, then the limit of the other function is the same. (3) Examples are given to show calculating limits using these strategies, like rationalizing the numerator and applying the limit laws for fractions.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    128 views1 page

    Math Calculus Worksheet Chap 1: Limits and Their Properties Section

    Uploaded by

    Brille Adrian Fernando
    (1) This document provides strategies and techniques for evaluating limits, including direct substitution, recognizing indeterminate forms, using functions that agree except at one point, and using rationalizing and dividing out techniques. (2) It also presents the theorem that if two functions agree everywhere except at one point, and the limit of one of the functions exists as the variable approaches that point, then the limit of the other function is the same. (3) Examples are given to show calculating limits using these strategies, like rationalizing the numerator and applying the limit laws for fractions.

    Copyright:

    © All Rights Reserved
    Download as PDF, TXT or read online from Scribd
    Download as pdf or txt
    of 1

    Math Calculus Worksheet Chap 1: Limits and Their Properties Section: Name:

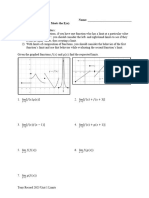
    33 A strategy for finding limits


    ! ! !!
    (2) lim!→! = _________
    (1) Direct Substitution: You should not think about the !!!
    substitution technique as function evaluation, but rather

    as the end result of our ________________________.


    (2) Indeterminate form: When evaluating a limit, if

    substitution results in the indeterminate form _________,


    ! ! !!!!
    then no conclusion can be made without doing more (3) lim!→! = _________
    ! ! !!!!!
    work. The limit

    _____________________________________________.
    (3) Theorem for functions that agree at all but one
    point: If the limit of f(x) as x approaches c cannot be
    evaluated by direct substitution, try to find a function g
    that agrees with f for all x other than x = c, and then do
    (!!∆!)! !! !!∆! !!!(! ! !!!!!)
    (4) lim∆!→! = __________
    ∆!
    ___________________________.

    (4) Use ___________________ and ________________


    techniques to find the limits.

    (5) Use ___________________ theorem to find the limit.

    (6) Use _______________________________________


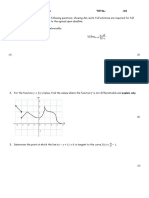
    to reinforce your conclusion. 37 Example: Rationalizing technique

    34 Example: Calculate the following limits. (1) lim!→!


    !!!!!
    = _________
    !
    ! ! !!!!!
    (1) lim!→!! = _________
    !!!

    !"# !
    (2) lim!→! = _________
    !

    35 Theorem: Limits of functions that agree at all but one


    point !!!!!
    (2) lim!→! = _________
    Let c be a real number and let f(x) = g(x) for all 𝑥 ≠ 𝑐 !!!
    in an open interval containing c. If the limit of g(x) as x
    approaches c exists, then the limit of f(x) also exists and

    lim!→! 𝑓(𝑥) = _______________________


    36 Example: Dividing out technique

    ! ! !!!! !!!! !
    (1) lim!→!! = _________ (3) lim!→! = _________
    !!! !

    Mr. Lin 6

    You might also like