Location via proxy:   [ UP ]  
[Report a bug]   [Manage cookies]                
Scribd Logo
Upload

Welcome to Scribd!

  • 13 views

    Tutorial 1

    Uploaded by

    Ridhwan Afiff
    The document discusses sampling and sampling distributions. It provides examples of population and sample parameters from studies. It also gives examples of calculating means, variances, standard deviations, and probabilities for samples from various populations.

    Copyright:

    © All Rights Reserved
    Download as DOCX, PDF, TXT or read online from Scribd

    Tutorial 1

    Uploaded by

    Ridhwan Afiff
    13 views3 pages
    The document discusses sampling and sampling distributions. It provides examples of population and sample parameters from studies. It also gives examples of calculating means, variances, standard deviations, and probabilities for samples from various populations.

    Original Title

    Tutorial 1 (1)

    Copyright

    © © All Rights Reserved

    Available Formats

    DOCX, PDF, TXT or read online from Scribd

    Did you find this document useful?

    Is this content inappropriate?

    The document discusses sampling and sampling distributions. It provides examples of population and sample parameters from studies. It also gives examples of calculating means, variances, standard deviations, and probabilities for samples from various populations.

    Copyright:

    © All Rights Reserved
    Download as DOCX, PDF, TXT or read online from Scribd
    Download as docx, pdf, or txt
    13 views3 pages

    Tutorial 1

    Uploaded by

    Ridhwan Afiff
    The document discusses sampling and sampling distributions. It provides examples of population and sample parameters from studies. It also gives examples of calculating means, variances, standard deviations, and probabilities for samples from various populations.

    Copyright:

    © All Rights Reserved
    Download as DOCX, PDF, TXT or read online from Scribd
    Download as docx, pdf, or txt
    of 3

    SMG2063 Statistics & Probability 2 1

    Tutorial 1: Sampling & Sampling Distributions

    1. A study of the dietary habits of 359, 264 Korean adolescents was conducted to find link
    between dietary habits and school performance. Identify the population and the sample
    in the study.

    2. Determine whether each number describes a population parameter or a sample statistic.


    Explain your reasoning.
    a) A survey of 100 Malaysian adults found that 52% think that the introduction of
    driverless cars will make roads less safe.
    b) At a college, 90% of the members of the Board of Trustees approved the contract of
    the new president.
    c) A survey of 727 small business owners found that 25% reported job openings they
    could not fill.

    3. The numbers of incorrect answers on a true-false competency test for a random sample
    of 15 students were recorded as follows: 2, 1, 3, 0, 1, 3, 6, 0, 3, 3, 5, 2, 1, 4, and 2. Find
    a) the mean
    b) the variance

    4. The grade-point averages of 20 college seniors selected at random from a graduating


    class are as follows:
    3.2 1.9 2.7 2.4 2.8 2.9 3.8 3.0 2.5 3.3
    1.8 2.5 3.7 2.8 2.0 3.2 2.3 2.1 2.5 1.9
    Calculate the standard deviation.

    5. The random variable X, representing the number of cherries in a cherry puff, has the
    following probability distribution:
    X 4 5 6 7
    P( X=x) 0.2 0.4 0.3 0.1

    (a) Find the mean μ and the variance σ 2 of X.


    (b) Find the mean μ X and the variance σ 2X of the mean X for random samples of 36
    cherry puffs.
    SMG2063 Statistics & Probability 2 2

    c) Find the probability that the average number of cherries in 36 cherry puffs will be
    less than 5.5.

    6. A random sample with n=16 is drawn from a population that is normally distributed
    with mean equal to 400 and standard deviation is 35.
    a) Find the mean and standard deviation of the sampling distribution of the sample
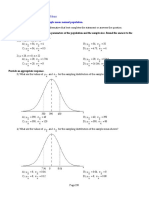
    mean X .
    b) Find the probability that X less than 410.
    c) Find the probability that X exceeds 415.
    d) Within what range of values does the X have 95.4% chance of falling.

    7. The average life of a bread-making machine is 7 years, with a standard deviation of 1


    year. Assuming that the lives of these machines follow approximately
    a normal distribution, find
    a) the probability that the mean life of a random sample of 9 such machines falls
    between 6.4 and 7.2 years;
    b) the value of x to the right of which 15% of the means computed from random
    samples of size 9 would fall.

    8. The distribution of a certain terrier dogs has a mean height of 72 centimetres and a
    standard deviation of 10 centimetres, whereas the distribution of heights of a certain
    breed of poodles has a mean height of 28 centimetres with a standard deviation of 5
    centimetres. Assuming that the sample means can be measured to any degree of
    accuracy, find the probability that the sample mean for a random sample of heights of
    64 terriers exceeds the sample mean for a random sample of heights of 100 poodles by
    at most 44.2 centimetres.

    9. Find

    a) P(T <3 .787 ), n=15

    b) P(−1. 476 <T <3. 365 ), n=6


    2
    c) P( χ α <32 .910 ), n=13
    SMG2063 Statistics & Probability 2 3

    2
    d) P(2 .733< χ α <17 . 535 ), n=9

    e)
    P( F v1 ,v2 <3.025),v 1=5,v 2=13

    10. Find

    a) k such that P(k <T <2 . 624 )=0 . 04 for a random sample of size 15 selected from
    a normal distribution .

    b) k such that P(−1. 440 <T <k )=0 .875 for a random sample of size 7 selected from
    a normal distribution .

    2
    11. For a chi-squared distribution find χ α such that
    2 2
    a) P( X > χ α )=0. 01 when v=21 ;
    2 2
    b) P( X > χ α )=0. 95 when v=6 ;

    c)
    P( X 2 < χ 2α )=0. 95 when v=6 ;
    2 2
    d) P( χ α < X <23 .209 )=0 . 015 when v=10 .

    12. Find the probability that a random sample of 25 observations, from a normal
    2
    population with variance σ =6 , will have a variance s
    2

    a) greater than 9.1


    b) between 3.462 and 10.745.
    Assume the sample variances to be continuous measurements.

    You might also like