Geometrical Optics
Geometrical Optics
Uploaded by
dildar123Copyright:
Available Formats
Geometrical Optics
Geometrical Optics
Uploaded by
dildar123Original Description:
Original Title
Copyright
Available Formats
Share this document
Did you find this document useful?
Is this content inappropriate?
Copyright:
Available Formats
Geometrical Optics
Geometrical Optics
Uploaded by
dildar123Copyright:
Available Formats
Geometric optics
Rays
rays point in direction that
light travels
crests occur on wave fronts
Ray Optics
A light ray can be defined by two co-ordinates:
x
in
, u
in
x
out
, u
out
its position, x
its slope, u
Optical axis
x
u
These parameters will change with distance and as the ray
propagates through optics.
Light rays
radiate
from a point
object in all
directions.
Reflection from a
plane mirror.
P is a virtual image.
Objects and images
Object Image
Rays from point
on object reflect
from mirror
Reflected rays
appear to come
from a point
Reflection
Specular reflection
(mirror)
Diffuse reflection
Reflection
The angle of incidence equals the angle of reflection
- u
i
= u
r
, where both angles are measured from the normal:
Note also, that all rays lie in the plane of incidence
u
i
u
r
u
i
u
r
x
E
i
u
i
E
r
u
r
u u
i r
=
Why?
This law is quite general; we supply a limited justification when
surface is a good conductor,
Electric field lines are perpendicular to the conducting surface.
The components of E parallel to the surface of the incident and reflected
wave must cancel!!
Law of reflection
u
u
Planar reflecting
surface (mirror)
u = angle of incidence
u = angle of reflection
Law of reflection
u u = '
Refraction
But it is really here!!
He sees the
fish here.
Index of Refraction
The wave incident on an interface can not only reflect, but it can also
propagate into the second material.
Claim the speed of an electromagnetic wave is different in matter than it is in
vacuum.
Recall, from Maxwells eqns in vacuum:
c =
1
0 0
c
n =
c
v
How are Maxwells eqns in matter different?
- c
0
c ,
0
Therefore, the speed of light in matter is related to the speed of light
in vacuum by:
1 n
0
> k =
c
c
~ where n = index of refraction of the material:
The index of refraction is frequency dependent: For example
n
blue
> n
red
Light inside a medium
Light interacts with particles inside materials
This slows down the light
The speed of light inside a medium is less than c
c
v
n
=
n is called the index of refraction
It is always bigger than 1
Material n
Air 1.000
Water 1.333
Glass 1.6
Diamond 2.419
Law of refraction
u
1
u
1
= angle of incidence
u
2
= angle of refraction
Snells Law
2 2 1 1
sin sin u u n n =
n
1
n
2
u
2
( n
2
> n
1
in diagram )
Refraction
Law of Refraction
(Snells Law)
n
1
sinu
1
= n
2
sinu
2
Refraction
Low to high index, light bends towards the normal line.
High to low index, light bends away from the normal line.
n
1
< n
2
n
1
sinu
1
= n
2
sinu
2
n
1
> n
2
n
1
n
2
Two simple effects
Object underwater appears
to be at a shallower depth
2
1
sin
n
n
C
= u
Total internal reflection
u
C
Total Internal Reflection (TIR)
sinu
c
= n
2
/ n
1
Critical Angle
Required:
n
1
> n
2
u
1
> u
c
TIR can only occur if
1. light goes from high index to low index AND
2. angle of incidence is greater than the critical angle
Total Internal Reflection (TIR)
n
1
n
2
u
c
water to air 1.33 1 48.8
glass to air 1.5 1 41.8
glass to water 1.5 1.33 62.5
Examples
Total Internal Reflection (TIR)
Total Internal Reflection
u
1
u
2
1 1
2
2
sin
sin
n
n
u
u =
What happens if the expression on the right
is bigger than one?
If the expression on the right is one or
bigger, than all the light gets reflected
1
2
sin
1
c
n
n
u
=
2
1
sin
c
n
n
u =
Called total internal reflection
Total internal reflection occurs only
when light moves into a medium of
lower index of refraction
Total internal reflection, Critical angle
1
2
sin
n
n
c
= |
1
u
n
2
n
1
> n
2
Incident
light
Transmitted
(refracted) light
Reflected
light
k
t
TIR
Evanescent wave
k
i
k
r
( a ) ( b )
( c )
Light wave travelling in a more dense medium strikes a less dense medium. Depending on
the incidence angle with respect to , which is determined by the ratio of the refractive
indices, the wave may be transmitted (refracted) or reflected. (a) (b) (c)
and total internal reflection (TIR).
2
|
1
|
c
|
90
2
= |
c
| | >
1
c
|
c
| | <
1
c
| | =
1
c
| | >
1
Critical angle
1
2
sin
n
n
c
= |
A light beam enters on the left in air, and we
want it to be reflected by the block of
glass. Do we have total internal reflection
in this case?
A) Yes
B) No
C) Insufficient Information
What if water gets
behind the prism?
Total Internal Reflection
Material n
Air 1.000
Water 1.333
Glass 1.6
Diamond 2.419
2
1
sin
c
n
n
u =
45
o
45
o
90
o
45
o
1 1
2
1
1
sin sin 38.7
1.6
C
n
n
u
| |
| |
= = =
|
|
\ .
\ .
1
2
1
sin
c
n
n
u
| |
=
|
\ .
Total Internal Reflection (TIR)
Application: Optical Fiber
clad
core
Optical Cable
Single Fiber
Optical Fiber
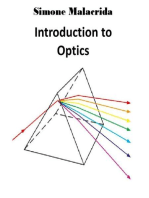
Prisms
down bends Light
sin sin
2 1
2 1
u u
u u
>
= n
down bends Light
sin sin
4 3
4 3
u u
u u
<
= n
u
1
u
2
Entering
u
3
u
4
Exiting
For air/glass interface, we
use n(air)=1, n(glass)=n
Prisms
u
1
u
2
u
3
u
4
Overall Deflection
At both deflections the amount of downward deflection
depends on n (and the prism apex angle, |).
Different colors will bend different amounts !
|
Dispersion
The amount by which light slows down can
depend on the wavelength of the light
The amount by which the light is bent can
depend on the wavelength of the light
1 1 2 2
sin sin n n u u =
For most materials, short wavelengths (blue)
are slowed more than long wavelengths (red)
White light (mixture of all
colors) gets split into its
constituent colors
c
v
n
=
Refraction & Dispersion
Light is bent and the resultant colors separate (dispersion).
Red is least refracted, violet most refracted.
Short wavelengths are bent
more than long wavelengths
rac
LIKE SO! In second rainbow
pattern is reversed
You might also like
- Shop Manual: 4D98E 4D106 S4D106Document204 pagesShop Manual: 4D98E 4D106 S4D106Julio Alvarado Morales100% (1)
- RefractionDocument42 pagesRefractionjanaNo ratings yet
- Reflection RefractionDocument20 pagesReflection RefractionCarl Daniel FandiñoNo ratings yet
- 02 Waves - LightDocument26 pages02 Waves - LightThamiso GolwelwangNo ratings yet
- 22 Refraction of LightDocument80 pages22 Refraction of LightoyamoopondoNo ratings yet
- Optics: The Science of Light Imaging: Phys 3616E, Winter 2017 Dr. Bassam AharmimDocument16 pagesOptics: The Science of Light Imaging: Phys 3616E, Winter 2017 Dr. Bassam AharmimjohnNo ratings yet
- Lecture 8Document52 pagesLecture 8Ashraf maghNo ratings yet
- 3.2 Refraction of LightDocument21 pages3.2 Refraction of Lighthassanoruko21No ratings yet
- Humara Nam Ravi HaiDocument8 pagesHumara Nam Ravi HaiRavi ShankarNo ratings yet
- 3.2 Refraction of LightDocument23 pages3.2 Refraction of LightakhonyaphaniceNo ratings yet
- Physics Holiday Homewory-Suchith Prabhu (Optics)Document75 pagesPhysics Holiday Homewory-Suchith Prabhu (Optics)Suchith PrabhuNo ratings yet
- Reflection, Refraction, and DiffractionDocument18 pagesReflection, Refraction, and DiffractionImmanuel Suman ShijuNo ratings yet
- Reflection, Refraction, and DiffractionDocument18 pagesReflection, Refraction, and Diffractionjaymart villartaNo ratings yet
- Ch2 Fundamentals of OpticsDocument47 pagesCh2 Fundamentals of OpticsAkram KhorsheidNo ratings yet
- OpticsDocument113 pagesOpticsGellirose S. BantayanNo ratings yet
- 03 Refraction of LightDocument22 pages03 Refraction of LightDonna100% (6)
- Class Notes - Total Internal ReflectionDocument3 pagesClass Notes - Total Internal ReflectionhorseridertomcatNo ratings yet
- Chatper 13Document84 pagesChatper 13Adellaine Lois GreyNo ratings yet
- Dutch Physicist Christian Huygens Captured This Propagation Mechanism MATHEMATICALLYDocument16 pagesDutch Physicist Christian Huygens Captured This Propagation Mechanism MATHEMATICALLYAijaz AhmedNo ratings yet
- OpticsDocument51 pagesOpticsUday Kiran ChNo ratings yet
- Nature of LightDocument34 pagesNature of Lightasalra05No ratings yet
- Chapter 22.1 4Document34 pagesChapter 22.1 4Richard Renz VillafuerteNo ratings yet
- OCN Unit - 1Document105 pagesOCN Unit - 1BinoStephenNo ratings yet
- Topic 4 - Reflection and RefractionDocument21 pagesTopic 4 - Reflection and RefractionAdexa PutraNo ratings yet
- PHYSICS 1E Module 13 Light and OpticsDocument46 pagesPHYSICS 1E Module 13 Light and OpticsClaire G. MagluyanNo ratings yet
- UP3 - 1.4. Total Internal Reflection - pg19-24Document6 pagesUP3 - 1.4. Total Internal Reflection - pg19-24mohmmadaliawadNo ratings yet
- PHY 104 (Optics Part)Document18 pagesPHY 104 (Optics Part)abdulkareemyusuf438No ratings yet
- Waves Completed Notes Part 2Document84 pagesWaves Completed Notes Part 2hijew86864No ratings yet
- Ray Theory of TransmissionDocument44 pagesRay Theory of TransmissionAkhil Arora100% (1)
- Ch-10 Light - Refraction - Notes PT 1Document5 pagesCh-10 Light - Refraction - Notes PT 1Raadhiya GogiaNo ratings yet
- Refr & Refl - Part1Document10 pagesRefr & Refl - Part1laptopemail270No ratings yet
- Chapter Three Light As Wave MotionDocument11 pagesChapter Three Light As Wave MotionSabrina NevilleLongbottom RitterNo ratings yet
- FertilizerDocument4 pagesFertilizerfafNo ratings yet
- Ray TheoryDocument13 pagesRay Theorygdmani008No ratings yet
- WP2 Light Waves (SDA) 1Document10 pagesWP2 Light Waves (SDA) 1botswanaboy9No ratings yet
- Fiber OpticsDocument79 pagesFiber OpticsSonakshi GuptaNo ratings yet
- Refraction of LightDocument26 pagesRefraction of Lightswagketa987No ratings yet
- Refraction 1Document8 pagesRefraction 1Sudharsan Gupta SudhirNo ratings yet
- Total Internal Reflection: Chapter 32: Light Reflection and RefractionDocument25 pagesTotal Internal Reflection: Chapter 32: Light Reflection and RefractionShahadat AwanNo ratings yet
- Analysis On FertilizersDocument22 pagesAnalysis On Fertilizersokesh27No ratings yet
- Fiber Optic CommunicationDocument181 pagesFiber Optic CommunicationECE HoD PSNCET100% (1)
- RefractionDocument15 pagesRefractionSudharsan Gupta Sudhir100% (1)
- Basic Physics 2 WEEK1 26022016Document36 pagesBasic Physics 2 WEEK1 26022016SERAPHINENo ratings yet
- Grade 8 ch25 refraction notes2024-25 (1)Document5 pagesGrade 8 ch25 refraction notes2024-25 (1)xlnc.arishaNo ratings yet
- Ray Optics - 2Document7 pagesRay Optics - 2jiluNo ratings yet
- Chemistry Project On Analysis of FertilizersDocument12 pagesChemistry Project On Analysis of FertilizersAtharva Maheshwari0% (1)
- RefractionDocument19 pagesRefractionYugandhar Veeramachaneni50% (2)
- Lecture 02 pptDocument96 pagesLecture 02 pptmequanintmeseret29No ratings yet
- Light - PhysicsDocument41 pagesLight - PhysicsChristopher TigranesNo ratings yet
- OpticsDocument84 pagesOpticsNoorzad Khalqi0% (1)
- 1 Reflection and RefractionDocument27 pages1 Reflection and RefractionAl John Paz FiedalanNo ratings yet
- CH12 PPT 1Document49 pagesCH12 PPT 1kaungforgameNo ratings yet
- Optics WorksheetDocument4 pagesOptics WorksheetAmelia RahmawatiNo ratings yet
- REFRACTION AT PLANE SURFACES Glass Block and Liquids A LevelDocument47 pagesREFRACTION AT PLANE SURFACES Glass Block and Liquids A Levelssenyonjotrevor01No ratings yet
- TE381Lecture2-Physics of LightDocument25 pagesTE381Lecture2-Physics of LightJeffrey MintahNo ratings yet
- Light Compare The Rival Theories of Light Held by ScientistsDocument12 pagesLight Compare The Rival Theories of Light Held by ScientistsPhilip MooreNo ratings yet
- Determination of Refraction IndexDocument3 pagesDetermination of Refraction IndexDrakalopNo ratings yet
- Reflection & RefractionDocument50 pagesReflection & Refractionannette.geesiawan1No ratings yet
- Maharshi Dayanand University, Rohtak: Paper DetailsDocument1 pageMaharshi Dayanand University, Rohtak: Paper Detailsdildar123No ratings yet
- Delhi Institute of Technology Management and Research: MondayDocument30 pagesDelhi Institute of Technology Management and Research: Mondaydildar123No ratings yet
- De 2Document1 pageDe 2dildar123No ratings yet
- ChalanDocument1 pageChalandildar123No ratings yet
- Curriculum Vitae: ObjectiveDocument3 pagesCurriculum Vitae: Objectivedildar123No ratings yet
- Month Wise Attendance & Sessional Test Marks (Semester-2)Document8 pagesMonth Wise Attendance & Sessional Test Marks (Semester-2)dildar123No ratings yet
- Student Detail Rollno Name Fathe Rname Mothe RnameDocument2 pagesStudent Detail Rollno Name Fathe Rname Mothe Rnamedildar123No ratings yet
- PCA LIST 3.5.2014 Updated On 14.5.14Document16 pagesPCA LIST 3.5.2014 Updated On 14.5.14dildar123No ratings yet
- Mechanical Actuation SystemDocument34 pagesMechanical Actuation Systemdildar123100% (2)
- Delhi Institute of Technology, Management & Research Firozpur Kalan, FaridabadDocument1 pageDelhi Institute of Technology, Management & Research Firozpur Kalan, Faridabaddildar123No ratings yet
- Umc PerformaDocument4 pagesUmc Performadildar123No ratings yet
- Subject: MM: Date: Sub. Code: Pass Marks: Atten D. (5/10) Practic Al File (5/10) Viva (10) Perform Ance (5 / 10) Total (25/50)Document9 pagesSubject: MM: Date: Sub. Code: Pass Marks: Atten D. (5/10) Practic Al File (5/10) Viva (10) Perform Ance (5 / 10) Total (25/50)dildar123No ratings yet
- Haryana State Board of Technical Education: Result Jan2014Document1 pageHaryana State Board of Technical Education: Result Jan2014dildar123No ratings yet
- Ditmr: Village - Firozepur Kalan, Faridabad BBA - II SemesterDocument18 pagesDitmr: Village - Firozepur Kalan, Faridabad BBA - II Semesterdildar123No ratings yet
- S No. Name of StudentDocument1 pageS No. Name of Studentdildar123No ratings yet
- 17 - Febuary - 2013Document3 pages17 - Febuary - 2013dildar123No ratings yet
- 2nd Asian Physics Olympiad: Taipei, Taiwan Experimental Competition Thursday, April 26, 2001Document7 pages2nd Asian Physics Olympiad: Taipei, Taiwan Experimental Competition Thursday, April 26, 2001dildar123No ratings yet
- Attendance - Record Bba IIDocument18 pagesAttendance - Record Bba IIdildar123No ratings yet
- Sessional 1Document40 pagesSessional 1dildar123No ratings yet
- Delhi Institute of Technology, Management & Research, Faridabad B.Tech Odd Semesters Internal Practical Examination Dec. 2012Document7 pagesDelhi Institute of Technology, Management & Research, Faridabad B.Tech Odd Semesters Internal Practical Examination Dec. 2012dildar123No ratings yet
- Operation and Maintenance Manual For WP2.1C/WP3.9C Marine DieselDocument28 pagesOperation and Maintenance Manual For WP2.1C/WP3.9C Marine DieselAbel GhellerNo ratings yet
- Workshop Manual Citea DAF Euro-5 EnglezaDocument466 pagesWorkshop Manual Citea DAF Euro-5 EnglezaIgor NistorNo ratings yet
- pp02 PDFDocument1 pagepp02 PDFc.a.g.p.No ratings yet
- Shared Environmental Responsibilities Kamil IssaDocument11 pagesShared Environmental Responsibilities Kamil IssaKamil IssaNo ratings yet
- Energy BalanceDocument2 pagesEnergy BalanceRodrigo Black SegurondoNo ratings yet
- GIL074 Domestic Condensing BoilersDocument4 pagesGIL074 Domestic Condensing BoilersIppiNo ratings yet
- Performance Test On 4-Stroke Petrol Engine With Pure OxygenDocument8 pagesPerformance Test On 4-Stroke Petrol Engine With Pure OxygenTJPRC PublicationsNo ratings yet
- Electricity PosterDocument2 pagesElectricity Posteraniketp11No ratings yet
- Information Desk Ajay SysconDocument8 pagesInformation Desk Ajay SysconSohel BangiNo ratings yet
- Oil CorrelationsDocument20 pagesOil CorrelationsGaby GalletaNo ratings yet
- No. 5, Jalan 22/6, Seksyen 22, 40300 Shah Alam, Selangor, MalaysiaDocument2 pagesNo. 5, Jalan 22/6, Seksyen 22, 40300 Shah Alam, Selangor, Malaysiavajiravel407No ratings yet
- Ventilation and Air Distribution Systems in Buildings: Hazim Bashir AwbiDocument4 pagesVentilation and Air Distribution Systems in Buildings: Hazim Bashir AwbiChristopher Plume De ChineNo ratings yet
- Ap 8012Document10 pagesAp 8012Shubham AdkeNo ratings yet
- 8 3a ChemistryOnEarth Booklet Mar21Document50 pages8 3a ChemistryOnEarth Booklet Mar21Bee Bee TanNo ratings yet
- Calculations rev.01Document1 pageCalculations rev.01adham.ahmedfouad2020No ratings yet
- NBC - Fire SafetyDocument200 pagesNBC - Fire Safetyvenkin84507488% (8)
- PLTD Suppa Equipment List For Relokasi Review Rev1Document112 pagesPLTD Suppa Equipment List For Relokasi Review Rev1'PeRsona NoUn GraTa'No ratings yet
- Power System AnalysisDocument25 pagesPower System AnalysisZuhaili Zawawi100% (1)
- VavDocument228 pagesVavWJHYYHNo ratings yet
- Ddms P.Obul Reddy Public School, Hyderabad Physics Xii Worksheet Potential and CapacitorDocument3 pagesDdms P.Obul Reddy Public School, Hyderabad Physics Xii Worksheet Potential and CapacitorPrasad PillarisettiNo ratings yet
- Inhouse Review Fault Analysys in Power SystemDocument2 pagesInhouse Review Fault Analysys in Power Systemmonique dianeNo ratings yet
- Polyethylene Terephthalate (PET)Document5 pagesPolyethylene Terephthalate (PET)angchengyi02No ratings yet
- AG312 Pin Diode PDFDocument18 pagesAG312 Pin Diode PDFtuwinnerNo ratings yet
- Water Hammer in Pumped Sewer MainsDocument45 pagesWater Hammer in Pumped Sewer MainsManikandanNo ratings yet
- 1nd Brochure2025Document11 pages1nd Brochure2025sanniverma2024No ratings yet
- ASCO Switching The Neutral ConductorDocument8 pagesASCO Switching The Neutral ConductorJOSE LUIS FALCON CHAVEZNo ratings yet
- Cover Letter FPCI - YanuarDocument1 pageCover Letter FPCI - YanuarYayan FadhillaNo ratings yet
- Design of CrystallizerDocument13 pagesDesign of CrystallizerABDULLAHI HAGAR FARAH HERSI STUDENTNo ratings yet
- Coolpack: Cycle SpecificationDocument2 pagesCoolpack: Cycle SpecificationHector Ariel HNNo ratings yet